
You are asked to “construct” an angle of
1.Using a compass and straightedge, copy an angle that appears to be close to
2.Using a compass and straightedge, construct two parallel lines and label one of the angles
3.Using a compass and straightedge, construct angles of
4.Using a protractor, draw an angle of
Answer
384.6k+ views
Hint: Here, in the given question, we need to construct an angle of
Complete answer:
1.Using a compass and straightedge, copy an angle that appears to be close to
Let us suppose we are given an angle of

Step 1: Draw a line segment AB of any length.
Step 2: Set the compass point on P and adjust it to any convenient width.
Step 3: Draw an arc across both sides of the angle, creating points J and K.
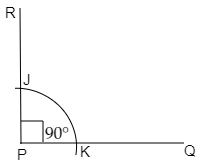
Step 4: Move the compass to A and draw a similar arc, crossing AB at M.
Step 5: Set the compass on K and set its width to J.
Step 6: Move the compass to M and draw an arc crossing the first, creating point L.
Step 7: Draw a ray AC from A through L.
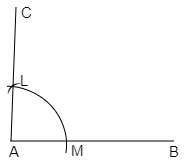
As you can see there is minute difference between both the angles. So from here we can conclude that this method is not appropriate for the construction of an angle.
2.Using a compass and straightedge, construct two parallel lines and label on of the angles
As we know angle between two parallel lines is
3.Using a compass and straightedge, construct angles of
Step 1: Draw a line segment PQ of any length.
Step 2: Using the compass with any width as radius and with P as a center, draw an arc that cuts the line segment PQ at a point and label it as R.
Step 3: With the same radius and with point R as the center, draw an arc that cuts the previous arc and name it as S.
Step 4: With S as a center and with the same radius draw another arc and name it as T.
Step 5: With the same radius and with S and T as center draw two arcs that cut each other and label it as U.
Step 6: Now join the points U and P. Now we can observe that
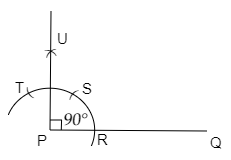
This method is appropriate for the construction of an angle of
4.Using a protractor, draw an angle of
Step 1: Draw a line segment AB of any measurement.
Step 2: Place the midpoint or center of the protractor on point A.
Step 3: Starting from
Step 4: Join the points C and A. Now,
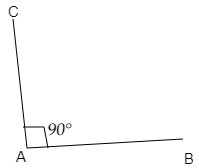
As here you can see we used only protractor to draw an angle of
Therefore, the correct option is 3.
Note:
Don’t construct any line segment without using a straightedge. Draw straight lines only by using a straightedge. Don’t use pen instead of pencil, because if any step gets wrong that can be corrected using an eraser. Don’t construct angles by just guessing the angle, use compass.
Complete answer:
1.Using a compass and straightedge, copy an angle that appears to be close to
Let us suppose we are given an angle of

Step 1: Draw a line segment AB of any length.
Step 2: Set the compass point on P and adjust it to any convenient width.
Step 3: Draw an arc across both sides of the angle, creating points J and K.

Step 4: Move the compass to A and draw a similar arc, crossing AB at M.
Step 5: Set the compass on K and set its width to J.
Step 6: Move the compass to M and draw an arc crossing the first, creating point L.
Step 7: Draw a ray AC from A through L.

As you can see there is minute difference between both the angles. So from here we can conclude that this method is not appropriate for the construction of an angle.
2.Using a compass and straightedge, construct two parallel lines and label on of the angles
As we know angle between two parallel lines is
3.Using a compass and straightedge, construct angles of
Step 1: Draw a line segment PQ of any length.
Step 2: Using the compass with any width as radius and with P as a center, draw an arc that cuts the line segment PQ at a point and label it as R.
Step 3: With the same radius and with point R as the center, draw an arc that cuts the previous arc and name it as S.
Step 4: With S as a center and with the same radius draw another arc and name it as T.
Step 5: With the same radius and with S and T as center draw two arcs that cut each other and label it as U.
Step 6: Now join the points U and P. Now we can observe that

This method is appropriate for the construction of an angle of
4.Using a protractor, draw an angle of
Step 1: Draw a line segment AB of any measurement.
Step 2: Place the midpoint or center of the protractor on point A.
Step 3: Starting from
Step 4: Join the points C and A. Now,

As here you can see we used only protractor to draw an angle of
Therefore, the correct option is 3.
Note:
Don’t construct any line segment without using a straightedge. Draw straight lines only by using a straightedge. Don’t use pen instead of pencil, because if any step gets wrong that can be corrected using an eraser. Don’t construct angles by just guessing the angle, use compass.
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Where did Netaji set up the INA headquarters A Yangon class 10 social studies CBSE

A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The British separated Burma Myanmar from India in 1935 class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What are the public facilities provided by the government? Also explain each facility




