
What is the zero error as shown in the figure?
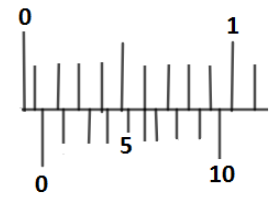
A.
B.
C.
D.

Answer
425.1k+ views
2 likes
Hint: In the given figure, we have a main scale which is
Complete step by step answer:
Since, the formula used to determine the zero error is given by
Zero error
Where,
Number of divisions on Vernier scale is
Unit of main scale division denoted as
So, least count is given as
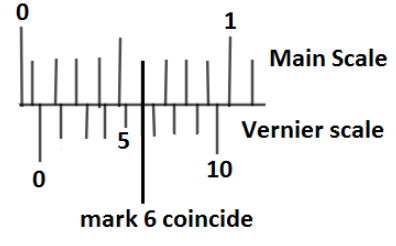
And,
Using the formula, Zero error
Zero error
Zero error
So, the zero error of the given scale is Zero error
Hence, the correct option is A.
Note: It should be remembered that, generally Vernier callipers are used to determine the radius or diameter of given circular bodies very accurately. If one needs to determine the zero error in suitable units we can use general conversion units as
Complete step by step answer:
Since, the formula used to determine the zero error is given by
Zero error
Where,
Number of divisions on Vernier scale is
Unit of main scale division denoted as
So, least count is given as

And,
Using the formula, Zero error
Zero error
Zero error
So, the zero error of the given scale is Zero error
Hence, the correct option is A.
Note: It should be remembered that, generally Vernier callipers are used to determine the radius or diameter of given circular bodies very accurately. If one needs to determine the zero error in suitable units we can use general conversion units as
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




