Revision Notes for CBSE Class 11 Maths Chapter 13 (Statistics) - Free PDF Download






FAQs on Statistics Class 11 Notes CBSE Maths Chapter 13 (Free PDF Download)
1. What are the properties of arithmetic mean?
The properties of the arithmetic mean are:
The AM does not depend on the change of scale or origin.
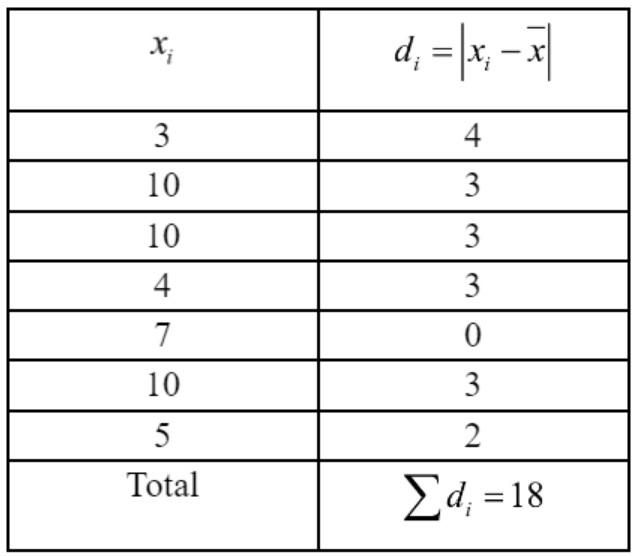
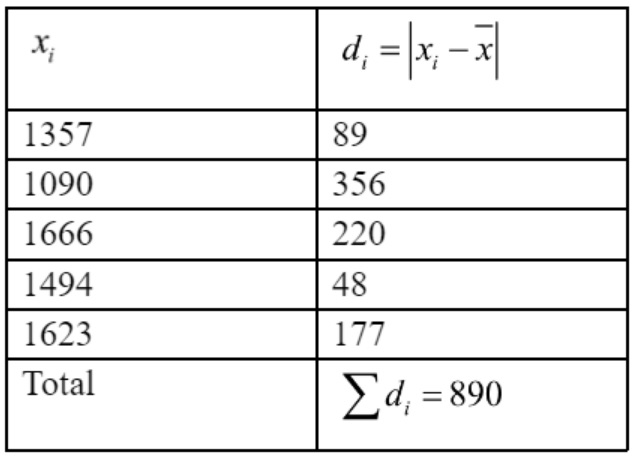
If you algebraically sum the deviations of a set of values from their arithmetic mean, it is equal to 0.
If you sum the squares of deviations of a set of values, its value is the least when it is taken about their arithmetic mean.
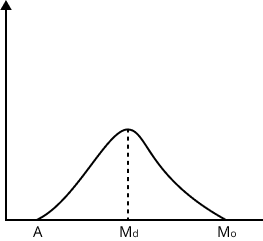
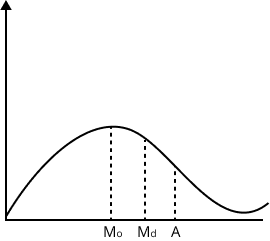
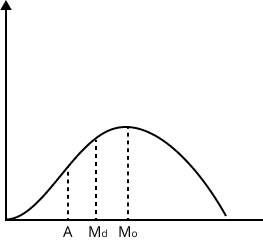
2. What is the median of a distribution?
When the variables are arranged either in ascending or descending order, then the middle variable's value gives the median for that distribution. It is the average position of the numbers in the set. Formulas for simple distribution median are:
If there are odd numbers (n) then median Md = (n + 1)/2 th term.
If there are even numbers then there are two medians given by n/2th term and n/2 + 1th term.
3. What points should I consider while downloading the PDF file of revision notes of Chapter 13 of Class 11 Maths?
The method that should be obeyed for downloading the PDF file of Chapter 13 of Class 11 Maths is:
Tap on the given CBSE Class 11 Chapter 13 .
The link will open the official webpage of Vedantu.
As the page appears, you will discover that the revision notes of Chapter 13 of Class 11 Maths are available on the website of Vedantu.
At the peak of the webpage of Vedantu, you will see the “Download PDF” option.
Tap that option.
The PDF file of the notes will be downloaded.
4. What do you mean by the term statistics? Name some terminologies associated with statistics.
The organization, collection, presentation, interpretation and analysis of the numerical data are termed statistics.The different keywords associated with statistics are:
Class limit – These are of two types, upper and lower limits. The limit of a class is defined as the end value of a class.
The interval of Class – It is defined as the difference between the upper and lower limit of a class.
Primary data – It is the data collected by the researcher himself.
Secondary data – It is the data not collected by the investigator but collected by another person.
5. Describe the following terms:
Cumulative frequency distribution
Discrete frequency distribution
Continuous frequency distribution
Cumulative frequency distribution – The adding of the frequency of the first class, second class, third class and so on, which results in the final frequency is known as the cumulative frequency. For this frequency distribution, class frequencies are required.
Discrete frequency distribution – In this type of frequency distribution, the presentation of data is such that the measurements of the units are clearly visible.
Continuous frequency distribution – The type of frequency distribution in which groups of classes are not measurable is known as continuous frequency distribution.
If you want to understand these concepts more accurately then go through the given link CBSE Class 11 Chapter 13 .This link will redirect you to the official website of Vedantu where you can access the content related to Chapter 12 Straight lines for free. Additionally, you can also download its PDF if you want to study offline.
6. What do you understand by the terms mean, median and mode?
(a) Mean – It is defined as the ratio of the sum of the values of items to the total quantity or amount of data. The types of arithmetic mean are:
Mean for unclassified data
Frequency distribution arithmetic mean
Mean for combination
Mean for classified data
Arithmetic mean for weights
(b) Median – The middle value of the data set when the given data is arranged in ascending or descending order is known as the median.
(c) Mode – It is the value of that point at which the data set is highly concentrated.
7. Name some different types of graphical representations of frequency distribution.
Bar diagrams – In this graphical representation, the data is represented as rectangular bars. The length of these bars shows the value of different classes.
Histogram – It is the graphical representation in which the x-axis is assigned for class intervals and the y-axis is assigned for frequencies. Long rectangular bars are used to represent data.
Frequency polygon – This representation is used for ungrouped frequency distribution.
Pie diagrams – The representation in which a circle is divided into sectors to represent data or classes and frequency is known as a pie diagram.