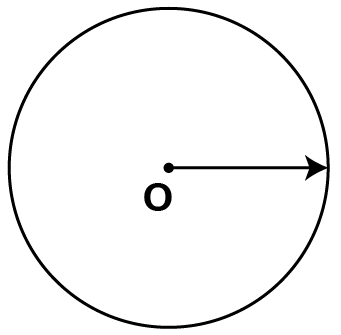
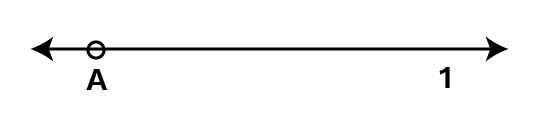
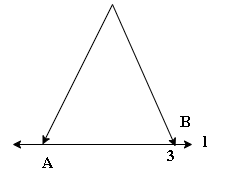
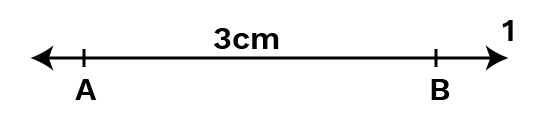
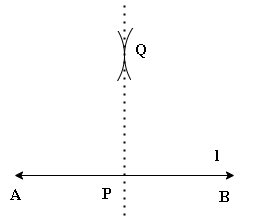
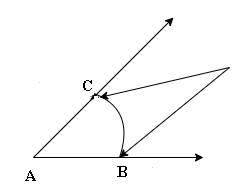
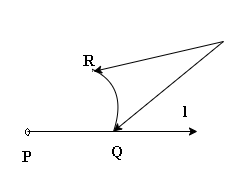
Revision Notes for CBSE Class 6 Maths Chapter 14 Practical Geometry - Free PDF Download
FAQs on Practical Geometry Class 6 Notes CBSE Maths Chapter 14 (Free PDF Download)
1. What is Practical Geometry, and why is it important for class 6 students?
Practical Geometry helps students apply geometric concepts to real-world problems, fostering a deeper understanding of shapes and measurements.
2. How can I use Vedantu’s Revision Notes to improve my understanding of Practical Geometry?
Vedantu’s notes provide simplified explanations, real-world examples, and key summaries, making it easier for you to grasp Practical Geometry concepts.
3. Are there specific tips for last-minute exam preparation in Practical Geometry?
Yes, the notes offer efficient tools and tips for last-minute exam preparation, ensuring you cover essential topics and boost your confidence.
4. Can revision notes by Vedantu help me solve practical problems related to geometry?
Absolutely! The notes prioritise practical examples, allowing you to apply geometric concepts to real-world scenarios and enhance your problem-solving skills.
5. How do Vedantu’s Revision Notes prioritise important topics in Practical Geometry?
The notes focus on key points and questions, helping you prioritise your study efforts and ensuring you're well-prepared for exams.