CBSE Physics Experiment Motion Under Gravity and Acceleration Due to Gravity

Scientists like Isaac Newton and Galileo Galilei investigated the link between the length and duration of one swing (the period) for a very long time. This research enabled them to derive an exact value for the gravitational acceleration, or 'g, with great accuracy. 

The motion under gravity and acceleration due to gravity is the acceleration brought on by the earth's gravitational pull. A person who jumps out of an aeroplane with a parachute falls freely for some time. The gravity of the earth causes him to accelerate.  However, Acceleration due to gravity depends upon the planet's radius, mass, and gravitational constant.

In this experiment, we will replicate their experiment and choose a suitable value for "g." Lastly, it will be measured and compared to the reference value of 9.806 m/s2. The experiment uses the basic pendulum experiment to determine an approximation of 'g' (gravity). We investigate several other factors, such as displacement, angle, damping, and bob mass. 

Table of Content

• Aim

• Observations

• Results

• Conclusion

Aim

This study aims to calculate the gravitational constant, 9.81 m/s2, and to explain the results' uncertainty.

Theory

A straightforward pendulum is a weight, the bob, suspended from and fastened to a non-elastic length (L) string with a light mass that permits a straightforward back-and-forth swinging motion. When the bob swings back and forth in an irregular pattern, it is said to be oscillating; this motion is also known as simple harmonic motion. The equilibrium transition from point A to point B is an oscillation. The specific swinging motions of the pendulum are propelled by the conversion of kinetic to potential energy. The period T is the length of time required to accomplish one oscillation. The maximum distance the mass can be moved away from its equilibrium position is known as the oscillation's amplitude.

\[{\rm{T = 2\pi }}\sqrt {\dfrac{{\rm{L}}}{{\rm{g}}}} \]

This is possible due to the equation's relationship between the period of a simple pendulum and the acceleration brought on by gravity.

where T = period, L is the pendulum's length, and g is its gravitational acceleration.

Apparatus Required

• Protractor

• String 

• Stopwatch

• Tape

• Ruler

• Mass (10 gm)

Procedure

• A pendulum bob should be attached to the string.

• Measure the pendulum arm's length using the meter ruler.

• Determine the length of the pendulum arm's square root.

• By pulling the pendulum bob back with your hand, you can cause it to move slightly out of equilibrium.

• When the pendulum bob is released, it will swing freely; at this point, simultaneously click "START" on the stopwatch.

• Click "STOP" on the stopwatch after 10 full oscillations, note the supplied time t, and get the periodic time T by dividing the total time by 10.

• Repeat the first three steps a final two times, recording the values as T1, T2, and T3, respectively.

• Record the average period's value next to its corresponding pendulum bob mass.

• Repeat the same data collection procedures four times, varying only the horizontal displacement and 5 cm of the pendulum arm's length.

• Make a graph showing the length of the pendulum arm squared against the average periodic time.

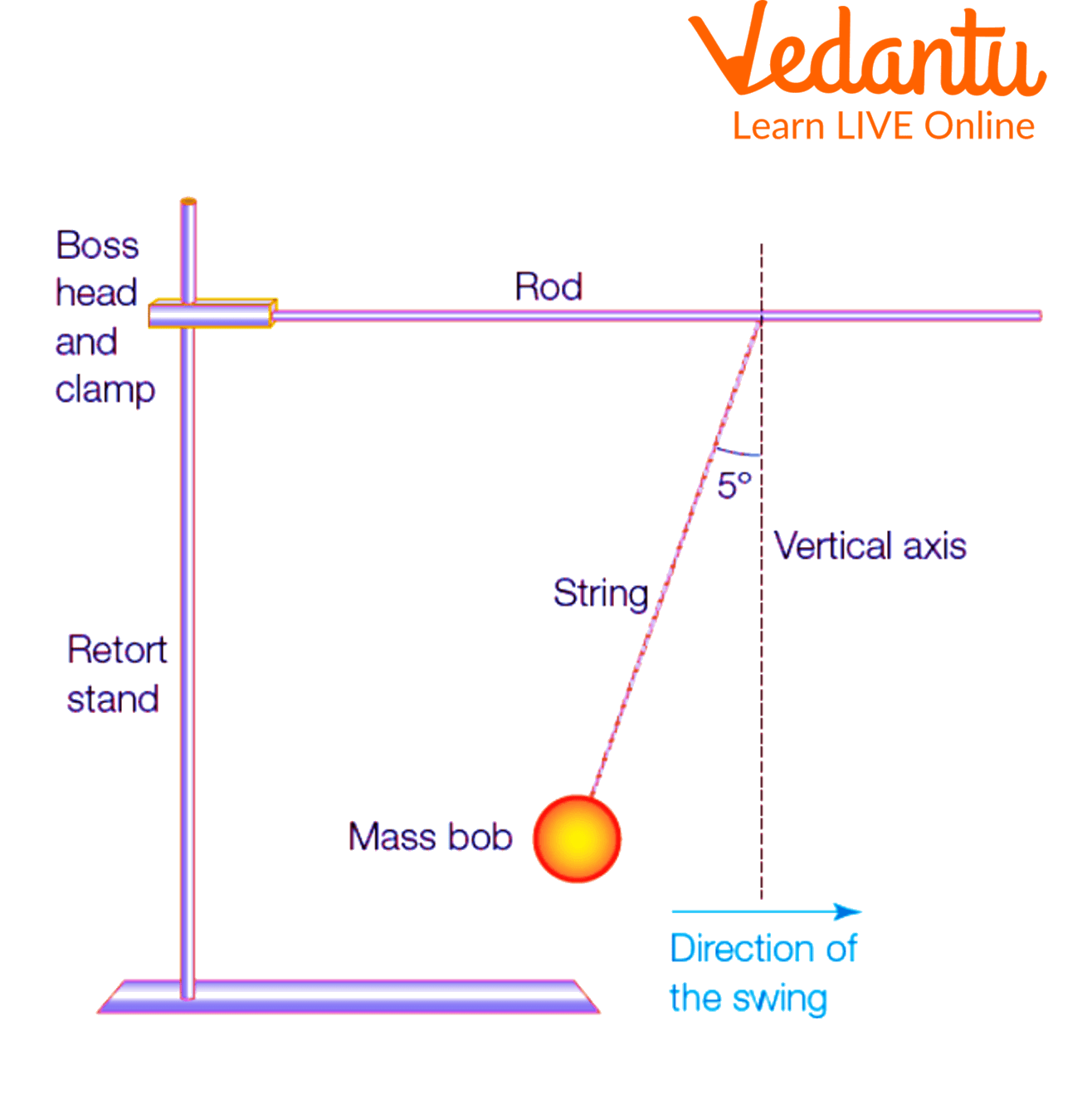
The Experimental Set-up

Observations

where\[{\rm{T = }}\dfrac{{\rm{t}}}{{{\rm{10}}}}\] and \[{\rm{g = }}\dfrac{{{\rm{4}}{{\rm{\pi }}^{\rm{2}}}{\rm{L}}}}{{{{\rm{T}}^{\rm{2}}}}}\]

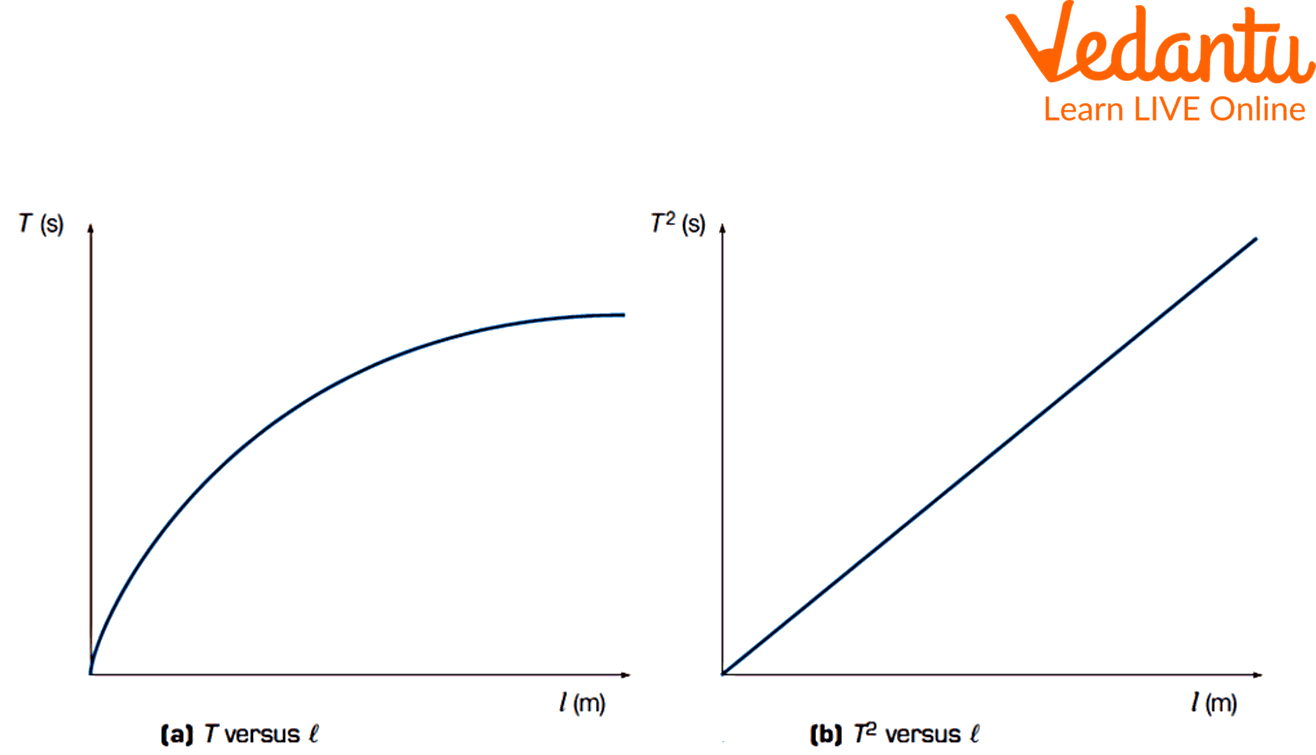
Graphical Outcomes of the L-T and L-T2 Graphs

Results

The slope is equivalent: 

According to the experimental data and graph. The experimental value of the acceleration caused by gravity: 

Precautions

• Line up the eye with a stationary object to ensure precise timing

• Reliable stop clock (decimal seconds)

• Using an average of two readings to account for human error

Lab Manual Questions

1. Find at what amplitude of oscillation, the time period begins to vary.

Ans. The amplitude is independent of the period. The more amplitude, the more the distance to be covered, but the faster it will do so. The period will not change because of the interaction between distance and speed.

2. How can you determine the value of 'g', acceleration due to gravity, from the T 2  vs L graph? 

Ans. The measured value of g is determined by the slope of the T2 vs. l graph of a pendulum. The equation\[{\rm{T = 2\pi }}\sqrt {\dfrac{{\rm{L}}}{{\rm{g}}}} \] determines the simple pendulum's time period T.

3. Does the time period depend on the material (density) of the pendulum bob? If yes, see the order in which the change occurs.

Ans. The length of the string solely influences a pendulum's period and not by the mass of the ball. The period of two pendulums that have the same length but have different masses will be the same. Two pendulums of various lengths will oscillate at different rates; the pendulum with the longer string will do so.

4. Does a heavier pendulum bob change the pendulum's period?

Ans. If we use a heavier bob instead of a lighter one the time period of the pendulum remains unchanged because it doesn’t depend upon the mass of the bob. It depends upon the length of the pendulum.

Viva Questions

1. Where does gravity's acceleration reach its maximum?

Ans. The square of the distance between the center and the surface determines the inverse proportionality of the acceleration caused by gravity. The equator is farther from the center than the poles are. Therefore, gravity's acceleration is greater at the poles.

2. Why can't aeroplanes be used to travel to the stars?

Ans. The minimum velocity needed to escape the earth’s gravitational pull is the escape velocity. At this speed, aeroplanes cannot fly.

3. Can a metallic wire be used in place of the thread?

Ans. Because the wire is rigid, the answer is no. 

4. What does restoring force mean?

Ans. The force that pushes a vibrating body toward the mean position or a force which tries to restore the equilibrium of the pendulum.

5. At the equilibrium (mean) position, what is the net force acting on the bob?

Ans. Zero, as the tension (T) in the string completely balances the bob's weight at its average position.

6. What is equilibrium?

Ans. When a body's linear and angular accelerations are both zero, or when the net force acting on the body is zero, it is considered to be in equilibrium.

7. Can the bob be replaced with a cricket ball?

Ans. No, a simple pendulum must have bob as small as feasible by definition.

8. Where is the pendulum's length measured?

Ans. The distance between the bob's center of gravity to the point of suspension is the answer.

9. Give the following definitions: Amplitude, Vibration or oscillation, Frequency, and Time. 

Ans. The greatest departure from the mean (equilibrium) position is referred to as amplitude. 

Oscillation: the transition between two extreme positions followed by a return to the first.

The number of vibrations per second is known as frequency.

Time is the amount of time needed for one vibration.

10. What is simple harmonic motion?

Ans. It is a motion of the body in which the restoring force acting on the body is directly proportional to its displacement from its mean position. Still, the direction of the force is opposite to that of displacement.  

Practical Based Questions

1. The value of the acceleration caused by gravity on the earth's surface at sea level is

1. 4.9 m/s2

2. 6 m/s2

3. 8 m/s2

4. 9.8 m/s2
   
   

Ans. D) 9.8 m/s2

2. A wooden ball and an iron ball with the same radius are both released into space at the same height (h). The time it took them both to get to the ground is either:

1. Unequal 

2. Exactly equal 

3. About equal

4. Zero

Ans. B) Exactly equal

3. What is the value of gravity's acceleration in polar regions?

1. Same as at the equator

2. More than at the equator

3. Less than at the equator

4. Zero

Ans. B) More than at the equator

4.  What is an object's weight on the moon's surface equals

1. 1/2 th of the object's weight

2. 1/4th of the object's weight

3. 1/6th of the object's weight 

4. 1/8th of the object's weight

Ans. C) 1/6th of the weight 

5. The period of ascension from the point of projection for an object thrown vertically upward will be

1. Less the Time of descent

2. Greater the Time of descent

3. Equal to the Time of descent

4. None of the above

Ans. C) Equal to the Time of descent

6. When a body is accelerated, the force that maintains it from rotating is

1. Centripetal force

2. Magnetic force

3. Electrostatic force

4. Force of gravitation

Ans. A) Centripetal force

7. The reaction force is referred to as when it is applied to a surface in a direction perpendicular to the surface and is created by the expulsion of mass in one direction.

1. Weight

2. Pressure

3. Centripetal force

4. Thrust

Ans. D) thrust

8. The gravitational force between two objects will decrease as their separation grows if:

1. Decrease

2. Increase

3. Remain the same

4. None of the above

Ans. B) Increase

9. In a lift, a straightforward pendulum is kept swinging. If the lift descends at a constant speed, the simple pendulum's periodic period will change: 

1. Will increase

2. Remains unchanged

3. Will lessen

4. Shall be 0

Ans.  B)  Remains unchanged

10. The simple pendulum's string tension is at its highest when the bob is: 

A) In an extreme situation

B) Position that lies halfway between the average and the extreme

C) At the average position

D) Begins oscillating with extremely high amplitudes

Ans. C) At the average position

Conclusion

A basic pendulum is a mechanical device that consists of a thin, inextensible string and a small, massed bob that is designed to oscillate between the left and right extremes of its mean position. The motion can be categorized as simple harmonic motion if the bob's displacement is minimal relative to the string's length or if the displacement angle is. The time period of oscillation of the simple pendulum depends upon its length and acceleration due to gravity. And by manipulating this relation we experimentally calculated the value of acceleration due to gravity.