CBSE Class 5 Maths Worksheet Chapter 3 How Many Squares - Download Free PDF
The chapter How Many Squares of Class 5 Maths is dedicated to fundamental concepts on what a square shape is made up of. A square is a shape that has four sides, and its key feature is that all the sides have equal dimensions. Learning squares by counting how many squares are represented in a given matrix of squares is an interesting way of teaching squares to students where they get to know the different ways to make a square shape.
How Many Squares Class 5 Worksheets will help students learn how to differentiate between sizes and shapes and also measure them to identify which is smaller and which is bigger. Students also learn definitions of various terms like perimeter, area of a regular shape, and area of an irregular shape. Through many activities like counting the number of complete squares, and counting the number of squares that are more than half-filled or less than half-filled, students are able to solidify their conceptual learnings.
At the end of this chapter, students would be able to modify basic shapes to create different tiling figures and shapes. With the Class 5 Maths Chapter 3 worksheet with answers, students will get enough practice questions to be able to solve any type of question on this topic.
Access Worksheet for Class 5 Maths Chapter 3 - How Many Squares?
1. In the figure below, each square is of side 1 cm. What is the area of the stamp?
Stamp
2. How many squares of one centimetre side does the figure cover?

Cover Figure
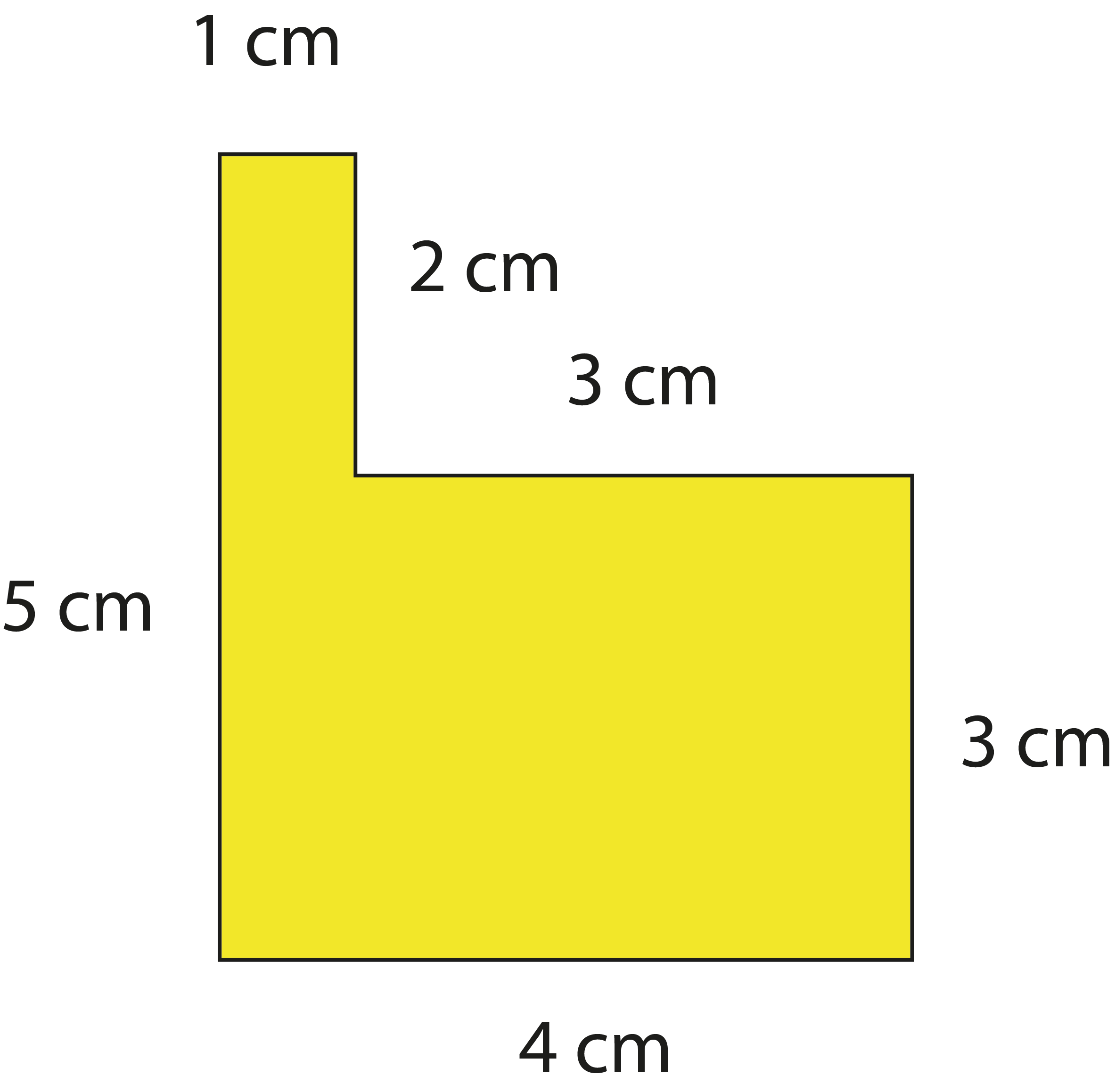
3. Find the perimeter of the given figure?
4. In the figure below, each square is of side 1 cm. Find the area of the shapes given below.
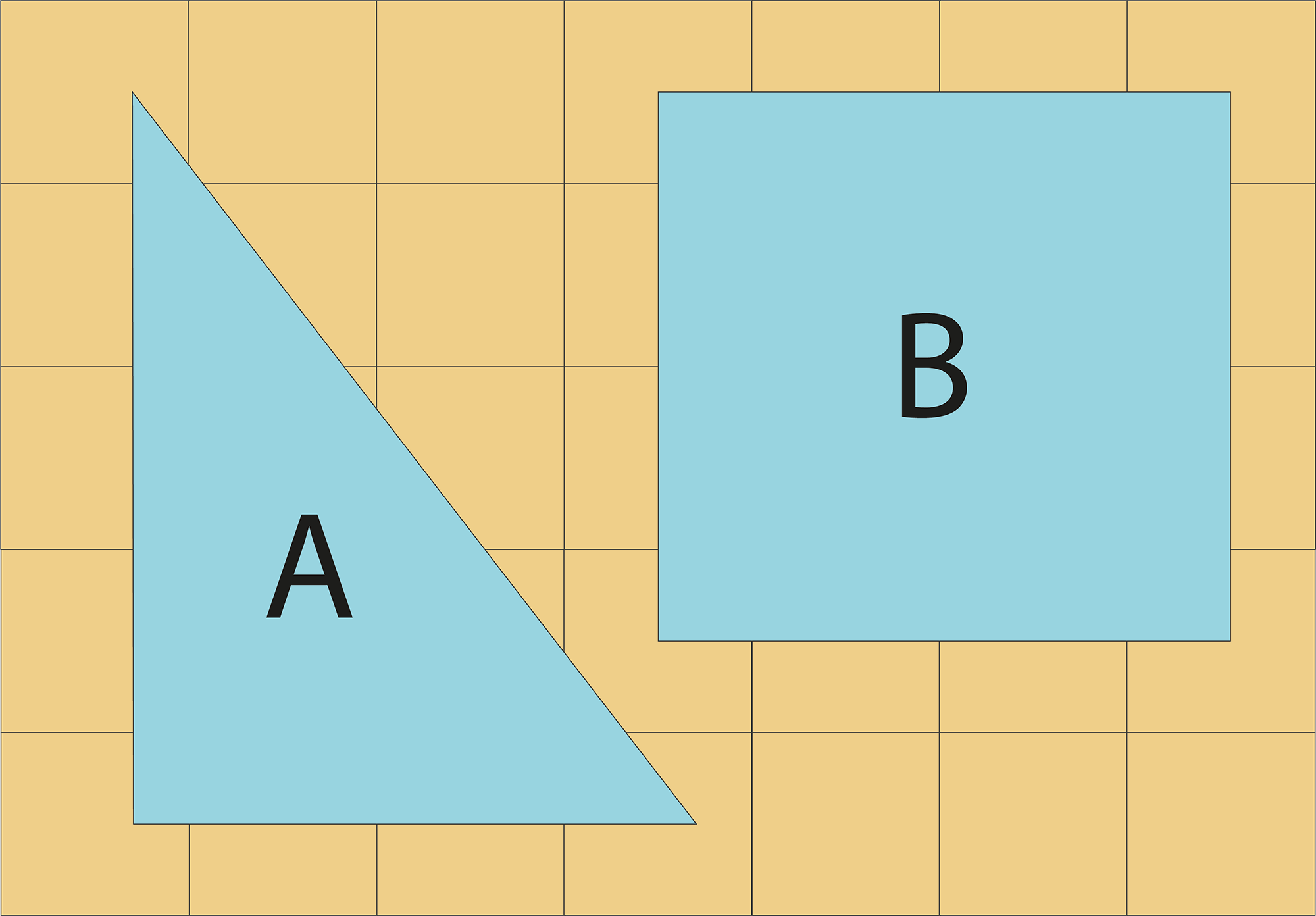
5. The figure below shows two halves of two different rectangles. One is of orange in colour, and the other is of green in colour. Find the area of the two rectangles. Also, find the area of the red triangle.
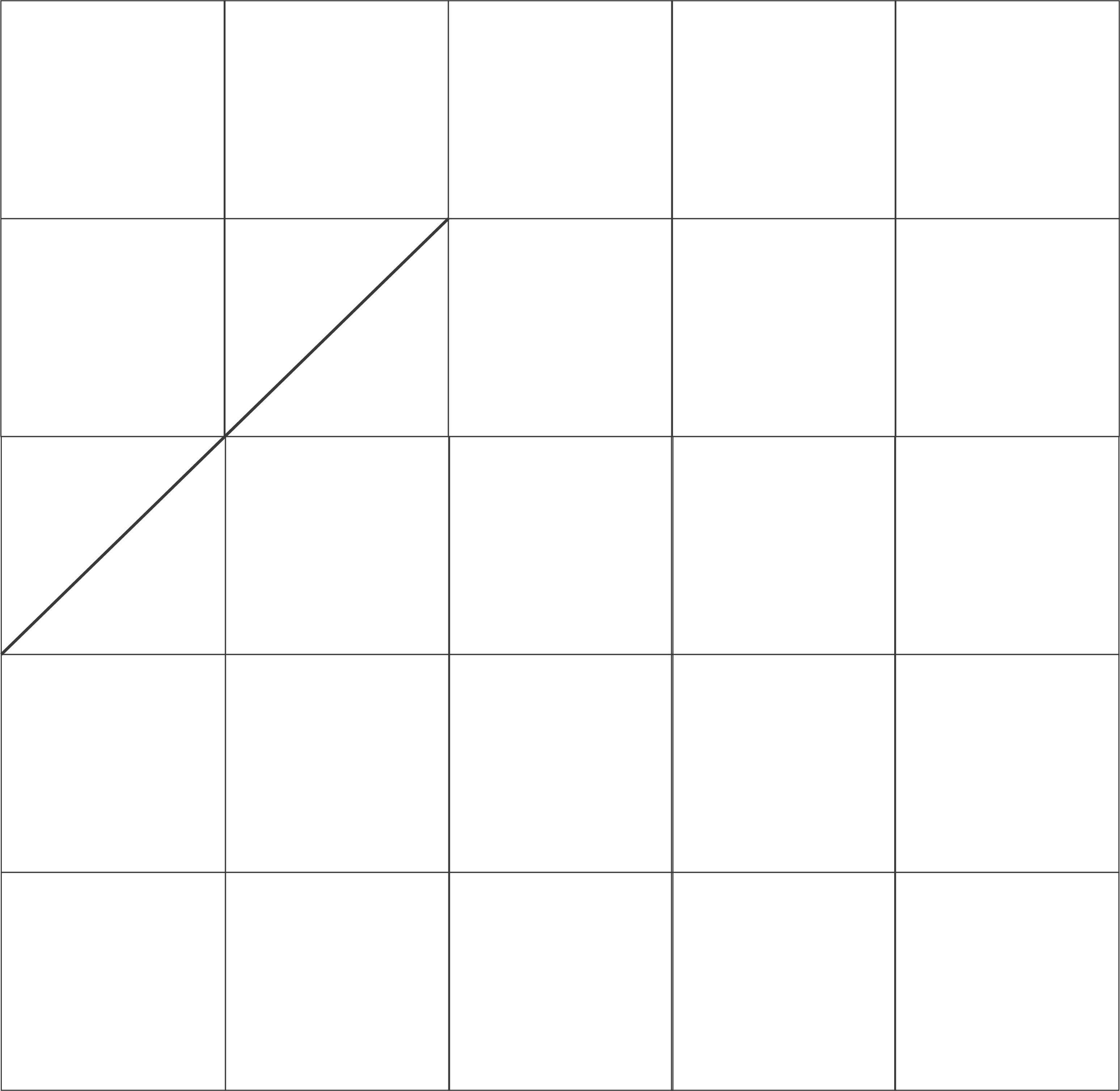
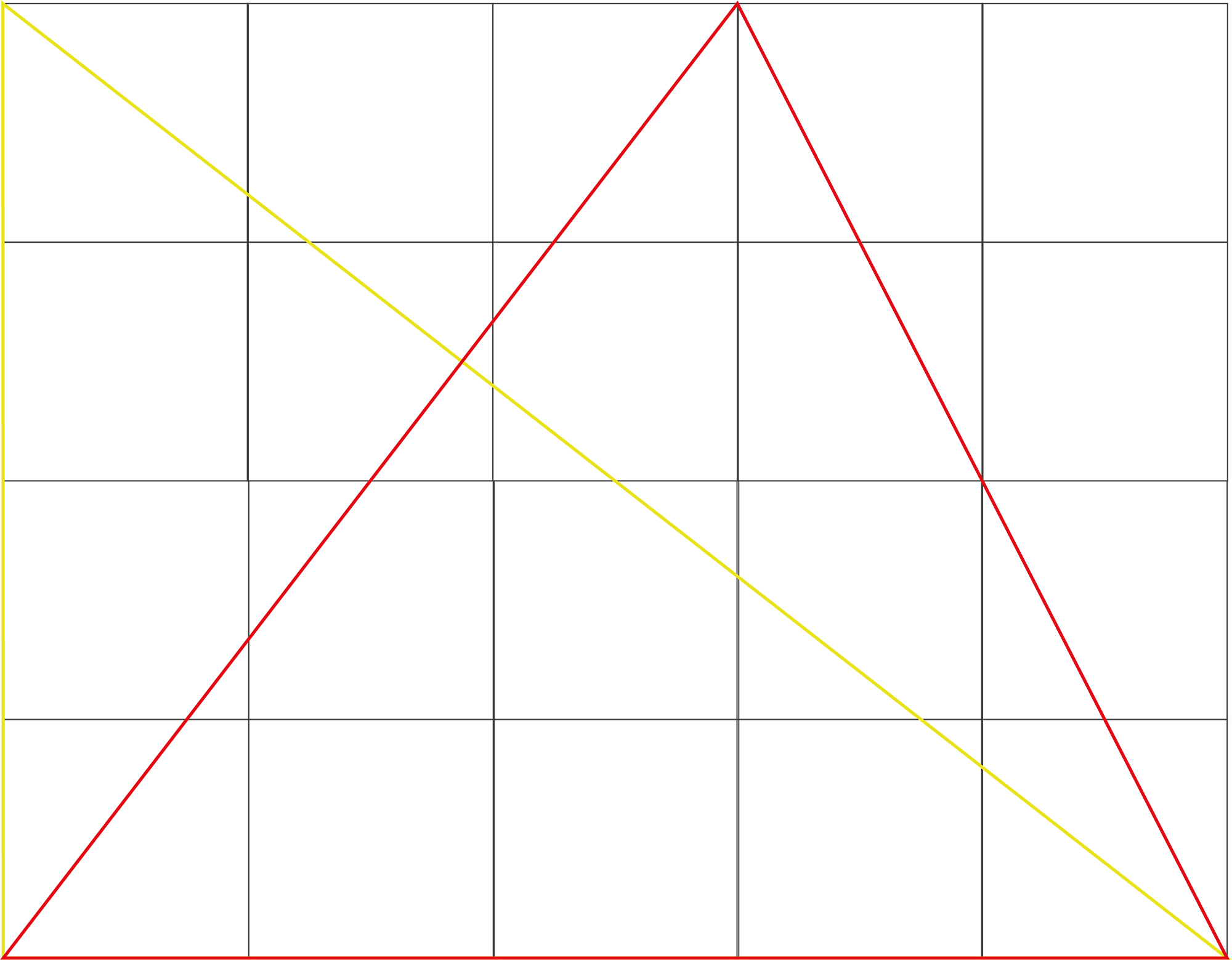
6. In the figure below, the area of a rectangle is 20 square cm.
Draw one straight line in the given rectangle and divide it into two equal triangles. Find the area of each triangle.
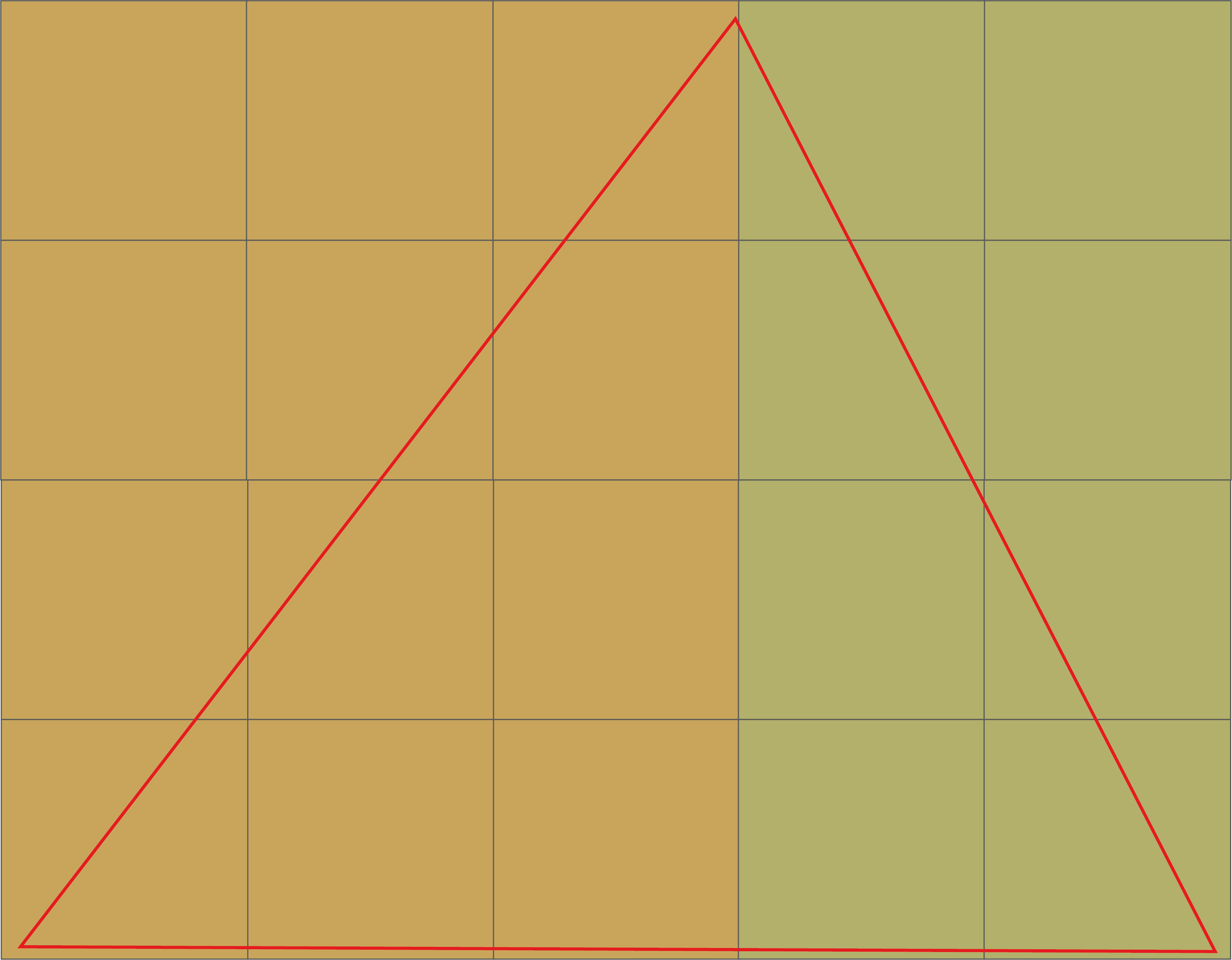
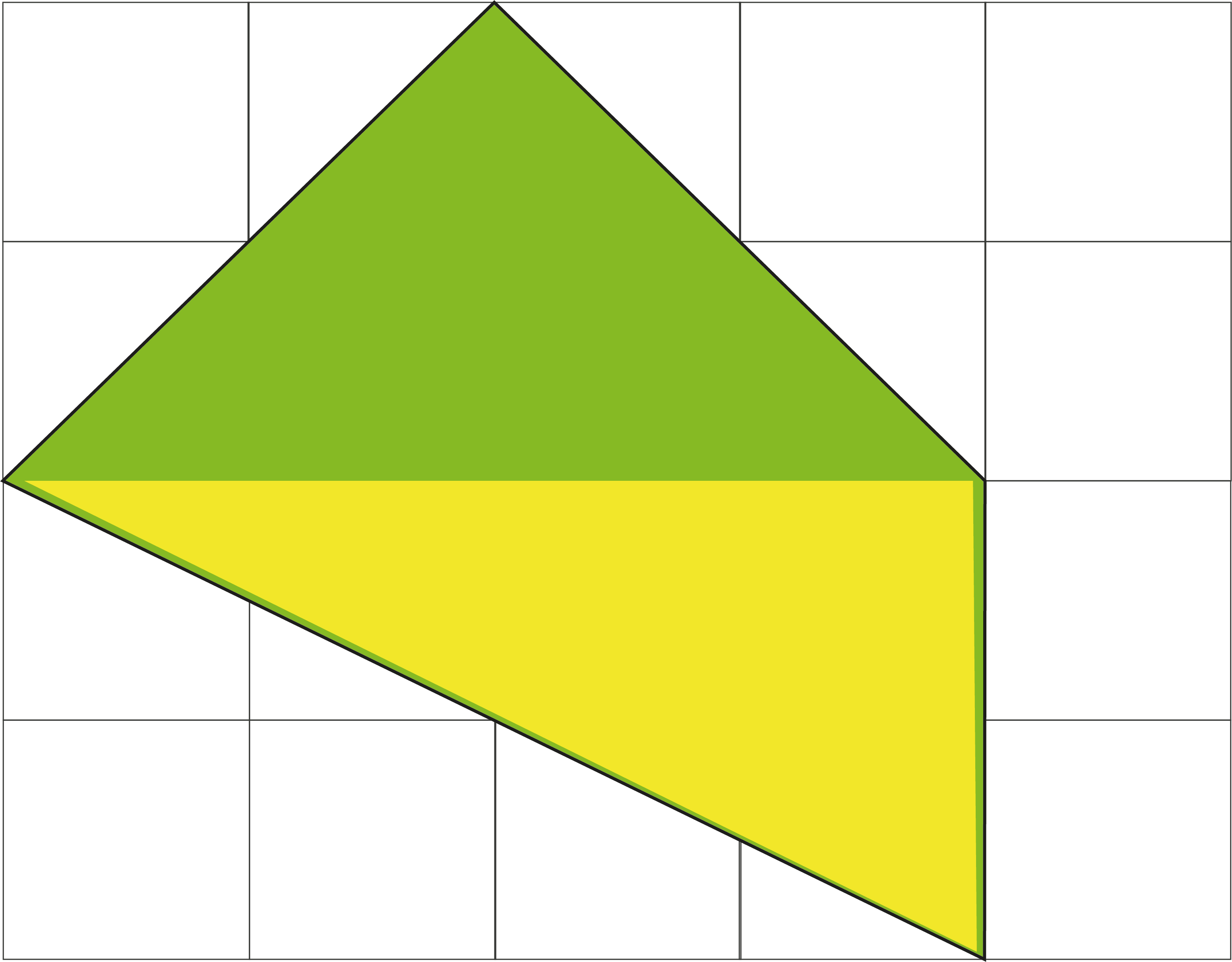
7. In the figure below, the green area is 4 square cm and the yellow area is 6 square cm. Explain.
8. In the figure below, the perimeter of a rectangular field is 20 cm. If the breadth is 5 cm, find the length of the field.

9. A classroom blackboard is 75 cm long and 80 cm wide. Find its area and perimeter.
10. Complete the shape to make two more sides such that its area become 4 square cm.
11. In the figure below, the area of a square field is 25 square cm. Find its perimeter?
12. In the figure below, the area of the large rectangle is 40 square cm. The yellow triangle is half of the large rectangle. So the area of the yellow triangle is _______ square cm.
10 square cm
20 square cm
40 square cm
50 square cm
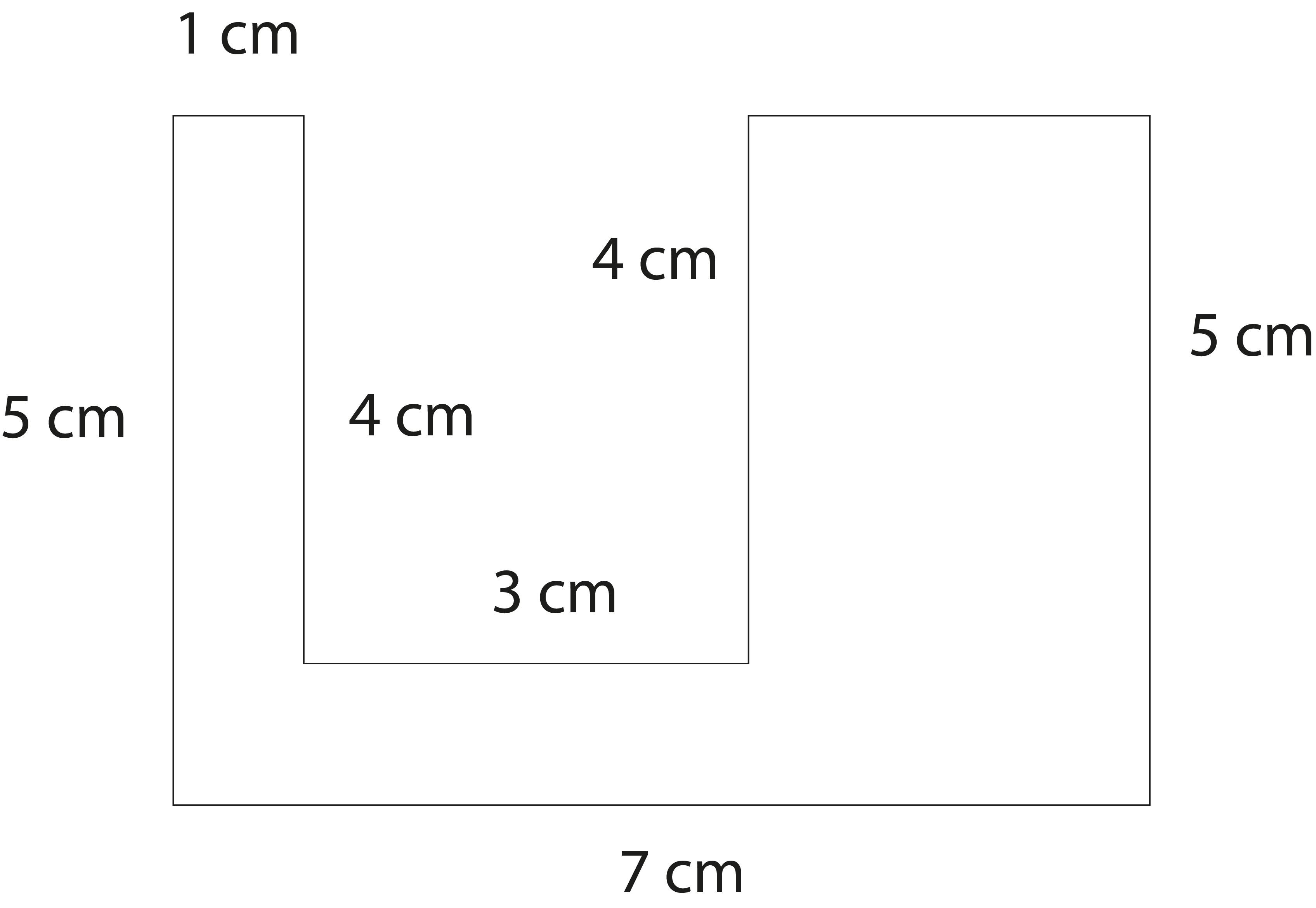
13. Fill in the blanks:
The perimeter of the given figure is ___________
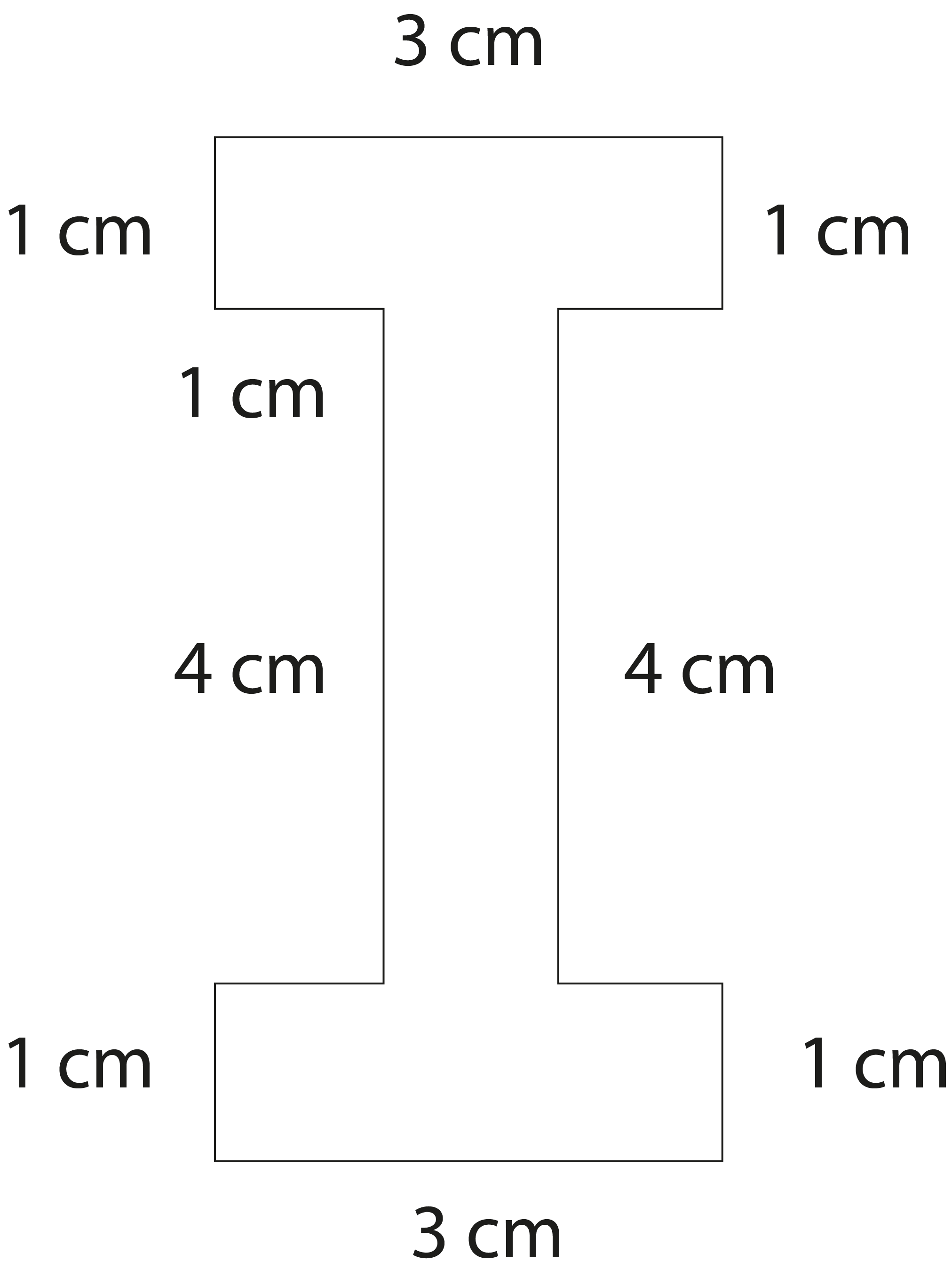
The perimeter of the given figure is ________
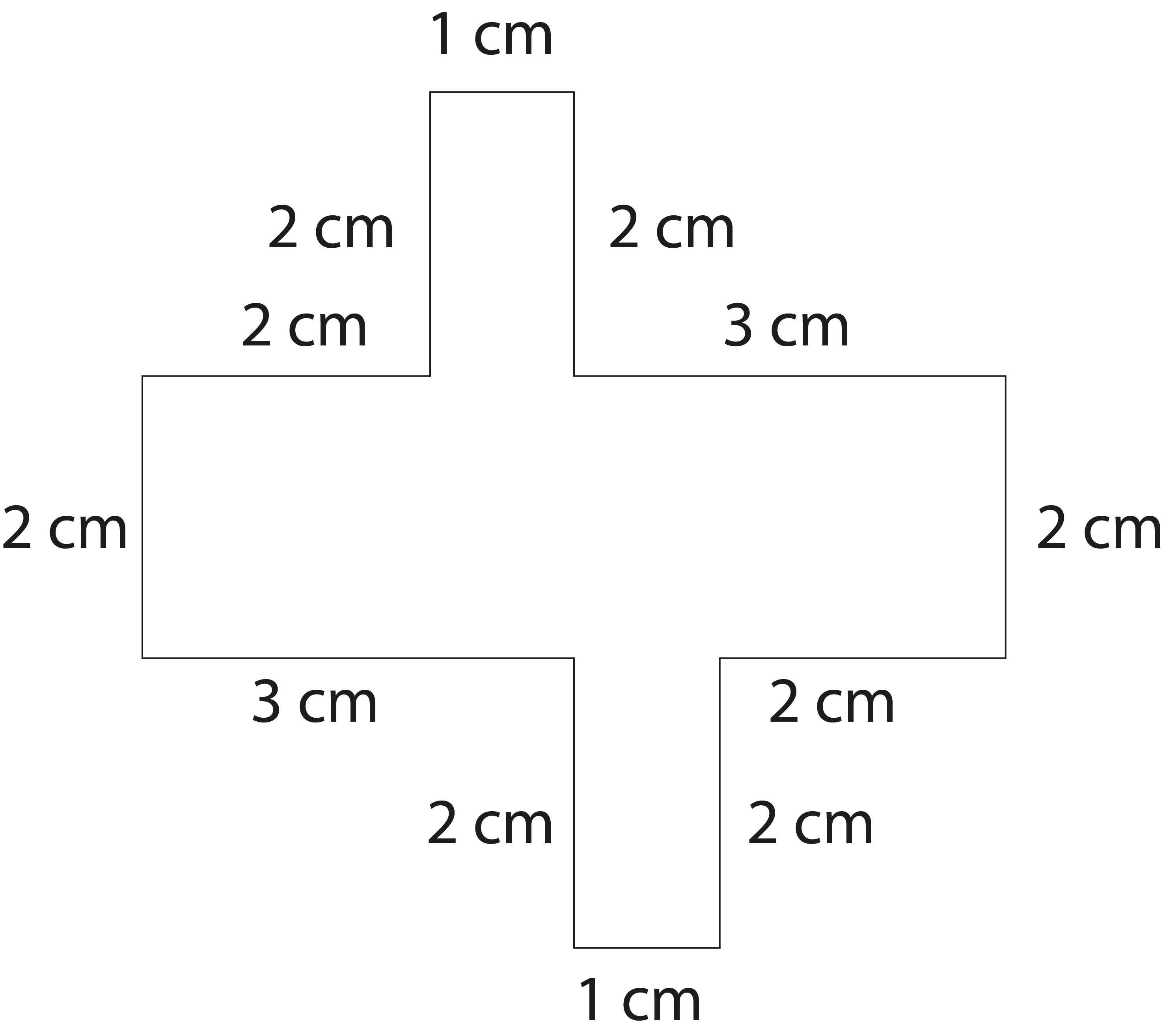
The perimeter of the given figure is ________
The perimeter of a square of side 10 cm is ___________
14. Match the column. Find the area of a rectangle.
Length = 5cm, breadth = 3cm | Area = 15 square cm |
Length = 8cm, breadth = 5cm | Area = 32 square cm |
Length = 5cm, breadth = 2cm | Area = 40 square cm |
Length = 8cm, breadth = 4cm | Area = 10 square cm |
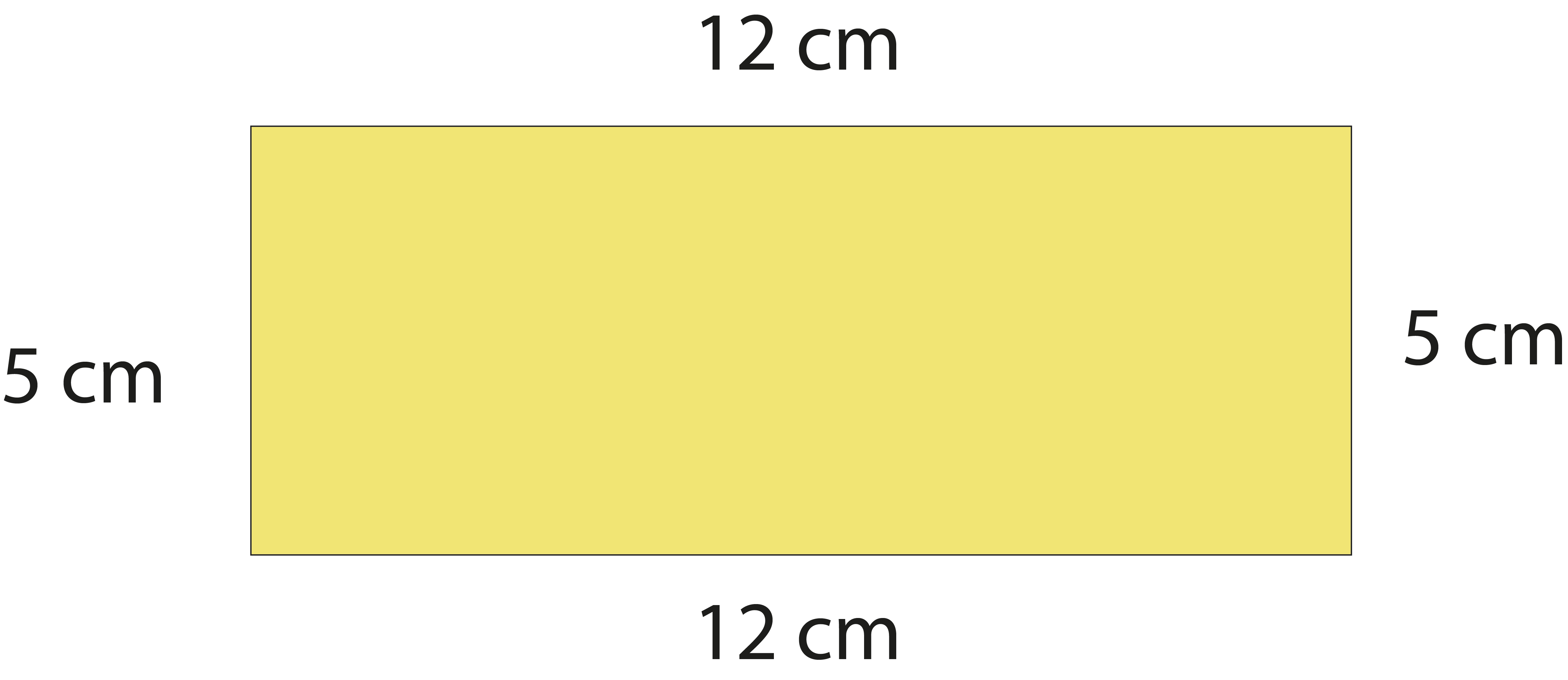
15. In the figure below, find the perimeter of the rectangle.
32 cm
34 cm
40 cm
24 cm
16. The perimeter of a rectangle with length $’l’$ and breadth $’b’$ is
$l+b$
$2(l+b)$
$l \times b$
$l-b$
17. In the figure below, the perimeter of a square field is 100 cm. Find the area of field?

50 square cm
625 square cm
100 square cm
250 square cm
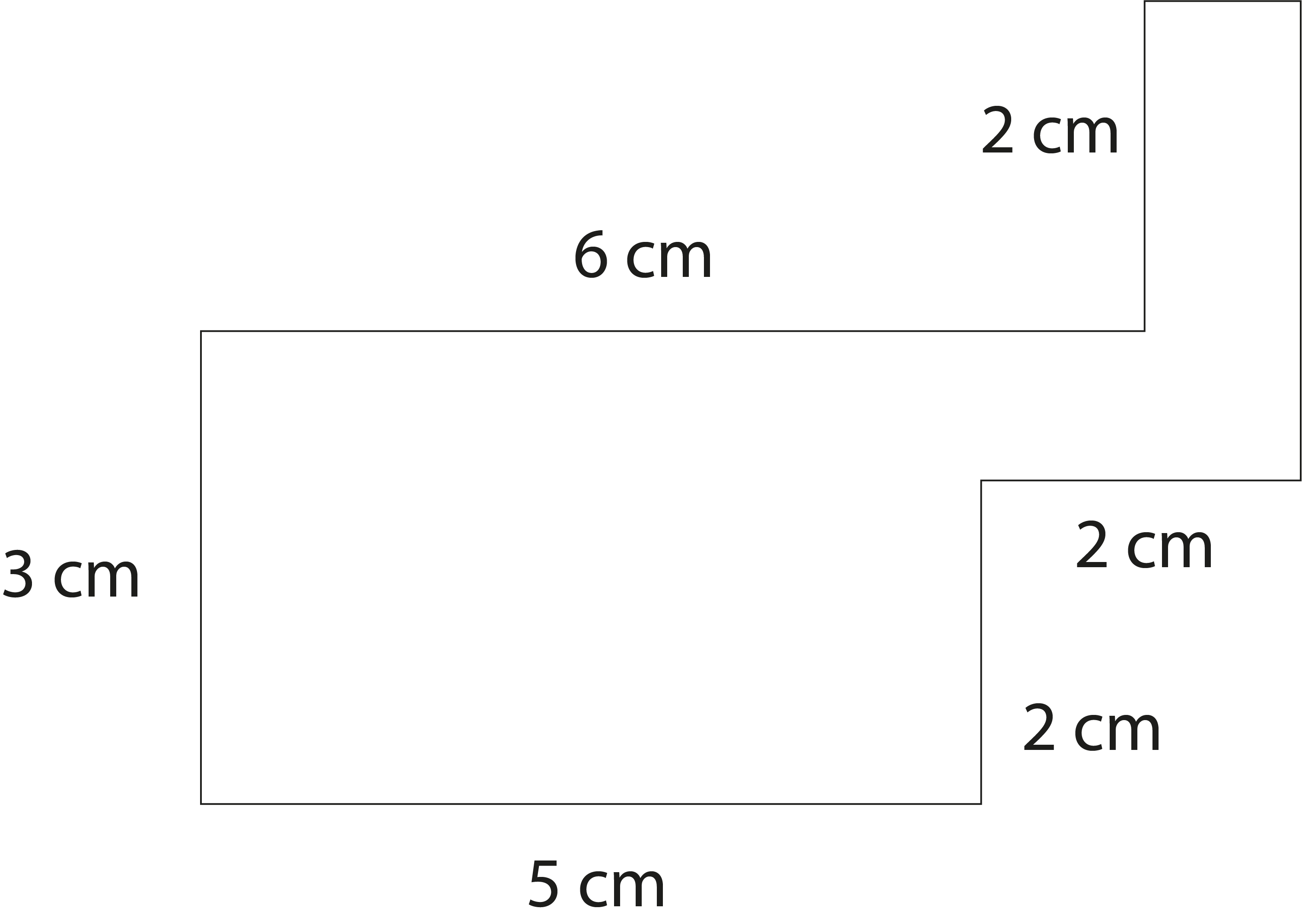
18. Find the perimeter of the given figure.
20 cm
24 cm
40 cm
32 cm
19. The area of a rectangle whose length is 5 cm and breadth is 4 cm is
9 square cm
20 square cm
12 square cm
24 square cm
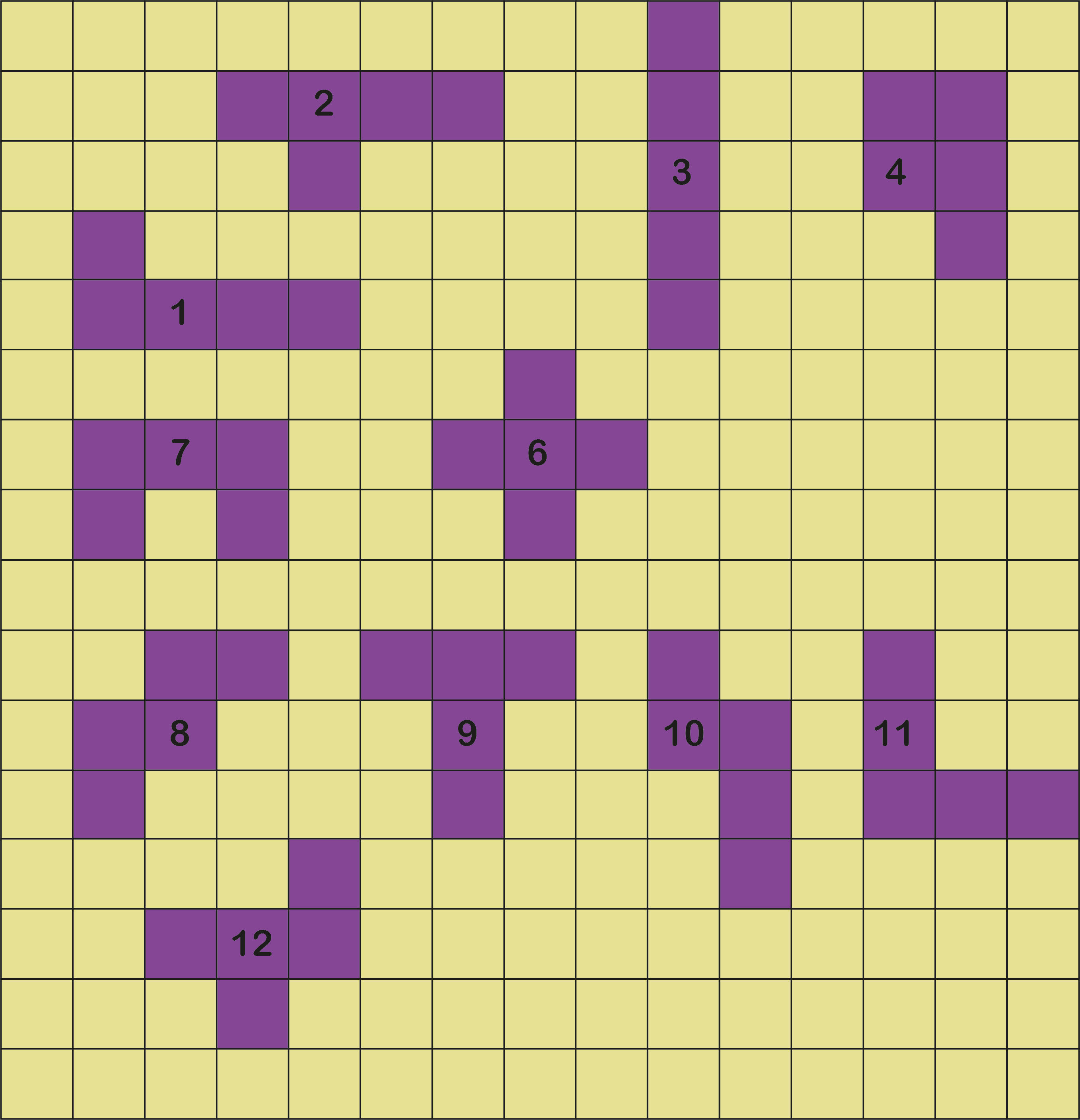
20.
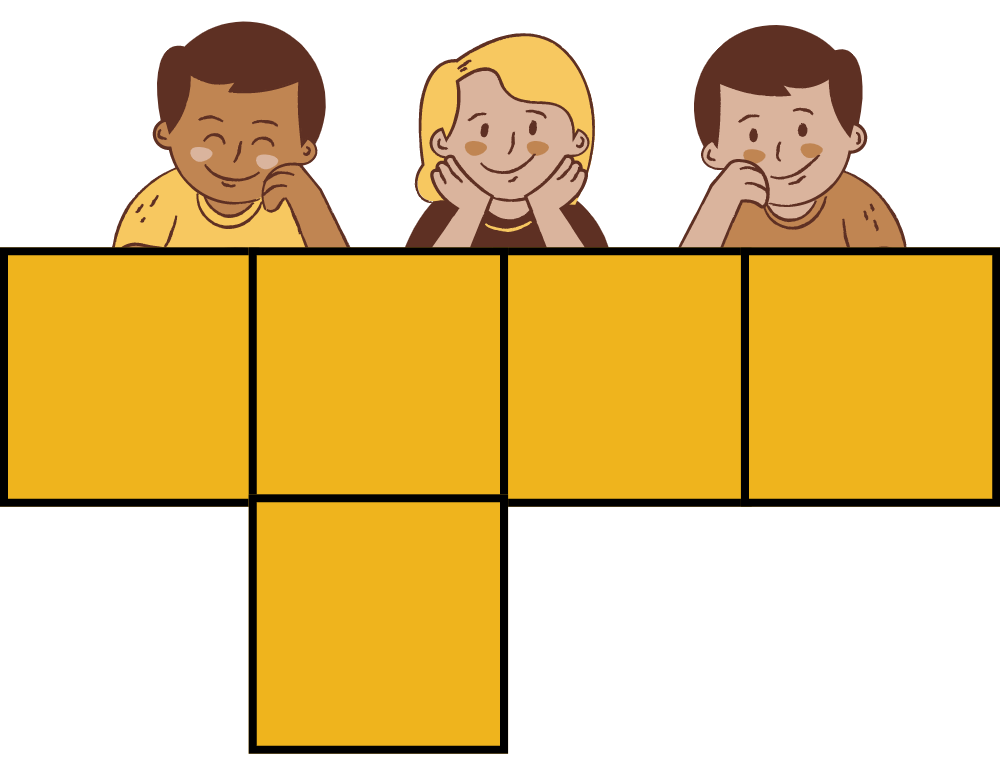
How many different shapes can we draw from the small square figure above? Three are drawn as examples.
Answers to the Worksheet:
1. The stamp covers 12 squares in the given figure. Each square is of side 1 cm.
Hence, the area of the stamp is 12 square cm.
2. The figure covers 8 squares of one-centimetre side.
3. As we know that,
Perimeter = Sum of all sides
$=1+2+3+3+4+5$
$=18cm$
4. In the given figure A, each square is of side 1 cm.
As we know that,
Area of triangle $= \dfrac{1}{2}\times \text{ }base\text{ }\times \text{ }height$
$= \dfrac{1}{2}\times \text{ 3 }\times \text{ 4}$
$\text{=6 c}{{\text{m}}^{2}}$
In the given figure B,
Area of square = 4 complete square + 8 half squares + 4 quarter squares
$=4+\left( \dfrac{1}{2}\times 8 \right)+\left( \dfrac{1}{4}\times 4 \right)$
$=4+4+1$
$=9\text{ }\!\!~\!\!\text{ c}{{\text{m}}^{2}}$
5. From the figure we have,
The orange rectangle contains 12 squares.
Therefore, the area of the orange rectangle = 12 square cm.
Now, the area of the orange portion of the triangle = $\dfrac{12}{2}$ = 6 square cm
Since the green rectangle contains 8 squares.
Therefore, the area of the green rectangle = 8 square cm
Area of the yellow portion of the triangle = $\dfrac{8}{2}$ = 4 square cm.
Therefore, the area of the red triangle = 6 + 4 = 10 square cm
6. From the figure, we get,
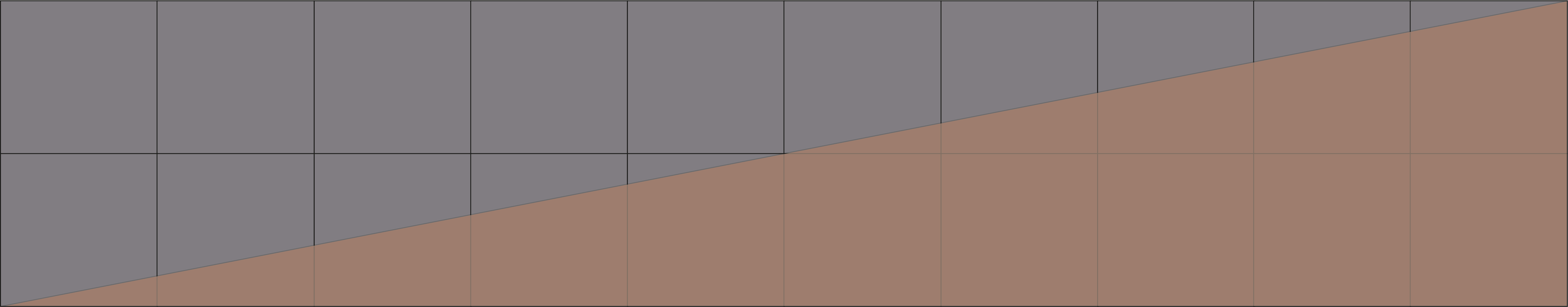
It is given that a straight line divides a rectangle into two equal triangles.
Area of rectangle = 10 × 2
= 20 square cm
Then, area of two equal triangles = $\dfrac{20}{2}$
= 10 square cm
7. Green colour area = 2 complete square + 4 half square
$=\text{ }2+\left( \dfrac{1}{2}\times \text{ }4 \right)$
$=\text{ }2\text{ }+\text{ }2$
$=\text{ }4\text{ }square\text{ }cm$
Yellow colour area = 3 complete square + 2 more than half + 2 half filled
$=\text{3+2}+\left( \dfrac{1}{2}\times \text{ 2} \right)$
$=3\text{ }+\text{ }2\text{ }+\text{ }1~=\text{ }6\text{ square cm}$
8. From the question, we have,
The Perimeter of a rectangular field = 20 cm.
Breadth = 5 cm
As we know that,
Perimeter of rectangle $=2(length\text{ + breadth)}$
$\Rightarrow 20 = 2 (length\text{ + 5)}$
$\Rightarrow (length\text{ + 5)}=\dfrac{20}{2}$
$\Rightarrow (length\text{ + 5)}=10cm$
$\Rightarrow length=10-5=5cm$
9. From the question, we have
Length = 75 cm and breadth = 12 cm
As we know that,
Area of rectangle $=length\text{ }\times \text{ breadth}$
$= 75\text{ }\times \text{ 12 sq}\text{.cm}$
$\text{= 900 sq}\text{.cm}$
Perimeter of rectangle $=2(length\text{ + breadth)}$
$= 2(75\text{ + 12)}$
$\text{= 2}\times \text{ 87}$
$\text{= 174 cm }$
10. From the figure, we get,
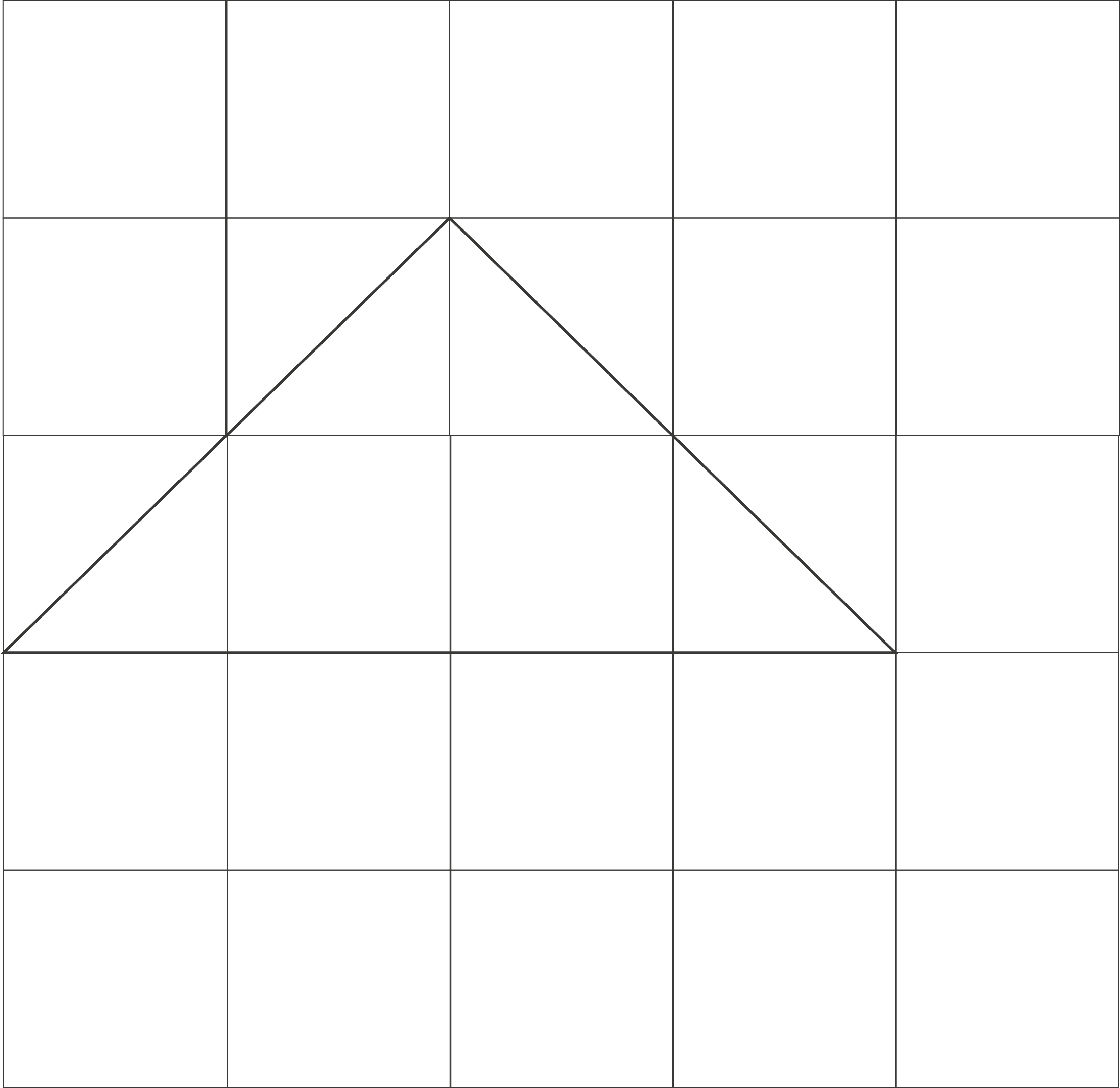
Area = 2 complete square + 4 half square
$=\text{ }2\text{ }+\text{ }\left( \dfrac{1}{2}\text{ }\times \text{ }4 \right)$
$=\text{ }2\text{ }+\text{ }2$
$=\text{ }4\text{ sq}\text{.cm}$
11. Given,
Area of a square field = 25 square cm
$\Rightarrow side\times side=\text{ }25\text{ }square\text{ }cm$
$\Rightarrow side\times side=\text{ 5}\times \text{5 }square\text{ }cm$
$\Rightarrow side=\text{ }5\text{ }cm$
Perimeter of a square field = $4\times side$
$=4\times 5 =20cm$
12. (b) 20 sq. cm
From the question, we have
Area of the big rectangle is 40 square cm.
Area of the yellow triangle = $\dfrac{40}{2}=20sq.cm$
13.
(a)
As we know that,
Perimeter = Sum of all sides
$=1+4+3+4+3+5+7+5$
$= 32$ cm
(b) As we know that,
Perimeter = Sum of all sides
$=3+1+4+1+1+3+1+4+1+1$
$=20$ cm
(c) As we know that,
Perimeter = sum of all sides
= 1+2+3+2+2+2+1+2+3+2+2+2
$= 24$ cm
(d) Given,
Side of square = 10 cm
As we know that,
Perimeter of square $=4\times side$
$=4\times 10$
$=40\text{cm}$
14.
1 | Length = 5cm, breadth = 3cm | Area = 15 square cm |
2 | Length = 8cm, breadth = 5cm | Area = 40 square cm |
3 | Length = 5cm, breadth = 2cm | Area = 10 square cm |
4 | Length = 8cm, breadth = 4cm | Area = 32 square cm |
Given,
Length = 5cm, breadth = 3cm
As we know that,
Area of rectangle $ = length\text{ }\times \text{ breadth}$
$= 5\text{ }\times \text{ 3 sq}\text{.cm}$
$\text{= 15 sq}\text{.cm}$
Length = 8cm, breadth = 5cm
As we know that,
Area of rectangle $= length\text{ }\times \text{ breadth}$
$= 8\text{ }\times \text{ 5 sq}\text{.cm}$
$\text{= 40 sq}\text{.cm}$
Length = 5cm, breadth = 2cm
As we know that,
Area of rectangle $= length\text{ }\times \text{ breadth}$
$= 5\text{ }\times \text{ 2 sq}\text{.cm}$
$\text{= 10 sq}\text{.cm}$
Length = 8cm, breadth = 4cm
As we know that,
Area of rectangle $= length\text{ }\times \text{ breadth}$
$ = 8\text{ }\times \text{ 4 sq}\text{.cm}$
$\text{= 32 sq}\text{.cm}$
15. (b) Given,
Length = 12 cm and breadth = 5 cm
As we know that,
Perimeter of rectangle $=2(length\text{ + breadth)}$
$=2(12\text{ + 5)}$
$=2\times 17$
$\text{=34 cm }$
16. (b)

As we know that,
Perimeter = Sum of all sides
Perimeter of rectangle $=l+b+l+b$
$=2(l+b)$
17. (b)
Given,
Perimeter of square = 100 cm
We know that,
Perimeter = 4 × side
100 = 4 × side
Side = 25 cm
Area of a square field = side × side
= 25 × 25
= 625 square cm.
18. (a)
As we know that,
Perimeter = Sum of all sides.
$=3+5+2+2+2+6$
$=20$ cm.
19. (b) Given,
Length = 5 cm and breadth = 4 cm
As we know that,
Area of rectangle $=length\text{ }\times \text{ breadth}$
$=5\text{ }\times \text{ 4 sq}\text{.cm}$
$\text{= 20 sq}\text{.cm}$
20. From the given 5 squares, we can draw 12 shapes as shown in below,
Benefits of Chapter 3 How Many Squares Class 5 Worksheets
The worksheet on squares has a variety of questions that will help students ace their exams on this topic. All the aspects of squares like area, perimeter, diagonal, etc. are covered in depth in How Many Squares Class 5 worksheets.
A strong understanding of shapes like squares helps strengthen a student's capabilities in many areas of Maths since the square is the building block for other shapes such as rectangles, rhombus, etc.
A square shape is widely used in our daily lives; tiles, floors, walls, game boards, chess, a slice of bread, etc., around us, are all mostly square or rectangular in shape. So if we understand squares and their properties, we will be able to calculate the price of covering floor area and perform other such computations easily.
The basic knowledge of squares and solving questions on this topic also helps in higher classes as the concept of a square is used in many advanced topics.
Examples of Class 5 How Many Squares
Students are presented with a grid-like structure as shown below, and they need to count how many squares and rectangles can be made out of that grid:
From the above grid, students need to find out the rectangle and square with maximum and minimum perimeters.
Take a new note of Rs. 10 and trace its outline to find its area and perimeter.
A grid is given to students, which is coloured differently in different regions, and students need to calculate the area of a specific coloured region.
Fill in the blanks exercises along with drawing and graphing activities.
Some Facts About Square Shape
A square is a regular quadrilateral since it has four sides which are all equal.
The area of a square is larger than all other quadrilaterals which have the same perimeter.
A square is also a rhombus with right angles and is also a rectangle that has equal sides.
All the lines in a square intersect each other at right angles (90 degrees), and the sum of angles within a square is 360 degrees.
A square's diagonals are perpendicular to each other, i.e., they bisect each other at 90 degrees.
In a cube, there are six square faces, and it is equivalent to a square in many ways. The only difference is a cube is in three dimensions, whereas a square is in two dimensions.
A square has its opposite sides parallel to each other.
You can see squares everywhere when you look outside your window, in traffic, buildings, windows, etc.
Important Topics of Class 5 Squares
The square, its area, and perimeter.
The different properties of a square.
Get Your Free PDF of Class 5 Maths Chapter 3 Worksheet with Answers
Vedantu's team of experts has prepared the How Many Squares Class 5 worksheets in accordance with the latest CBSE curriculum. The experts understand the exam pattern and important questions of this chapter, hence students practising with our worksheets are sure to score extremely well in their exams.
In the PDF, you would find a clear explanation of all the concepts related to squares through pictures and many solved examples.
The PDF is downloadable, so you can download it on your device and revise it from anywhere, even without an internet connection.
Parents would find the PDF extremely useful in monitoring their kids' progress in Maths.
For those who like to take a quick look at all the formulas and concepts right before the exam, the printable copy of the PDF comes in handy.
The Class 5 Maths Chapter 3 Worksheet with answers prepared by Vedantu is an extremely valuable online resource which kids will benefit immensely from while they are preparing for their Maths exam.
FAQs on CBSE Class 5 English Worksheet Chapter 3 How Many Squares - PDF
1. What is the perimeter of a square?
The perimeter of any shape is the sum of all sides of that shape. The perimeter of a square is four times the dimension of one of its sides since a square has four sides and all its sides have equal magnitude.
2. How do you find the area of a square?
If a is the length of a square, then its area is given by a x a = a2.
3. Is a square a special type of rectangle?
Yes, a square is a special type of rectangle, i.e., it is an equilateral rectangle where all the sides are equal.
















