Important Questions for CBSE Class 12 Physics Chapter 10 - Wave Optics 2024-25

Very Short Questions and Answers (1 Mark Questions)

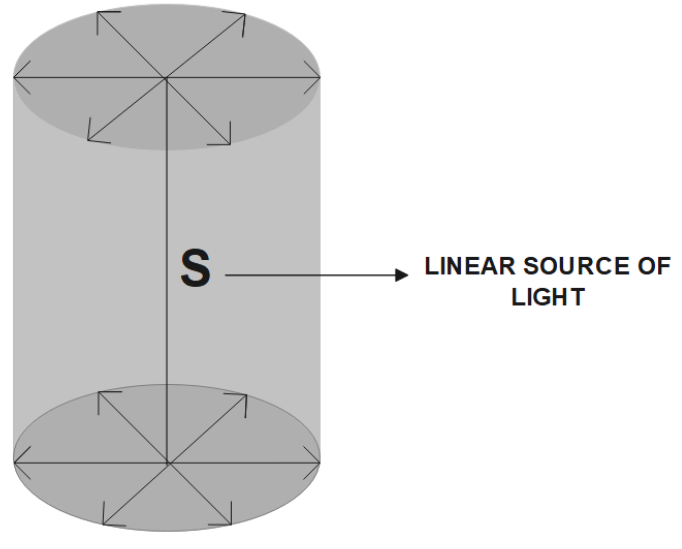
1. Draw a diagram to show cylindrical wave front. 

Ans:

2. A light wave enters from air to glass. How will the following be affected? 

(a) Energy of the wave 

Ans: When light wave enters from air to glass, part of light is reflected back into the air. Therefore, energy of the wave would be lower in the glass. 

(b) Frequency of the wave

Ans: When light wave enters from air to glass, frequency of the wave would remain unchanged. 

3. What is the Brewster angle for air to glass transition?$({{\mu }_{g}}=1.5)$ 

Ans: We can find Brewster angle for air to glass transition by the following,

$\mu =\tan \left( {{i}_{p}} \right)$

\[\Rightarrow 1.5=\tan \left( {{i}_{p}} \right)\]

\[\therefore {{i}_{p}}={{\tan }^{-1}}(1.5)\]

Therefore, Brewster angle for air to glass transition is found to be \[{{\tan }^{-1}}(1.5)\].

4. What is the shape of the wave front when light is diverging from a point source?

Ans: When light diverges from a point source the wave front would show spherical shape.

5. State the conditions that must be satisfied for two light sources to be coherent. 

Ans: The conditions to be satisfied are the following:

a) They must emit waves continuously of same wavelengths. 

b) The phase difference between the waves must be zero or constant.

6. In Young’s double slit experiment. The distance between the slits is halved, what change in the fringe width will take place? 

Ans: The equation for fringe width could be defined as, \[\beta =\frac{\lambda D}{d}\].

When the distance between slits gets halved, \[{d}'=\frac{d}{2}\],

The new fringe width would be, \[{\beta }'=\frac{2\lambda D}{d}\]

\[\therefore {\beta }'=2\beta \]

Hence, the fringe width becomes double when distance between slits get halved.

Short Questions and Answers (2 Marks Questions)

1. Obtain an expression for the ratio of intensities at maxima and minima in an interference pattern. 

Ans: Suppose ${{a}_{1}}$ and ${{a}_{2}}$ be the amplitudes and ${{I}_{1}}$ and ${{I}_{2}}$ be the intensities of light waves which interfere each other.

$\text{Intensity }\propto \text{ (Amplitude}{{\text{)}}^{2}}$ 

$\frac{{{I}_{1}}}{{{I}_{2}}}=\frac{{{a}_{1}}^{2}}{{{a}_{2}}^{2}}$

After interference (applying superposition principle) 

Amplitude at maxima $={{a}_{1}}+{{a}_{2}}$  

Amplitude at minima \[={{a}_{1}}-{{a}_{2}}\]

$\Rightarrow \frac{{{I}_{\max }}}{{{I}_{\min }}}=\frac{{{({{a}_{1}}+{{a}_{2}})}^{2}}}{{{({{a}_{1}}-{{a}_{2}})}^{2}}}$ 

$\therefore \frac{{{I}_{\max }}}{{{I}_{\min }}}=\frac{{{\left( \frac{{{a}_{1}}}{{{a}_{2}}}+1 \right)}^{2}}}{{{\left( \frac{{{a}_{1}}}{{{a}_{2}}}-1 \right)}^{2}}}={{\left( \frac{r+1}{r-1} \right)}^{2}}$

where $r=\frac{{{a}_{1}}}{{{a}_{2}}}=\sqrt{\frac{{{I}_{1}}}{{{I}_{2}}}}=$ amplitude ratio of two waves. 

Hence, we found the required ratio to be, $\frac{{{I}_{\max }}}{{{I}_{\min }}}=\frac{{{\left( \frac{{{a}_{1}}}{{{a}_{2}}}+1 \right)}^{2}}}{{{\left( \frac{{{a}_{1}}}{{{a}_{2}}}-1 \right)}^{2}}}={{\left( \frac{r+1}{r-1} \right)}^{2}}$.

2. A slit $\mathbf{S}$ is illuminated by a monochromatic source of light to give two coherent sources ${{P}_{1}}$  and ${{P}_{2}}$ . These given bright and dark bands on a screen. At a point $R$, on the screen, there is a dark fringe. What relation must exist between the lengths ${{P}_{1}}R$ and ${{P}_{2}}R$ ? 

Ans: At point $R$, there will be a dark fringe when path difference $={{P}_{2}}R-{{P}_{1}}R$. 

Where, $\lambda $  is the wavelength of the light and $n=0,1,2,3\ldots $ 

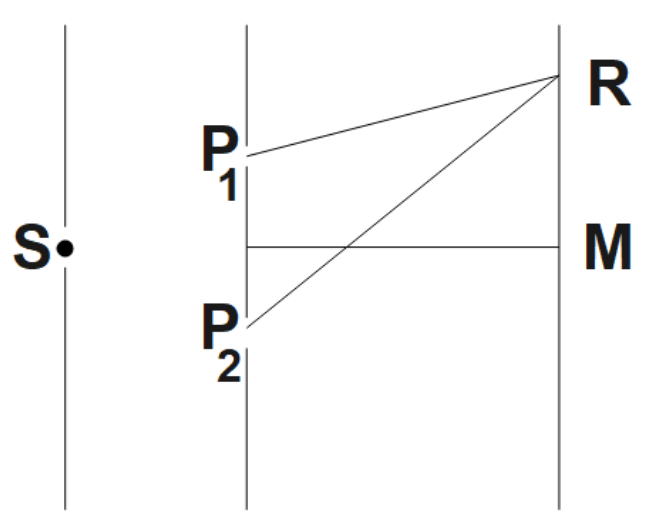
3. In young’s double slit experiment how is the fringe width change when 

1. Light of smaller frequency is used

Ans: We know, fringe width could be expressed as, 

$\beta =\frac{D\lambda }{d}$. If light of smaller frequency, in other words, higher wavelength is used, the fringe width would increase.

2. Distance between the slits is decreased? 

Ans:  We know that, fringe width is inversely proportional to the distance between the slits, that is, 

$\beta \propto \frac{1}{d}$

So, if distance between the slits is decreased, the fringe width will increase. 

4. Write two points of difference between interference and diffraction. 

Ans: Some differences between interference and diffraction are the following:

5. Consider interference between two sources of intensities $I$ and $4I$. What will be the intensity at points where phase differences are: 

1. $\frac{\pi }{2}$

Ans: We know that, $I={{a}^{2}}+{{b}^{2}}+2ab\cos \phi $ 

Where, $a$ and $b$ are amplitudes of two coherent waves that are having phase difference of $\phi $ .

Here \[{{a}^{2}}=I,{{b}^{2}}=4I\] 

$\Rightarrow I=I+4I+2\sqrt{I}\sqrt{4I}\cos \phi $  

$\Rightarrow I=5I+4I\cos \phi $

 When, $\phi =\frac{\pi }{2}$  

$\Rightarrow I=5I+4I\cos \frac{\pi }{2}$ 

$\therefore I=5I$

Therefore, the intensity will be five times more.

2. $\pi $

Ans:  When, $\phi =\pi $ 

$I=5I+4I\cos \phi $ 

$\Rightarrow I=5I-4I$

$\therefore I=I$

Hence, intensity will be same.

3. Can white light produce interference? What is the nature? 

Ans: White light would produce interference. But because of different colours present in white light, the interference pattern overlaps the central bright fringe for all the colours at the position, so its colour is found to be white. The white central bright fringe is seen to be surrounded by few coloured rings. 

6. 

1. The refractive index of glass is 1.5. What is the speed of light in glass? Speed of light in vacuum is $3.0\times {{10}^{8}}m{{s}^{-1}}$ .

Ans: Given refractive index of glass, $\mu =1.5$ 

Speed of light, $c=3\times {{10}^{8}}m/s$  

Now, we have the speed of light in glass is given by the relation,

$v=\frac{c}{\lambda }$

Substituting the given values, 

$\Rightarrow v=\frac{3\times {{10}^{8}}}{1.5}$

$\therefore v=2\times {{10}^{8}}m/s$

Hence, the speed of light in glass is found to be $2\times {{10}^{8}}m/s$ . 

2. Is the speed of light in glass independent of the colour of light? If not, which of the two colours red and violet travels slower in a glass prism? 

Ans: The speed of light in glass is found to be dependent on the colour of light. 

The refractive index of a violet component of white light is known to be greater than that of the refractive index of a red component. Hence, the speed of violet light is less than the speed of red light in glass. Hence, the violet light travels slower than red light in a glass prism. 

7. What is the Brewster angle for air to glass transition? (Refractive index of glass $=1.5$)

Ans: We are given the refractive index of glass to be,  $\mu =1.5$ 

Brewster angle $=\theta $ 

Brewster angle is known to be related to refractive index as:

$\tan \theta =\mu $  

$\Rightarrow \theta ={{\tan }^{-1}}(1.5)$

$\therefore \theta =56.31{}^\circ $

Therefore, the Brewster angle for air to glass transition is found to be ${{56.31}^{\circ }}$.

8. Estimate the distance for which ray optics is a good approximation for an aperture of $4mm$ and wavelength $400nm$. 

Ans: Fresnel's distance \[\left( {{Z}_{F}} \right)\] is the distance for which the ray optics is a good approximation. It is given by the relation, ${{Z}_{F}}=\frac{{{a}^{2}}}{\lambda }$ 

Where, Aperture width,$a=4\text{ mm }=4\times {{10}^{-3}}\text{ m}$  

Wavelength of light, $\lambda =400\text{ nm =}400\times {{10}^{-9}}\text{ m}$ 

Substituting the given values, 

$\Rightarrow {{Z}_{F}}=\frac{{{\left( 4\times {{10}^{-3}} \right)}^{2}}}{400\times {{10}^{-9}}}$ 

$\therefore {{Z}_{F}}=40m$

Therefore, the distance for which the ray optics is a good approximation is found to be $40m$.

9. Let us list some of the factors, which could possibly influence the speed of wave propagation: 

1. Nature of the source

2. Direction of propagation

3. Motion of the source and/or observer

4. Wave length

5. Intensity of the wave. On which of these factors, if any, does

1. The speed of light in vacuum

Ans: We know that the speed of light in vacuum, $3\times {{10}^{8}}\text{ }m/s$  (approximately) is a universal constant. It is not affected by the motion of the source, the observer, or both. So, the given factor does not affect the speed of light in a vacuum. 

2. The speed of light in a medium (say, glass or water), depends.

Ans: Out of the listed factors, the speed of light in a medium would depend on the wavelength of light in that medium. 

10.  For sound waves, the Doppler formula for frequency shift differs slightly between the two situations given and explain why this should be so. Would you expect the formulas to be strictly identical for the two situations in case of light travelling in a medium? 

1. source at rest; observer moving, and 

2.  source moving; observer at rest. The exact Doppler formulas for the case of light waves in vacuum are, however, strictly identical for these situations.

Ans: We know that sound waves can propagate only through a medium. The two given situations are not scientifically identical as the motion of an observer relative to a medium is different in the given two situations. So, the Doppler formulas for the given two situations cannot be the same. 

Now, in case of light waves, sound can travel in a vacuum. But in vacuum, the above two cases are found to be identical as the speed of light is independent of the motion of the observer and the motion of the source. While, when light travels in a medium, the above two cases are not identical as the speed of light would now depend on the wavelength of the medium. 

11. In double-slit experiment using light of wavelength $600nm$, the angular width of a fringe formed on a distant screen is  $0.1{}^\circ $. What is the spacing between the two slits?

Ans: We are given:

Wavelength of light used, $\lambda =6000\text{ nm }=600\times {{10}^{-9}}\text{ m}$ 

Angular width of fringe, $\theta =0.1{}^\circ =0.1\times \frac{\lambda }{180}=\frac{3.14}{1800}\text{ rad}$ 

Angular width of a fringe is related to slit spacing $(d)$ as:

\[\theta =\frac{\lambda }{d}\] 

\[\Rightarrow d=\frac{\lambda }{\theta }\]

\[\therefore d=\frac{600\times {{10}^{-9}}}{\frac{3.14}{1800}}=3.44\times {{10}^{-4}}\text{ m}\]

Therefore, the spacing between the slits is found to be $3.44\times {{10}^{-4}}\text{ m}$. 

12.  In deriving the single slit diffraction pattern, it was stated that the intensity is zero at angles of $n\lambda /a$ . Justify this by suitably dividing the slit to bring out the cancellation. 

Ans: Consider that a single slit of width $d$ is divided into $n$ smaller slits. 

That is, width of each slit,  $d=\frac{d}{n}$ 

Angle of diffraction is given by the relation, 

\[\theta =\frac{\frac{d}{d}\lambda }{d}=\frac{\lambda }{d}\] 

Now, each of these infinitesimally small slits send zero intensity in direction $\theta$. Thus, the combination of these slits will give zero intensity. 

13. Answer the following questions:

1. When a low flying aircraft passes overhead, we sometimes notice a slight shaking of the picture on our TV screen. Suggest a possible explanation. 

Ans: We know that weak radar signals are sent by a low flying aircraft and this can interfere with the TV signals received by the antenna. As a result of this, the TV signals might get distorted. Hence, when a low flying aircraft passes overhead, we could sometimes notice a slight shaking of the picture on our TV screen.

2. As you have learnt in the text, the principle of linear superposition of wave displacement is basic to understanding intensity distributions in diffraction and interference patterns. What is the justification of this principle? 

Ans: For our understanding of intensity distributions and interference patterns, the principle of linear superposition of wave displacement is essential. This is because superposition follows from the linear character of a differential equation that is known to govern wave motion. Let ${{y}_{1}}$ and ${{y}_{2}}$ are the solutions of the second order wave equation, then any linear combination of ${{y}_{1}}$ and ${{y}_{2}}$ might also be the solution of the wave equation. 

Very Long Questions and Answers (3 Marks Questions)

1. State Brewster law? Using this law prove that, at the polarizing angle of incidence, the reflected and transmitted rays are perpendicular to each other? 

Ans:  We know that, Brewster law states that the longest of the angle of polarization for a transparent medium is equal to the refractive index of the medium.

$\mu =\tan {{i}_{p}}$  

Proof: Using Snell’s law 

$\mu =\frac{\sin i}{\sin r}$

when $i={{i}_{p}},\mu =\frac{\sin {{i}_{p}}}{\sin {{r}_{p}}}$ …………………….. (1) 

Also, we have,  \[\tan {{i}_{p}}=\frac{\sin {{i}_{p}}}{\cos {{i}_{p}}}\]…………………. (2)

From (1) and (2), 

$\frac{\sin {{i}_{p}}}{\sin {{r}_{p}}}=\frac{\sin {{i}_{p}}}{\cos {{i}_{p}}}$

$\Rightarrow \sin {{r}_{p}}=\cos {{i}_{p}}$

$\Rightarrow \sin {{r}_{p}}=\sin (90{}^\circ -{{i}_{p}})$

$\therefore {{r}_{p}}+{{i}_{p}}=90{}^\circ $

Therefore, at the polarizing angle of incidence, the reflected and transmitted rays are found to be perpendicular to each other.

2. In a single slit experiment, how is the angular width of central bright fringe maximum changed when 

1. The slit width increased 

Ans: For single slit diffraction, we have the fringe width, $\beta =\frac{2D\lambda }{d}$ 

Therefore, when slit width $'d'$  is increased, $\beta $ decreases.

2. The distance between the slit and the screen is increased.

Ans: When $'D'$ is increased, width of central bright fringe will become maximum i.e., increase as both quantities are directly proportional to each other. 

3. Light of a smaller wavelength is used. 

Ans: When the light of a smaller wavelength is used, the width of the central bright maximum would decrease. 

3. In a Young’s double slit experiment, the slits are repeated at $0.24\text{ mm}$. The screen is $1.2\text{ m}$ away from the slits. The fringe width is $0.03cm$. Calculate the wavelength of light used in the experiment? 

Ans: We are given:

$\beta =0.3\text{ cm =3}\text{.0}\times \text{1}{{\text{0}}^{-3}}\text{ m}$ 

$D=1.2\text{ m}$ 

$d=0.24\text{ mm =2}\text{.4}\times \text{1}{{\text{0}}^{-4}}\text{ m}$

$\beta \text{=}\frac{D\lambda }{d}$

$\Rightarrow \lambda =\frac{\beta d}{D}$

Substituting the given values, 

$\Rightarrow \lambda =\frac{3.0\times {{10}^{-3}}\times 2.4\times {{10}^{-4}}}{1.2}$

$\therefore \lambda =6\times {{10}^{-7}}m$

Therefore, the wavelength of light used is found to be $6\times {{10}^{-7}}m$.

4. Two coherent sources whose intensity ratio is $81:1$ produce interference fringes. Calculate the ratio of intensity of maxima and minima in the interference pattern? 

Ans:  From the given ratio we can write,

 \[\frac{{{I}_{1}}}{{{I}_{2}}}=\frac{81}{1}\]

We know that, $\text{Intensity }\propto \text{ (Amplitude}{{\text{)}}^{2}}$ 

$\Rightarrow \frac{{{a}_{1}}}{{{a}_{2}}}=\sqrt{\frac{81}{1}}=\frac{9}{1}=r$

$\Rightarrow \frac{{{I}_{\max }}}{{{I}_{\min }}}=\frac{{{\left( r+1 \right)}^{2}}}{{{\left( r-1 \right)}^{2}}}={{\left( \frac{9+1}{9-1} \right)}^{2}}={{\left( \frac{10}{8} \right)}^{2}}$

$\Rightarrow \frac{{{I}_{\max }}}{{{I}_{\min }}}=\frac{100}{64}$

$\Rightarrow \frac{{{I}_{\max }}}{{{I}_{\min }}}=\frac{24}{16}$

$\therefore {{I}_{\max }}:{{I}_{\min }}=25:16$

Therefore, the ratio of intensities of maxima and minima in the interference pattern is found to be \[25:16\].

5. Using Huygens’s principle, deduce the laws of refraction. 

Ans: Let us consider a plane wavefront AB that is incident on a plane surface that is separating two media. Also, note that, here the medium 1 is rarer and medium 2. And if ${{v}_{1}}\text{ and }{{\text{v}}_{2}}$are velocities of light in medium 1 and medium 2 respectively, we have, ${{v}_{2}}\rangle {{v}_{1}}$. 

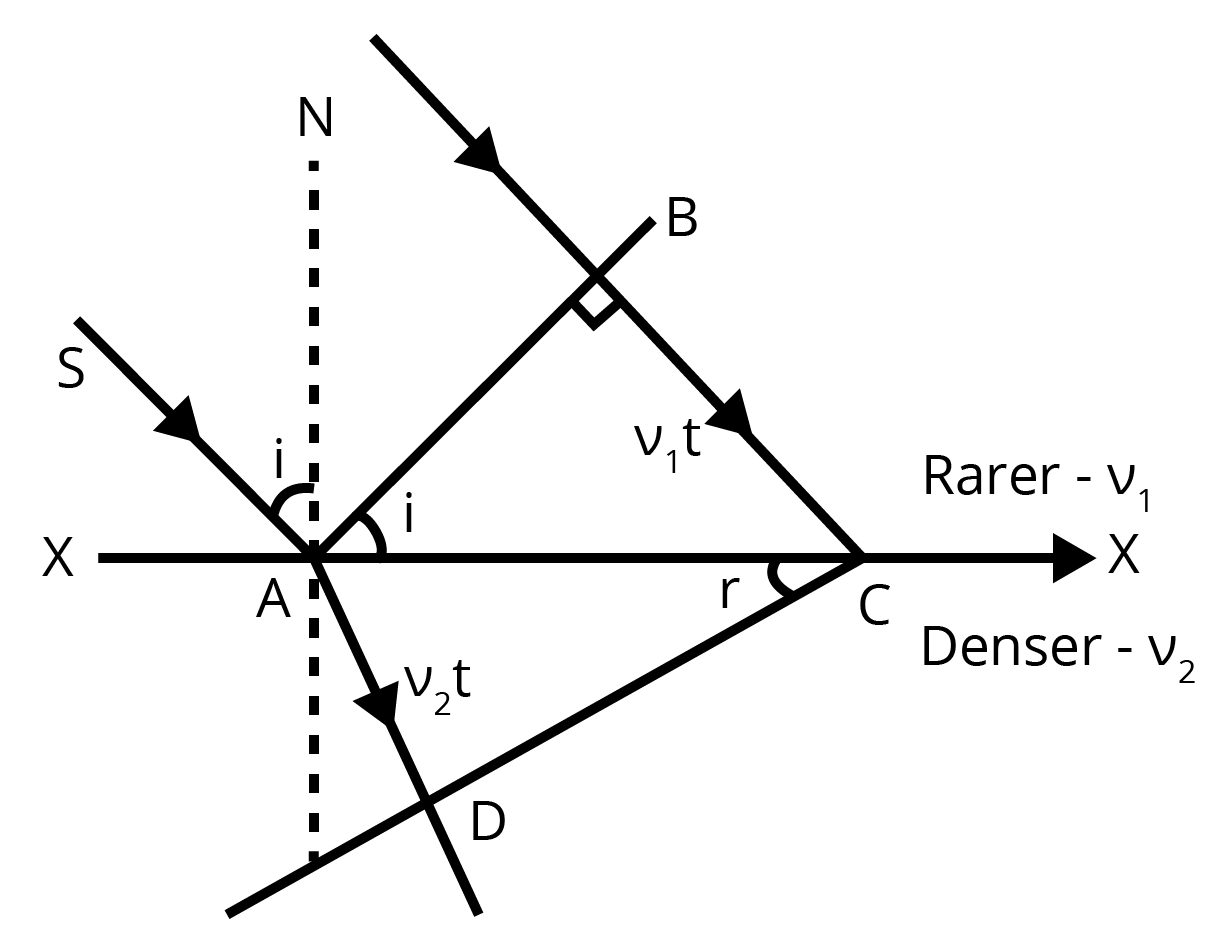
We know that according to Huygen’s principle each point on AC would give rise to secondary wavelets that would start to grow in the second medium. If t is the time taken by the disturbance to reach C from B, 

$BC={{v}_{1}}t$

And, secondary wavelets would spread over a hemisphere of radius, 

$AD={{v}_{2}}t$

Now, the tangent plane CD that is drawn at C will be the new refracted wavefront. 

Let i and r be the angles of incidence and refraction respectively, then, 

In $\Delta ABC$,

$\sin \angle BAC=\sin i=\frac{BC}{AC}$

And for $\Delta ADC$,

$\sin \angle DCA=\sin r=\frac{AD}{AC}$

$\Rightarrow \frac{\sin i}{\sin r}=\frac{BC}{AD}=\frac{{{v}_{1}}t}{{{v}_{2}}t}=\frac{{{v}_{1}}}{{{v}_{2}}}$

$\therefore \frac{\sin i}{\sin r}=\frac{{{v}_{1}}}{{{v}_{2}}}=\mu $

And therefore, we proved Snell’s law. 

6. A young’s double slit experiment using light of wavelength $400nm$, interference fringes of width to $600nm$, and the separation between the slits is halved. If one wants the observed fringe width on the screen to be the same in the two cases, find the ratio of the distance between the screen and the plane of the interference.

Ans: Let ${{D}_{1}}$  be the distance between the screen and the sources, when light of wavelength $400$ nm is used. 

$\beta =\frac{D\alpha }{d}$ 

\[\Rightarrow X=\frac{{{D}_{1}}\times 400\times {{10}^{-9}}}{d}\] ……………………… (1)

In order to obtain the same fringe width 

\[\frac{{{D}_{2}}\times 600\times {{10}^{-9}}}{d}=X\] ………………………….. (2)

From equation (1) and (2),

\[\Rightarrow \frac{{{D}_{1}}}{{{D}_{2}}}=\frac{600\times {{10}^{-9}}}{400\times {{10}^{-9}}}\]

\[\therefore \frac{{{D}_{1}}}{{{D}_{2}}}=1.5\] 

Therefore, the ratio of distance between the given arrangements is found to be$3:2$.

7. In Young’s double slit experiment while using a source of light of wavelength $5000A{}^\circ $, the fringe width obtained is $0.6\text{ cm}$. If the distance between the slit and the screen is reduced to half, calculate the new fringe width. 

Ans:  We are given that,

Wavelength, $\lambda =5000A{}^\circ =5\times {{10}^{-7}}\text{ m}$ 

Fringe width, $\beta =0.6\text{ cm}=0.6\times {{10}^{-2}}\text{ m}$

$\beta =\frac{\lambda D}{d}$ 

$\Rightarrow \frac{\beta }{\lambda }=\frac{D}{d}$

$\Rightarrow \frac{D}{d}=\frac{0.6\times {{10}^{-2}}}{5\times {{10}^{-7}}}$

$\Rightarrow \frac{D}{d}=1.2\times {{10}^{4}}$

Let new Distance $D'=\frac{D}{d}$

Let new fringe width $\beta '=\frac{\lambda D'}{d}=\frac{\lambda D'}{2d}$

Then, 

$\beta '=\frac{5\times {{10}^{-7}}\times 1.2\times {{10}^{4}}}{2}$

$\therefore \beta '=3\times {{10}^{-3}}\text{ m}$

Therefore, the new fringe width will be $3\times {{10}^{-3}}\text{ m}$.

8. What is polarization of light? What type of waves show the property of polarization? Name any two methods to produce plane polarized light.

Ans: The phenomenon by virtue of which the vibrations of a light vector is restricted in a particular direction in a plane perpendicular to the direction of propagation of light is called polarisation of light. Transverse waves are known to show the property of polarisation. Two methods to produce plane polarised light are:

1. Polarisation by Reflection and

2. Polarization by scattering.

9. Draw the curve depicting variation of intensity in the interference pattern in young’s double slit experiment. State conditions for obtaining sustained interference of light? 

Ans:  The curve could be drawn as,

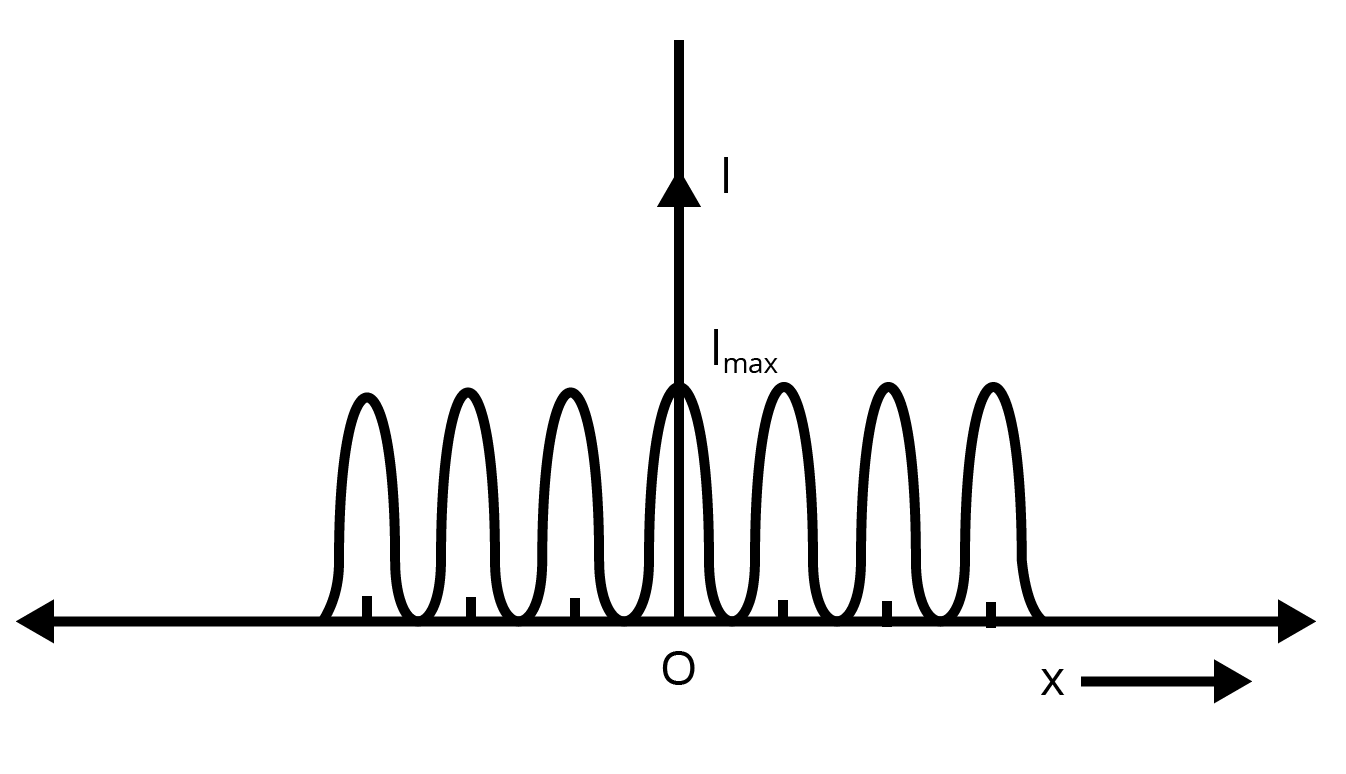
Conditions for sustained interference of light are:

1. Two sources causing the interference must be coherent sources of light. 

2. Two sources causing the interference should have nearly equal amplitudes and intensities and should be monochromatic.

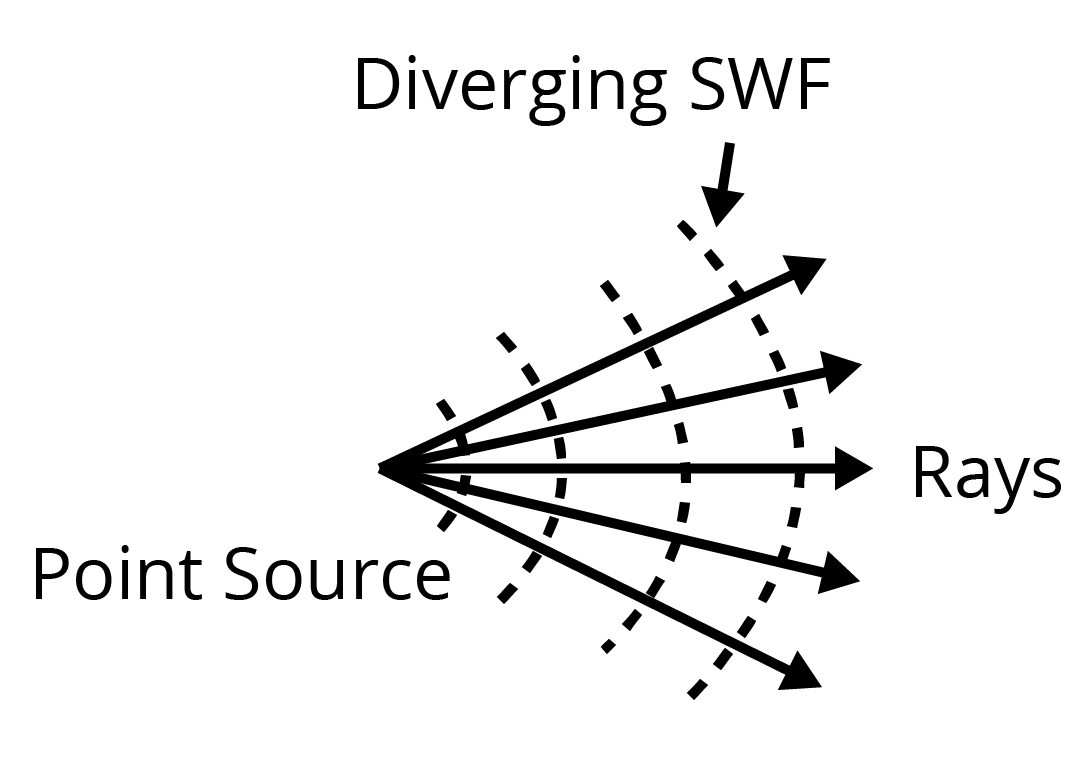
10. What is the shape of the wave front in each of the following cases:

1. Light diverging from a point source. 

Ans: The shape of the wave front in case of a light that is diverging from a point source is spherical. The wave front emanating from a point source would be as shown in the below figure. 

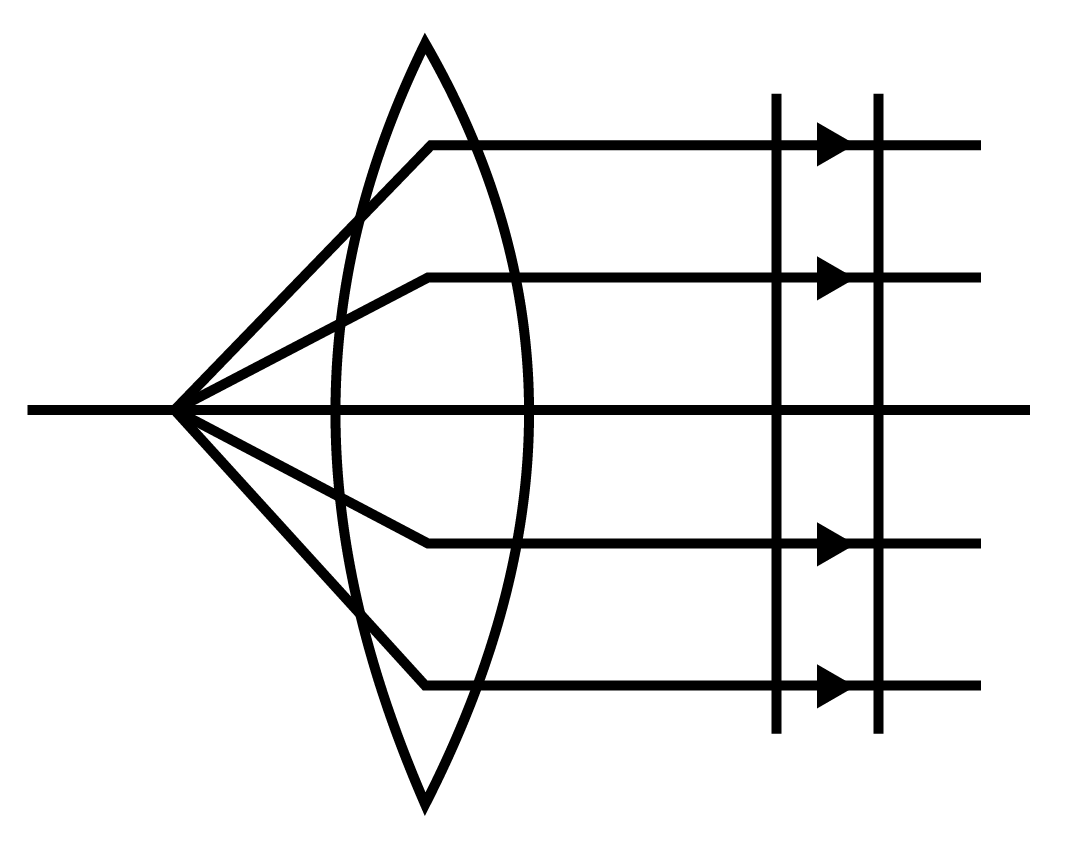
2. Light emerging out of a convex lens when a point source is placed at its focus.

Ans: The shape of the wave front in case of a light emerging out of a convex lens when a point source is placed at its focus would be a parallel grid. This would be as shown in the below figure. 

3. The portion of the wave front of light from a distant star intercepted by the Earth. 

Ans: The portion of the wavefront of light from a distant star intercepted by the Earth would be a plane. 

11.  In a Young's double-slit experiment, the slits are separated by $0.28mm$ and the screen is placed $1.4m$ away. The distance between the central bright fringe and the fourth bright fringe is measured to be $1.2cm$. Determine the wavelength of light used in the experiment.

Ans: We are given, 

Distance between the slits, \[d=0.28\text{ }mm=~0.28\times {{10}^{-3}}m\] 

Distance between the slits and the screen, \[D\text{ }=1.4\text{ }m\] 

Distance between the central fringe and the fourth \[\left( n\text{ }=\text{ }4 \right)\] fringe, 

\[u=1.2cm=1.2\times {{10}^{-2}}~m\] 

In case of a constructive interference, we have the relation for the distance between the two fringes as:

$u=n\lambda \frac{D}{d}$ 

where, 

\[n=\text{Order of fringes}=4\] 

\[\lambda =\text{Wavelength of light used}\] 

$\Rightarrow \lambda =\frac{ud}{nD}$

$\Rightarrow \lambda =\frac{1.2\times {{10}^{-2}}\times 0.28\times {{10}^{-3}}}{4\times 1.4}=6\times {{10}^{-7}}$

$\therefore \lambda =600nm$  

Therefore, the wavelength of the light is found to be \[600\text{ }nm\]. 

12. In a double-slit experiment the angular width of a fringe is found to be $0.2{}^\circ $on a screen placed \[\mathbf{1}\text{ }\mathbf{m}\] away. The wavelength of light used is \[\mathbf{600nm}\] . What will be the angular width of the fringe if the entire experimental apparatus is immersed in water? Take refractive index of water to be \[\frac{4}{3}\] .

Ans: We are given that, 

Distance of the screen from the slits, \[D=1m\]  

Wavelength of light used, ${{\lambda }_{1}}=600nm$ 

Angular width of the fringe in air, ${{\theta }_{1}}=0.2{}^\circ $ 

Angular width of the fringe in water $={{\theta }_{2}}$ 

Refractive index of water, $\mu =\frac{4}{3}$ 

Refractive index is related to angular width as:

$\mu =\frac{{{\theta }_{1}}}{{{\theta }_{2}}}$ 

$\Rightarrow {{\theta }_{2}}=\frac{3}{4}{{\theta }_{1}}$

$\therefore {{\theta }_{2}}=\frac{3}{4}\times 0.2=0.15{}^\circ $

Therefore, the angular width of the fringe in water would reduce to $0.15{}^\circ $ .

13. Light of wavelength \[5000\overset{\bigcirc }{\mathop{\text{A}}}\,\]  falls on a plane reflecting surface. What are the wavelength and frequency of the reflected light? For what angle of incidence is the reflected ray normal to the incident ray? 

Ans: We are given, 

Wavelength of incident light, \[\lambda ,~~=5000~\overset{\bigcirc }{\mathop{\text{A}}}\,=~5000\times {{10}^{-10}}m\]  

Speed of light, \[c=3\times {{10}^{3}}m\]

Frequency of incident light is given by the relation,

$v=\frac{c}{\lambda }$ 

$\Rightarrow v=\frac{3\times {{10}^{8}}}{5000\times {{10}^{-10}}}=6\times {{10}^{14}}Hz$ 

The wavelength and frequency of incident light would be the same as that of reflected ray. So, the wavelength of reflected light is found to be \[5000\overset{\bigcirc }{\mathop{\text{A}}}\,\]  and its frequency would be $6\times {{10}^{14}}Hz$.

When reflected ray is normal to incident ray, the sum of the angle of incidence, $\angle i$   and angle of reflection, $\angle r$  is $90{}^\circ $  .

As per the law of reflection, the angle of incidence is always equal to the angle of reflection. Hence, we can write the sum as:

$\angle i+\angle r=90$

$\Rightarrow \angle i+\angle i=90$

$\therefore \angle i=\frac{90}{2}=45{}^\circ $

Therefore, the angle of incidence for the given condition is found to be $45{}^\circ $ .

14. The $I$ ${{H}_{0}}$ line emitted by hydrogen in a star is found to be red shifted by $15\overset{{}^\circ }{\mathop{\text{A}}}\,$. Estimate the speed with which the star is receding from the Earth. 

Ans: From given data, wavelength of ${{H}_{0}}$  line emitted by hydrogen, 

$\lambda =6563\overset{{}^\circ }{\mathop{\text{A}}}\,=6563\times {{10}^{-10}}m$ 

Star's red-shift, $(\lambda '-\lambda )=15\overset{{}^\circ }{\mathop{\text{A}}}\,=15\times {{10}^{-10}}m$   

Speed of light, $c=3\times {{10}^{8}}m/s$

Let the velocity of the star receding away from the Earth be $v$ . 

The red shift is related with velocity as:

$\lambda '-\lambda =\frac{v}{c}\lambda $

$\Rightarrow v=\frac{c}{\lambda }\times (\lambda '-\lambda )$

$\Rightarrow v=\frac{3\times {{10}^{8}}\times 15\times {{10}^{-10}}}{6563\times {{10}^{-10}}}$ 

$\therefore v=6.87\times {{10}^{5}}m/s$ 

Therefore, the speed with which the star is receding away from the Earth is found to be $6.87\times {{10}^{5}}m/s$.

15. You have learnt in the text how Huygens' principle leads to the laws of reflection and refraction. Use the same principle to deduce directly that a point object placed in front of a plane mirror produces a virtual image whose distance from the mirror is equal to the object distance from the mirror. 

Ans: Let an object at $O$  be placed in front of a plane mirror \[MO'\] at a distance \[r\] (as shown in the given figure).

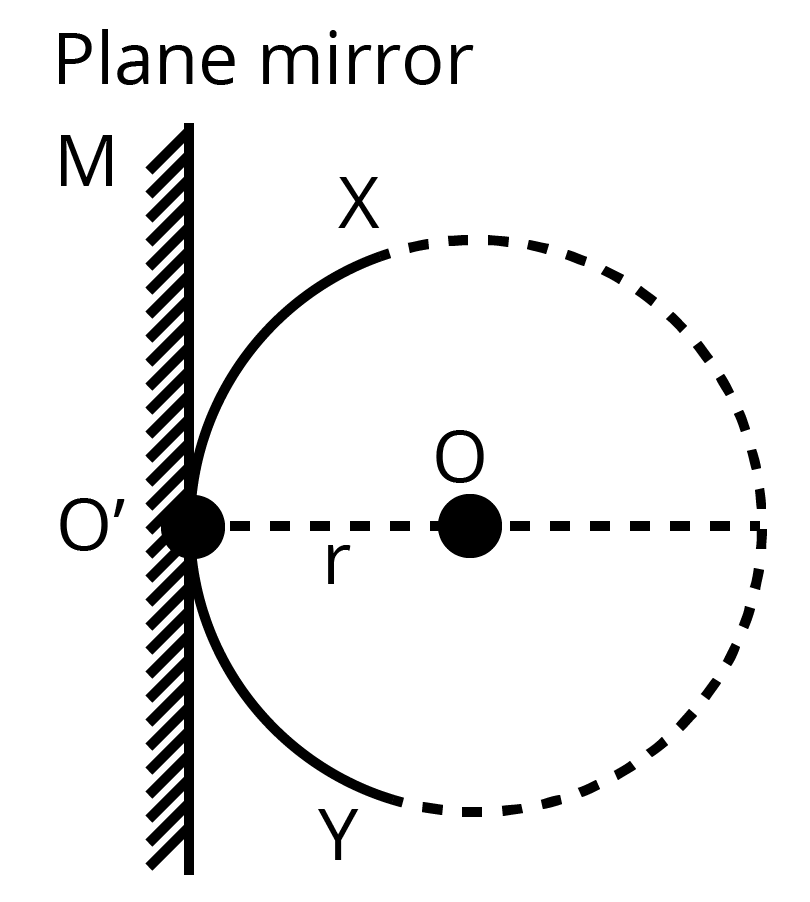
A circle is drawn from the centre \[(O)\]such that it would just touch the plane mirror at point \[O'\]. According to Huygens' Principle, \[XY\] is the wave front of incident light. 

If the mirror is absent, then a similar wave front \[X'Y'\] (as \[XY\]) would form behind \[O'\] at distance \[r\] (as shown in the given figure).

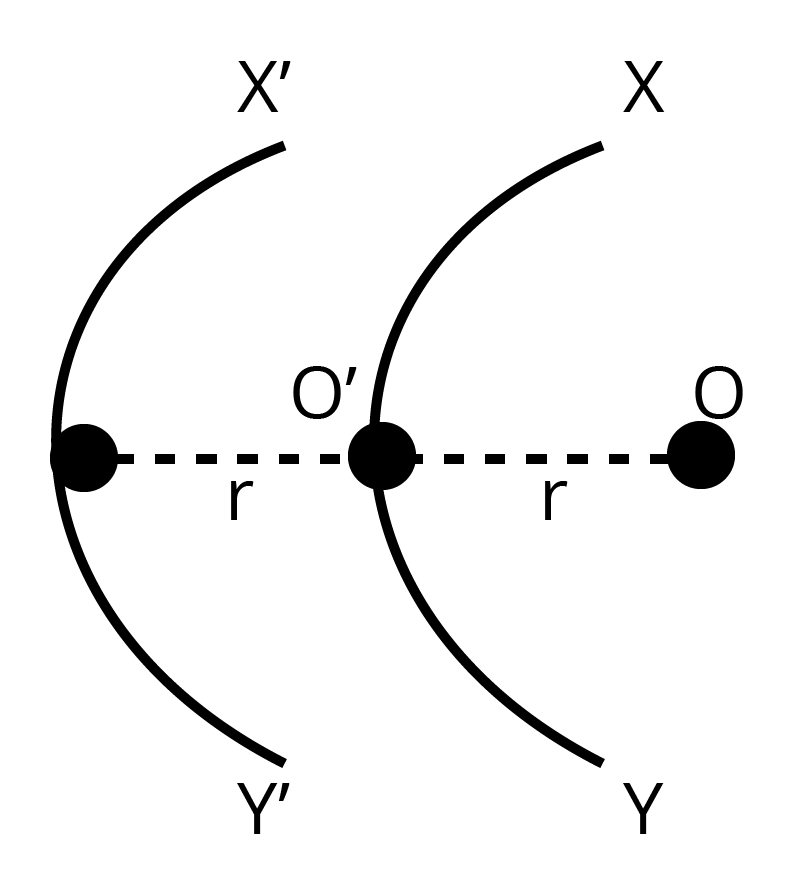
\[X'Y'\] could be considered as a virtual reflected ray for the plane mirror.  Therefore, a point object placed in front of the plane mirror would produce a virtual image whose distance from the mirror is equal to the object distance (\[r\]). 

16. A parallel beam of light of wavelength \[\mathbf{500}\text{ }\mathbf{nm}\] falls on a narrow slit and the resulting diffraction pattern is observed on a screen \[\mathbf{1}\text{ }\mathbf{m}\] away. It is observed that the first minimum is at a distance of \[\mathbf{2}.\mathbf{5}\text{ }\mathbf{nm}\]  from the centre of the screen. Find the width of the slit. 

Ans: We are given that, 

Wavelength of light beam, $\lambda ~=500\text{ }nm=~~500\times {{10}^{-9}}m$ 

Distance of the screen from the slit, \[D=1m\] 

For first minima, \[n=1\] 

Distance between the slits $=d$ 

Distance of the first minimum from the centre of the screen can be obtained as: \[x=2.5mm=2.5\times {{10}^{-3}}m~\] 

It is related to the order of minima as:

$n\lambda =x\frac{d}{D}$ 

$\Rightarrow d=\frac{n\lambda D}{x}$ 

\[\Rightarrow d=\frac{1\times 500\times {{10}^{-9}}\times 1}{2.5\times {{10}^{-3}}}=2\times {{10}^{-4}}m=0.2mm\] 

Therefore, the width of the slits is found to be \[0.2mm\]. 

Long Questions and Answers (5 Marks Questions)

1.

1. State Huygens’s principle for constructing wave fronts. 

Ans: According to Huygens’s principle:

1. Each source of light would spread waves in all directions.

2. Each point on the wave front would give rise to new disturbance which in-turn produces secondary wavelets which travel with the speed of light.

3. Only the forward envelope which encloses the tangent would give the new position of wave front.

4. Rays are always found to be perpendicular to the wave front.

2. Using Huygens’s principle, deduce the laws of reflection of light.

Ans: A plane wave front \[AB\] incident at \[A\] hence every point on \[AB\] gives rise to new waves. Time taken by the ray to reach from \[P\] to \[R\].

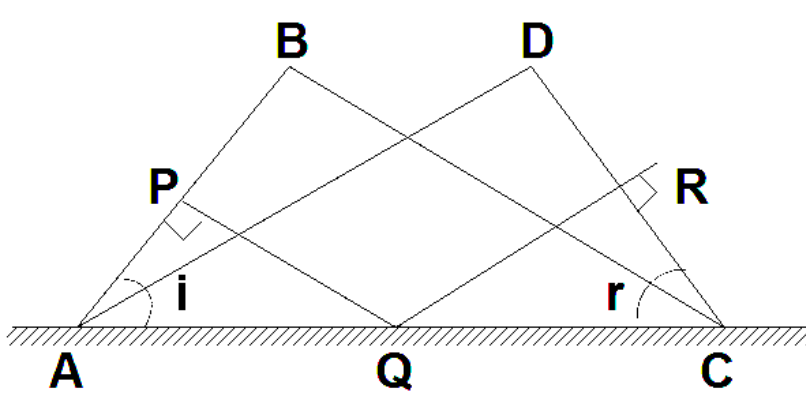
$t=\frac{PQ}{v}+\frac{QR}{v}$ ……………….. (1)

In $\Delta PAQ$ , $\sin i=\frac{PQ}{AQ}$ 

$\Rightarrow PQ=AQ\sin i$

In $\Delta RCQ$ , $\sin r=\frac{QR}{QC}$ 

$\Rightarrow QR=QC\sin r$

Substituting in equation (1), 

$\Rightarrow t=\frac{AQ\sin i}{v}+\frac{(AC-AQ)\sin r}{v}$

$\Rightarrow t=\frac{AQ\sin i}{v}+\frac{AC\sin r}{v}-\frac{AQ\sin r}{v}$

$\Rightarrow t=\frac{AQ(\sin i-\sin r)}{v}+\frac{AC\sin r}{v}$ 

Since all the secondary wavelets takes the same time to go from the incident wave front to the reflected wave front hence it must be independent of $Q$ 

 i.e., \[\sin i\sin r=0\]

$\therefore \sin i=\sin r$ 

or $i=r$ (Law of Reflection of light)

3. What changes in diffraction pattern of a single slit will you observe when the monochromatic source of light is replaced by a source of white light? 

Ans: The changes would be:

1) The diffracted light consists of different colours.

2) It results in overlapping of different colours. 

2. 

1. Coloured spectrum is seen when we look through a muslin cloth. Why?

Ans: Muslin cloth is known to consist of very fine threads which acts as fine slits and when light passes through it, light would get diffracted giving rise to a coloured spectrum. 

2. What changes in diffraction pattern of a single slit will you observe when the monochromatic source of light is replaced by a source of white light? 

Ans: The changes are: 

1. Diffracted lights consist of different colours. 

2. It would result in overlapping of different colours. 

3. A slit of width $'a'$  is illuminated by light of wavelength $6000\overset{\bigcirc }{\mathop{\text{A}}}\,$ . For what value of $'a'$ will the:

1. First maximum fall at an angle of diffraction of  $30{}^\circ $ ? 

Ans: We are given:

$\lambda =6000\overset{\bigcirc }{\mathop{\text{A}}}\,=6000\times {{10}^{-10}}m$

${{\theta }_{1}}=30{}^\circ ,m=1$ 

For first maximum

$\sin {{Q}_{m}}=\frac{\left( m+\frac{1}{2} \right)\lambda }{a}$ 

$\Rightarrow \sin {{Q}_{1}}=\frac{3\lambda }{2a}$

or $a=\frac{3\lambda }{2\sin {{\theta }_{1}}}=\frac{3\times 6\times {{10}^{-7}}}{2\times \sin 30{}^\circ }$

2. First minimum fall at an angle of diffraction $30{}^\circ $. 

Ans: For first minimum 

\[\sin {{Q}_{m}}=\frac{m\lambda }{a}\]

Or $\sin {{Q}_{1}}=\frac{\lambda }{a}$

$\Rightarrow a=\frac{\lambda }{\sin {{\theta }_{1}}}$

$\Rightarrow a=\frac{6\times {{10}^{-7}}}{\sin 30{}^\circ }$

$\therefore a=1.2\times {{10}^{-5}}m$

4.  

1. Derive all expressions for the fringe width in Young’s double slit experiment. 

Ans: Path difference between 

${{S}_{1}}P$  and ${{S}_{2}}P$

$\Delta x={{S}_{2}}P-{{S}_{1}}P$…………………………… (A)

In $\Delta {{S}_{2}}P$, 

$\Rightarrow ({{S}_{2}}P)={{\left[ {{\left( {{S}^{2}}B \right)}^{2}}+\left( P{{B}^{2}} \right) \right]}^{\frac{1}{2}}}$…………………………… (1)

${{S}_{2}}P=D{{\left[ 1+\frac{\left( y+\frac{d}{2} \right)}{{{D}^{2}}} \right]}^{\frac{1}{2}}}$

Using Binomial theorem expand equation$(1)$ and then neglect higher terms to get,  

${{S}_{2}}P=D+\frac{{{\left( y+\frac{d}{2} \right)}^{2}}}{2D}$

Similarly,  ${{S}_{1}}P=D+\frac{{{\left( y-\frac{d}{2} \right)}^{2}}}{2D}\cdots (2)$

Substituting equation (1) and (2) in equation $(\text{A)}$, 

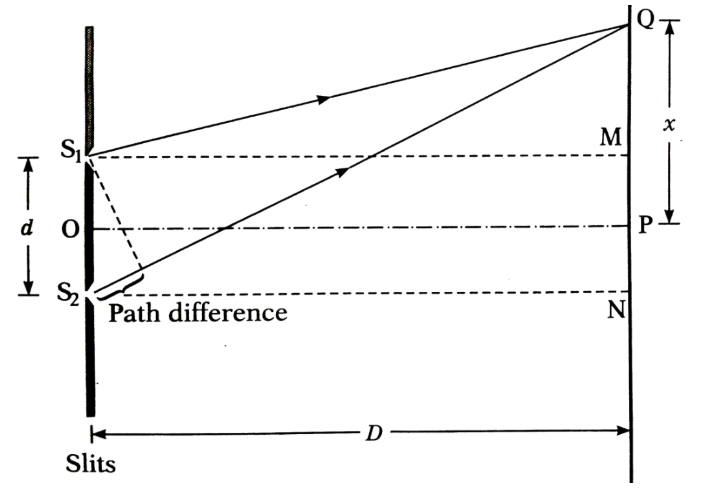
$\Rightarrow \Delta x=\frac{{{\left( y+\frac{d}{2} \right)}^{2}}-{{\left( y-\frac{d}{2} \right)}^{2}}}{2D}$

$\Rightarrow \Delta x=\frac{{{y}^{2}}+{{\frac{d}{4}}^{2}}+yd-{{y}^{2}}-{{\frac{d}{4}}^{2}}+yd}{2D}$

$\Delta x=\frac{2yd}{2D}$

$\Delta x=\frac{yd}{D}$

For bright fringes 

Path difference $=x\lambda $ 

$x\lambda =\frac{yd}{D}$

\[\Rightarrow y=\frac{x\lambda D}{d}\]

For $m=1,\text{ }{{y}_{1}}=\frac{\lambda D}{d}$ 

$n=2,\text{ }{{y}_{2}}=\frac{\lambda D}{d}$

For fringe width, 

$\beta ={{y}_{2}}-{{y}_{1}}$ 

$\therefore \beta =\frac{\lambda d}{d}$

2. If the two slits in Young’s double slit experiment have width ratio \[\mathbf{4}:\mathbf{1}\] , deduce the ratio of intensity of maxima and minima in the interference pattern. 

Ans: From the given ratio, $\frac{a_{1}^{2}}{a_{2}^{2}}=\frac{{{w}_{1}}}{{{w}_{2}}}=\frac{4}{1}$ 

\[\frac{{{a}_{1}}}{{{a}_{2}}}=\frac{2}{1}\]

Or \[{{a}_{1}}=2{{a}_{2}}\] 

Using  \[\frac{{{I}_{\max }}}{{{I}_{\min }}}=\frac{{{\left( {{a}_{1}}+{{a}_{2}} \right)}^{2}}}{{{\left( {{a}_{1}}-{{a}_{2}} \right)}^{2}}}\] 

\[\Rightarrow \frac{{{I}_{\max }}}{{{I}_{\min }}}=\frac{{{\left( 2{{a}_{2}}+{{a}_{2}} \right)}^{2}}}{{{\left( 2{{a}_{2}}-{{a}_{2}} \right)}^{2}}}={{\left( \frac{3{{a}_{2}}}{{{a}_{2}}} \right)}^{2}}\]

\[\therefore \frac{{{I}_{\max }}}{{{I}_{\min }}}=\frac{9}{1}\]

Therefore, the ratio of intensity of maxima and minima in the interference pattern is $9:1$.

5. Monochromatic light of wavelength \[\mathbf{589}\text{ }\mathbf{nm}\]is incident from air on a water surface. What are the wavelength, frequency and speed of : 

1. Reflected, and

(Refractive index of water is \[\mathbf{1}.\mathbf{33}\]) 

Ans: We are given, 

Wavelength of incident monochromatic light, $\lambda =589nm=589\times {{10}^{-9}}m$ 

Speed of light in air, $c=3\times {{10}^{8}}m/s$

Refractive index of water, $\mu =1.33$  

The ray would reflect back in the same medium as that of the incident ray. Hence, the wavelength, speed, and frequency of the reflected ray will be the same as that of the incident ray. 

Frequency of light is given by the relation, 

$v=\frac{c}{\lambda }$ 

$\Rightarrow v=\frac{3\times {{10}^{8}}}{5000\times {{10}^{-10}}}=6\times {{10}^{14}}Hz$ 

$\angle i+\angle r=90{}^\circ $ 

$\Rightarrow \angle i+\angle i=90{}^\circ $

$\Rightarrow {{Z}_{F}}=\frac{{{\left( 4\times {{10}^{-3}} \right)}^{2}}}{400\times {{10}^{-9}}}=40m$

$\Rightarrow (\lambda '-\lambda )=15\overset{\bigcirc }{\mathop{\text{A}}}\,=15\times {{10}^{-10}}m$

$\Rightarrow \lambda '-\lambda =\frac{v}{c}\lambda $ 

$\Rightarrow v=\frac{c}{\lambda }\times (\lambda '-\lambda )$ 

Also, $\frac{v}{c}=\frac{\sin i}{\sin r}=\mu $

$\Rightarrow \theta =0.1{}^\circ =0.1\times \frac{\lambda }{180}=\frac{3.14}{1800}rad$

And, $d=\frac{\lambda }{\theta }$ 

$\Rightarrow d=\frac{600\times {{10}^{-9}}}{\frac{3.14}{1800}}=3.44\times {{10}^{-4}}m$ 

$\Rightarrow \lambda =\frac{{{a}^{2}}}{{{Z}_{F}}}$ 

Now, we have, $n\lambda =x\frac{d}{D}$ 

$\Rightarrow d=\frac{n\lambda D}{d}$

$\Rightarrow \theta =\frac{\frac{d}{d}\lambda }{d}=\frac{\lambda }{d}$

$\Rightarrow \theta =\frac{3\times {{10}^{8}}}{589\times {{10}^{-9}}}$ 

$\therefore \theta =5.09\times {{10}^{14}}Hz$

Hence, the speed, frequency, and wavelength of the reflected light are found to be $3\times {{10}^{8}}m$,  $5.09\times {{10}^{14}}Hz$, and $589nm$ respectively. 

2. refracted light?

Ans: Frequency of light does not depend on the property of the medium in which it is travelling. So, the frequency of the refracted ray in water will be equal to the frequency of the incident or reflected light in air. 

We have the refracted frequency to be $5.09\times {{10}^{14}}Hz$

Speed of light in water is related to the refractive index of water as:

$V=\frac{c}{\mu }$ 

$\Rightarrow V=\frac{3\times {{10}^{8}}}{1.33}=2.26\times {{10}^{8}}m/s$ 

Wavelength of light in water is given by the relation,

$\lambda =\frac{V}{v}$  

$\Rightarrow \lambda =\frac{2.26\times {{10}^{8}}}{5.09\times {{10}^{14}}}$ 

$\Rightarrow \lambda =444.007\times {{10}^{-9}}m$ 

$\therefore \lambda =444.01nm$ 

Hence, the speed, frequency, and wavelength of refracted light are found to be$2.26\times {{10}^{8}}m/s$ , $444.01nm$  and $5.09\times {{10}^{14}}Hz$respectively.

6. In Young's double-slit experiment using monochromatic light of wavelength  $\lambda $ , the intensity of light at a point on the screen where path difference is $\lambda $ , is $K$ units. What is the intensity of light at a point where path difference is   $\lambda /3$? 

Ans: Let ${{I}_{1}}$and ${{I}_{2}}$ be the intensities of the two light waves. Their resultant intensities can be obtained as: 

$I'={{I}_{1}}+{{I}_{2}}+2\sqrt{{{I}_{1}}{{I}_{2}}}\cos \phi $

Where, 

$\phi =$ Phase difference between the two waves 

For monochromatic light waves, 

${{I}_{1}}={{I}_{2}}$ 

$\Rightarrow I'={{I}_{1}}+{{I}_{1}}+2\sqrt{{{I}_{1}}{{I}_{1}}}\cos \phi $

$\Rightarrow I'=2{{I}_{1}}+2{{I}_{1}}\cos \phi $

Phase difference $=\frac{2\pi }{\lambda }\times \text{ Path difference}$ 

Since path difference $=\lambda $ , 

Phase difference,  $\phi =2\pi $ 

$\therefore I=2{{I}_{1}}+2{{I}_{1}}=4{{I}_{1}}$

Given, 

\[I'=K\] 

$\therefore I'=\frac{K}{4}$ ………………………. (1)

When path difference $=\frac{\lambda }{3}$ , 

Phase difference, $\phi =\frac{2\pi }{3}$  

Hence, resultant intensity, ${{I}_{R}}'={{I}_{1}}+{{I}_{1}}+2\sqrt{{{I}_{1}}{{I}_{1}}}\cos \frac{2\pi }{3}$

$\Rightarrow {{I}_{R}}'=2{{I}_{1}}+2{{I}_{1}}\left( -\frac{1}{2} \right)={{I}_{1}}$

Using equation $(1)$, we can write:

${{I}_{R}}={{I}_{1}}=\frac{K}{4}$

Therefore, the intensity of light at a point where the path difference is $\frac{\lambda }{3}$ is found to be $\frac{K}{4}$  units.

7. A beam of light consisting of two wavelengths, \[\mathbf{650}\text{ }\mathbf{nm}\] and\[\mathbf{520}\text{ }\mathbf{nm}\], is used to obtain interference fringes in a Young's double-slit experiment. 

1. Find the distance of the third bright fringe on the screen from the central maximum for wavelength \[\mathbf{650}\text{ }\mathbf{nm}\] .

Ans: We have the expression for fringe width as, 

$x=\frac{D\lambda }{d}$

As per the given conditions, $x=3\times 650\frac{D}{d}=1950\left( \frac{D}{d} \right)nm$ 

Also, $n{{\lambda }_{2}}=(n-1){{\lambda }_{1}}$

But, ${{\lambda }_{1}}=650nm$

$\therefore n=5$ 

Wavelength of another light beam, ${{\lambda }_{2}}=520nm$

Distance of the slits from the screen \[=D\] 

Distance between the two slits $=d$ 

Distance of the nth bright fringe on the screen from the central maximum is given by the relation,

$x=n{{\lambda }_{1}}\left( \frac{D}{d} \right)$ 

For third bright fringe,$N=3$  

$\therefore x=3\times 650\frac{D}{d}=1950\left( \frac{D}{d} \right)nm$

2. What is the least distance from the central maximum where the bright fringes due to both the wavelengths coincide? ${{\lambda }_{1}}=\mathbf{600}\text{ }\mathbf{nm}$ 

Ans: Let the ${{n}^{th}}$ bright fringe due to wavelength ${{\lambda }_{2}}$ and $(n-1)$th bright fringe due to wavelength ${{\lambda }_{1}}$coincide on the screen. We can equate the conditions for bright fringes as:

$n{{\lambda }_{2}}=(n-1){{\lambda }_{1}}$ 

\[\Rightarrow 520n=650n-650\]

\[\Rightarrow 650=130n\]

$\therefore n=5$ 

Hence, the least distance from the central maximum can be obtained by the relation: 

$x={{\lambda }_{2}}\left( \frac{D}{d} \right)$

$\therefore x=5\times 520\frac{D}{d}=260\frac{D}{d}nm$

Note: The value of $d$ and $D$ are not given in the question. 

8. Explain how Corpuscular theory predicts the speed of light in a medium, say, water, to be greater than the speed of light in vacuum. Is the prediction confirmed by experimental determination of the speed of light in water? If not, which alternative picture of light is consistent with experiment? 

Ans: No; It is wave theory. 

We know that Newton's corpuscular theory of light states that when light corpuscles strike the interface of two media from a rarer (air) to a denser (water) medium, the particles experience forces of attraction normal to the surface. Thus, the normal component of velocity increases while the component along the surface remains unchanged. 

So, we can write the expression: 

\[C\text{ }sin\text{ }i=v\text{ }sin\text{ }r\] …………………. (1)

Where, 

\[i=\text{Angle of incidence}\] 

\[r=\text{Angle of reflection}\] 

\[c=\text{Velocity of light in air}\] 

\[v=\text{Velocity of light in water}\] 

We have the relation for relative refractive index of water with respect to air given as: 

$\mu =\frac{v}{c}$ 

Hence, equation (1) would reduce to

$\frac{v}{c}=\frac{\sin i}{\sin r}=\mu $ ……………………… (2)

But, \[\mu >1\]  

So, it can be inferred from equation (2) that\[v>c\] . This is not possible since this prediction is opposite to the experimental results of \[c>v.\] 

Therefore, we found that the wave picture of light is consistent with the experimental results. 

9. Answer the following questions:

1. In a single slit diffraction experiment, the width of the slit is made double the original width. How does this affect the size and intensity of the central diffraction band? 

Ans: In the case of a single slit diffraction experiment, if the width of the slit is made double the original width, then the size of the central diffraction band would reduce to half and the intensity of the central diffraction band would increase up to four times. 

2. In what way is diffraction from each slit related to the interference pattern in a double-slit experiment? 

Ans: The interference pattern in a double-slit experiment could be modulated by diffraction from each slit. The pattern would be the result of the interference of the diffracted wave from each slit.

3. When a tiny circular obstacle is placed in the path of light from a distant source, a bright spot is seen at the centre of the shadow of the obstacle. Explain why?

Ans: When a tiny circular obstacle is placed in the path of light from a distant source, a bright spot could be seen at the centre of the shadow of the obstacle. This is because light waves are being diffracted from the edge of the circular obstacle, which would interfere constructively at the centre of the shadow. This constructive interference would produce a bright spot.

4. Two students are separated by a \[\mathbf{7}\text{ }\mathbf{m}\] partition wall in a room \[\mathbf{10}\text{ }\mathbf{m}\] high. If both light and sound waves can bend around obstacles, how is it that the students are unable to see each other even though they can converse easily?

Ans: Bending of waves by obstacles by a large angle could be possible when the size of the obstacle is comparable to the wavelength of the waves. On the one hand, the wavelength of the light waves is too small when compared to the size of the obstacle. Thus, the diffraction angle would be very small. Hence, the students would be unable to see each other. On the other hand, the size of the wall is seen to be comparable to that of the wavelength of the sound waves. Thus, the bending of the waves would take place at a large angle. Hence, the students are able to hear each other.

5. Ray optics is based on the assumption that light travels in a straight line. Diffraction effects (observed when light propagates through small apertures/slits or around small obstacles) disprove this assumption. Yet the ray optics assumption is so commonly used in understanding location and several other properties of images in optical instruments. What is the justification? 

Ans: The justification here is that in ordinary optical instruments, the size of the aperture involved would be much larger than the wavelength of the light used. 

10. Two towers on top of two hills are \[\mathbf{40}\text{ }\mathbf{km}\]apart. The line joining them passes \[\mathbf{50}\text{ }\mathbf{m}\] above a hill halfway between the towers. What is the longest wavelength of radio waves, which can be sent between the towers without appreciable diffraction effects? 

Ans: We are given that, 

Distance between the towers, \[d=40km\] 

Height of the line joining the hills, \[d=50m\]. 

Thus, the radial spread of the radio waves should not exceed \[50km\]. 

Since the hill is located halfway between the towers, Fresnel's distance can be obtained as: ${{Z}_{p}}=20km=2\times {{10}^{4}}m$

Aperture can be taken as: 

\[a=d=50m\]

Fresnel's distance is given by the relation,

${{Z}_{p}}=\frac{{{a}^{2}}}{\lambda }$ 

Where,

$\lambda =$ Wavelength of radio waves 

$\Rightarrow \lambda =\frac{{{a}^{2}}}{{{Z}_{p}}}$ 

$\Rightarrow \lambda =\frac{{{(50)}^{2}}}{2\times {{10}^{4}}}=1250\times {{10}^{-4}}=0.1250m$ 

$\therefore \lambda =12.5cm$

Therefore, the wavelength of the radio waves is found to be \[12.5\text{ }cm\].

Download Important Questions For Class 12 Physics Chapter 10 PDF

Wave Optics Class 12 Important Questions

Wave optics chapter is one of those chapters in Class 12 Physics students find difficult to understand. The chapter includes many illustrative diagrams, Experiments, and their experimental setups, the idea of the nature of lights, and many more. The best way of learning optics, in particular, is by visualizing the light and phenomenons of life. Students who are aiming for the CBSE board exams and entrance examinations should not skip this unit.

For solving Class 12 Physics Chapter 10 important questions, students must be well versed with the concepts like Huygen’s principle, the principle of superposition, interference of light, Young’s double-slit experiment, etc. Students seeking detailed notes of this chapter are advised to download Revision notes available on the Vedantu website. 

There are numerous interesting topics to be studied in this chapter. Students should prepare for their upcoming examinations with Class 12 Physics Chapter 10 extra questions for securing good grades. There are some key points to be recalled before starting with wave optics Class 12 important questions given as follows:

• Wave optics mainly focuses on the wave nature of light. We study the phenomenon of light such as interference of light, the reflection of light, refraction of light, etc.

• In Chapter 10 Wave optics we learn about how to determine the phase difference between two wavefronts. Position of the wavefronts by using Huygen’s principle.

• In the wave optics chapter, we learn the concepts that play a vital role in explaining the wave nature of the light like the Superposition principle, definitions, and differences of phase difference, and path differences.

• We define the Inference of light with types of interference, and one of the important results of interference of light i.e., Young’s Double-slit experiment.

These are some key points to be remembered for solving Class 12 Physics wave optics important questions. Students are advised to learn every bit of the chapter for a better understanding. 

Conclusion

Vedantu is one of the leading online learning websites available. Many students have benefited from the free material available on the Vedantu platform. The Vedantu website consists of many study material for most of the subjects, register today for the Vedantu and have access to many more extended study materials for boosting your preparation.

The Class 12 Physics Chapter 10 important questions PDF covered different patterns of questions like MCQs, numerical type, diagrams, etc. The important questions for Class 12 Physics wave optics material provided include all the topics and the subtopics as prescribed by the NCERT syllabus with detailed explanations for student’s clear understanding.

Important Related Links for CBSE Class 12 Physics