CBSE Class 7 Maths Chapter 12 Important Questions Symmetry - Free PDF Download
FAQs on Important Questions for CBSE Class 7 Maths Chapter 12 - Symmetry
Q1. Do I need to practice all the questions provided in the Important Questions for Chapter 12 Symmetry of Class 7 Mathematics?
Ans: Yes, students should practice all the questions from the important questions PDF provided by Vedantu. This will help the students to understand the concepts clearly. Practising once or twice will be a quick revision of all the concepts and also students will know if they have difficulty in understanding any problem. For some problems, students are required to draw diagrams to get clarity on the questions while for few problems, students should use their logical and analytical skills to solve them.
Q2. How many questions are there in Chapter 12 Symmetry of Class 7 Mathematics of NCERT textbook?
Ans: There are a total of 19 questions provided in the NCERT textbook of Chapter 12 Symmetry of Class 7 Mathematics. The students will find 12 questions that are relatively easier to solve, four questions that are moderately easy and finally, three questions in which students would have to think logically to solve. The final three questions might take a bit longer. Vedantu’s Important Questions will help you to solve all these problems.
Q3. What is symmetry in Chapter 12 Symmetry of Class 7 Mathematics?
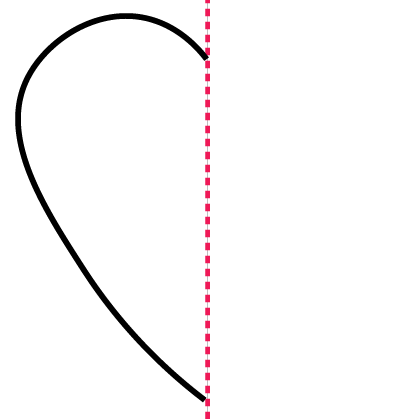
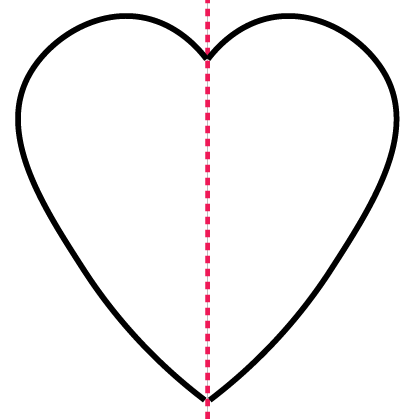
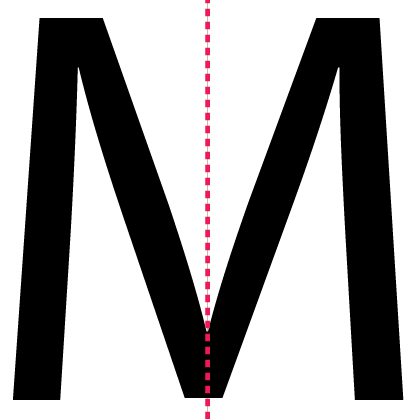
Ans: In Maths, symmetry is an important geometrical concept. It is said to be symmetrical if two or more parts of a figure or shape are identical after folding or flipping. It becomes symmetrical only when the two halves of a shape have the same shape and size. They basically form a mirror image of each other. It becomes asymmetrical if the shape is not symmetrical. Examples of symmetric figures are square, pentagon, hexagon etc.
Q4. Are Important Questions for Chapter 12 Symmetry of Class 7 Mathematics important from the exam point of view?
Ans: Yes. To get high grades in the board exams, all the questions from the Important Questions PDF for Chapter 12 Symmetry of Class 7 Mathematics are important. So, students must practise all the questions from the Important Questions for Chapter 12 Symmetry of Class 7 Mathematics to score really good marks. These notes provided by Vedantu are very accurate and are detailed. It will help the students to understand the concepts and analyse the types of questions that would appear in the exam.
Q5. Why should students download Important Questions for Chapter 12 Symmetry of Class 7 Mathematics from Vedantu?
Ans: Vedantu provides students with the most accurate Important Questions that are most likely to be asked in the exam in the Important Questions for Chapter 12 Symmetry of Class 7 Mathematics PDF. These notes can be viewed online and can also be downloaded in PDF format from the official website of Vedantu or the Vedantu App. They provide all the material free of cost. The concepts for this chapter are explained in detail very clearly by the Maths experts. They also provided neat diagrams wherever necessary.