CBSE Class 8 Maths Chapter 3 Important Questions Understanding Quadrilaterals - FREE PDF Download
FAQs on CBSE Class 8 Maths Important Questions Chapter 3 - Understanding Quadrilaterals
1. What is a quadrilateral?
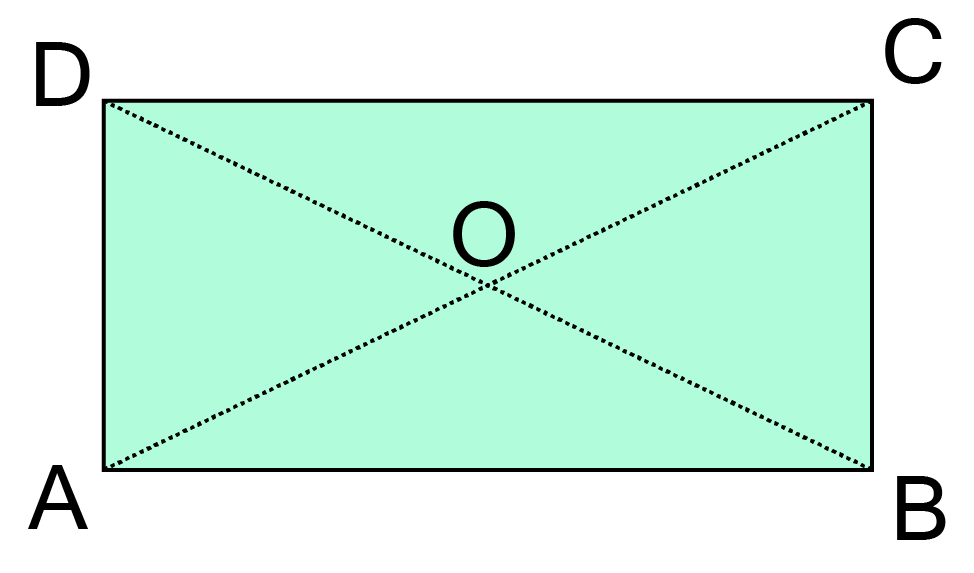
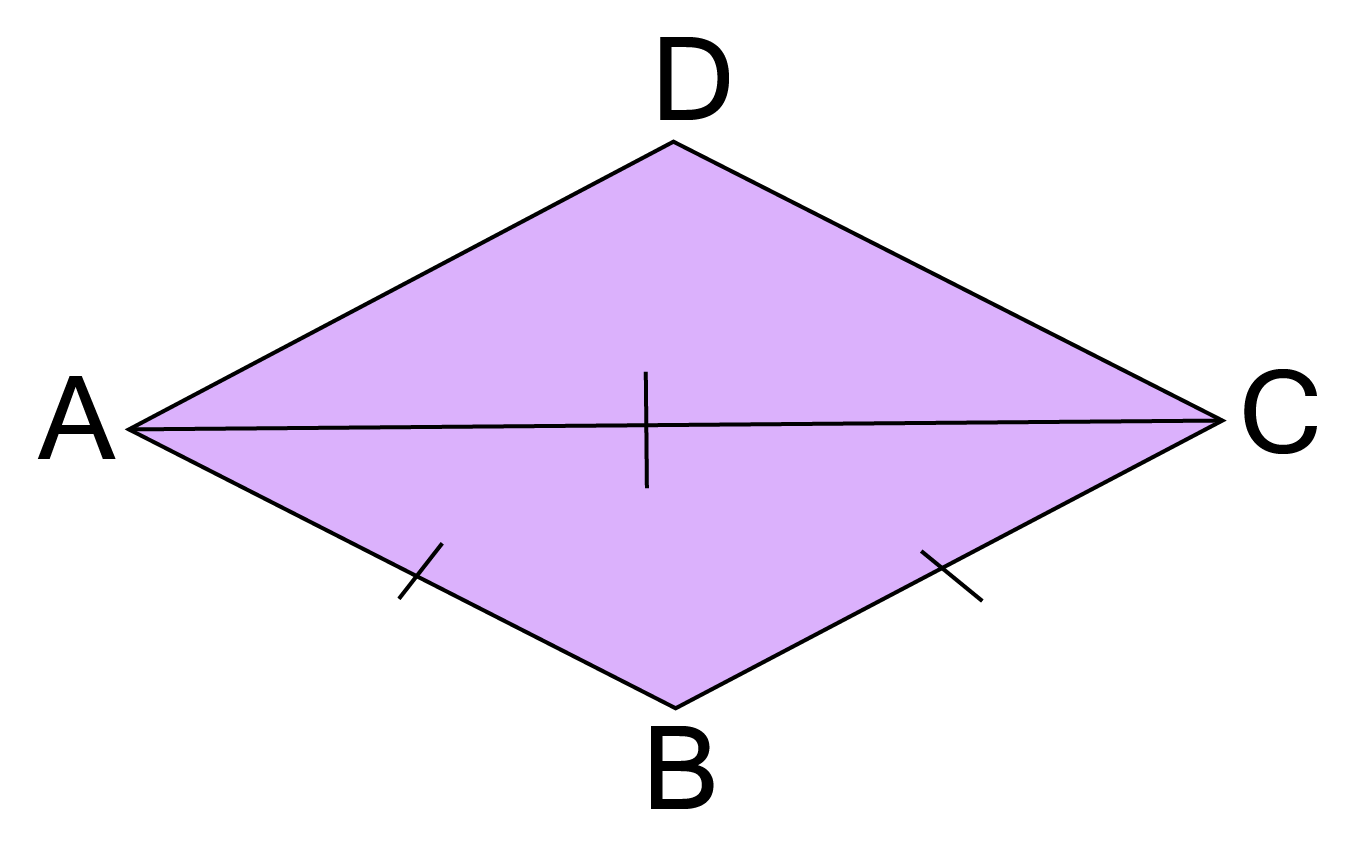
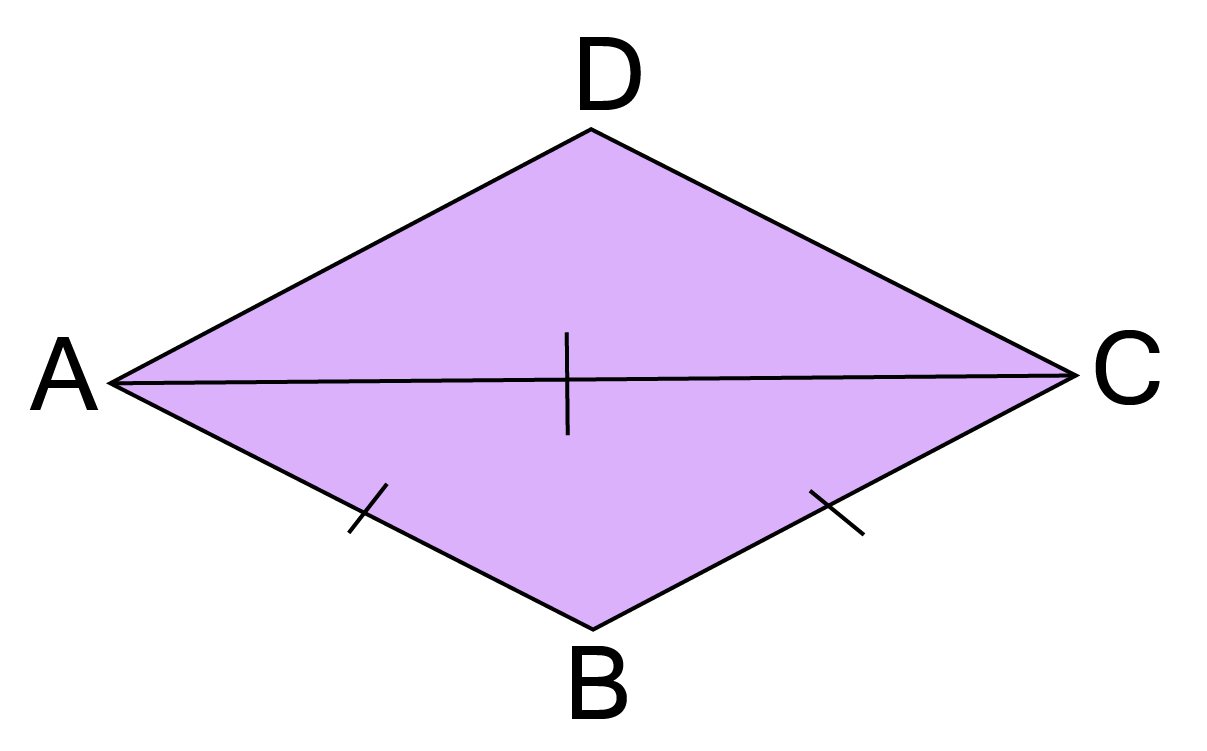
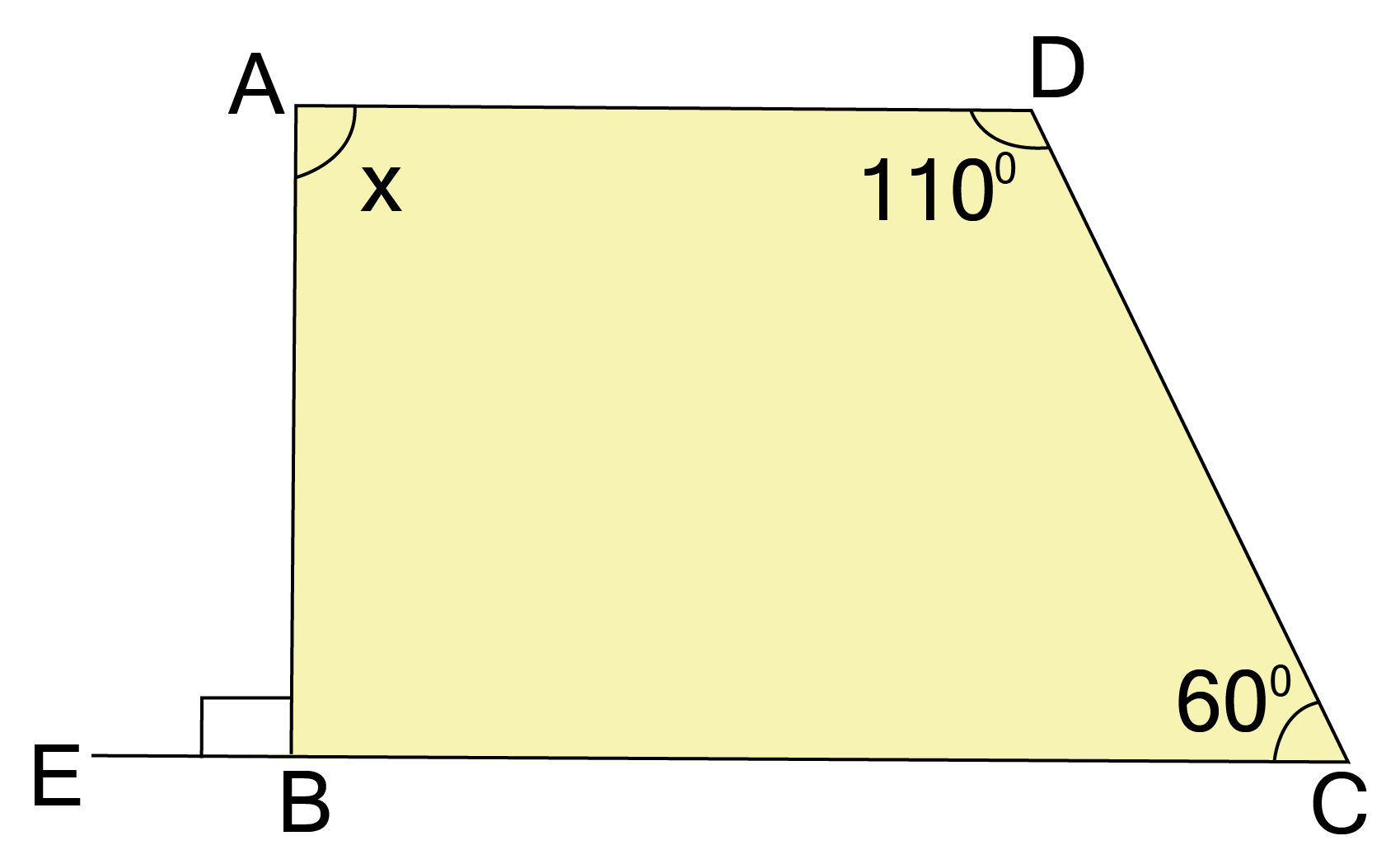
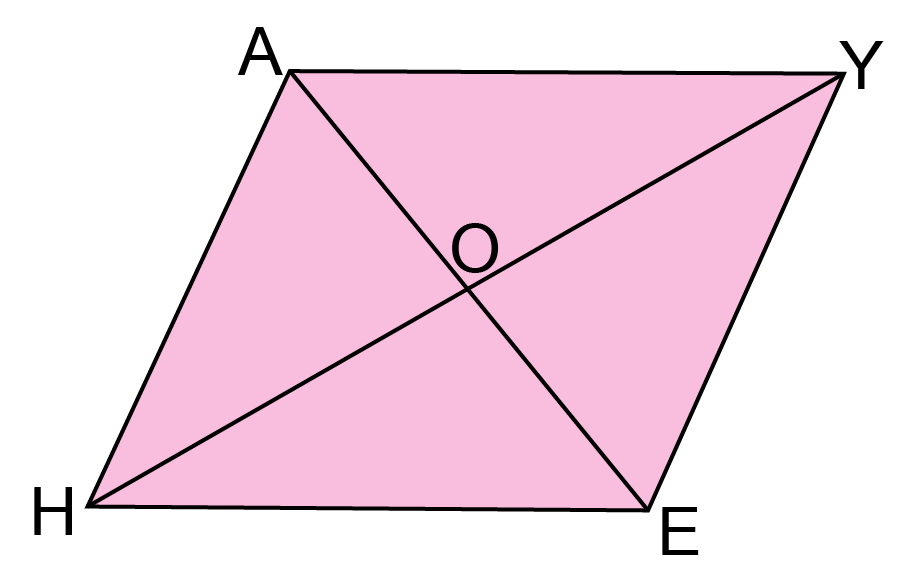
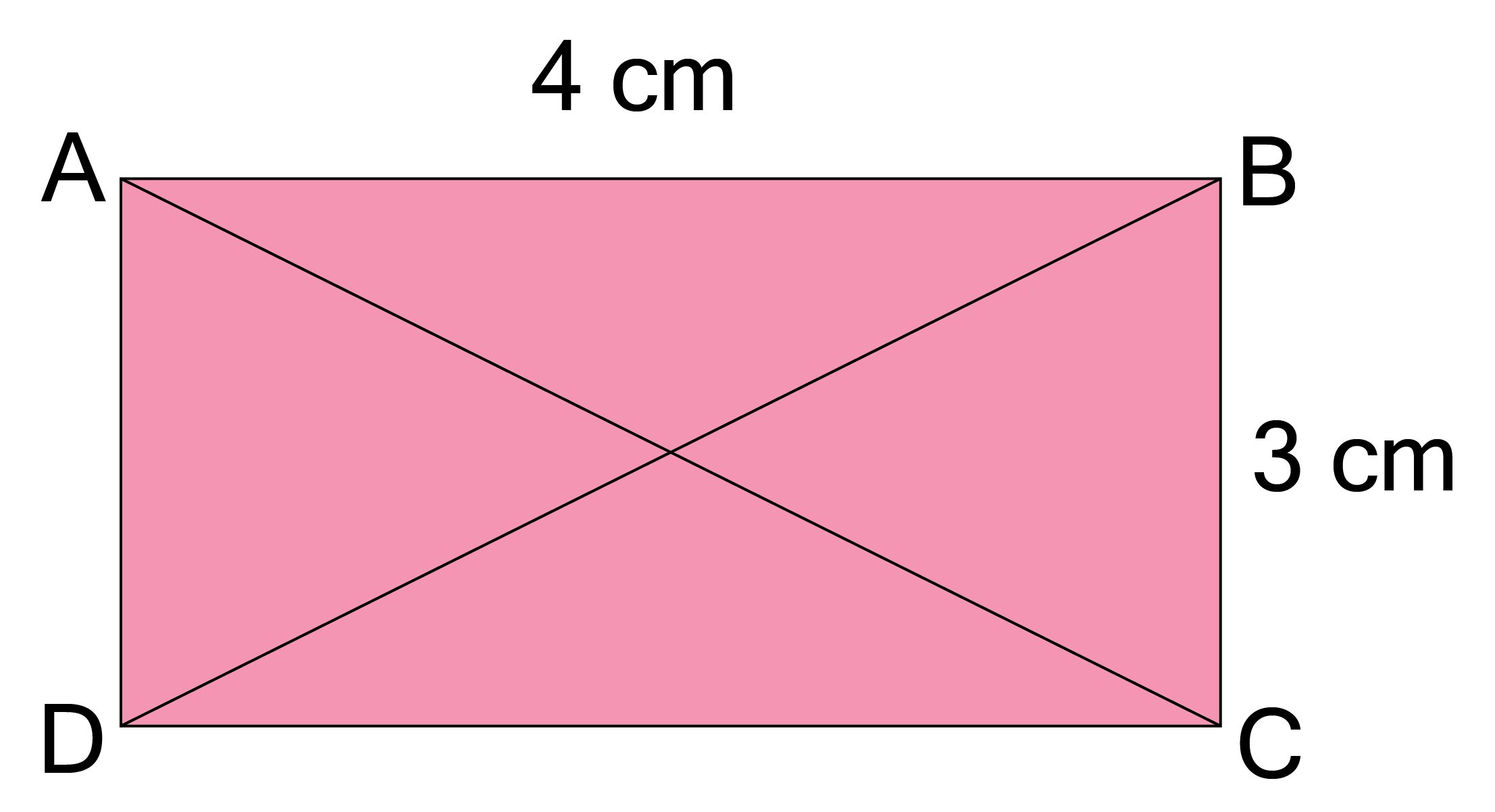
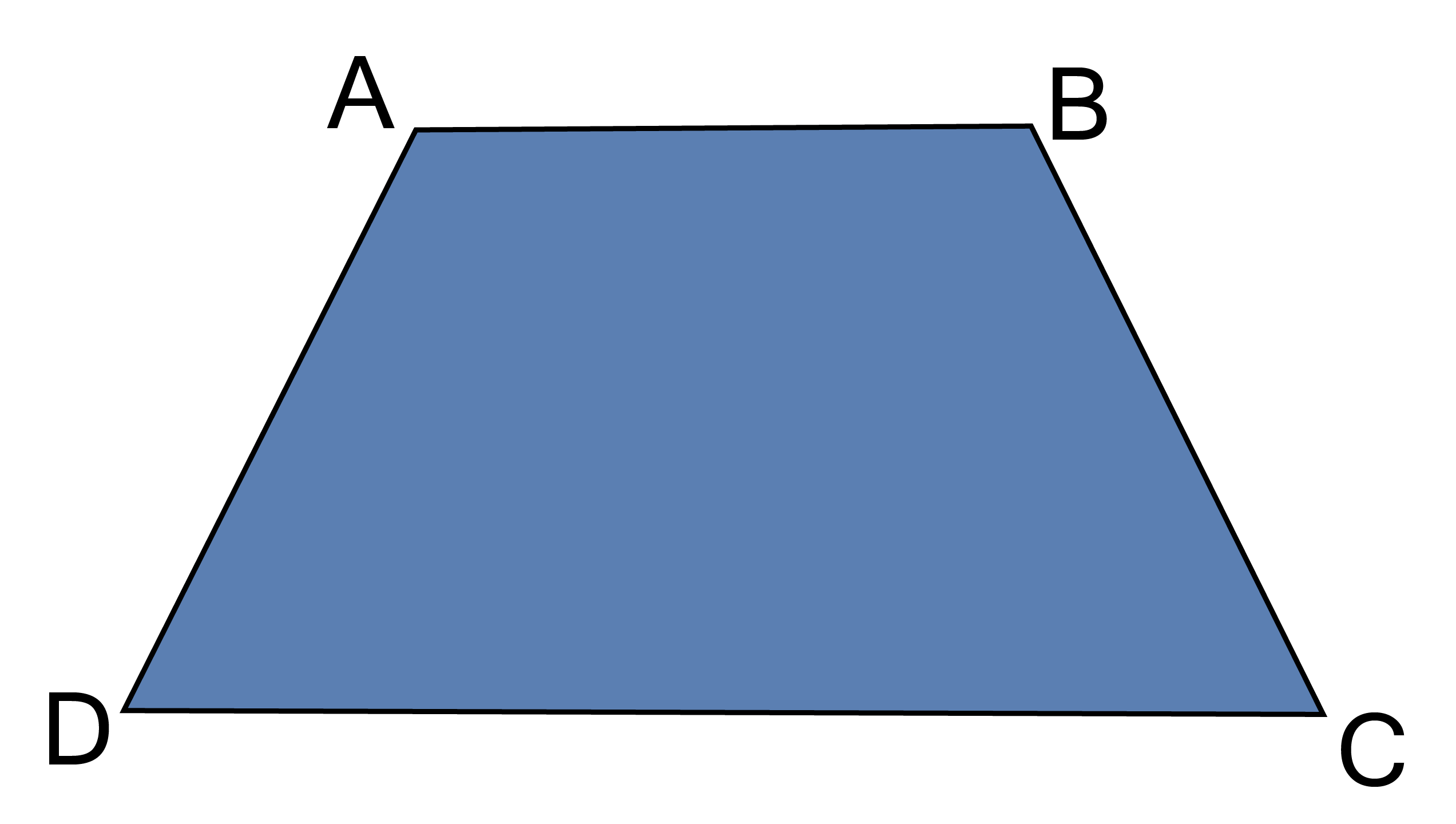
A quadrilateral is a polygon with four sides, four angles, and four vertices. Common examples include squares, rectangles, and trapeziums.
2. Why should I study quadrilaterals in Class 8 Chapter 3 Understanding Quadrilaterals?
Studying quadrilaterals helps you understand basic geometric concepts, which are essential for higher-level mathematics and real-world applications.
3. What kind of important questions can I expect for this Class 8 Chapter 3 Understanding Quadrilaterals?
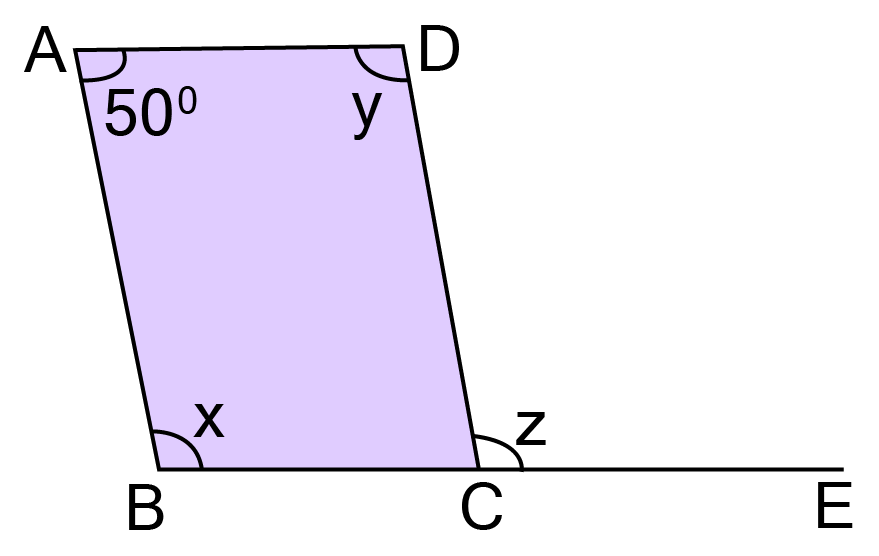
Important questions may include identifying types of quadrilaterals, calculating their angles, and solving problems related to their area and perimeter.
4. How do Class 8 Chapter 3 Understanding Quadrilaterals aid in exam preparation?
They help you focus on key concepts and common problem types, making it easier to recall information and apply it during exams.
5. Where can I find resources for important questions on quadrilaterals?
You can find resources on our Vedantu website, which offer organised important questions and additional study materials.
6. Are Class 8 Chapter 3 Understanding Quadrilaterals suitable for all students?
Yes, the important questions are designed to be helpful for all students, regardless of their level of understanding of the topic.
7. How can I improve my understanding of quadrilaterals through Class 8 Chapter 3 Understanding Quadrilaterals?
Besides practising important questions, you can watch video tutorials, read explanations, and engage in interactive quizzes to deepen your understanding.
8. Can I discuss these Class 8 Chapter 3 Understanding Quadrilaterals with others?
Yes, discussing questions with classmates or teachers can help clarify doubts and enhance your understanding of the subject.
9. What if I have difficulty with some of the important questions?
You can seek help from tutors, online forums, or study groups to get assistance with challenging questions.
10. How can I effectively review Class 8 Chapter 3 Understanding Quadrilaterals before exams?
Regularly practising important questions, reviewing your notes, and testing yourself with quizzes can help you prepare effectively for your exams.