Important Questions for CBSE Class 9 Maths Chapter 10 - Heron's Formula

Section A

1. An isosceles right triangle has an area $8\;{\text{c}}{{\text{m}}^2}$. The length of its hypotenuse is

1. $\sqrt {16} \,{\text{cm}}$

2. $\sqrt {48} \,{\text{cm}}$

3. $\sqrt {32} \,{\text{cm}}$

4. $\sqrt {24} \;{\text{cm}}$

Ans: Height of triangle $ = {\text{h}}$

As the triangle is isosceles,

Let base \[ = \]height $ = {\mathbf{h}}$

Area of triangle $ = 8\;{\text{c}}{{\text{m}}^2}$

$\Rightarrow \dfrac{1}{2} \times $ Base $ \times $ Height $ = 8$

$\Rightarrow \dfrac{1}{2} \times {\text{h}} \times {\text{h}} = 8$

\[ \Rightarrow {{\text{h}}^2} = 16\]

$\Rightarrow {\text{h}} = 4\;{\text{cm}}$

Base \[ = \] Height $ = 4\;{\text{cm}}$

Since the triangle is right angled,

Hypotenuse $^2 = $ Base $^2 + $ Height $^2$

$\Rightarrow $Hypotenuse $^2 = {4^2} + \,\,{4^2}$

$\Rightarrow $Hypotenuse $^2 = 32$

$\Rightarrow $Hypotenuse $ = \sqrt {32} $

Therefore, Options C is the correct answer.

2. The sides of a triangle are $35\;\,{\text{cm}},\,54\;{\text{cm}}$, and $61\;\,{\text{cm}}$, respectively. The length of its longest altitude is

1. $26\sqrt 5 \,{\text{cm}}$

2. $28\;{\text{cm}}$

3. $10\;\sqrt 5 \,{\text{cm}}$

4. $24\sqrt 5 \;{\text{cm}}$

Ans: Semi-perimeter of a triangle,

(Image will be uploaded soon)

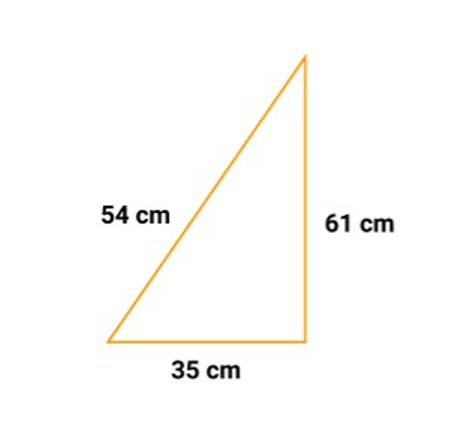
${{s}} = \dfrac{{{\text{a}} + {\text{b}} + {\text{c}}}}{2}$

$\,\,\, = \dfrac{{35 + 54 + 61}}{2}$

$\,\,\, = 75cm$

Area \[A\],

$\,\,\,\,\, A = \sqrt {s(s - a)(s - b)(s - c)}$

$\,\,\,\,\, = \sqrt {75(75 - 35)(75 - 54)(75 - 61)}$

$\,\,\,\,\, = 420\sqrt 5 \;{\text{c}}{{\text{m}}^2}$

Area of the triangle is also given as ${\text{A}} = \dfrac{1}{2} \times {\mathbf{a}} \times {\mathbf{h}}$

Where, ${\text{h}}$ is the longest altitude. 

Therefore, $\dfrac{1}{2} \times {\text{a}} \times {\text{h}} = 420\sqrt 5 $

$ \Rightarrow h = \dfrac{420 \times 2 \times \sqrt{5}}{a}$

$ \Rightarrow h = \dfrac{420 \times 2 \times \sqrt{5}}{35}$

Hence, the length of the altitude ${\text{h}} = 24\sqrt 5 \;{\text{cm}}$

3. The sides of a triangle are $56\;\,{\text{cm}},60\;\,{\text{cm}}.$ and $52\;{\text{cm}}$. long. The area of the triangle is.

1. $4311\;{\text{c}}{{\text{m}}^2}$

2. $4322\;{\text{c}}{{\text{m}}^2}$

3. $2392\;{\text{c}}{{\text{m}}^2}$

4. None of these

Ans: The three sides of a triangle are $a = 56\;{\text{cm}},b = 60\;{\text{cm}}$ and $c = 52\;{\text{cm}}$. Then, semi-perimeter of a triangle,

$s = \dfrac{{a + b + c}}{2} = \dfrac{{56 + 60 + 52}}{2} = \dfrac{{168}}{2} = 84\;{\text{cm}}$

Area of a triangle 

= $\sqrt {s(s - a)(s - b)(s - c)} $

= $\sqrt {84(84 - 56)(84 - 60)(84 - 52)} $

= $\sqrt {4 {\times} 7 {\times} 3 {\times} 4 {\times} 7 {\times} 4 {\times} 2 {\times} 3 {\times} 4 {\times} 4 {\times 2}} $

= $\sqrt {{{(4)}^6} \times {{(7)}^2} \times {{(3)}^2}} $

= ${(4)^3} \times 7 \times 3 = 1344\;{\text{c}}{{\text{m}}^2}$

The area of triangle is $1344\;{\text{c}}{{\text{m}}^2}$.

Therefore, the option (4) is the correct answer.

4. The area of an equilateral triangle is $16\sqrt 3 \;\,{{\text{m}}^2}.$ Its perimeter is

1. $24\;{\text{m}}$

2. $12\;{\text{m}}$

3. $306\;{\text{m}}$

4. \[48\,{\text{m}}\]

Ans: Let the side of the equilateral triangle be am 

Now, area of equilateral $\vartriangle  = \dfrac{{\sqrt 3 }}{4}{({\text{ side }})^2}$

$\Rightarrow 16\sqrt 3  = \dfrac{{\sqrt 3 }}{4}{({\text{a}})^2}$

$\Rightarrow {{\text{a}}^2} = \dfrac{{16\sqrt 3  \times 4}}{{\sqrt 3 }} = 64$

$\Rightarrow {\text{a}} = \sqrt {64}$

$= 8\;{\text{m}}$

Substitute the value of \[a\],

Perimeter of equilateral $\vartriangle  = 3{\text{a}} = 3 \times 8$.

$\, = 24\;{\text{m}}$

Therefore, option (4) is the correct answer.

5.  The perimeter of a triangle is $30\;{\text{cm}}$. Its sides are in the ratio \[1:{\text{ }}3:{\text{ }}2,\]then its

smallest side is.

1. $15\;{\text{cm}}$

2. $5\,{\text{cm}}$

3. $1\;{\text{cm}}$

4. $10\;{\text{cm}}$.

Ans: Perimeter of triangle $ = 30\;{\text{cm}}$

Ratio of its sides are $ = 1:3:2$

sides are ${\text{x}},3{\text{x}},2{\text{x}}$

$ \Rightarrow {\text{x}} + 3{\text{x}} + 2{\text{x}} = 30\;{\text{cm}}$

$ \Rightarrow 6{\text{x}} = 30$

$ \Rightarrow {\text{x}} = 5\;{\text{cm}}$

Therefore the smallest side is \[5\].

Hence, the option (2) is the correct answer.

Section – B

6. Find the area of a triangular garden whose sides are $40\;{\text{m}}.,90\;{\text{m}}$ and $70\;{\text{m}}$. (use $\sqrt 5  = 2.24$ )

Ans: Let $a = 40\;\,{\text{m}},\;{\text{b}} = 90\;\,{\text{m}}$ and ${\text{c}} = 70\;{\text{m}}$

The half perimeter,

$s = \dfrac{{(a + b + c)}}{2}$

$\Rightarrow \dfrac{{(40 + 90 + 70)}}{2}$

$\Rightarrow \dfrac{{200}}{2}$

$s = 100$

By Heron's formula of area of triangle $=\sqrt{s (s - a)(s - b)(s - c)}$

$\Rightarrow \sqrt{{100} (100 - 40)(100 - 90)(100 - 70)}$

$\Rightarrow \sqrt{{100}  \times 60 \times 10 \times 30}$

$\Rightarrow 10\sqrt{18000}$

$\Rightarrow 10 \times 60 - \sqrt 5$

$= 10 \times 134.4$

$ = 1344\;{{\text{m}}^2}$.

The area of the triangular garden $ = 1344\;{{\text{m}}^2}$.

7. Find the cost of leveling a ground in the form of a triangle with sides \[16m,{\text{ }}12m{\text{ }} and {\text{ }}20m\] at Rs.\[\;4\] per sq.meter.

Ans:Let the sides be $a = 16,b = 12,c = 20.$ 

By herons formula 

${\text{s}} = \dfrac{{{\text{a}} + {\text{b}} + {\text{c}}}}{2}$

$= \dfrac{{16 + 12 + 20}}{2}$

$= \dfrac{{48}}{2}$

$= 24$ 

The area of the triangle,

$ \Rightarrow A = \sqrt{s(s - a)(s - b)(s - c)}$

$ \Rightarrow A = \sqrt{(24 - 16)(24 - 12)(24 - 20)}$

$ \Rightarrow {\text{A}} = \sqrt {24 \times 8 \times 12 \times 4}$

$ \Rightarrow {\text{A}} = \sqrt {( 2 \times 2 \times 3 \times 2)(2 \times 2 \times 2)(2 \times 3 \times 2)(2 \times 2)}$

$\Rightarrow {\text{A}} = 2 \times 2 \times 2 \times 2 \times 2 \times 3$

$ \Rightarrow {\text{A}} = 96\;{\text{m sq}}$

Cost per meter $ = 4$ 

Cost for $96\;{\text{m}} = 4 \times 96$

$ = 384\,{\text{hrs}}$.

8. Find the area of a triangle, two sides of which are $8\;{\text{cm}}$ and $11\;{\text{cm}}$ and the perimeter is $32\;{\text{cm}}$.

Ans: Let \[a,b,c\] be the sides of the given triangle and \[2s\] be its perimeter such that ${\text{a}} = 8\;{\text{cm}},\;{\text{b}} = 11\;{\text{cm}}$ and $2\;{\text{s}} = 32\;{\text{cm}}$

Now, ${\text{a}} + {\text{b}} + {\text{c}} = 2\;{\text{s}}$

$8 + 11 + {\text{c}} = 32$

${\text{c}} = 13$

Therefore,

${\text{s}} - {\text{a}} = 16 - 8 = 8,\;$

${\text{s}} - {\text{b}} = 16 - 11 = 5,\;$

${\text{s}} - {\text{c}} = 16 - 13 = 3$ 

Hence, the area of given triangle $ = \sqrt {s(s - a)(s - b)(s - c)} $ 

$= \sqrt {16 \times 8 \times 5 \times 3}$

$= 8\sqrt {30} \;{\text{c}}{{\text{m}}^2}.$ 

9. The area of an isosceles triangle is $12\;{\text{c}}{{\text{m}}^2}$. If one of its equal side is $5\;{\text{cm}}$. Find its base.

Ans: Let equal sides be $(\mathrm{a})=5 \mathrm{~cm}$ and base (b) =?

Area of an isosceles triangle $=12$ sq. $\mathrm{cm}$

Area of an isosceles triangle

$=\dfrac{b}{4} \sqrt{4 a^{2}-b^{2}}$

$12=\dfrac{b}{4} \sqrt{4 \times(5)^{2}-b^{2}}$

$48=b \sqrt{100-b^{2}}$

Squaring both the sides, we get

$2304=b^{2}\left(100-b^{2}\right)$ 

$\quad b^{4}-100 b^{2}+2304=0$ 

$b^{2}-64 b^{2}-36 b^{2}+2304=0$

$b^{2}\left(b^{2}-64\right)-36\left(b^{2}-64\right)=0$

$\quad\left(b^{2}-64\right)\left(b^{2}-36\right)=0$

either $b^{2}-64=0$

$\Rightarrow b^{2}=64 \Rightarrow b=\pm 8$

or $b^{2}-36=0$

$\Rightarrow b^{2}-36 \Rightarrow b=\pm 6$

Hence base $=8 \mathrm{~cm}$, or $6 \mathrm{~cm}$.

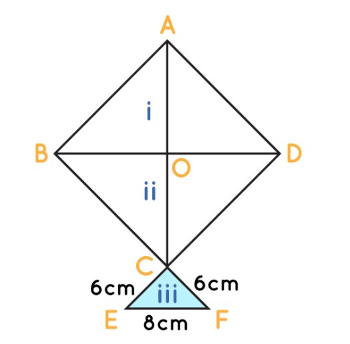
11. Find the area of the adjoin figure if \[{\text{AB}}\,{\text{& }}\,{\text{BC}}\]

(Image will be uploaded soon)

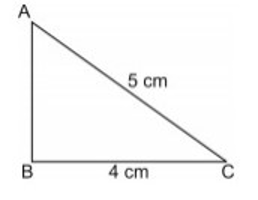
Ans: Since, $\angle B = {90^\circ }$ \[ABC\] is a right angle triangle.

Pythagoras Theorem,

$ \Rightarrow A{B^2} + B{C^2} = A{C^2}$ 

$ \Rightarrow A{B^2} + {4^2} = {5^2}$

$ \Rightarrow A{B^2} = 25 - 16$

$ \Rightarrow A{B^2} = 9$

$ \Rightarrow AB = 3\;{\text{cm}}$

$\operatorname{Area} \,(\vartriangle {\text{ABC)}}\,{\text{ = }}\dfrac{1}{2}\, \times \,\,{\text{AB}}\,{{ \times }}\,{\text{BC}}$

$= \dfrac{1}{2} \times 3 \times 4$

$= 6\,{\text{c}}{{\text{m}}^{\text{2}}}$ 

Section - C

12. The diagonals of a rhombus are \[24{\text{ cm}}\] and \[10{\text{ cm}}{\text{.}}\] Find its area and perimeter.

Ans: Find the area, 

Area $ = \dfrac{1}{2} \times 24 \times 10$

$ = 120\;{\text{c}}{{\text{m}}^2}$

Perimeter ${s^2} = {\left( {\dfrac{{24}}{2}} \right)^2} + {\left( {\dfrac{{10}}{2}} \right)^2}$

$\quad  = {12^2} + {5^2}$

$ = 169$

$\;{\text{s}} = 13$

The perimeter of the rhombus $ = 4 \times 13 = 52\;{\text{cm}}$.

13. Two side of a parallelogram are \[10{\text{ cm}}\] and \[7{\text{cm}}\]. One of its diagonals is \[13{\text{ cm}}{\text{.}}\] Find the area.

Ans:

(Image will be uploaded soon)

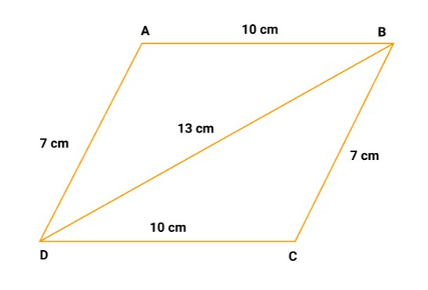
${\text{ABCD}}$ is parallelogram

${\text{AB}} = {\text{CD}} = 10\;{\text{cm}}$

${\text{AD}} = {\text{CB}} = 7\;{\text{cm}}$

Diagonal ${\text{BD}} = 13\;{\text{cm}}$

Diagonal divides the parallelogram into two equal triangles

\[{\text{Find the area of triangle ABD}}\]

Area $ = \sqrt {s(s - a)(s - b)(s - c)} $

$s = \dfrac{{a + b + c}}{2}$

$a = 10$

$b = 7$

$c = 13$

Substitute the values in the formula :

$s = \dfrac{{10 + 7 + 13}}{2}$

$s = \dfrac{{10 + 7 + 13}}{2}$

Area $ = \sqrt {15(15 - 10)(15 - 7)(15 - 13)} $

Area $ = 34.6410161514$

Area of parallelogram $ = 2 \times $ Area of triangle $ = 2 \times 34.6410161514 = 69.2820\;{\text{c}}{{\text{m}}^2}$

Hence the area of parallelogram is \[69.2820{\text{ }}sq.cm.\]

14. A rhombus shaped sheet with perimeter \[40{\text{ }}cm\] and one diagonal\[12{\text{ }}cm\], is painted on both sides at the rate of \[5\] per \[{{\text{m}}^{\text{2}}}\]. Find the cost of painting

Ans: Let ${\text{ABCD}}$ be a rhombus, then ${\text{AB}} = {\text{BC}} = {\text{CD}} = {\text{DA}} = {\text{x}}$

Perimeter of rhombus $ = 40\;{\text{cm}}$

$\Rightarrow 4{\text{x}} = 40\;{\text{cm}} \Rightarrow {\text{x}} = 10\;{\text{cm}}$

$\therefore {\text{AB}} = {\text{BC}} = {\text{CD}} = {\text{DA}} = 10\;{\text{cm}}$

In $\vartriangle {\text{ABC}},{\text{S}} = \dfrac{{{\text{a}} + {\text{b}} + {\text{c}}}}{2} = \dfrac{{10 + 10 + 12}}{2} = 16\;{\text{cm}}$

ar $\vartriangle {\text{ABC}} = \sqrt {16(16 - 10)(16 - 10)(16 - 12)}  = \sqrt {16 \times 6 \times 6 \times 4}  = 48\;{\text{c}}{{\text{m}}^2}$

ar. ${\text{ABCD}} = 2 \times 48 = 96\;{\text{c}}{{\text{m}}^2}$

Cost of painting the sheet $ = \operatorname{Rs} (5 \times 96 \times 2) = $ \[Rs{\text{ }}960\] [Both sides]

15. The sides of a quadrilateral ABCD are $6\;{\text{cm}},8\;{\text{cm}},12\;{\text{cm}}$ and $14\;{\text{cm}}$ (taken in order) respectively, and the angle between the first two sides is a right angle. Find its area.

Ans: Applying Pythagoras theorem in $\vartriangle {\text{ABC}}$, we get

${\text{AC}} = \sqrt {{\text{A}}{{\text{B}}^2} + {\text{B}}{{\text{C}}^2}}  = \sqrt {{6^2} + {8^2}}  = \sqrt {36 + 64}  = \sqrt {100}  = 10\;{\text{cm}}$

So, the area of $\vartriangle {\text{ABC}} = \dfrac{1}{2} \times $ base $ \times $ height

$ = \dfrac{1}{2} \times {\text{AB}} \times {\text{BC}}$

$ = \dfrac{1}{2} \times 6 \times 8 = 24$

Now, in $\vartriangle {\text{ACD}}$, we have,

${\text{AC}} = 10\;{\text{cm}},$

${\text{CD}} = 12\;{\text{cm}},$

${\text{AD}} = 14\;{\text{cm}}$ 

Now, in $\vartriangle {\text{ACD}}$, we have ${\text{AC}} = 10\;{\text{cm}},{\text{CD}} = 12\;{\text{cm}},{\text{AD}} = 14\;{\text{cm}}$

According to Heron's formula the area of triangle $(A) = \sqrt {[{\text{s}}({\text{s}} - {\text{a}})({\text{s}} - {\text{b}})({\text{s}} - {\text{c}})]} $

where, $2s = (a + b + c)$

Here, ${\text{a}} = 10\;{\text{cm}},\;{\text{b}} = 12\;{\text{cm}},{\text{c}} = 14\;{\text{cm}}$

${\text{s}} = \dfrac{{(10 + 12 + 14)}}{2} = \dfrac{{36}}{2} = 18$

Area of $\vartriangle {\text{ACD}} = \sqrt {[18 \times (18 - 10)(18 - 12)(18 - 14)]}$

$= \sqrt {(18 \times 8 \times 6 \times 4)}$

$= \sqrt {(2 \times 3 \times 3 \times 2 \times 2 \times 2 \times 2 \times 3 \times 2 \times 2)}$

$= \sqrt {[(2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 3 \times 3) \times 2 \times 3]}$

$= 2 \times 2 \times 2 \times 3 \times \sqrt 6$

$= 24\sqrt 6$

So, total area of quadrilateral ${\text{ABCD}} = \vartriangle {\text{ABC}} + \vartriangle {\text{ACD}}$

$= 24 + 24\sqrt 6$

$= 24(\sqrt 6  + 1)$.

16. The perimeter of an isosceles triangle is $32\;{\text{cm}}$. The ratio if the equal side to its base is\[32\]. Find the area of the triangle.

Ans: The ratio of the equal side to the base is \[32.\]

Let the sides be $3{\text{x}},2{\text{x}}$. Let the third $ = 3{\text{x}}$

Given, perimeter $ = 32$

We know that the perimeter is equal to the sum of the sides. Thus, $\Rightarrow 3x + 2x + 3x = 32$

$\Rightarrow 8x = 32$

$\Rightarrow x = 4$

$\Rightarrow \dfrac{{32}}{2} = 16$

Thus, the sides are $12\;{\text{cm}},8\;{\text{cm}},12\;{\text{cm}}$

Thus, Area of the triangle $= \sqrt {\dfrac{{32}}{2}(16 - 12)(16 - 8)(16 - 12)}$

$= \sqrt {16 \times 4 \times 8 \times 4}$

$= 32\sqrt 2 \;{\text{c}}{{\text{m}}^2}$.

17.  The sides of a triangular field are $41\;{\text{m}},40\;{\text{m}}$ and $9\;{\text{m}}$. Find the number of flower beds that can be prepared in the field, if each flower bed needs $900\;{\text{c}}{{\text{m}}^2}$ space.

Ans: By Heron's formula. Area of a triangular= $ \cdot \sqrt s  \times (s - a)(s - b)(s - c)$, where \[a,b,c\] are sides of the triangle and $s$ is the semi perimeter. so, area of the field $=\sqrt {[45 \times (45 - 41)(45 - 40)(45 - 9)]}$

$= \sqrt {(45 \times 4 \times 5 \times 36)}$

$= \sqrt 3 2400$ 

$= 180\;{{\text{m}}^2}$

$= 1800000\;{\text{c}}{{\text{m}}^2}$ 

now, space needed for a flower bed $= 900\;{\text{c}}{{\text{m}}^2}$

so, number of flower beds $= \dfrac{{1800000}}{{900}}$

$= 2000.$ 

18. The perimeter of a triangular ground is $420\;{\text{m}}$ and its sides are in the ratio\[6:7:8\]. Find the area of the triangular ground.

Ans: The perimeter of triangular field $ = 420\;{\text{m}}$.

Given that the ratios of the sides are \[6:7:8\]

Sum of the ratios $ = 6 + 7 + 8 = 21$

Length of first side of the field 

$= \dfrac{6}{{21}} \times 420$

$= 6 \times 20$

$= 120\;{\text{m}}$ 

Length of second side of the field $ = \dfrac{7}{{21}} \times 420 = 7 \times 20 = 140\;{\text{m}}$

Length of third side of the field $ = \dfrac{8}{{21}} \times 420 = 8 \times 20 = 160\;{\text{m}}$

According to Heron's formula the area (A) of triangle with sides \[a,{\text{ }}b{\text{ }}\& {\text{ }}c\] is given as,

${\text{A}} = \sqrt[2]{{{\text{s}}({\text{s}} - {\text{a}})({\text{s}} - {\text{b}})({\text{s}} - {\text{c}})}}]$ where $2\;{\text{s}} = ({\text{a}} + {\text{b}} + {\text{c}})$

Here ${\text{a}} = 120\;{\text{m}},\;{\text{b}} = 140\;{\text{m}},{\text{c}} = 160\;{\text{m}},\;$

${\text{s}} = \dfrac{{(120 + 140 + 160)}}{2} = \dfrac{{420}}{2} = 210$ 

Area of triangular field $= \sqrt[{}]{{210 \times (210 - 120)(210 - 140)(210 - 160)}}$

$= \sqrt[{}]{{(210 \times 90 \times 70 \times 50)}}$

$= \sqrt {(3 \times 7 \times 3 \times 3 \times 7 \times 5 \times 10000)}$

$= \sqrt[{}]{{[(7 \times 3 \times 100 \times 7 \times 3 \times 100) \times 3 \times 5]}}$

$= 2100\sqrt[{}]{{15}}$.

Section - D

19. Calculate the area of the shaded region.

(Image will be uploaded soon)

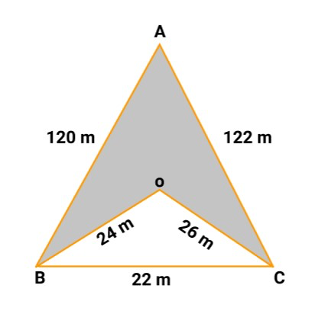
Ans: Area of shaded region $ = \operatorname{ar} \Delta {\text{ABC}} - \operatorname{ar} \Delta {\text{DBC}}$

$\operatorname{ar} \Delta {\text{ABC}} = \sqrt {{\text{s}}({\text{s}} - {\text{a}})({\text{s}} - {\text{b}})({\text{s}} - {\text{c}})} $

${\text{s}} = \dfrac{{{\text{a}} + {\text{b}} + {\text{c}}}}{2} = \dfrac{{122 + 22 + 120}}{2} = 132\;{\text{m}}$

$\therefore \operatorname{ar} \Delta {\text{ABC}} = \sqrt {132(132 - 122)(132 - 22)(132 - 120)}$

$= \sqrt {132 \times 10 \times 110 \times 12}$

$= \sqrt {11 \times 12 \times 10 \times 10 \times 11 \times 12}$

$= 10 \times 11 \times 12 = 1320\;{{\text{m}}^2}$

$ar\Delta BDC = \sqrt {s(s - a)(s -  - b)(s - c)}$

${\text{s}} = \dfrac{{{\text{a}} + {\text{b}} + {\text{c}}}}{2} = \dfrac{{36 + 22 + 24}}{2} = 36\;{\text{m}}$

$\therefore \operatorname{ar} \Delta {\text{BDC}} = \sqrt {36(36 - 26)(36 - 22)(36 - 24)} $

$= \sqrt {36 \times 10 \times 14 \times 12}$

$= \sqrt {12 \times 3 \times 2 \times 5 \times 2 \times 7 \times 12}$

$= 2 \times 12\sqrt {105}  = 24 \times 10.24 = 245.76\;{{\text{m}}^2}$

$\therefore $ Area of shaded region $ = 1320 - 245.76 = 1074.24\;{{\text{m}}^2} \approx 1074\;{{\text{m}}^2}$

20. If each sides of a triangle is double, then find the ratio of area of the new triangle thus formed and the given triangle.

Ans: Let ${\text{a}},{\text{b}}$ and ${\text{c}}$ denotes the length of the sides of the triangle.

Area of the triangle, ${A_1} = \sqrt {s(s - a)(s - b)(s - c)} $, where s is the semi-perimeter of the triangle. So, semi perimeter $s = \dfrac{{a + b + c}}{2}$

When the sides of the triangle are doubled, we get ${{\text{s}}^\prime } = \dfrac{{2{\text{a}} + 2\;{\text{b}} + 2{\text{c}}}}{2} = {\text{a}} + {\text{b}} + {\text{c}} = 2\;{\text{s}}$, where ${{\text{s}}^\prime }$ is the semi-perimeter of the new triangle

Area of the new triangle, ${{\text{A}}_2} = \sqrt {{{\text{s}}^\prime }\left( {{{\text{s}}^\prime } - 2{\text{a}}} \right)\left( {{{\text{s}}^\prime } - 2\;{\text{b}}} \right)\left( {{{\text{s}}^\prime } - 2{\text{c}}} \right)} $

$= \sqrt {2\;{\text{s}}(2\;{\text{s}} - 2{\text{a}})(2\;{\text{s}} - 2\;{\text{b}})(2\;{\text{s}} - 2{\text{c}})}$

$= \sqrt {16\;{\text{s}}(\;{\text{s}} - {\text{a}})({\text{s}} - {\text{b}})({\text{s}} - {\text{c}})}$

$= 4\sqrt {{\text{s}}({\text{s}} - {\text{a}})({\text{s}} - {\text{b}})({\text{s}} - {\text{c}})}  = 4\;{{\text{A}}_1}$

Therefore, the ratio of the area of new triangle to the given triangle $ = \dfrac{{{{\text{A}}_2}}}{{\;{{\text{A}}_1}}} = \dfrac{{4\;{{\text{A}}_1}}}{{\;{{\text{A}}_1}}} = 4:1$

21. A field is in the shape of a trapezium whose parallel sides are $25\;{\text{m}}$ and $10\;{\text{m}}$. If its non-parallel sides are $14\;{\text{m}}$ and $13\;{\text{m}}$, find its area.

Ans: 

(Image will be uploaded soon)

Let ${\text{ABCD}}$ be a trapezium with,

${\text{AB}} = 25\;{\text{m}}$

${\text{CD}} = 10\;{\text{m}}$

${\text{BC}} = 14\;{\text{m}}$

${\text{AD}} = 13\;{\text{m}}$

Draw CE || DA. So, ADCE is a parallelogram with, ${\text{CD}} = {\text{AE}} = 10\;{\text{m}}$

${\text{CE}} = {\text{AD}} = 13\;{\text{m}}$

${\text{BE}} = {\text{AB}} - {\text{AE}} = 25 - 10 = 15\;{\text{m}}$

In $\Delta {\text{BCE}}$, the semi perimeter will be, $s = \dfrac{{a + b + c}}{2}$

${\text{s}} = \dfrac{{14 + 13 + 15}}{2}$

${\text{s}} = 21\;{\text{m}}$

Area of $\Delta {\text{BCE}}$,

${\text{A}} = \sqrt {{\text{s}}({\text{s}} - {\text{a}})({\text{s}} - {\text{b}})({\text{s}} - {\text{c}})}$

$= \sqrt {21(21 - 14)(21 - 13)(21 - 15)}$

$= \sqrt {21(7)(8)(6)}$

$= \sqrt {7056} $

$= 84\;{{\text{m}}^2}$

Also, area of $\Delta {\text{BCE}}$ is, ${\text{A}} = \dfrac{1}{2} \times$ base $\times$ height

$84 = \dfrac{1}{2} \times 15 \times {\text{CL}}$

$\dfrac{{84 \times 2}}{{15}} = {\text{CL}}$

${\text{CL}} = \dfrac{{56}}{5}\;{\text{m}}$

The area of trapezium is, ${\text{A}} = \dfrac{1}{2}$ (sum of parallel sides) (height)

${\text{A}} = \dfrac{1}{2} \times (25 + 10)\left( {\dfrac{{56}}{5}} \right)$

${\text{A}} = 196\;{{\text{m}}^2}$

Therefore, the area of the trapezium is $196\;{{\text{m}}^2}$.

22. An umbrella is made by stitching \[10\] triangular pieces of cloth of \[5\] different colour each piece measuring $20\;{\text{cm}},50\;{\text{cm}}$ and $50\;{\text{cm}}$. How much cloth of each colour is required for one umbrella? 

\[(\sqrt 6  = 2.45)\] 

Ans: Area of a trangle $ = \dfrac{1}{2}$ bh

Hear, $b = 20$

$h = \sqrt {{{50}^2} - {{10}^2}}  = \sqrt {2500 - 100}$

$= \sqrt {2400}$

$= \sqrt {6 \times 400}  \cdot 20\sqrt 6$ 

$\therefore $ Area $ = \dfrac{1}{{{2^2}}} \times 20.20\sqrt 6$

$= 10 \times 20\sqrt 6  = 200\sqrt 6$

$= 200 \times 245 = 490\;{\text{c}}{{\text{m}}^2}.$

Each colour. cloth is used \[2\] times.

$\therefore$ The area of each colour cloth required for one umbrella $= 490 \times 2$

$= 980\,{\text{c}}{{\text{m}}^2}$

23. A triangle and a parallelogram have the same base and some area. If the sides of the triangle are $26\;{\text{cm}},28\;{\text{cm}}$ and $30\;{\text{cm}}$ and the parallelogram stands on the base $28\;{\text{cm}}$, find the height of the parallelogram.

Ans: Find Perimeter of Triangle,

$2\;{\text{S}} = 26 + 28 + 30 = 84$

$\Rightarrow {\text{S}} = 42\;{\text{cm}}$

Use Heron's formula,

Area $\sqrt {s(s - a)(s - b)(s - c)} $ 

Area $= \sqrt {42(42 - 26)(42 - 28)(42 - 30)}$

$= \sqrt {42 \times 16 \times 14 \times 12}$ 

Area $ = 336\;{\text{c}}{{\text{m}}^2}$

Area of parallelogram \[ = \] Area of triangle

$\Rightarrow {\text{h}} \times 28 = 336$

$\Rightarrow {\text{h}} = 12\;{\text{cm}}$

Height of parallelogram$= 12\;{\text{cm}}$.

1 Mark Questions:

1. The measure of each side of an equilateral triangle whose area is $\sqrt 3 \;{\text{c}}{{\text{m}}^2}$ is,

(A) $8\;{\text{cm}}$

(B) $2\;{\text{cm}}$

(C) $4\;{\text{cm}}$

(D) $16\;{\text{cm}}$

Ans: Correct answer option (B) $2\;{\text{cm}}$

2. Measure of each side of an equilateral triangle is $12\;{\text{cm}}$. Its area is given by

(A) $9\sqrt 3 {\text{ sq cm}}$

(B) $18\sqrt 3 {\text{ sq cm}}$

(C) $27\sqrt 3 $${\text{sq cm}}$

(D) $36\sqrt 3 $${\text{sq cm}}$

Ans: Correct answer option (D) $36\sqrt 3 {\text{ sq cm}}$

3. Two adjacent side of a parallelogram are ${\mathbf{74cm}}$ and ${\mathbf{40cm}}$ one of its diagonals is 102cm. Area of the ||gram is

(A) $612\,{\text{sq}}\,{\text{m}}$

(B) $1224\,{\text{sq}}\,{\text{m}}$

(C) $2448\,{\text{sq}}\,{\text{m}}$

(D) $4896\,{\text{sq}}\,{\text{m}}$

Ans: Correct answer option (C) $2448\,{\text{sq}}\,{\text{m}}$

4. The perimeter of a triangle is \[60\;{\text{cm}}.\] If its sides are in the ratio \[1:{\text{ }}3:{\text{ }}2,\] then its smallest side is

(A) \[15\]

(B) \[5\]

(C) \[10\] 

(d) None of these.

Ans: Correct answer option (C) \[10\] 

5. The perimeter of a triangle is \[36\;{\text{cm}}.\] If its sides are in the ratio \[1:{\text{ }}3:{\text{ }}2,\] then its largest side is

(a) \[6\]

(b) \[12\]

(c) \[18\]

(d) none of these.

Ans: Correct answer option (c) \[18\]

6. If the perimeter of a rhombus is $20\;{\text{cm}}$ and one of the diagonals is $8\;{\text{cm}}$. The area of the rhombus is

(a) $24\,{\text{sq}}\,{\text{cm}}$

(b) ${\text{48}}\,{\text{sq}}\,{\text{cm}}$

(c) $50\,{\text{sq}}\,{\text{cm}}$

(d) $30\,{\text{sq}}\,{\text{cm}}$

Ans: Correct answer option (a) $24\,{\text{sq}}\,{\text{cm}}$

7. One of the diagonals of a rhombus is $12\;{\text{cm}}$ and area is $54\,{\text{sq}}\,{\text{cm}}$. the perimeter of the rhombus is

(a) $72\;{\text{cm}}$

(b) $\sqrt[3]{{10}}\;{\text{cm}}$

(c) $\sqrt[6]{{10}}\;{\text{cm}}$

(d) $\sqrt[{12}]{{10}}\;{\text{cm}}$

Ans: Correct answer option (d) $\sqrt[{12}]{{10}}\;{\text{cm}}$

8. The side of a triangle is $12\;{\text{cm}},16\;{\text{cm}}$, and $20\;{\text{cm}}$. Its area is

(A) $100\,{\text{c}}{{\text{m}}^2}$

(B) $90\,{\text{c}}{{\text{m}}^2}$

(C) ${\text{96}}\,{\text{c}}{{\text{m}}^2}$

(D) $120\,{\text{c}}{{\text{m}}^2}$.

Ans: Correct answer option (C) ${\text{96}}\,{\text{c}}{{\text{m}}^2}$

9. The side of an equilateral triangle is $4\sqrt 3 \;{\text{cm}}$. Its area is.

(A) $12\sqrt 3 \,{\text{c}}{{\text{m}}^2}$

(B) $12\sqrt 6 \,{\text{c}}{{\text{m}}^2}$ 

(C) $12\sqrt {10} \,{\text{c}}{{\text{m}}^2}$

(D) $6\sqrt {10} \,{\text{c}}{{\text{m}}^2}$.

Ans: (A)  $12\sqrt 3 \,{\text{c}}{{\text{m}}^2}$

10. It the perimeter of a rhombus is 20 sq ${\text{cm}}$ and one of the diagonals is $8\;{\text{cm}}$. Then the area of the rhombus is

(A) $40\,{\text{sq}}\,{\text{cm}}$

(B) $24\,{\text{sq}}\,{\text{cm}}$

(C) $20\,{\text{sq}}\,{\text{cm}}$

(D) $13\,{\text{sq}}\,{\text{cm}}$.

Ans: Correct answer is option (B) $24\,{\text{sq}}\,{\text{cm}}$

11. One of the diagonals of a rhombus is $12\;{\text{cm}}$ and Its area is $54\,{\text{sq}}\,{\text{cm}}$. The perimeter of

the rhombus is.

(A) $10\;{\text{cm}}$

(B) $8\;{\text{cm}}$

(C) $6\;{\text{cm}}$

(D) $12\sqrt {10} \;{\text{cm}}$.

Ans: (D) $12\sqrt {10} \;{\text{cm}}$.

12. The lengths of the side of a triangular park are $90\;\,{\text{m}},70\,\;{\text{m}}$ and $40\;\,{\text{m}}$, find Its area.

(A) $1340\,{\text{sq}}\,{\text{m}}$

(B) $134\,{\text{sq}}\,{\text{m}}$

(C) ${\text{140}}\,{\text{sq}}\,{\text{m}}$

(D) $1444\,{\text{sq}}\,{\text{m}}$

Ans: (B) $1344\,{\text{sq}}\,{\text{m}}$

13. An equilateral triangle has a side $50\;{\text{cm}}$ long. Find the area of the triangles.

(A) $625\sqrt 3 \,{{\text{m}}^{\text{2}}}$

(B) $625\sqrt 6 \,{{\text{m}}^{\text{2}}}$ 

(C) $256\sqrt 6 \,{{\text{m}}^2}$

(D) $625\sqrt {10} \,{{\text{m}}^2}$ 

Ans: (A) $625\sqrt 3 \,{{\text{m}}^2}$ 

14. The area of an isosceles triangle is ${\text{12}}\,{\text{c}}{{\text{m}}^2}$. If one of the equal side is $5\;{\text{cm}}$, then the length of the base is

(A) $4\;{\text{cm}}$

(B) $5\;{\text{cm}}$

(C) $6\;{\text{cm}}$

(D) $8\;{\text{cm}}$

Ans: (C) $6\;{\text{cm}}$

15. Find the area of triangle whose side is $6\;{\text{cm}},10\;{\text{cm}}$ and $15\;{\text{cm}}$.

(A) $404.9\,{\text{sq}}\,{\text{cm}}$

(B) $405.9\,{\text{sq}}\,{\text{cm}}$

(C) $402.9\,{\text{sq}}\,{\text{cm}}$

(D) $410\,{\text{sq}}\,{\text{cm}}$

Ans: (A) $404.9\,{\text{sq}}\,{\text{cm}}$

16. If side of equilateral triangle is $25\;{\text{m}}$. Its area is

(a) $\dfrac{{625}}{4}\sqrt 3 \,{\text{sq}}\,{\text{cm}}$

(b) $54\sqrt 3 \,{\text{sq}}\,{\text{cm}}$

(c) $5\sqrt 3 \,{\text{sq}}\,{\text{cm}}$

(d) $\sqrt 3 \,{\text{sq}}\,{\text{cm}}$

Ans: (a) $\dfrac{{625}}{4}\sqrt 3 \,{\text{sq}}\,{\text{cm}}$ 

17. The perimeter of an equilateral triangle is $48\;{\text{cm}}$. Its area is

(a) $18\sqrt 3 \,{\text{sq}}\,{\text{cm}}$

(b) $72\sqrt 3 \,{\text{sq}}\,{\text{cm}}$

(c) $64\sqrt 3 \,{\text{sq}}\,{\text{cm}}$

(d) $60\sqrt 3 \,{\text{sq}}\,{\text{cm}}$

Ans: (c) $64\sqrt 3 \,{\text{sq}}\,{\text{cm}}$

18. If area of isosceles triangle is ${\text{48c}}{{\text{m}}^2}$ and length of one of its equal sides is $10\;{\text{m}}$, then what is the base?

(a) $16\;{\text{cm}}$ or $12\;{\text{cm}}$

(b) $12\;{\text{cm}}$ or $14\;{\text{cm}}$

(c) $14\;{\text{cm}}$ or $16\;{\text{cm}}$

(d) $16\;{\text{cm}}$ or $18\;{\text{cm}}$

Ans: (a) $16\;{\text{cm}}$ or $12\;{\text{cm}}$

19. If ${\text{AB}} = 14\;{\text{cm}},{\text{BC}} =13\;{\text{cm}},{\text{CD}} = 17\;{\text{cm}},{\text{DA}} = 8\;{\text{cm}}$ and ${\text{AC}} = 15\;{\text{cm}}$ then area of quadrilateral \[ABCD\] is

(Image will be uploaded soon)

(a) $150{\text{sq cm}}$

(b) ${\text{144sq cm}}$

(c) $142{\text{sq cm}}$

(d) $100{\text{sqcm}}$

Ans: (b) ${\text{144 sq cm}}$

2 Marks Questions:

1. There is slide in a park. One of its side walls has been painted in some colour with a message "KEEP THE PARK GREEN AND CLEAN", (see figure). If the sides of the wall are

$15\;{\text{m}},11\;{\text{m}}$ and $6\;{\text{m}}$, find the area painted in colour.

(Image will be uploaded soon)

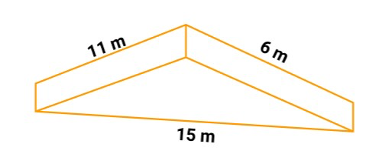
Ans: Sides of coloured triangular wall are $15\;{\text{m}},11\;{\text{m}}$ and $6\;{\text{m}}$.

$\therefore $ Semi-perimeter of coloured triangular wall

$ = \dfrac{{15 + 11 + 6}}{2} = \dfrac{{32}}{2} = 16\;{\text{m}}$

Now, Using Heron's formula,

Area of coloured triangular wall

$ = \sqrt {s(s - a)(s - b)(s - c)} $

$ = \sqrt {16(16 - 15)(16 - 11)(16 - 6)} $

$ = \sqrt {16 \times 1 \times 5 \times 10}  = 20\sqrt 2 {m^2}$

Therefore, the painted in blue colour $ = 20\sqrt 2 {m^2}$

2. Find the area of a triangle two sides of which are $18\;{\text{cm}}$ and $10\;{\text{cm}}$ and the perimeter is $42\;{\text{cm}}$.

Ans: Given: $a = 18\;{\text{cm}},b = 10\;{\text{cm}}$

Since Perimeter $ = 42\;{\text{cm}}$

$\Rightarrow a + b + c = 42$

$\Rightarrow 18 + 10 + c = 42$

$\Rightarrow c = 42 - 28 = 14\;{\text{cm}}$

Therefore, Semi-perimeter of triangle

$ = \dfrac{{18 + 10 + 14}}{2} = 21\;{\text{cm}}$

Area of triangle $ = \sqrt {s(s - a)(s - b)(s - c)} $

$ = \sqrt {21(21 - 18)(21 - 10)(21 - 14)} $

$ = \sqrt {21 \times 3 \times 11 \times 7} $

$ = \sqrt {7 \times 3 \times 3 \times 11 \times 7} $

$ = 21\sqrt {11} $

$ = 21 \times 3.3$

$ = 69.3\;{\text{c}}{{\text{m}}^2}$.

3. Sides of a triangle are in the ratio of \[12:17:25\] and its perimeter is $540\;{\text{cm}}$. Find its area.

Ans: Let the sides of the triangle be 12x, 17x and 25x 

Therefore, $12x + 17x + 15x = 540$

$\Rightarrow 54x = 540 \Rightarrow x = 10$

- The sides are $120\;{\text{cm}},170\;{\text{cm}}$ and $250\;{\text{cm}}$.

Semi-perimeter of triangle $(s) = \dfrac{{120 + 170 + 250}}{2} = 270\;{\text{cm}}$

Now, Area of triangle $= \sqrt {s(s - a)(s - b)(s - c)}$

$\sqrt {270(270 - 120)(270 - 170)(270 - 250)}$

$= \sqrt {270 \times 150 \times 100 \times 20}$

$= 9000\;{\text{c}}{{\text{m}}^2}$

4. An isosceles triangle has perimeter $30\;{\text{cm}}$ and each of the equal sides is $12\;{\text{cm}}$. Find the area of the triangle.

Ans: Given: $a = 12\;{\text{cm}},b = 12\;{\text{cm}}$

Since Perimeter $ = 30\;{\text{cm}} \Rightarrow a + b + c = 30$

$\Rightarrow 12 + 12 + c = 30$

$\Rightarrow c = 30 - 24 = 6\;{\text{cm}}$

Semi-perimeter of triangle $ = \dfrac{{12 + 12 + 6}}{2} = 15\;{\text{cm}}$

$\therefore $ Area of triangle $ = \sqrt {s(s - a)(s - b)(s - c)} $

$\sqrt {15(15 - 12)(15 - 12)(15 - 6)} $

$ = \sqrt {15 \times 3 \times 3 \times 9} $

$ = \sqrt {5 \times 3 \times 3 \times 3 \times 3 \times 3} $

$ = 9\sqrt {15} \;{\text{c}}{{\text{m}}^2}$

5. A park, in the shape of a quadrilateral ${\text{ABCD}}$ has $\angle {\text{C}} = {90^\circ },{\text{AB}} = 9\;{\text{m}},{\text{BC}} = 12\;{\text{m}},{\text{CD}} = $

$5\;{\text{m}}$ and ${\text{AD}} = 8\;{\text{m}}$. How much area does it occupy?

(Image will be uploaded soon)

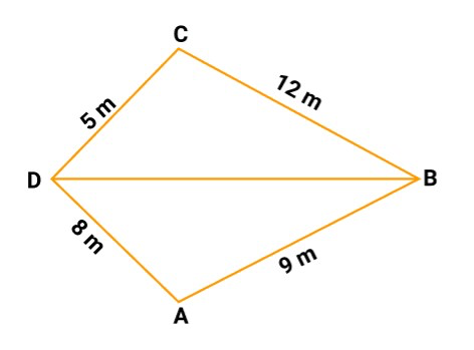
Ans: Since \[BD\] divides quadrilateral \[ABCD\] in two triangles:

(i) Right triangle ${\text{BCD}}$ and (ii) $\Delta {\text{ABD}}$.

In right triangle ${\text{BCD}}$, right angled at ${\text{C}}$,

Therefore, Base $ = {\text{CD}} = 5\;{\text{m}}$ and Altitude $ = {\text{BC}} = 12\;{\text{m}}$

Area of $\Delta {\text{BCD}} = \dfrac{1}{2} \times CD \times BC$

$ = \dfrac{1}{2} \times 5 \times 12 = 30\;{{\text{m}}^2}$

In $\vartriangle ABD,AB = 9\;{\text{m}},AD = 8\;{\text{m}}$

And ${\text{BD}} = \sqrt {{\text{C}}{{\text{D}}^2} + {\text{B}}{{\text{C}}^2}} $ [Using Pythagoras theorem]

$\Rightarrow {\text{BD}} = \sqrt {{{(5)}^2} + {{(12)}^2}} $

$ = \sqrt {25 + 144}  = \sqrt {169}  = 13\;{\text{m}}$

Semi=perimeter of $\Delta {\text{ABD}} = \dfrac{{9 + 8 + 13}}{2} = 15\;{\text{m}}$

Using Heron's formula,

Area of $\Delta {\text{ABD}} = \sqrt {s(s - a)(s - b)(s - c)} $

$ = \sqrt {15(15 - 9)(15 - 8)(15 - 13)} $

$ = \sqrt {15 \times 6 \times 7 \times 2} $

$ =  = 6\sqrt {35}  = 6 \times 5.91\;{{\text{m}}^2}$

$ = 35.4\;{{\text{m}}^2}$ (approx.)

Area of quadrilateral ${\text{ABCD}} = $ Area of $\Delta {\text{BCD}} + $ Area of $\Delta {\text{ABD}}$

$ = 30 + 35.4$

$ = 65.4\;{{\text{m}}^2}$

6. Find the area of a quadrilateral ${\text{ABCD}}$ in which ${\text{AB}} = 3\;{\text{cm}},{\text{BC}} = 4\;{\text{cm}},{\text{CD}} = 4\;{\text{cm}},{\text{DA}} = {\text{s}}$

${\text{cm}}$ and ${\text{AC}} = 5\;{\text{cm}}$.

(Image will be uploaded soon)

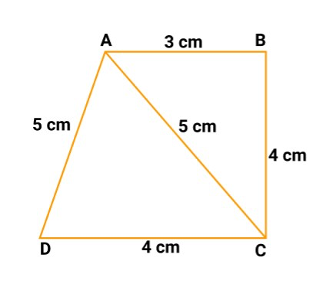
Ans: In quadrilateral \[\;ABCE\], diagonal \[AC\] divides it in two triangles, $\Delta {\text{ABC}}$ and $\Delta {\text{ADC}}$.

In $\Delta {\text{ABC}}$, Semi-perimeter of $\Delta {\text{ABC}} = \dfrac{{3 + 4 + 5}}{2} = 6\;{\text{cm}}$

Using Heron's formula,

Area of $\Delta {\text{ABC}} = \sqrt {s(s - a)(s - b)(s - c)} $

$ = \sqrt {6(6 - 3)(6 - 4)(6 - 5)} $

$ = \sqrt {6 \times 3 \times 2 \times 1}  = 6\;{\text{c}}{{\text{m}}^2}$

Again, In $\vartriangle {\text{ADC}}$, Semi-perimeter of $\Delta {\text{ADC}} = \dfrac{{4 + 5 + 5}}{2} = 7\;{\text{cm}}$

Using Heron's formula, Area of $\Delta {\text{ABC}} = \sqrt {s(s - a)(s - b)(s - c)} $

$ = \sqrt {7(7 - 4)(7 - 5)(7 - 5)} $

$ = \sqrt {7 \times 3 \times 2 \times 2}  = 2\sqrt {21} $

$ = 2 \times 4.6 = 9.2\;{\text{c}}{{\text{m}}^2}$ (approx.)

Area of quadrilateral ${\text{ABCD}} = $ Area of $\Delta {\text{ABC}} + $ Area of $\Delta {\text{ADC}}$

$ = 6 + 9.2$

$ = 15.2\;{\text{c}}{{\text{m}}^2}$

7. A triangle and a parallelogram have the same base and the same area. If the sides of the triangle are $26\;{\text{cm}},29\;{\text{cm}}$ and $30\;{\text{cm}}$ and the parallelogram stands on the base$28\;{\text{cm}}$, find the height of the parallelogram.

(Image will be uploaded soon)

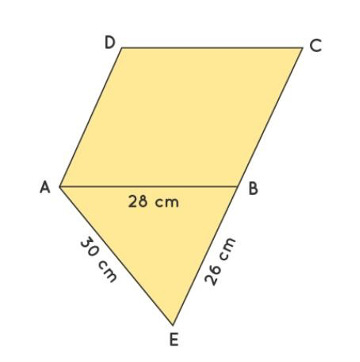
Ans: For $\triangle \mathrm{ABE}, \mathrm{a}=30 \mathrm{~cm}, \mathrm{~b}=26 \mathrm{~cm}, \mathrm{c}=28 \mathrm{~cm}$

Semi Perimeter: $(\mathrm{s})=$ Perimeter $/ 2$

$s=(a+b+c) / 2$

$=(30+26+28) / 2$

$=42 \mathrm{~cm}$

By using Heron's formula,

Area of a $\triangle A B E=\sqrt{s(s-a)(s-b)(s-c)}$

$=\sqrt{42(42-30)(42-28)(42-26)}$ 

$=\sqrt{42 \times 12 \times 14 \times 16}$ $=336 \mathrm{~cm}^{2}$

Area of parallelogram $A B C D=$ Area of $\triangle A B E$ (given)

Base $\times$ Height $=336 \mathrm{~cm}^{2}$

$28 \mathrm{~cm} \times$ Height $=336 \mathrm{~cm}^{2}$

On rearranging, we get

Height $=336 / 28 \mathrm{~cm}=12 \mathrm{~cm}$

Thus, height of the parallelogram is $12 \mathrm{~cm}$.

8. A kite is in the shape of a square with a diagonal $32\;{\text{cm}}$ and an isosceles triangle of base $8\;{\text{cm}}$ and sides $6\;{\text{cm}}$ each is to be made of three different shades as shown in figure.

(Image will be uploaded soon)

How much paper of each side has been used in it?

Ans: Heron's formula for the area of a triangle $=\sqrt{s(s-a)(s-b)(s-c)}$

Where $a, b$, and $c$ are the sides of the triangle, and

$s=$ Semi-perimeter $=$ Half the Perimeter of the triangle $=(a+b+c) / 2$

Given diagonal $B D=A C=32 \mathrm{~cm}$, then $O A=1 / 2 A C=16 \mathrm{~cm}$.

So square $A B C D$ is divided into two isosceles triangles $A B D$ and $C B D$ of base $32 \mathrm{~cm}$ and height $16 \mathrm{~cm}$.

Area of $\triangle \mathrm{ABD}=1 / 2 \times$ base $\times$ height $=(32 \times 16) / 2$ $=256 \mathrm{~cm}^{2}$

Since the diagonal divides the square into two equal triangles. Therefore,

Area of $\triangle \mathrm{ABD}=$ Area of $\Delta \mathrm{CBD}=256 \mathrm{~cm}^{2}$

Now, for $\Delta C E F$

Semi Perimeter(s) $=(a+b+c) / 2$

$s=(6+6+8) / 2$

$s=20 / 2$

$s=10 \mathrm{~cm}$

By using Heron's formula,

Area of $\Delta C E F=\sqrt{s(s-a)(s-b)(s-c)}$

$=\sqrt{10(10-6)(10-6)(10-8)}$

$=\sqrt{10 \times 4 \times 4 \times 2}$

$=8 \sqrt{5}$

$=8 \times 2.24$

$=17.92 \mathrm{~cm}^{2}$

Thus, the area of the paper used to make region $\mathrm{I}=256 \mathrm{~cm}^{2}$, region $\mathrm{II}=$ $256 \mathrm{~cm}^{2}$, and region $I I I=17.92 \mathrm{~cm}^{2}$

10. The perimeter of a rhombus ABCD is $40\;{\text{cm}}$. find the area of rhombus of Its diagonals \[BD\] measures $12\;{\text{cm}}$

Ans: $\text { Side }=\dfrac{\text { perimeter }}{4}=10 \mathrm{~cm}$

(Image will be uploaded soon)

$10^{2}-6^{2}=\mathrm{x}^{2}$

$\mathrm{x}^{2}=64 \Rightarrow \mathrm{x}=8$

$\therefore \text { other diagonal }=2 \mathrm{x}=16 \mathrm{~cm}$

$\therefore \text { Area }=\dfrac{1}{2} \mathrm{~d}_{1} \mathrm{~d}_{2}$

$=\dfrac{1}{2} \times 16 \times 12=16 \times 6=96 \mathrm{~cm}^{2}$

11. Find area of triangle with two sides as $18\;{\text{cm \& }}10\;{\text{cm}}$ and the perimeter is $42\;{\text{cm}}$.

Ans: Let $a = 18\;{\text{cm}},\;{\text{b}} = 10\;{\text{cm}}$

Perimeter $ = 42\;{\text{cm}}$

$a + b + c = 42\;{\text{cm}}$

So, ${\text{C}} = 14\;{\text{cm}}$

$S = \dfrac{{a + b + c}}{2} = \dfrac{{18 + 10 + 14}}{2} = 21\;{\text{cm}}$

new area of triangles $ = \sqrt {21(21 - 18)(21 - 10)(21 - 14)} $

$ = \sqrt {21 \times 3 \times 11 \times 7} $

$ = 21\sqrt {11} {\text{sqcm}}$

12. Find the area of in isosceles triangle, the measure of one of Its equals side being $ \cdot b$ and the third side ' ${\text{a}}$ '.

Ans: Here

$S = \dfrac{{a + b + c}}{2}$ units $ = \dfrac{{a + 2b}}{2}$ units

$\therefore $ area of $\Delta  = \sqrt {\left( {\dfrac{{a + 2b}}{2}} \right)\left( {\dfrac{{a + 2b}}{2} - a} \right)\left( {\dfrac{{a + 2b}}{2} - b} \right)\left( {\dfrac{{a + 2b}}{2} - c} \right)} $

$ = \sqrt {\left( {\dfrac{{a + 2b}}{2}} \right)\left( {\dfrac{{2b - a}}{2}} \right)\dfrac{a}{2} \times \dfrac{a}{2}} sq\,units$ 

$ = \dfrac{a}{4}\sqrt {4{b^2} - {a^2}} sq$ units

13. Find the cost of leveling the ground in the form of a triangle having its sides are

${\text{40m}},70\;{\text{m}}$ and $90\;{\text{m}}$ at \[Rs{\text{ }}8\]per square meter. (use $\sqrt 5  = 2.24$)

Ans: Here ${\text{S}} = \dfrac{{40 + 70 + 90}}{2}\;{\text{m}} = 100\;{\text{m}}$

- Area of a triangular ground $ = \sqrt {100(100 - 40)(100 - 70)(100 - 90)} sq\,m$ 

$ = \sqrt {100 \times 60 \times 30 \times 10} {\text{sqm}}$

$ = (10 \times 10 \times \sqrt[6]{5}){\text{sqm}}$

$ = (600 \times 2.24){\text{sqm}}$

$ = 1344sq\,m$ 

- Cost of leveling the ground $ = \operatorname{Rs} (8 \times 1344)$

$ = \operatorname{Rs} 10752$

14. The triangular side's walls of a flyover have been used for advertisements. The sides of the walls are $122\;{\text{m}},22\;{\text{m}}$ and $120\;{\text{m}}$. The advertisement yield on earning of \[Rs{\text{ }}5000\]per${{\text{m}}^2}$ per year. A company hired one of its walls for \[4\] months. How much rent did it pay?

(Image will be uploaded soon)

Ans: The lengths of the sides of the walls are $122\;{\text{m}},22\;{\text{m}}$ and $120\;{\text{m}}$.

As,

${120^2} + {22^2}$

$ = 14400 + 484$

$ = 14884$

$ = {(122)^2}$

$\therefore $ Walls are in the form of right triangles

Area of one wall $ = \dfrac{1}{2} \times $Base $ \times $ height

$ = \dfrac{1}{2} \times 120 \times 22\operatorname{sq} {\text{m}}$

$ = 1320\operatorname{sq} {\text{m}}$ 

Rent $ = Rs\,5000/\operatorname{sq} {\text{m}}$ per year

$\therefore $ Rent for \[4\] month $ = \operatorname{Rs} \left[ {\dfrac{{5000 \times 1320 \times 4}}{{12}}} \right]$\[ = Rs\,22,00,000\].

15. Find the perimeter and area of a triangle whose sides are of length $2\;{\text{cm}},5\;{\text{cm}}$ and ${\text{5}}\,{\text{cm}}.$

Ans: Here, $a = 2\;{\text{cm}},\;{\text{b}} = 5\;{\text{cm}}$ and ${\text{c}} = 5\;{\text{cm}}$

$\therefore $ Perimeter $ = a + b + c = (2 + 5 + 5) = 12\;{\text{cm}}$

${\text{S}} = $ semi perimeter

$ = \dfrac{{12}}{2} = 6\;{\text{cm}}$

Using Heron's formula,

$\therefore $ Area of triangle $ = \sqrt {s(s - a)(s - b)(s - c)} sq\,cm$ 

$ = \sqrt {6(6 - 2)(6 - 5)(6 - 5)} {\text{sqcm}}$

$ = \sqrt {24} {\text{sqcm}}$

$ = 4.9{\text{sqcm}}$

16. There is a slide in a park. One of its sides wall has been painted in some colour with a message 'KFF.F THE CITY GRFFN AND CLEAN".

If the sides of the wall are $15\;{\text{m}},11\;{\text{m}}$ and $6\;\,{\text{m}}$. Find the area painted in colour.

Ans: The sides of the wall is in the triangular from with sides,

$A = 15\;{\text{m}},b = 6\;{\text{m}}$ and $c = 11\;{\text{m}}$

$\therefore s = \dfrac{{15 + 6 + 11}}{2}\;{\text{m}}$

$ = 16\;{\text{m}}$

- Area to be painted in colour = Areas of the side wall

$\sqrt {s(t - a)(s - b)(s - c)} \operatorname{sq} cm$

$\sqrt {16(16 - 5)(16 - 6)(16 - 11)} sq\,m$ 

$ = \sqrt[4]{{50}}{\text{sqm}}$

$ = \sqrt[2]{2}sq\,m$ 

17. Find the area of isosceles triangle whose side is $14\;{\text{m}},12\;{\text{m}},14\;{\text{m}}$ ?

Ans: Find the semi perimeter,

$S = \dfrac{{14 + 12 + 14}}{2} = 20{\text{mt}}$

Ares of isosceles triangle $ = \sqrt {s(s - a)(s - b)(s - c)} $

$ = \sqrt {20(20 - 14)(20 - 12)(20 - 14)} $

$ = \sqrt {20 \times 6 \times 8 \times 6} $

$ = 6\sqrt {160}  = 6 \times 12.6$

$ = 75.6$

18. The perimeter af a rhombus ${\text{MBCD}}$ is $60\;{\text{cm}}$. find the area of the rhombus of Its diagonal \[BD\] measures $16\;{\text{cm}}?$

(Image will be uploaded soon)

Ans: As side of rhombus are equal.

$\therefore AB = BC = CD = DA = \dfrac{{60}}{4} = 15\;{\text{cm}}$

$in\Delta {\text{ABD}}$

$S = \dfrac{{15 + 15 + 16}}{2} = 23\;{\text{cm}}$

So,

Area of, $\vartriangle {\text{ABD}} = \sqrt {23(23 - 15)(23 - 15)(23 - 16)} $

$= \sqrt {23 \times 8 \times 8 \times 7}  = 8\sqrt {23 \times 7}$

$= 8 \times 12.7$

$= 101.6\,{\text{sq}}\,{\text{cm}}$ 

Area of rhombus $= 2 \times 101.6$

$= 203.2\,{\text{sq}}\,{\text{cm}}$ 

19. Find the cost of leveling the ground in the from of a triangle having Its side as $70\;{\text{cm}}$, $50\;{\text{cm}}$, and $60\;{\text{cm}}$, at \[Rs{\text{ }}7\]per square meter.

Ans: Find the perimeter

$S = \dfrac{{70 + 50 + 60}}{2}$

$ = \dfrac{{180}}{2}$

$ = 90\;\,{\text{cm}}$

$\therefore $ area of triangle $ = \sqrt {90(90 - 70)(90 - 50)(90 - 60)} $

$ = \sqrt {90 \times 20 \times 40 \times 30} $

$ = 1469.7\,{\text{sq}}\,{\text{m}}$

Cost of leveling the ground $ = {\text{RS}}(7 \times 1469.7)$

$ = 10287.9$

20. Find the area of a triangle two side of the triangle are $18\;{\text{cm}}$, and $12\;{\text{cm}}$. and the perimeter is $40\;{\text{cm}}$.

Ans: Let ${\text{a}} = 18\;{\text{cm}},\;{\text{b}} = 12\;{\text{cm}}$ and ${\text{C}} = $ ?

${\text{So}},{\text{a}} + {\text{b}} + {\text{c}} = 40\;{\text{cm}}$

$18 + 12 + {\text{C}} = 40$

$C = (40 - 30){\text{cn}} = 10\;{\text{cm}}$

${\text{S}} = \dfrac{{18 + 12 + 10}}{2} = 20\;\,{\text{cm}}$

Therefore, the area of triangle $ = \sqrt {20(20 - 18)(20 - 12)(20 - 10)} $

$ = \sqrt {20 \times 2 \times 8 \times 10} \,{\text{sq}}\,{\text{cm}}$

$ = 56.56\,{\text{sq}}\,{\text{cm}}$

21. Find the area of triangle whose side is $42\;{\text{m}},56\;{\text{m}}$ and $70\;{\text{m}}$ ?

Ans: Find the semi perimeter

$S = \dfrac{{42 + 56 + 70}}{2}\;{\text{m}} = \dfrac{{168}}{2}\;{\text{m}}$ or \[84\]

$\therefore $ Area of $\Delta {\text{ABC}} = \sqrt {s(\;{\text{s}} - {\text{a}})({\text{s}} - {\text{b}})({\text{s}} - {\text{c}})} $

$ = \sqrt {84(84 - 42)(84 - 56)(84 - 70)} \,{\text{sq}}\,{\text{m}}$

$ = 42 \times 28\,{\text{sq}}\,{\text{cm}}$ 

$ = 1176\,{\text{sq}}\,{\text{cm}}$ 

22. Find the area of an isosceles triangle, the measure of one of Its equal side being \[b\] and the third side \[a.\]

Ans: Find the perimeter,

${\text{S}} = \dfrac{{a + b + b}}{2}$ units 

$ = \dfrac{{a + 2b}}{2}$ units

Area of triangle $ = \sqrt {\dfrac{{a + 2b}}{2} \times \left( {\dfrac{{a + 2b}}{2} - a} \right)\left( {\dfrac{{a + 2b}}{2} - a} \right)\left( {\dfrac{{a + 2b}}{2} - a} \right)} \,{\text{units}}$ 

$ = \sqrt {\left( {\dfrac{{a + 2b}}{2}} \right) \times \left( {\dfrac{{2b - a}}{2}} \right) \times \dfrac{a}{2} \times \dfrac{a}{2}} $ 

$ = \dfrac{a}{4}\sqrt {4{b^2} - {a^2}} {\text{ sq}}\,{\text{units}}$ 

23. Find the area of equilateral triangle whose side is $12\;{\text{cm}}$ using Heron's formula.

Ans: Find the area of equilateral triangle,

${\text{S}} = \dfrac{{12 + 12 + 12}}{2}\;{\text{cm}}$

$ = \dfrac{{36}}{2}\;{\text{cm}} = 18\;{\text{cm}}$

$\therefore $ Area of equilateral $ = \sqrt {s(s - a)(s - b)(s - c)} $

$ = \sqrt {18(18 - 12)(18 - 12)(18 - 12)} $

$ = \sqrt {18 \times 6 \times 6 \times 6} $

$ = 36\sqrt 3 {\text{sqcm}}$

24. Find the area of isosceles triangle whose equal side is $6\;{\text{cm}},6\;{\text{cm}}$ and $8\;{\text{cm}}$.

Ans: Find the area of isosceles triangle,

$S = \dfrac{{6 + 6 + 8}}{2}\;{\text{cm}}$

$ = \dfrac{{20}}{2} = 10\;{\text{cm}}$

$\therefore $ Area of isosceles triangle $ = \sqrt {10(10 - 6)(10 - 6)(10 - 8)} $

$ = \sqrt {10 \times 4 \times 4 \times 2} \,{\text{sq}}\,{\text{cm}}$

$ = 17.8\,{\text{sq}}\,{\text{cm}}$

25. Find the area of an isosceles triangles, the measure of one of its equal sides being 10 ${\text{cm}}$ and the third side is $6\;{\text{cm}}$.

Ans: $S = \dfrac{{10 + 10 + 6}}{2} = \dfrac{{26}}{2} = 13\;{\text{cm}}$

$\therefore $ Area if triangle $ = \sqrt {13(13 - 5)(13 - 5)(13 - 6)} \,{\text{sq}}\,{\text{cm}}$

$ = \sqrt {13 \times 3 \times 3 \times 7} \,{\text{sq}}\,{\text{cm}}$

\[ = 3\sqrt {91} \,{\text{sq}}\,{\text{cm}}\]

26. Find the area of equilateral triangle the length of one of its sides being $24\;{\text{cm}}$.

Ans: Let,${\text{a}} = {\text{b}} = {\text{c}} = 24\;{\text{cm}}$

${\text{S}} = \dfrac{{24 + 24 + 24}}{2}\,\;{\text{cm}}$

${\text{  }} = \dfrac{{72}}{2}\;\,{\text{cm}}$

$ = 36\;{\text{cm}}$

$\therefore $ Area of triangle $ = \sqrt {36(36 - 24)(36 - 24)(36 - 24)} sq\,cm$ 

$ = 246.12\,{\text{sq}}\,{\text{cm}}$ 

27. Find the perimeter and area of a triangle whose sides are $3\;{\text{cm}},4\;{\text{cm}}$ and $10\;{\text{cm}}?$

Ans: Perimeter $ = 3 + 4 + 5$

$ = 12\;{\text{cm}}$

$\therefore {\text{S}} = $ semi perimeter $ = \dfrac{{12}}{2}$

${\text{Or}} = 6\;{\text{cm}}$

Area of triangle $ = \sqrt {6(6 - 3)(6 - 4)(6 - 5)} \,{\text{sq}}\,{\text{cm}}$ 

$ = 6\,{\text{sq}}\,{\text{cm}}$

28. Using Heron's formula, find area of triangle whose sides are $6\;{\text{cm}},8\;{\text{cm}}$ and $10\;{\text{cm}}?$

Ans: Find the area of the triangle,

$S = \dfrac{{6 + 8 + 10}}{2}$

$\,\,\,\, = \dfrac{{24}}{2}$ 

$ = 12\;{\text{cm}}$

Area of triangle $ = \sqrt {12(12 - 6)(12 - 8)(12 - 10)} \,{\text{sq}}\,{\text{cm}}$ 

 $ = 24{\text{sqcm}}$.

3 Marks Questions:

1. A traffic signal board, indicating 'SCHOOLAHEAD' is an equilateral triangle with side \['a'\]. Find the area of the signal board, using Heron's formula. If its perimeter is $180\;{\text{cm}}$, what will be the area of the signal board?

(Image will be uploaded soon)

Ans: Let the Traffic signal board is $\Delta {\text{ABC}}$. According to question, Semi-perimeter of $\Delta {\text{ABC}}(s) = \dfrac{{a + a + a}}{2} = \dfrac{{3a}}{2}$ Using Heron's Formula, Area of triangle ${\text{ABC}} = \sqrt {s(s - a)(s - b)(s - c)} $

$ = \sqrt {\dfrac{{3a}}{2}\left( {\dfrac{{3a}}{2} - a} \right)\left( {\dfrac{{3a}}{2} - a} \right)\left( {\dfrac{{3a}}{2} - a} \right)} $

$= \sqrt {\dfrac{{3a}}{2} {\times} \dfrac{a}{2} {\times} \dfrac{a}{2} {\times } \dfrac{a}{2}}$

$= \sqrt {3{{\left( {\dfrac{a}{2}} \right)}^4}}$ 

$ = \dfrac{{\sqrt 3 {a^2}}}{4}$

Now, Perimeter of this triangle $ = 180\;{\text{cm}}$

$\Rightarrow $ Side of triangle $(a) = \dfrac{{180}}{3} = 60\;{\text{cm}}$

$\Rightarrow $ Semi-perimeter of this triangle $ = \dfrac{{180}}{2} = 90\;{\text{cm}}$

Using Heron's Formula, Area of this triangle $ = \sqrt {s(s - a)(s - b)(s - c)} $

$ = \sqrt {90(90 - 60)(90 - 60)(90 - 60)} $

$ = \sqrt {90 \times 30 \times 30 \times 30} $

$ = 30 \times 30\sqrt 3 $

$ = 900\sqrt 3 \;{\text{c}}{{\text{m}}^2}$

2. The triangular side walls of a flyover has been used for advertisements. The sides of the walls are $122\;{\text{m}},22\;{\text{m}}$ and $120\;{\text{m}}$ (see figure). The advertisement yieldan earning of \[Rs.{\text{ }}5000/{{\text{m}}^{\text{2}}}\]per year. A company hired one of its walls for \[3\]months, how much rent did it pay?

(Image will be uploaded soon)

Ans: Given: $a = 122\;{\text{m}},b = 22\;{\text{m}}$ and $c = 120\;{\text{m}}$

Semi-perimeter of triangle $(s) = \dfrac{{122 + 22 + 120}}{2}$

$\,\,\,\,\,\,\, = \dfrac{{264}}{2}$

$\,\,\,\,\,\,\, = 132\;{\text{m}}$ 

Using Heron's Formula,

Area of triangle $= \sqrt {s(s - a)(s - b)(s - c)}$

$= \sqrt {132(122 - 132)(132 - 22)(132 - 120)}$

$= \sqrt {132 \times 10 \times 110 \times 12}$

$= \sqrt {11 \times 12 \times 10 \times 10 \times 11 \times 12}$

$= 10 \times 11 \times 12$

$= 1320\;{{\text{m}}^2}$

Rent for advertisement on wall for \[1\] year $= Rs{\text{ }}5000/{{\text{m}}^{\text{2}}}$

$\therefore$ Rent for advertisement on wall for \[3\] months for $1320{m^2} = \dfrac{{5000}}{{12}} \times 3 \times 1320$

\[ = Rs\,1650000\]

Hence rent paid by company\[ = Rs\,1650000\]. 

3. Radha made a picture of an aeroplane with coloured paper as shown in figure. Find the total area of the paper used.

Ans: Area of triangular part I: 

Here, Semi-perimeter \[(s) = \dfrac{{5 + 5 + 1}}{2} = 5.5\;{\text{cm}}\]

Therefore, Area $= \sqrt {s(s - a)(s - b)(s - c)}$

$= \sqrt {5.5(5.5 - 5)(5.5 - 5)(5.5 - 1)}$

$= \sqrt {5.5 \times 0.5 \times 0.5 \times 4.5}  = 0.75\sqrt {11}$

$= 0.75 \times 3.31$

$= 2.4825\;{\text{c}}{{\text{m}}^2}$ 

Area of triangular part II = Length $ \times $ Breadth

$= 6.5 \times 1 = 6.5\;{\text{c}}{{\text{m}}^2}$

Area of triangular part III (trapezium): $= \dfrac{1}{2}(AB + DC) \times AE$

$= \dfrac{1}{2}(AB + DC) \times \sqrt {A{D^2} - D{E^2}} $

$= \dfrac{1}{2}(1 + 2) \times \sqrt {1 - .025}$

$= \dfrac{1}{2} \times 3 \times \dfrac{{\sqrt 3 }}{2}$

$= \dfrac{{3 \times 1.732}}{4}$

$= 1.299\;{\text{c}}{{\text{m}}^2}$

Area of triangular parts $IV{\text{ }}\& {\text{ }}V = 2\left( {\dfrac{1}{2} \times 1.5 \times 6} \right)$

$= 9\;{\text{c}}{{\text{m}}^2}$

$\therefore $ Total area $ = 2.4825 + 6.2 + 1.299 + 9$

$= 19.28\;{\text{c}}{{\text{m}}^2}$

4. A rhombus shaped field has green grass for \[18\] cows to graze. If each side of the rhombus is $30\;{\text{m}}$ and its longer diagonal is $48\;{\text{m}}$, grass of how much area of grass field will each cow be getting?

Ans: Here, $AB = BC = CD = DA = 30\;{\text{m}}$ and Diagonal $AC = 48\;{\text{m}}$ which divides the rhombus \[ABCD\] in two congruent triangle.

$\therefore $ Area of $\Delta {\text{ABC}} = $ Area of $\Delta {\text{ACD}}$

Semi-perimeter of $\Delta {\text{ABC}}(s) = \dfrac{{30 + 30 + 48}}{2} = 54\;{\text{m}}$

Now Area of rhombus ${\text{ABCD}} = $ Area of $\Delta {\text{ABC}} + $ Area of $\Delta {\text{ACD}}$

$= 2{\text{x}}$ Area of $\Delta {\text{ABC}}[\because $ Area of $\Delta {\text{ABC}} = $ Area of $\Delta {\text{ACD}}]$

$ = 2\sqrt {s(s - a)(s - b)(s - c)} $ [ Using Heron's formula]

$ = 2 \times \sqrt {54(54 - 30)(54 - 30)(54 - 48)} $

$ = 2 \times \sqrt {54 \times 24 \times 24 \times 6}  = 2 \times 6 \times 24$

$ = 864\;{{\text{m}}^2}$

$\because $ Field available for 18 cows to graze the grass $ = 864{m^2}$

$\therefore $ Field available for 1 cow to graze the grass $ = \dfrac{{864}}{{18}} = 48\;{{\text{m}}^2}$

5. An umbrella is made by stitching \[10\] triangular pieces of cloth of two different colours (see figure), each piece measuring $20\;{\text{cm}},50\;{\text{cm}}$ and $50\;{\text{cm}}.$ How much cloth of each colour is required for the umbrella?

Ans: Here, sides of each of 10 triangular pieces of two different colours are $20\;{\text{cm}},50\;{\text{cm}}$ and

$50\;{\text{cm}}.$

(Image will be uploaded soon)

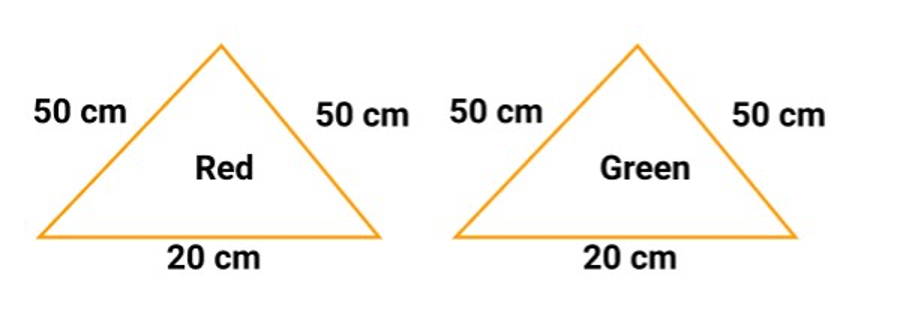
Semi-perimeter of each triangle \[(s) = \dfrac{{20 + 50 + 50}}{2} = 60\;{\text{cm}}\]

Now, Area of each triangle $ = \sqrt {s(s - a)(s - b)(s - c)} $

$ = \sqrt {60(60 - 20)(60 - 50)(60 - 50)} $

$ = \sqrt {60 \times 40 \times 10 \times 10}  = 200\sqrt 6 \;{\text{c}}{{\text{m}}^2}$

According to question, there are 5 pieces of red colour and \[5\] pieces of green colour.

Cloth required for \[5\] red pieces $ = 5 \times 200\sqrt 6  = 1000\sqrt 6 \;{\text{c}}{{\text{m}}^2}$

And Cloth required to \[5\] green pieces $ = 5 \times 200\sqrt 6  = 1000\sqrt 6 \;{\text{c}}{{\text{m}}^2}$

6. A floral design on a floor is made up of 16 tiles which are triangular, the sides of the triangle being $9\;{\text{cm}},28\;{\text{cm}}$ and $35\;{\text{cm}}$ (see figure). Find the cost of polishing the tiles at the rate of \[50\] paise per ${\text{c}}{{\text{m}}^2}$.

(Image will be uploaded soon)

Ans: Here, Sides of a triangular shaped tile area $9\;{\text{cm}},28\;{\text{cm}}$ and $35\;{\text{cm}}$.

Semi-perimeter of tile $(s) = \dfrac{{9 + 28 + 35}}{2} = 36\;{\text{cm}}$

Area of triangular shaped tile $ = \sqrt {s(s - a)(s - b)(s - c)} $

$ = \sqrt {36(36 - 9)(36 - 28)(36 - 35)} $

$ = \sqrt {36 \times 27 \times 8 \times 1}  = 36\sqrt 6 $

$ = 36 \times 2.45 = 88.2\;{\text{c}}{{\text{m}}^2}$ (approx.)

$\therefore $ Area of 16 such tiles $ = 16 \times 88.2 = 1411.2\;{\text{c}}{{\text{m}}^2}$ (Approx.)

$\because $ Cost of polishing $1\;{\text{c}}{{\text{m}}^2}$ of tile \[ = {\text{ }}Rs.0.50\]

$\therefore $ Cost of polishing $1411.2\;{\text{c}}{{\text{m}}^2}$ of tile \[ = {\text{ }}Rs.\;0.50 \times 1411.2 = Rs.{\text{ }}705.60\](Approx.)

7. A field is in the shape of a trapezium whose parallel sides are $25\;{\text{m}}$ and $10\;{\text{m}}$. The non-parallel sides are $14\;{\text{m}}$ and $13\;{\text{m}}$. Find the area of the field.

(Image will be uploaded soon)

Ans: Let $\mathrm{ABCD}$ be a trapezium with,

$\mathrm{AB} \| \mathrm{CD}$

$\mathrm{AB}=25 \mathrm{~m}$

$\mathrm{CD}=10 \mathrm{~m}$

$\mathrm{BC}=14 \mathrm{~m}$

$\mathrm{AD}=13 \mathrm{~m}$

Draw CE $\|$ DA. So, ADCE is a parallelogram with,

$\mathrm{CD}=\mathrm{AE}=10 \mathrm{~m}$

$\mathrm{CE}=\mathrm{AD}=13 \mathrm{~m}$

$\mathrm{BE}=\mathrm{AB}-\mathrm{AE}=25-10=15 \mathrm{~m}$

In $\Delta \mathrm{BCE}$, the semi perimeter will be,

$\mathrm{s}=\dfrac{\mathrm{a}+\mathrm{b}+\mathrm{c}}{2}$

$\mathrm{~s}=\dfrac{14+13+15}{2}$

$\mathrm{~s}=21 \mathrm{~m}$

Area of $\Delta \mathrm{BCE}$,

$\mathrm{A}=\sqrt{\mathrm{s}(\mathrm{s}-\mathrm{a})(\mathrm{s}-\mathrm{b})(\mathrm{s}-\mathrm{c})}$

$=\sqrt{21(21-14)(21-13)(21-15)}$

$=\sqrt{21(7)(8)(6)}$

$=\sqrt{7056}$

$=84 \mathrm{~m}^{2}$

Also, area of $\Delta \mathrm{BCE}$ is,

$\mathrm{A}=\dfrac{1}{2} \times \text { base } \times \text { height }$

$84=\dfrac{1}{2} \times 15 \times \mathrm{CL}$

$\dfrac{84 \times 2}{15}=\mathrm{CL}$

$\mathrm{CL}=\dfrac{56}{5} \mathrm{~m}$

Now, the area of trapezium is,

$\mathrm{A}=\dfrac{1}{2} \times(25+10)\left(\dfrac{56}{5}\right)$

$\mathrm{A}=196 \mathrm{~m}^{2}$

Therefore, the area of the trapezium is $196 \mathrm{~m}^{2}$.

8. From a point in the interior of an equilateral triangle perpendiculars drawn to the three sides are $8\;{\text{cm}},10\;{\text{cm}}$ and $11\;{\text{cm}}$ respectively. Find the area of the triangle to the nearest ${\text{cm}}$. (use $\sqrt 3  = 1.73$ )

(Image will be uploaded soon)

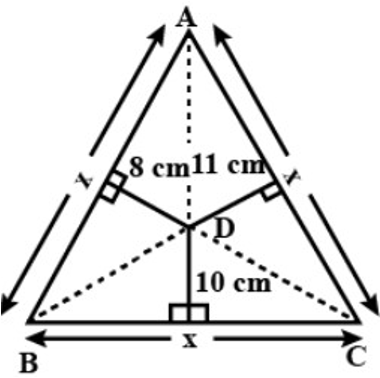
Ans: Let $x$ be the side of an equilateral triangle.

Therefore, its area $=\dfrac{\sqrt{3}}{4} \mathrm{x}^{2}$

Also, area $\mathrm{ABC}=\operatorname{ar}(\Delta \mathrm{ADB})+\operatorname{ar}(\Delta \mathrm{BDC})+\operatorname{ar}(\Delta \mathrm{CDA})$

$=\dfrac{1}{2} \times \mathrm{x} \times 8+\dfrac{1}{2} \times \mathrm{x} \times 10+\dfrac{1}{2} \times \mathrm{x} \times 11$

$=\dfrac{1}{2} \mathrm{x} \times(8+10+11)=14.5 \mathrm{x}$

Again, both areas are equal

$\because \dfrac{\sqrt{3}}{4} \mathrm{x}^{2}=14.5 \mathrm{x}$

$\Rightarrow \mathrm{x}=\dfrac{58}{\sqrt{3}} \quad \ldots[\because \mathrm{x} \neq 0]$

Therefore, area of the equilateral triangle $=\dfrac{\sqrt{3} \mathrm{x}^{2}}{4}=\dfrac{\sqrt{3}}{4} \times\left(\dfrac{58}{\sqrt{3}}\right)^{2}=\dfrac{\sqrt{3}}{4} \times$ $\dfrac{58 \times 58}{3} \sim 486 \mathrm{~cm}^{2}$

9. A parallelogram, the length of whose side is $60\;{\text{m}}$ and $25\;{\text{m}}$ has ane diagonal $65\;{\text{m}}$ long. Find the area of the parallelogram.

Ans: Let, AB = DC = 60 cm

BC = AD = 25m

and $AC = 65m$

Area of parallelogram ${\text{ABCD}} = $ Area of $\Delta {\text{ABC}} + $ area of $\Delta {\text{ACD}}$

$ = 2$ Area of $\Delta {\text{ABC}}[\therefore ar\Delta {\text{ABC}} = ar - \Delta {\text{ABD}}]$

(Image will be uploaded soon)

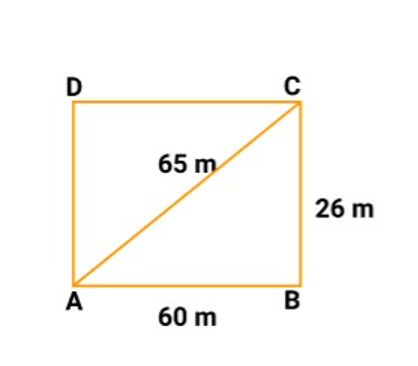
Now

$S = \dfrac{{60 + 65 + 25}}{2}m = 75m$

area of $\Delta {\text{ABC}} = \sqrt {S(s - a)(s - b)(s - c)} $

$ = \sqrt {75(75 - 60)(75 - 65)(75 - 25)} \,\,{\text{sq}}\,{\text{m}}$ 

$ = (5 \times 3 \times 5 \times 2 \times 5)\,{\text{sq}}\,{\text{m}}$ 

$ = 750\,{\text{sq}}\,{\text{m}}$....(II)

From (i) and (ii), we get

Area of

${\text {Parallelogram}}{\text{ABCD}} = 2 \times 750 = 15000\,{\text{sq}}\,{\text{m}}$.

10. A parallelogram, the measures of whose adjacent sides are $28\;{\text{cm}}$ and $42\;{\text{cm}}$, has one diagonals $38\;{\text{cm}}$. Find Its altitude on the side $42\;{\text{cm}}$.

(Image will be uploaded soon)

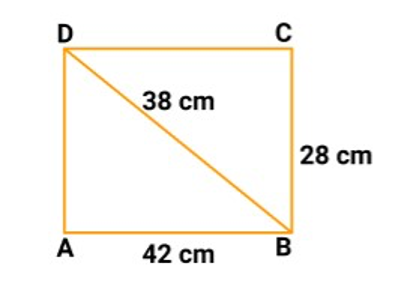
Ans: ${\text{AB}} = {\text{DC}} = 42\;{\text{cm}} = {\text{C}}$

${\text{BC}} = {\text{AD}} = 28\;{\text{cm}} = {\text{b}}$

And ${\text{BD}} = 38\;{\text{cm}} = {\text{a}}$

Let ${\text{A}}$ be the area of $\Delta {\text{ABD}}$

Now, ${\text{S}} = \dfrac{{38 + 28 + 42}}{2} = 54\;{\text{cm}}$

${\text{A}} = \sqrt {54(54 - 38)(58 - 28)(54 - 42)} $

$ = \sqrt {54 \times 16 \times 26 \times 12} {\text{sqcm}}.$

$ = 144\sqrt {13} {\text{sqcm}}$

Area of $\vartriangle {\text{ABD}}$$ = 144\sqrt {13} {\text{sqcm}}$

Again area of $\Delta {\text{ABD}} = \dfrac{1}{2}$ base $ \times $ altitude

$ = \dfrac{1}{2} \times 42 \times \,\,{\text{h}}\,{\text{sq}}\,{\text{cm}}$, where ${\text{h}}\,{\text{cm}}$ is altitude

$ = 21\;\,{\text{h}}\,{\text{sq}}\,{\text{cm}}$

From (i) and (ii), we get,

$21\;{\text{h}} = 144\sqrt {13} $

${\text{h}} = \dfrac{{144\sqrt {13} }}{{21}} = \dfrac{{48\sqrt {13} }}{7}\;{\text{cm}}$

Thus, required altitude $ = \dfrac{{48\sqrt {13} }}{7}\;{\text{cm}}$.

12. Find the area of a quadrilateral ABCD in which ${\text{AB}} = 3\;{\text{cm}},{\text{BC}} = 4\;{\text{cm}},{\text{CD}} = 4\;{\text{cm}}$,

${\text{DA}} = 5\;{\text{cm}}$ and ${\text{AC}} = 5\;{\text{cm}}$

(Image will be uploaded soon)

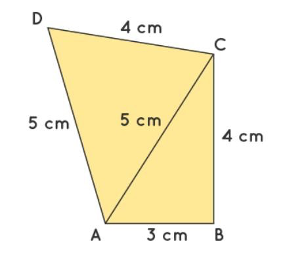
Ans: For $\triangle \mathrm{ABC}$, consider

$A B^{2}+B C^{2}=3^{2}+4^{2}=25=5^{2}$

$\Rightarrow 5^{2}=A C^{2}$

Since $\triangle \mathrm{ABC}$ obeys the Pythagoras theorem, we can say $\triangle \mathrm{ABC}$ is rightangled at $\mathrm{B}$.

Therefore, the area of $\triangle \mathrm{ABC}=1 / 2 \times$ base $\times$ height

$=1 / 2 \times 3 \mathrm{~cm} \times 4 \mathrm{~cm}=6 \mathrm{~cm}^{2}$

Area of $\triangle \mathrm{ABC}=6 \mathrm{~cm}^{2}$

Now, In $\triangle \mathrm{ADC}$

we have $a=5 \mathrm{~cm}, b=4 \mathrm{~cm}$ and $c=5 \mathrm{~cm}$

Semi Perimeter: $s=$ Perimeter $/ 2$

$s=(a+b+c) / 2$

$s=(5+4+5) / 2$

$s=14 / 2$

$s=7 \mathrm{~cm}$

By using Heron's formula,

Area of $\triangle\mathrm{ADC}=\sqrt{s(s-a)(s-b)(s-c)}$

$=\sqrt{7(7-5)(7-4)(7-5)}$

$=\sqrt{7 \times 2 \times 3 \times 2}$

${=}{2 \sqrt{2} 1 \mathrm{~cm}^{2}}$

Area of $\triangle \mathrm{ADC}=9.2 \mathrm{~cm}^{2}$ (approx.)

Area of the quadrilateral $A B C D=$ Area of $\triangle A D C+$ Area of $\triangle A B C$

$=9.2 \mathrm{~cm}^{2}+6 \mathrm{~cm}^{2}$

Thus, the area of the quadrilateral $A B C D$ is $15.2 \mathrm{~cm}^{2}$.

14. The perimeter of a triangle is $450\;{\text{m}}$ and its sides are in the ratio of \[13:12:5.\] Find the area of the triangle.

Ans: Let the sides of the triangle be $13{\text{x}},12{\text{x}}$ and $5{\text{x}}$

Perimeter of a triangle $ = 450\;{\text{m}}$

$\therefore 13x + 12x + 5x = 450\;{\text{m}}$

or $30{\text{x}} = 450$

$\therefore {\text{x}} = 15$

$\therefore $ The sides are $13 \times 15,12 \times 15$, and $5 \times 15$

I.e. $195\;{\text{m}},180\;{\text{m}}$ and $75\;{\text{m}}$

$\therefore {\text{S}} = \dfrac{{a + b + c}}{2} = \dfrac{{450}}{2} = 225\;{\text{m}}$

$\therefore $ Area of the triangle $ = \sqrt {s(s - a)(s - b)(s - c)\,\,} {\text{ sq}}\,{\text{m}}$

$ = \sqrt {225(225 - 195)(225 - 180)(225 - 75)} \,{\text{sq}}\,{\text{m}}$

$ = \sqrt {225 \times 30 \times 45 \times 150} \,{\text{sq}}\,{\text{m}}$

$ = (15 \times 15 \times 2 \times 3 \times 5)\,{\text{sq}}\,{\text{m}}$

$ = 6750\,{\text{sq}}\,{\text{m}}$.

15. The sides of a triangle are $39\;{\text{cm}},42\;{\text{cm}}$ and $45\;{\text{cm}}$. A parallelogram stands on the greatest side of the triangle and has the same area as that of the triangle. Find the height of the parallelogram.

(Image will be uploaded soon)

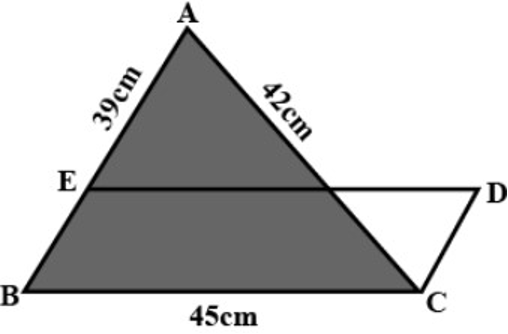
Ans: To find the area of $\Delta {\text{ABC}}$

$S = \dfrac{{45 + 42 + 39}}{2}\;{\text{cm}}$

$ = 63\;{\text{cm}}$

Therefore, Area of $\Delta {\text{ABC}} = \sqrt {63(63 - 45)(63 - 42)(63 - 39)} \,{\text{sq}}\,{\text{cm}}$

$ = \sqrt {63 \times 18 \times 21 \times 24} \,{\text{sq}}\,{\text{cm}}$

$ = 9 \times 7 \times 2 \times 3 \times 2\,{\text{sq}}\,{\text{cm}}$

$ = 756\,{\text{sq}}\,{\text{cm}}$

Let ${\text{h}}$ be the height of the parallelogram

Now,

Area of parallelogram ${\text{BCDE}} = $ Area of $\Delta {\text{ABC}}$

$\therefore {\text{h}} \times {\text{BC}} = 756$

or $45{\text{h}} = 756$

\[{\text{h}} = \dfrac{{756}}{{45}}\]

${\text{h}} = 16.8\;{\text{cm}}$

Hence, height of the parallelogram $ = 16.8\;{\text{cm}}$

16. The students of a school staged a rally for cleanliness campaign. They walked through the lanes in two groups. One group walked through the lanes ${\text{AB}},{\text{BC}}$ and CA whiles the other group through the lanes AC, CD and DA [fig1.1]. Then they cleaned the area enclosed within their lanes. If ${\text{AB}} = 9\;{\text{m}},{\text{BC}} = 40\;{\text{m}},{\text{CD}} = 15\;{\text{m}},{\text{DA}} = 28\;{\text{m}}$ and $\angle B = {90^\circ }$, which group cleaned more area and by how much? Find also the total area cleaned by the students.

(Image will be uploaded soon)

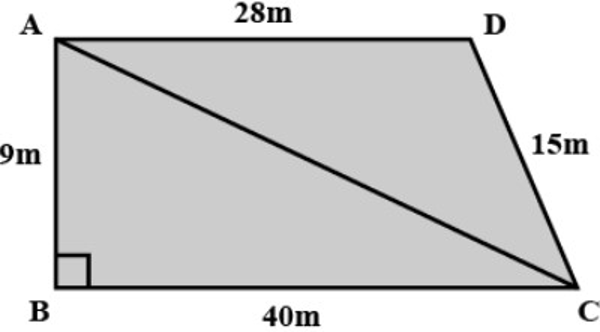
Ans: We have, right angle $\Delta {\text{AB}}$

${\text{A}}{{\text{C}}^{\text{2}}}{\text{ = A}}{{\text{B}}^{\text{2}}}{\text{ + B}}{{\text{C}}^{\text{2}}}$

${\text{A}}{{\text{C}}^{\text{2}}}{\text{ = }}{{\text{9}}^{\text{2}}}{\text{ + 4}}{{\text{0}}^{\text{2}}}$

${\text{A}}{{\text{C}}^{\text{2}}}{\text{ = 1681}}$

$\therefore {\text{AC}} = 41$

The first group has to clean the area of \[\Delta {\text{ABC}}\]which is right angled triangle

Now,

Area of $\Delta {\text{ABC}} = \dfrac{1}{2} \times 40\;{\text{m}} \times 9\;{\text{m}}$

$ = 180\,{\text{sq}}\,{\text{m}}$

The second group has to clean the area of $\Delta {\text{ACD}}$ which has ${\text{AD}} = 28\;{\text{m}}$,

${\text{DC}} = 15\;{\text{m}}$ and ${\text{AC}} = 41$

Hence,

${\text{S}} = \dfrac{{28 + 15 + 41}}{2}$

$ = 42\;{\text{m}}$

$\therefore $ Area of $\Delta {\text{ACD}} = \sqrt {42(42 - 28)(42 - 15)(42 - 41)} \,{\text{sq}}\,{\text{m}}$

$ = \sqrt {42 \times 14 \times 27 \times 1} \,{\text{sq}}\,{\text{m}}$

$ = \sqrt {7 \times 3 \times 2 \times 7 \times 2 \times 9 \times 3} \,{\text{sq}}\,{\text{m}}$

$ = 126\,{\text{sq}}\,{\text{m}}$

$\therefore $ First group cleaned more $ = (180 - 126)\,{\text{sq}}\,{\text{m}}$ 

$ = 54\,{\text{sq}}\,{\text{m}}$.

Therefore, Total area cleaned by students $ = (180 + 126)\,{\text{sq}}\,{\text{m}}$ 

$ = 306\,{\text{sq}}\,{\text{m}}$.

17. A traffic signal board indicating 'school ahead' is an equilateral triangle with side 'a' find the area of the signal board using heron's. Its perimeter is $180\;{\text{cm}}$, what will be its area?

Ans: Find the area of the single board,

$S = \dfrac{{a + a + a}}{2}\,{\text{units}}$ $ = \dfrac{{3a}}{2}{\text{units}}$ 

$\therefore $ Area of triangle $ = \sqrt {\dfrac{{3a}}{2} \times \left( {\dfrac{{3a}}{2} - a} \right)\left( {\dfrac{{3a}}{2} - a} \right)\left( {\dfrac{{3a}}{2} - a} \right)} $

$ = \sqrt {\dfrac{{3a}}{2} \times \dfrac{a}{2} \times \dfrac{a}{2} \times \dfrac{a}{2}} $

$ = \dfrac{{{a^2}}}{4}\sqrt 3 \,{\text{sq}}\,{\text{units}}$

Perimeter $ = 180\;{\text{cm}}$

Thus, each side $ = \dfrac{{180}}{3} = 60\;{\text{cm}}$

Area of signal board $ = \dfrac{{\sqrt 3 }}{4}{(60)^2}{\text{sq}}\,{\text{cm}}$ 

$ = 900\sqrt 3 \,{\text{sq}}\,{\text{cm}}$

18. A parallelogram the length of whose sides are $80\;{\text{m}}$, and $40\;{\text{m}}$ has one diagonal $75\;{\text{m}}$ long. Find the area of the parallelogram?

Ans: As according to the question, ${\text{AB}}\,{\text{ = }}\,{\text{DC}}\,{\text{ = }}\,{\text{80\;cm}}$

${\text{BC}}\,{\text{ =  AD}}\,{\text{ = }}\,{\text{40\;cm}}$ and ${\text{AC = }}75\;{\text{cm}}$

In $\vartriangle {\text{ABC}},{\text{S}} = \dfrac{{80 + 40 + 75}}{2} = 97.5\;{\text{cm}}$

Area of triangle ${\text{ABC}} = \sqrt {s(s - a)(s - b)(s - c)} $

$ = \sqrt {97.5(97.5 - 80)(97.5 - 40)(97.5 - 75)} \,\,{\text{sq}}\,{\text{m}}$

$ = \sqrt {97.5 \times 17.5 \times 57.5 \times 22.5} \,{\text{sq}}\,{\text{m}}$

$ = \sqrt {2207460.94} $

$ = 1485.75\,{\text{sq}}\,{\text{m}}$

Area of parallelogram ${\text{ABCD}} = 2 \times $ Area of $\vartriangle A{\text{BC}}$

$ = 2 \times 1485.7$

$ = 2971.4\,{\text{sq}}\,{\text{m}}$

19. The side of a triangular field is $52\;{\text{m}},56\;{\text{m}}$, and $60\;{\text{m}}$ find the cost of leveling the field \[{\text{Rs}}\,{\text{18/m}}\]if a space of $4\;{\text{cm}}$ is to be left for entry gate.

Ans:The side of a triangular field is $52 \mathrm{~m}, 56 \mathrm{~m}$, and $60 \mathrm{~m}$

The cost of leveling is Rs. $18 / \mathrm{m}$.

To find:

The total cost of leveling.

Solution:

1) Leveling is done at the boundary of the field so we will find the perimeter of the field first

The perimeter of the field:

$52+56+60$

$168 \mathrm{~m}$.

The length which needs to be leveled is $168-4=164 \mathrm{~m}$ (space of $4 \mathrm{~m}$ is left for the entry gate)

2) Cost of leveling is $164 \times 18$ =Rs. 2952

The total cost of leveling is Rs. 2952

20. A floral design of a floor is made up of 16 tiles which are triangular. The side of the triangle being $9\;{\text{cm}},28\;{\text{cm}}$, and $35\;{\text{cm}}$. find the cost of polishing the tiles, at ${\text{Rs }}\,50\,{\text{paisa}}\,{\text{/ sq}}\,{\text{cm}}$ 

Ans: For each triangular tile, we have

${\text{S}} = \dfrac{{35 + 28 + 9}}{2}\;{\text{cm}} = 36\;{\text{cm}}$

$\therefore $ Area of Each tile $ = \sqrt {s(s - a)(s - b)(s - c)} $

  $ = \sqrt {36(36 - 35)(36 - 28)(36 - 9)} \,{\text{sq}}\,{\text{cm}}$

$ = 36\sqrt 6 \,{\text{sq}}\,{\text{cm}}$

Area of 16 tile $ = 16 \times 36\sqrt 6 \,{\text{sq}}\,{\text{cm}}$ 

Therefore, cost of polishing $ = \operatorname{Rs} \left[ {\dfrac{1}{2} \times 16 \times 36\sqrt 6 } \right] = \operatorname{Rs} 288\sqrt 6 $

$   = \operatorname{Rs} (288 \times 2.45)$

$   = \operatorname{Rs} 705.60$ 

21. The measure of one side of a right triangle is $42\;{\text{m}}$. If the difference in lengths of Its hypotenuse and other side is $14\;{\text{cm}}$, find the measure of two unknown side?

Ans: Let ${\text{AB}} = {\text{y}}$ and ${\text{AC}} = {\text{x}}$ and ${\text{BC}} = 42\;{\text{cm}}$

Therefore, By the given condition,

${\text{x}} - {\text{y}} = 14({\text{i}})$

By Pythagoras theorem,

${x^2} - {y^2} = 1764$

$(x + y)(x - y) = 1764$

$\therefore 14({\text{x}} + {\text{y}}) = 1764$ using (ii)

$\therefore x + y = \dfrac{{1764}}{{14}} = 126\,\,\,{\text{(iii)}}$

(Image will be uploaded soon)

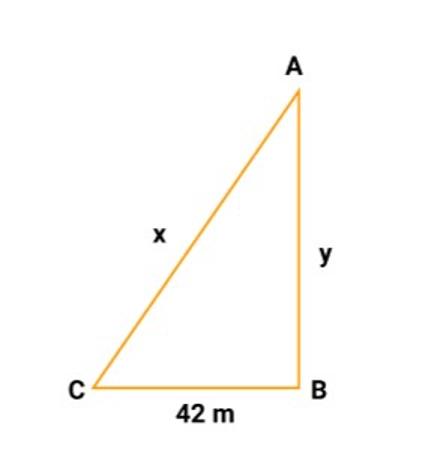
Adding (ii) and (iii), we get

$2{\text{x}} = 140$

i.e. ${\text{x}} = 70$

$\therefore {\text{y}} = 126 - {\text{x}}$

$y = 126 - 70$

$ = 56$

22. The perimeter of a rhombus ${\text{ABCD}}$ is $80\;{\text{cm}}$. find the area of rhombus if Its diagonal BD measures $12\;{\text{cm}}$.

(Image will be uploaded soon)

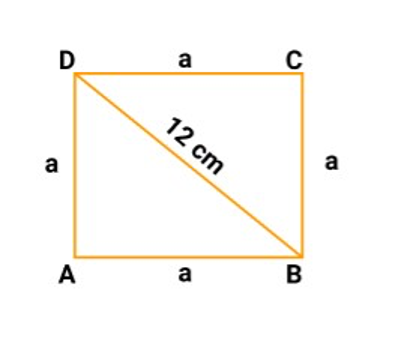
Ans: Given that,

Perimeter of rhombus $=80 \mathrm{~m}$

Perimeter of rhombus $=4 \times$ side

$\Rightarrow 4 \mathrm{a}=80$

$\Rightarrow \mathrm{a}=20 \mathrm{~m}$

Now in $\vartriangle {\text{ABD}}$,

$\therefore {\text{S}} = \dfrac{{20 + 20 + 12}}{2} = 26$

so,

Area of $\Delta {\text{ABD}} = \sqrt {26 \times 6 \times 6 \times 14} \,{\text{sq}}\,{\text{cm}}$ 

$ = 114.4\,{\text{sq}}\,{\text{cm}}$

Area of rhombus $ = 2 \times $ area of $\vartriangle ABD$

$ = 2 \times 114.4\,{\text{sq}}\,{\text{cm}}$

$ = 228.8\,{\text{sq}}\,{\text{cm}}$

23. Find area of quadrilateral ${\text{ABCD}}$ in which ${\text{AB}} = 5\;{\text{cm}},{\text{BC}} = 6\;{\text{cm}},{\text{CD = }}6\;{\text{cm}},{\text{DA}} = 7\;{\text{cm}}$,

And ${\text{AC}} = 7\;{\text{cm}}$

(Image will be uploaded soon)

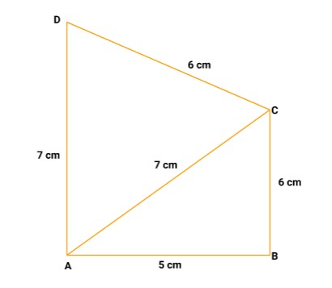
Ans: Area of quadrilateral ABCD = Area of $\Delta {\text{ABC}} + $ Area of $\Delta {\text{ACD}}$ (i)

In $\Delta {\text{ABC}}$,

${\text{S}} = \dfrac{{5 + 6 + 7}}{2} = 9\;{\text{cm}}$

Area of $\Delta {\text{ABC}} = \sqrt {9(9 - 5)(9 - 6)(9 - 7)} \,{\text{sq}}\,{\text{cm}}$

$ = \sqrt {9 \times 4 \times 3 \times 2} \,{\text{sq}}\,{\text{cm}}$

$ = 6\sqrt 6 \,{\text{sq}}\,{\text{cm}}$

$ = 14.4\,{\text{sq}}\,{\text{cm}}$ 

In $\Delta {\text{ACD}}$,

${\text{S}} = \dfrac{{7 + 7 + 6}}{2} = 10\;{\text{cm}}$

$\therefore $ Area of $\Delta {\text{ACD}} = \sqrt {10(10 - 7)(10 - 7)(10 - 6)} \,{\text{sq}}\,{\text{cm}}$

$ = \sqrt {10 \times 3 \times 3 \times 4} \,{\text{sq}}\,{\text{cm}}$

$ = 18.9{\text{sqcm}}$

Area of quadrilateral ${\text{ABCD}} = (14.4 + 18.9)\,{\text{sq}}\,{\text{cm}}$

$ = 33.3\,{\text{sq}}\,{\text{cm}}$.

24. Shashi Kant has a vegetable garden in the shape of a rhombus. The length of each side of garden is $35\;{\text{m}}$ And Its diagonal is $42\;{\text{m}}$ long. After growing the vegetables in it. He wants to divide it in seven equal parts And look after each part once a week. Find the area of the garden which he has to look after daily.

Ans: Let ${\text{ABCD}}$ be garden

$\therefore {\text{DC}} = 35\;{\text{m}}$ 

${\text{DB}} = 42\;{\text{m}}$ 

Draw \[\;CE \bot DB\]

(Image will be uploaded soon)

The diagonals of a rhombus bisect each other at right angles.

$\therefore {\text{DE}} = \dfrac{1}{2}\quad {\text{DB}} = \dfrac{1}{2} \times 42\quad $ or $21\;{\text{m}}$

Now

$C{E^2} = C{D^2} - D{E^2}$

$ = {35^2} - {21^2}$

$ = 784$

${\text{CE}} = 28\;{\text{m}}$

Area of $\Delta {\text{DBC}}, = \dfrac{1}{2} \times DB \times CE$

$ = \dfrac{1}{2} \times 42 \times 28$

$ = 588\,{\text{sq}}\,{\text{cm}}$

$\therefore $ Area of the garden ${\text{ABCD}} = 2 \times 588\,{\text{sq}}\,{\text{m}}$

$ = 1176\,{\text{sq}}\,{\text{m}}$

Area of the garden he has to look after, daily $ = \dfrac{{1176}}{7}\,{\text{sq}}\,{\text{m}}$ 

$ = 168\,{\text{sq}}\,{\text{m}}$

25. The perimeter of a triangle is \[480\] meters and its sides are in the ratio of \[1:2:3.\]Find the area of triangle?

Ans: Let the sides of the triangle be \[x,2x,3x\]

Perimeter of the triangle $ = 480\;{\text{m}}$

$\therefore x + 2x + 3x = 480\;{\text{m}}$

$6{\text{x}} = 480\;{\text{m}}$

${\text{x}} = 80\;{\text{m}}$

Therefore, The sides are $80\;{\text{m}},160\;{\text{m}},240\;{\text{m}}$

So,

$S = \dfrac{{80 + 160 + 240}}{2} = \dfrac{{480}}{2}$

$ = 240\;{\text{m}}$

And,

$\therefore \quad $ Area of triangle $ = \sqrt {s(s - a)(s - b)(s - c)} \,{\text{sq}}\,{\text{m}}$

$ = \sqrt {240(240 - 80)(240 - 160)(240 - 240)} \,{\text{sq}}\,{\text{m}}$

$ = 0\,{\text{sq}}\,{\text{m}}$

Therefore, Triangle doesn't exist with the ratio \[1:2:3\] whose perimeter is $480\;\,{\text{m}}$.

26. Find the cost of leveling the ground in the form of equilateral triangle whose side is $12\;{\text{m}}$ at \[{\text{Rs 5}}\]per square meter.

Ans: Ans: Here, sides are $12\;{\text{m}},12\;{\text{m}},12\;{\text{m}}$,

$\therefore {\text{S}} = \dfrac{{12 + 12 + 12}}{2}$

$ = 18\;{\text{cm}}$

And,

Area of equilateral triangle $ = \sqrt {s(s - a)(s - b)(s - c)} \,{\text{sq}}\,{\text{m}}$ 

$ = \sqrt {18(18 - 12)(18 - 12)(18 - 12)} \,{\text{sq}}\,{\text{m}}$

$= \sqrt {18 {\times} 6 {\times} 6 {\times} 6} \,{\text{sq}}\,{\text{m}}$

 $ = \sqrt {6 \times 3 \times 6 \times 6 \times 6} \,{\text{sq}}\,{\text{m}}$

$ = 36\sqrt 3 \,{\text{sq}}\,{\text{m}}$

$\therefore $ Cost of leveling ground $ = 5 \times 36 \times 1.73$

${\text{ = Rs}}\,{\text{311}}{\text{.4\;m}}$

27. A kite in the shape of a square with diagonal $32\;{\text{cm}}$ and an isosceles triangle of base $8\;{\text{cm}}$ and side $6\;{\text{cm}}$ each is to be made of three different shades. How much paper of each shade has been used in it? ( use $\sqrt 5  = 2.24$ )

(Image will be uploaded soon)

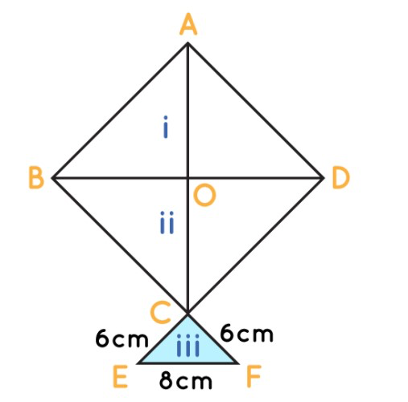
Ans: Let ${\text{ABCD}}$ be the square and $\Delta {\text{CEF}}$ be an isosceles triangle.

Let the diagonals bisect each other at \[o.\]

Then, ${\text{AO}} = \dfrac{1}{2} \times 32\;{\text{cm}}$

 $ = 16\;{\text{cm}}$

Area of shaded portion ${\text{I}} = \dfrac{1}{2} \times 16 \times 32\,{\text{sq}}\,{\text{cm}}$

$ = 256\,{\text{sq}}\,{\text{cm}}$

And,

Area of portion III $ = \dfrac{{\text{a}}}{{\text{4}}}\sqrt {{\text{4}}{{\text{b}}^{\text{2}}}{\text{ - }}\,{{\text{a}}^{\text{2}}}} $ $ = \dfrac{8}{4}\sqrt {4 \times {{(6)}^2} - 8} $

$ = 17.92{\text{sqcm}}$

Thus, the papers of three shades required are $256{\text{ sq}}\,{\text{cm}},256\,\,{\text{sq}}\,{\text{cm}}$ and $17.92\,{\text{sq}}\,{\text{cm}}$.

28. The sides of a quadrangular field, taken in order are $29\;{\text{m}},36\;{\text{m}},7\;{\text{m}}$ and $24\;{\text{m}}$

respectively. The angle contained by the last two sides is a right angle. Find its area.

(Image will be uploaded soon)

Ans: As the sides are provided in order. Therefore, the length of the last two sides are $7 \mathrm{~m}$ and $24 \mathrm{~m}$ respectively.

As we know that:

In a right angle triangle using Pythagoras theorem, 

$(\text { Hypotenuse })^{2}=(\text { Base })^{2}+(\text { Height })^{2}$

Let us assume:

- The diagonal of the field be $\mathbf{d}$.

Substituting the values,

$\mathrm{d})^{2}=(24)^{2}+(7)^{2}$

$\mathrm{d})^{2}=576+49$

$\mathrm{d})^{2}=625$

$\mathrm{d}=25$

Hence,

Diagonal of the park $=25 \mathrm{~m}$

Semi-perimeter $=$ Perimeter $/ 2$

$=(29+36+25) \mathrm{m} / 2$

$=90 \mathrm{~m} / 2$

$=45 \mathrm{~m}$

Substituting the values,

$\text { Area }=\sqrt{45(45-29)(45-36)(45-25)}$

$=\sqrt{45 \times 16 \times 9 \times 20}$

$=\sqrt{129600}$

$=360$

Finding area of $2 \mathrm{nd}$ triangle:

1st side $=7 \mathrm{~m}$

2nd side $=24 \mathrm{~m}$

3rd side $=25 \mathrm{~m}$

Finding semi-perimeter of the triangle:

Semi-perimeter$=\text { Perimeter/2 }$

$=(7+24+25) \mathrm{m} / 2$

$=56 \mathrm{~m} / 2$

$=28 \mathrm{~m}$

Finding area of the 2nd triangle using Heron's formula:

$\text { Area }=\sqrt{28(28-7)(28-24)(28-25)}$

$=\sqrt{28 \times 21 \times 4 \times 3}$

$=\sqrt{7056}=84$

Hence, area of the 2 nd triangle is $84 \mathrm{~m}^{2}$.

Finding area of the quadrangular field:

Ar. of the field $=($ Ar. of 1 st $\Delta)+($ Ar. of 2 nd $\Delta$ )

$=(360+84) \mathrm{m}^{2}$

$=444 \mathrm{~m}^{2}$

Hence, area of the field $=\mathbf{4 4 4 \mathrm { m } ^ { 2 }}$

4 Marks Questions:

1. A field in the shape of a trapezium whose parallel side are $25\;{\text{m}}$ and $10\;{\text{m}}$. The non- parallel side are $14\;{\text{m}}$ and $13\;{\text{m}}$. Find the area of the field.

(Image will be uploaded soon)

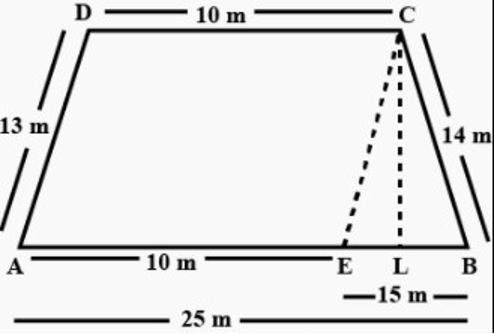
Ans: Let $\mathrm{ABCD}$ be a trapezium with,

$\mathrm{AB} \| \mathrm{CD}$

$\mathrm{AB}=25 \mathrm{~m}$

$\mathrm{CD}=10 \mathrm{~m}$

$\mathrm{BC}=14 \mathrm{~m}$

$\mathrm{AD}=13 \mathrm{~m}$

Draw CE | DA. So, ADCE is a parallelogram with,

$\mathrm{CD}=\mathrm{AE}=10 \mathrm{~m}$

$\mathrm{CE}=\mathrm{AD}=13 \mathrm{~m}$

$\mathrm{BE}=\mathrm{AB}-\mathrm{AE}=25-10=15 \mathrm{~m}$

In $\Delta \mathrm{BCE}$, the semi perimeter will be,

$\mathrm{s}=\dfrac{\mathrm{a}+\mathrm{b}+\mathrm{c}}{2}$

$\mathrm{~s}=\dfrac{14+13+15}{2}$

$\mathrm{~s}=21 \mathrm{~m}$

Area of $\triangle \mathrm{BCE}$,

$A=\sqrt{s(s-a)(s-b)(s-c)}$

$=\sqrt{21(21-14)(21-13)(21-15)}$

$=\sqrt{21(7)(8)(6)}$

$=\sqrt{7056}$

$=84 \mathrm{~m}^{2}$

Also, area of $\Delta \mathrm{BCE}$ is,

$\mathrm{A}=\dfrac{1}{2} \times \text { base } \times \text { height }$

$84=\dfrac{1}{2} \times 15 \times \mathrm{CL}$

$\dfrac{84 \times 2}{15}=\mathrm{CL}$

$\mathrm{CL}=\dfrac{56}{5} \mathrm{~m}$

Now, the area of trapezium is,

$\mathrm{A}=\dfrac{1}{2} \text { (sum of parallel sides) (height) }$

$\mathrm{A}=\dfrac{1}{2} \times(25+10)\left(\dfrac{56}{5}\right)$

$\mathrm{A}=196 \mathrm{~m}^{2}$

Therefore, the area of the trapezium is $196 \mathrm{~m}^{2}$.

2. The perimeter of a right triangle is $24\;{\text{cm}}$. If its hypotenuse is $10\;{\text{cm}}$, find the other two sides. Find its area by using the formula area of a right triangle. Verify your result by using Heron's formula.

Ans: Let the sides of right $\Delta$ be ' $a$ ' $\mathrm{cm}$ and ' $b$ ' $\mathrm{cm}$.

Then,

(Image will be uploaded soon)

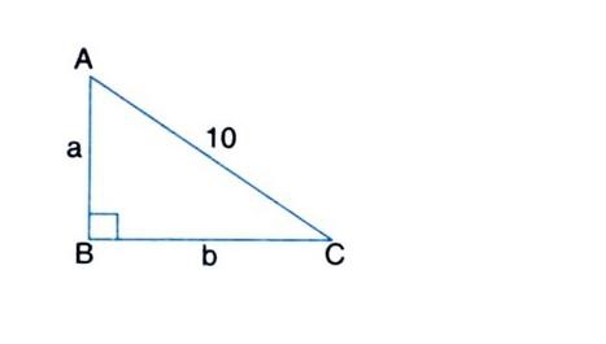
$a+b+c=24$

$\Rightarrow a+b+10=24$

$\Rightarrow a+b=24-10$

$\Rightarrow a+b=14 \ldots \ldots \ldots . . .(1)$

$a^{2}+b^{2}=(10)^{2}$

Also, $a^{2}+b^{2}=100$

$\Rightarrow a^{2}+b^{2}=100 \ldots \ldots(2)$

We know that

$(a+b)^{2}=a^{2}+2 a b+b^{2}$

$(14)^{2}=100+2 a b$

$-2 a b=100-196$

$-2 a b=-96$

$a b=\dfrac{96}{2}=48$

$=>a b=48 \ldots \ldots$

Also,

$(a-b)^{2}=a^{2}-2 a b+b^{2}$

$(a-b)^{2}=100-2 \times 48$

$(a-b)^{2}=100-96$

$(a-b)^{2}=4$

$(a-b)=\sqrt{4}=2$

$\Rightarrow(a-b)=2$

Solving (1) and (4) we get;

$\therefore a=8$ and $b=6$

Now,

$\dfrac{a+b+c}{2}$

$\mathrm{~S}=\dfrac{24}{2}=12$

Area of $\Delta=\sqrt{s(s-a)(s-b)(s-c)}$

$\Rightarrow \sqrt{12(12-8)(12-6)(12-10)}$

$=>\sqrt{12 \times 4 \times 6 \times 2}$

$=>\sqrt{2 \times 2 \times 3 \times 2 \times 2 \times 2 \times 3 \times 2}$

$=>\sqrt{2^{2} \times 2^{2} \times 2^{2} \times 3^{2}}$

$=>2 \times 2 \times 2 \times 3$

$=>24$

Hence,

The area of $\Delta$ is $24 \mathrm{~cm}$.

3. Radha made a picture of an aero plane with colored paper as shown in fig. find the total area of the paper used.

(Image will be uploaded soon)

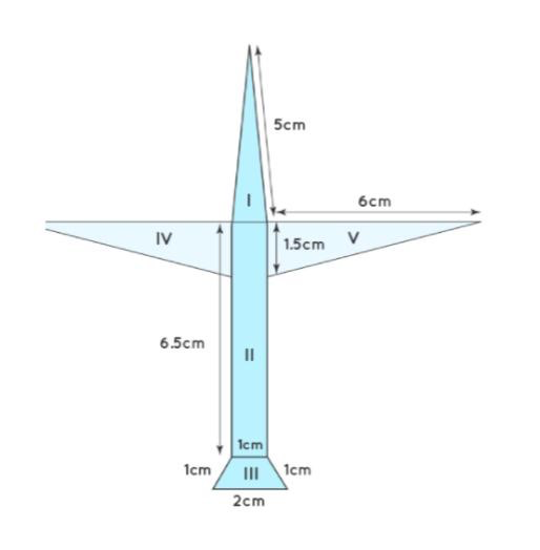
Ans: Area (1) \[ = \]area of is iosceles triangle with ${\text{a}} = 1\;{\text{cm}}$ and ${\text{b}} = 5\;{\text{cm}}$

$ = \dfrac{a}{4}\sqrt {4{b^2} - {a^2}} $

$ = \dfrac{1}{4}\sqrt {100 - 1}  = \dfrac{{\sqrt {99} }}{4}\,\,{\text{sq cm}}($ approx)

Area (ii) = area of rectangle with

${\text{L}} = 6.5\;{\text{cm}}$ and ${\text{b}} = 1\;{\text{cm}}$

$ = 6.5 \times 1\,{\text{sq}}\,{\text{cm}}$

$   = 6.5\,{\text{sq}}\,{\text{cm}}$ 

Area (iii) = Area of trapezium

$ = 3 \times $ Area of equilateral $\Delta $ with side $ = 1\;{\text{cm}}$

$ = 3 \times \dfrac{{\sqrt 3 }}{4} \times {(1)^2}\,{\text{sq}}\,{\text{cm}}$

$ = \dfrac{{3 \times 1.732}}{4}$ or $\dfrac{{5.196}}{4}{\text{sqcm}}$

$ = 1.3\,{\text{sqcm}}$ (approx.)

Area of $({\text{IV}} + {\text{V}}) = 2 \times \dfrac{1}{2} \times 6 \times 1.5\,{\text{sq}}\,{\text{cm}} = 9\,{\text{sq}}\,{\text{cm}}$

Total area of the paper used = Area $({\text{I}} + {\text{II}} + {\text{III}} + {\text{IV}} + {\text{V}})$

$ = (2.5 + 6.5 + 1.3 + 9)\,{\text{sq}}\,{\text{cm}}$

$ = 19.3\,{\text{sq}}\,{\text{cm}}$

Class 9 Heron’s Formula Important Questions - Free PDF Download

Students often find mathematics as a confusing and challenging subject. It becomes difficult for them to understand the formulas, and they also get confused about using the formulas at the right place. Chapter 10 of mathematics involves multiple formulas and different concepts which makes it a challenging chapter to understand. Without the help of proper notes and study material, it would be challenging for the students to understand the chapter. The students can get a complete hold on the chapter by practising the important questions. So, we at Vedantu have designed the important questions for class 9 maths heron’s formula for the betterment of the students. These important questions come with the answers, which makes it easier for the students to understand. For your convenience, we have prepared the PDF of the important questions and answers. The ch 12 maths class 9 important questions are available on our website and the students and download it for free.

The important questions for class 9 maths chapter 10 are designed as per the latest CBSE guidelines. The students who face difficulty in understanding chapter 10 of maths can take help of these questions. It will clear the doubts of the students and make learning interesting. 

Important Questions of Heron’s Formula Class 9

Chapter 10 of mathematics, class 9 will explain to the students how to calculate the area of a triangle. The chapter will teach the students to calculate the area of the triangle using heron’s formula. The chapter will also provide an account of information about using heron’s formula in calculating the area of other polygons. By practising the heron’s formula class 9 important questions, you can get a detailed overview of the chapter. Here we have discussed a brief of the chapter.

Triangles

A triangle is a three-dimensional closed figure. It has three sides and three angles. A triangle can be divided into different types based on different characteristics, such as sides or angles.

Based on the sides, triangles can be three types, and they are, Equilateral triangle, Scalene triangle and Isosceles triangle. Similarly, based on the angles, a triangle can be again subdivided into three types. Namely, Acute angled triangle, Right-angled triangle, and Obtuse angled triangle.

Area of A Triangle

We can find the area of a triangle by using the following formula:

Area of a triangle = (½) X b X h

Where b represents the length of the base of the triangle.

And h represents the height of the triangle.

Area of An Equilateral Triangle

In the case of an equilateral triangle, where all the sides of a triangle are equal in length, we can find the height using Pythagoras theorem. Let us take an example; ΔPQR is an equilateral triangle. And we have PS as the perpendicular bisector to the side QR. To find the area, we first need to find the height PS. So, if we use Pythagoras theorem we get,

PQ2 = SP2 + SQ2 

Therefore, 

SP2 = PQ2 - SQ2 

Now, if we substitute (a) in place of PQ and (a/2) in place of SQ in the following equation we get,

SP2 = a - a2/4

And therefore,

The height, SP = √3a/2 

Now, As we know that the Area of a Triangle = ½ X b X h

Therefore, the Area of an equilateral triangle = ½ X a X √3a/2 

=  √3a2/4

Area of An Isosceles Triangle

Let’s consider an Isosceles Triangle BCD. We have BE as the perpendicular bisector to the base CD. Now, we can find the height of the isosceles triangle by using Pythagoras theorem, where we get,

BC2 = BE2 + CE2

Therefore, 

BE2 = BC2 - CE2 

Now, if we substitute (a) in place of BC and (b/2) in place of CE, we get

BE2 = a2 - (b2/4) = (4a2 - b2)/4

Now,

BE = (½) √(4a2 - b2)

Now Area of the triangle is ½ X b X h

Therefore

Area of Isosceles triangle = ¼  X b X  √(4a2 - b2)

Area of A Triangle Using Heron’s Formula

We can use Heron's formula if all the sides of a triangle are unequal.

According to heron’s formula, 

Area of a Triangle = √

s(s−a)(s−b)(s−c)

s(s−a)(s−b)(s−c)

Here, a, b, and c are the length of the three sides of the triangle.

And S is the semi-perimeter of the triangle, and we can find the semi-perimeter by:

S = (a+b+c)/2

Area of Any Polygon using Heron’s Formula

We can find the area of any polygon by using the heron’s formula. If we consider a quadrilateral, we can split the quadrilateral into two triangles using one of its diagonals. Now, we can find the area of each triangle by utilising the heron’s formula and then we can add both the areas. By the following method, we can calculate the area of any polygon using heron’s formula.

Class 9 Maths Chapter 10 Important Questions

To provide you with a proper understanding of what to study for mathematics, we have added some of the important questions for class 9 maths heron’s formula in this article. You can refer to these questions and boost your exam preparations.

• What is an Isosceles triangle?

• What is a Scalene triangle?

• An Isosceles right-angled triangle has an area of 8cm2. Find the length of its hypotenuse.

• The three sides of a triangle are 30cm, 50cm, 65cm respectively. Find the area of the triangle using heron’s formula.

• Suppose the area of an equilateral triangle is 16 √3m2. Find its perimeter. 

• Find the area of a triangular field whose sides are 40cm, 90cm and 70cm.

• Find the area of a triangle whose perimeter is 32cm and two of its side are 8cm and 11cm respectively.

• Two sides of a parallelogram are 10cm and 7cm respectively. One of its diagonal is 13 cm, find its area.

• The side of an equilateral triangle is 4√3 cm. Find its area.

• Find the area of a triangle using heron’s formula. Two sides of the triangle are 18cm and 10 cm respectively. The perimeter of the triangle is 42cm.