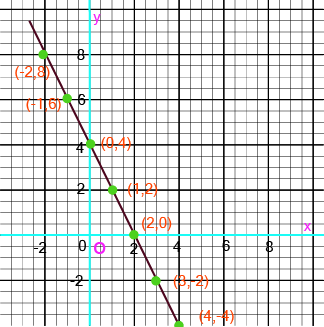
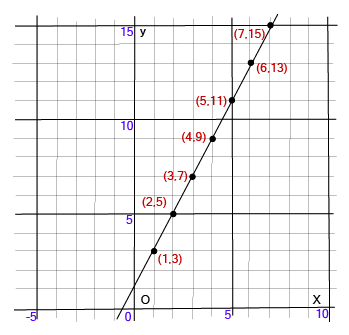
Important Questions for CBSE Class 9 Maths Chapter 3 Coordinate Geometry: FREE PDF Download
FAQs on CBSE Class 9 Maths Important Questions - Chapter 3 Coordinate Geometry
1. Which chapter is the most important in Class 9 Maths?
Chapter 3 of Class 9 Maths is about geometry which is the branch of Mathematics concerned with spatial connections among diverse things, individual object forms, and surrounding space characteristics. Class 9 is an important year for high school students since it is during this year that students lay the groundwork for all of the major topics and ideas covered in the Class 10 Board examinations. Students will learn how to identify points in a cartesian system or an XY plane in the chapter Coordinate Geometry. This notion is critical for determining the position of an object in a certain location.
2. How can I practise Chapter 3 Coordinate Geometry of Class 9 Maths?
Geometry is the component of Mathematics that demands a comprehension of the topic as well as certain visualising abilities that students should work on to improve. Vedantu's Crucial Questions for Class 9 Mathematics Coordinate Geometry are valuable and trustworthy sources of study material supplied for students in a well-structured and easily understandable format. These vital questions are available for free on Vedantu (vedantu.com). It will help pupils understand the chapters thoroughly. Students will get a grasp of the chapters needed to answer the difficult problems in class. The essential questions will help pupils cover all of the issues and achieve good grades.
3. What are case study questions in Class 9 Maths?
Case study questions in Class 9 Maths are the questions that introduce a particular scenario and its mathematical aspect and then proceeds to ask some questions that are relevant to the given chapter i.e. Coordinate Geometry in this case. Case study questions usually involve a real life-based situation where the questions check the students’ analytical ability to follow through and subsequently apply the Maths to it. They are extremely important as they carry a lot of marks and are really simple to get through.
4. How to plot a point?
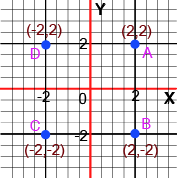
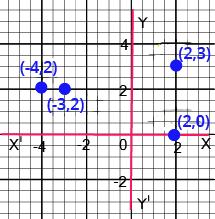
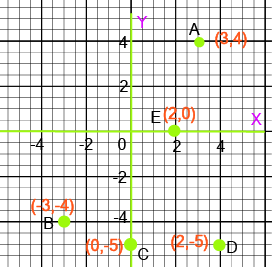
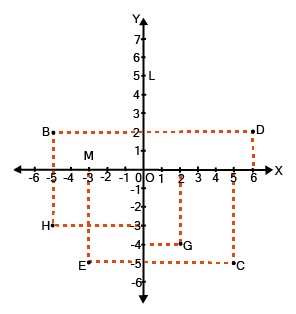
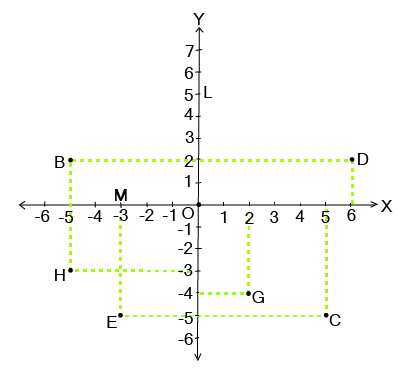
To plot or graph points, we can use two perpendicular lines known as the x- and y-axes. The horizontal x-axis is parallel to the vertical y-axis. The positive x-axis is located to the right of the origin, while the negative x-axis is located to the left of the origin. Likewise, the positive y-axis is the part of the y-axis above the origin, and the negative y-axis is the part of the y-axis below the origin. Every point in the coordinate plane is defined by a given pair of x and y coordinates.
5. What is coordinate geometry?
Coordinate geometry is concerned with identifying points on a plane when the aligned values, known as coordinates for a certain point, are supplied. It introduces geometric concepts in Algebra and helps students to solve geometric problems. The origin is defined as the point at where the x-axis and y-axis connect. At this moment, both x and y are zero. To identify a point on the plane, a collection of two numbers is used.
6. What is the significance of the Cartesian plane in Class 9 Maths Chapter 3 - Coordinate Geometry?
The Cartesian plane is a two-dimensional grid formed by the intersection of the x-axis (horizontal) and y-axis (vertical). In Class 9 Maths Chapter 3 - Coordinate Geometry, the Cartesian plane is used to plot points and solve geometric problems, helping students understand the relationship between algebra and geometry.
7. What is the Distance Formula in Class 9 Maths Chapter 3 - Coordinate Geometry?
The distance formula is used to calculate the distance between two points (x₁, y₁) and (x₂, y₂) on a coordinate plane. The formula in Class 9 Maths Chapter 3 - Coordinate Geometry is:
Distance = √[(x₂ - x₁)² + (y₂ - y₁)²]
This formula helps students find the length of a line segment between two points in the coordinate plane.
8. What is the Section Formula in Class 9 Maths Chapter 3 - Coordinate Geometry?
The section formula is used to find the coordinates of a point dividing a line segment in a given ratio in Class 9 Maths Chapter 3 - Coordinate Geometry. If a point divides the line segment joining two points (x₁, y₁) and (x₂, y₂) in the ratio m:n, the coordinates of the point are given by:
(x, y) = [(m * x₂ + n * x₁) / (m + n), (m * y₂ + n * y₁) / (m + n)]
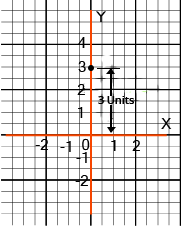
9. What is the formula for the Midpoint of a Line Segment in Class 9 Maths Chapter 3 - Coordinate Geometry?
In Class 9 Maths Chapter 3 - Coordinate Geometry, the midpoint of a line segment joining two points (x₁, y₁) and (x₂, y₂) is the point whose coordinates are the average of the coordinates of the two points. The formula is:
Midpoint = [(x₁ + x₂) / 2, (y₁ + y₂) / 2]
10. How do you find the coordinates of a point on the coordinate plane in Class 9 Maths Chapter 3 - Coordinate Geometry?
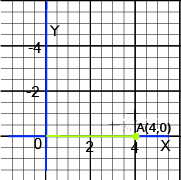
In Class 9 Maths Chapter 3 - Coordinate Geometry, to find the coordinates of a point, locate it on the Cartesian plane. The first value of the coordinate is the distance from the origin along the x-axis (horizontal), and the second value is the distance from the origin along the y-axis (vertical). The point is represented as (x, y).
11. What are the common types of questions in Class 9 Maths Chapter 3 - Coordinate Geometry?
Common questions in Class 9 Maths Chapter 3 - Coordinate Geometry include finding the distance between two points, calculating the midpoint, determining the coordinates of a point dividing a line segment in a given ratio, and solving problems based on plotting points and lines on the coordinate plane.
12. What are the key formulas for solving problems in Class 9 Maths Chapter 3 - Coordinate Geometry?
The key formulas for Class 9 Maths Chapter 3 - Coordinate Geometry include:
Distance formula to calculate the distance between two points.
Midpoint formula to find the midpoint of a line segment.
Section formula to find a point dividing a line segment in a ratio.
Understanding the representation of points (x, y) on the coordinate plane.
13. How can I improve my understanding of Class 9 Maths Chapter 3 - Coordinate Geometry?
In Class 9 Maths Chapter 3 - Coordinate Geometry, practice regularly by solving various problems from textbooks, sample papers, and important questions. Focus on understanding the basic concepts and use visual aids like graphs to better understand the relationships between points and lines.
14. What are some real-life applications of Class 9 Maths Chapter 3 - Coordinate Geometry?
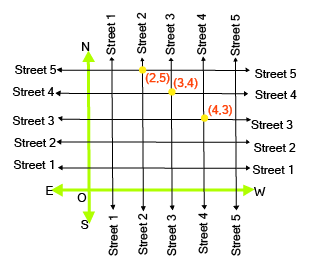
Class 9 Maths Chapter 3 - Coordinate Geometry is used in many real-life applications, such as navigation systems (GPS), architecture (designing buildings and structures), computer graphics, and even in sports for analysing player positions and movements.
15. How do case study questions help in understanding Class 9 Maths Chapter 3 - Coordinate Geometry?
Case study questions in Class 9 Maths Chapter 3 - Coordinate Geometry are based on real-life scenarios where students need to apply the concepts of coordinate geometry to solve practical problems. These questions help improve analytical and problem-solving skills and are useful for scoring well in exams, as they carry significant marks.

















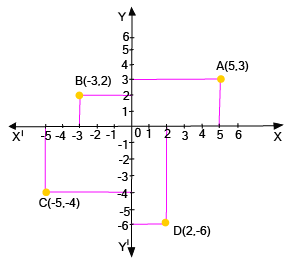
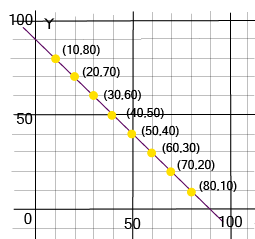
![\[{\bf{y}} = {\bf{x}}\]](https://www.vedantu.com/seo/content-images/1c42c8cc-ae85-4051-93ba-4a0e360840ce.png)