




A Brief Introduction to Progressive Harmonic Wave
A wave in a medium can be defined as a disturbance that travels in the medium without changing its shape. Example: Water ripples that occur when a stone falls into the water. Waves are a mode of energy transfer or disturbance that propagates through an elastic medium. This occurs because the particles in the medium oscillate around the mean position.
A simple vibration wave is a wave that propagates continuously in a predetermined direction without changing its shape, and the particles of the medium make a simple vibration around the mean position with the same amplitude and period as the wave passes through.
What is Progressive Wave?
Each particle in the medium produces a vibration centered on its mean position. The disturbance propagates from one particle to the next. The particles in the medium oscillate around the center position with the same amplitude. Each particle in the medium, or each subsequent particle, travels along the wave propagation path in the same direction as the previous particle, but at a later point in time. In most cases, the particles will not remain stationary indefinitely. The particles temporarily rest twice in extreme locations during each vibration. Different particles arrive at different positions at different times. When the particles move in the mean locus, they all have the same maximum velocity. The displacements of the particle velocity and acceleration are equal when separated by equation m. Where m is an integer.
What is Harmonic Wave?
Harmonics are waves with frequency which are positive integer multiple of the fundamental frequency, and that is also the frequencies of the original periodic signal (such as sinusoidal waves). The original signal is also called the first harmonic, and the other harmonics are called harmonics.
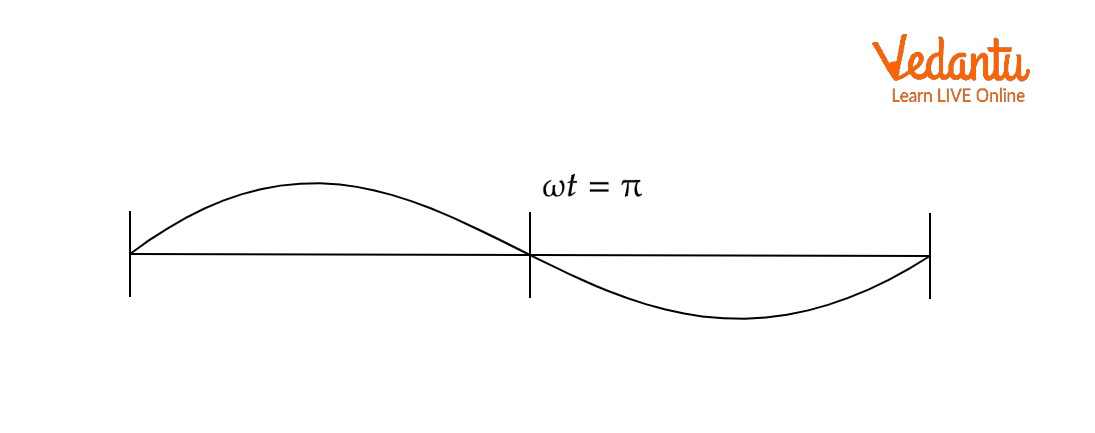
First Harmonic Motion
What is Two Plane Progressive simple Harmonic Wave?
A plane-progressive harmonic wave is a wave that propagates in a particular direction without changing its shape, and each particle in the medium performs a simple harmonic motion around its average position with equal amplitude and duration.
Consider a particle at point P at a distance x from origin O. The particles perform simple vibrations of equal amplitude and duration. The disturbance at O moves and reaches point P after a while.

Plane Progressive Wave
Therefore, the displacement of the particle from the equilibrium position at time t is given as follows.
where a = amplitude,
phase difference depends on the path difference OP
where x = path difference,
Derive Equation for Progressive Wave
To derive the equation of progressive wave, consider a simple harmonic wave that propagates along the positive direction of the x-axis.
The vibration of the particles in the medium is parallel or perpendicular to the x-axis.
At any time t, the displacement of the particles of the medium at the origin is given by the following equation.
where a is the amplitude and 2π /
Now consider the particles of the medium at the point P at a distance x from O.
This particle is exhibiting S.H.M., but with the same amplitude and period; the disturbance of O reaches P after a while.
In other words, the P particle lags behind the O phase particle.
Therefore, the displacement of the particle at P at time t is given by
Where
Angle
In waves, two particles separated by a distance are 2π radians out of phase.
In other words, the path difference à corresponds to a 2p radian phase difference.
Therefore, the path difference x corresponds to the phase difference of
If T is the period of vibration,
This is a one-dimensional traveling wave traveling along the x-axis positive for the period. It is an equation of the waves.
If n is the vibration frequency of the particle, T = 1/n, substituting into equation (1) gives:
This is a simple harmonic progressive wave representation of the frequency of the wave.
If v is the velocity of the wave in the medium,
This is a simple harmonic progressive wave equation for wave velocity. Equations (2) ) and (3) are two different forms of the equation for a one-dimensional simple harmonic wave propagating along the positive x-axis.
Application of Plane Progressive Wave
All particles in the medium are in S.H.M., whenever a wave passes through the medium.
All particles oscillate with the same amplitude.
All particles vibrate at the same frequency.
Simple-oscillation traveling wave propagation is a double-periodic phenomenon that is temporally and spatially periodic.
Energy is transmitted through the medium.
Wavelength of Progressive Wave
For progressive waves, the wavelength "
Intensity of Progressive Wave Strength
The sounds of violins and instrument flutes, and reed organs are pleasant to the ear, but the sounds of guns, horns, and cars are unpleasant. The loudness of a sound is determined by the strength of the sound wave and the sensitivity of the ear. Intensity is generally defined as the amount of energy that passes per unit area per unit time in the direction perpendicular to the wave propagation path. W m-2 is a unit of strength measurement.
Summary
Regardless of the medium, the amplitude of the traveling wave remains constant as it propagates in the same direction over time. You can use transverse or longitudinal waves. Imagine a plane of simple vibration traveling along the X axis from the O origin. If you calculate backward from the moment of creation, you can see that the particles at this origin only move in the positive direction at the center position.
FAQs on Progressive Harmonic Wave - JEE Important Topic
1. Discuss the law of transverse vibration of taut strings.
(i) Law of Length:
For a particular wire with tension T (fixed) and mass per unit length μ (fixed), the frequency changes inversely with the vibration length.
Therefore,
where C is a constant.
(ii) Law of Tension:
Given the length l (fixed) of vibration and the mass μ (fixed) per unit length, the frequency is the square root of voltage T,
(iii) Law of Mass:
For a given vibration length l (fixed) and voltage T (fixed), the frequency changes in inverse proportion to the square root μ of the mass per unit length.
Where B is a constant.
2. What are progressive waves? Define longitudinal waves.
A propagating wave is defined as the propagation of the vibrational motion of an object in an elastic medium from one particle to the next. Simply put, a wave that propagates continuously through a medium in the same direction, minus changes, is called a progressive or progressive wave. A progressive wave is a term for a wave that propagates from one point A in the medium to another point B. Longitudinal waves are waves in which the displacement of the particles is in the same direction as the wave. For longitudinal waves, the displacement of the particles stays in the same direction as the waves.










