Projectile on an Inclined Plane for JEE

Before we start with the projectile motion, let's understand what a projectile is. A projectile is an object that has been launched, typically using some kind of force or energy, and continues to move through the air under its own momentum. It can be anything from a small stone thrown by a child to a large missile fired from a rocket launcher. The word comes from the Latin term, projectus, which means "thrown forward." 

In this article, we will be discussing projectile motion in detail. We will cover the basics of projectile motion, its physics, and how it is used in everyday life. We will also explore some interesting applications of projectile motion that you may not have known about. So read on to learn more about this fascinating topic.

What is Projectile Motion?

In Physics, projectile motion is the motion of an object thrown or projected into the air, and is subject to only the force of gravity. The object is called a projectile, and its path is called its trajectory. Projectile motion only occurs when there is one dominant force acting upon an object in any given direction. In other words, projectile motion occurs when an object experiences a force that acts upon it in a single direction only.

Parabolic Motion of Projectiles

Let us consider a ball projected at an angle θ with respect to the horizontal x-axis with the initial velocity u as shown below:

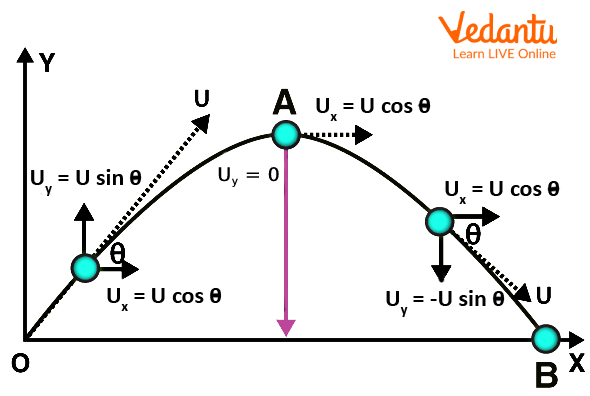
Parabolic Motion of Projectiles

The point O is called the point of projection; $\theta $ is the angle of projection and OB = Horizontal Range or Simply Range. The total time taken by the particle from reaching O to B is called the time of flight. For finding the different parameters related to projectile motion, we can make use of differential equations of motions:

$v=u-gt$

$s=ut-\dfrac{1}{2}g{{t}^{2}}$

${{v}^{2}}={{u}^{2}}-2gs$

Where,

• u = initial velocity 

• g = acceleration due to gravity

• t = time

• s = displacement

• v = final velocity

Total Time of Flight 

The horizontal range of a projectile is the distance it travels horizontally while in motion. This can be determined by the projectile's initial velocity, its angle of launch, and the gravitational force acting on it. The range is affected by air resistance, but this is usually negligible for most projectiles.

Here, the resultant displacement (s) = 0 in Vertical direction. Therefore, the time-of-flight formula is given by using the equation of motion:

\[g{{t}^{2}}=2({u_y}t-sy)\] 

Here \[{u_y}=u \sin \theta~ \text{ and} ~ {s_y}=0\] 

\[ \text{i}\text{.e, }g{{t}^{2}}=2t\times \text{u sin}\theta \]

Time of Flight of Projectile Formula

Therefore, the time-of-flight formula (t) is given by:

$\text{Total Time of Flight (t)=}\dfrac{2u\text{ sin}\theta }{g}$

Horizontal Range

The horizontal range of a projectile is the distance it travels horizontally while in motion. This can be determined by the projectile's initial velocity, its angle of launch, and the gravitational force acting on it. The range is affected by air resistance, but this is usually negligible for most projectiles.

Horizontal Range (OA) = Horizontal Component of Velocity (u_x) × Total Flight Time (t)

$R=u\cos \theta \times 2u\times \sin \theta g$

Horizontal Range of a Projectile Formula

Therefore, in a projectile motion, the horizontal range is given by (R):

$\text{Horizontal Range(R)=}\dfrac{{{u}^{2}}\sin 2\theta }{g}$

Maximum Height of Projectile

The height of a projectile is the maximum vertical distance that it reaches during its flight. The height depends on the initial velocity of the projectile, the angle at which it is launched, and the acceleration due to gravity.

If a projectile is launched horizontally, then its height will be equal to its horizontal range (the horizontal distance it travels before hitting the ground). However, if a projectile is launched at an angle other than 0° (horizontal), then its height will be less than its horizontal range.

Maximum Height of Projectile Formula

The formula for calculating the maximum height of a projectile is:

\[H=\dfrac{{{v}_{0}}^{2}{{\sin }^{2}}\theta }{2g}\]

The Equation of Trajectory

When an object is launched into the air, it is called a projectile. The path that the projectile follows is called its trajectory. The equation of trajectory can be used to predict the path of a projectile.

The equation of trajectory is $y=x\tan \theta -\dfrac{g{{x}^{2}}}{2{{u}^{2}}{{\cos }^{2}}\theta }$

Here:

• y is the height of the projectile at any given point along its trajectory (measured from the launch point).

• x is the horizontal distance travelled by the projectile from the launch point.

• $\theta $ is the angle at which the projectile was launched (measured from the horizontal).

• v is the initial velocity of the projectile (measured in metres per second).

• g is acceleration due to gravity ($9.8\text{ m/}{{\text{s}}^{2}}$).

Summary

We can summarise the whole article in a few lines. In projectile motion, an object is launched at some initial velocity in a given direction. It then continues to move in a curved path due to the force of gravity acting on it. The path of the object is called its trajectory. Projectile motion is a type of motion in which an object (called a projectile) is thrown or launched into the air and then falls back down to the ground. Projectiles follow a curved path as they travel through the air because they are affected by gravity. 

The shape of this trajectory depends on the initial velocity of the projectile and how high it is launched into the air. If a projectile is launched with a high velocity, it will have a flatter trajectory and will travel further before hitting the ground than one with a lower velocity. If it is launched at an angle, it will have a curved trajectory and will land at a different point than if it was launched straight up into the air. Projectile motion can be used to model many real-world situations, such as throwing a ball, shooting an arrow from a bow, or firing cannonballs from cannons.