Purely Resistive Inductive and Capacitive Circuits for JEE Main 2025 Physics

When studying electrical circuits for JEE Main, it's essential to understand the role of different components and how they interact with current and voltage. Purely resistive inductive and capacitive circuits each have unique characteristics that impact the flow of electricity. These types of circuits form the basis of many AC (alternating current) systems and are crucial in electrical engineering.

Understanding these circuits is key to designing and analysing more complex systems, as they influence the overall impedance, energy storage, and the relationship between voltage and current in AC circuits. On this page, we’ll explore these fundamental concepts in more detail, helping you grasp how each circuit type works and their practical applications.

Pure Resistive Circuit Definition, Circuit Diagram, Properties, Phasor diagram and Formula

Definition: A pure(ly) resistive circuit has a very negligible amount of inductance such that the reactance offered by such circuits is very small when compared to the resistance even at normal frequency.

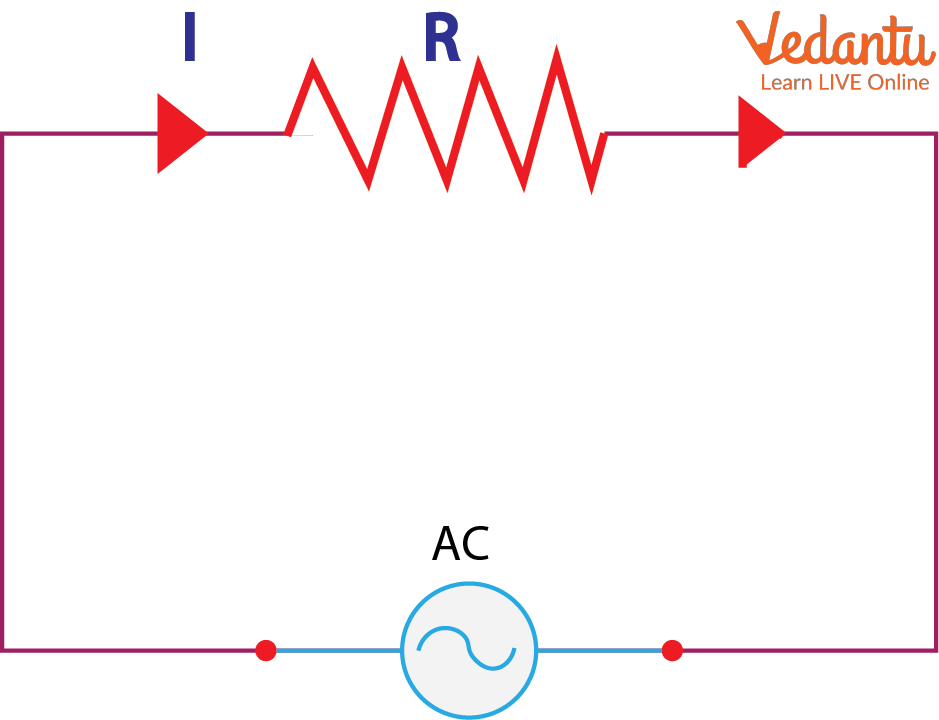
Pure Resistive Circuit Diagram

The entire voltage applied over this circuit is exploited in overcoming the extremely high amount of resistance. Hence, these are often called non-inductive circuits.

Purely Resistive Circuit Properties

• No presence of inductance and capacitance.

• The backward movement of both the alternating current and the voltage can be observed. Hence, they form a sine wave or sinusoidal waveform.

• The value of current and voltage reaches the maximum at the same time.

• The resistor is a passive device in such a circuit that converts the electrical energy into heat.

Phasor Diagram of Pure Resistive Circuit 

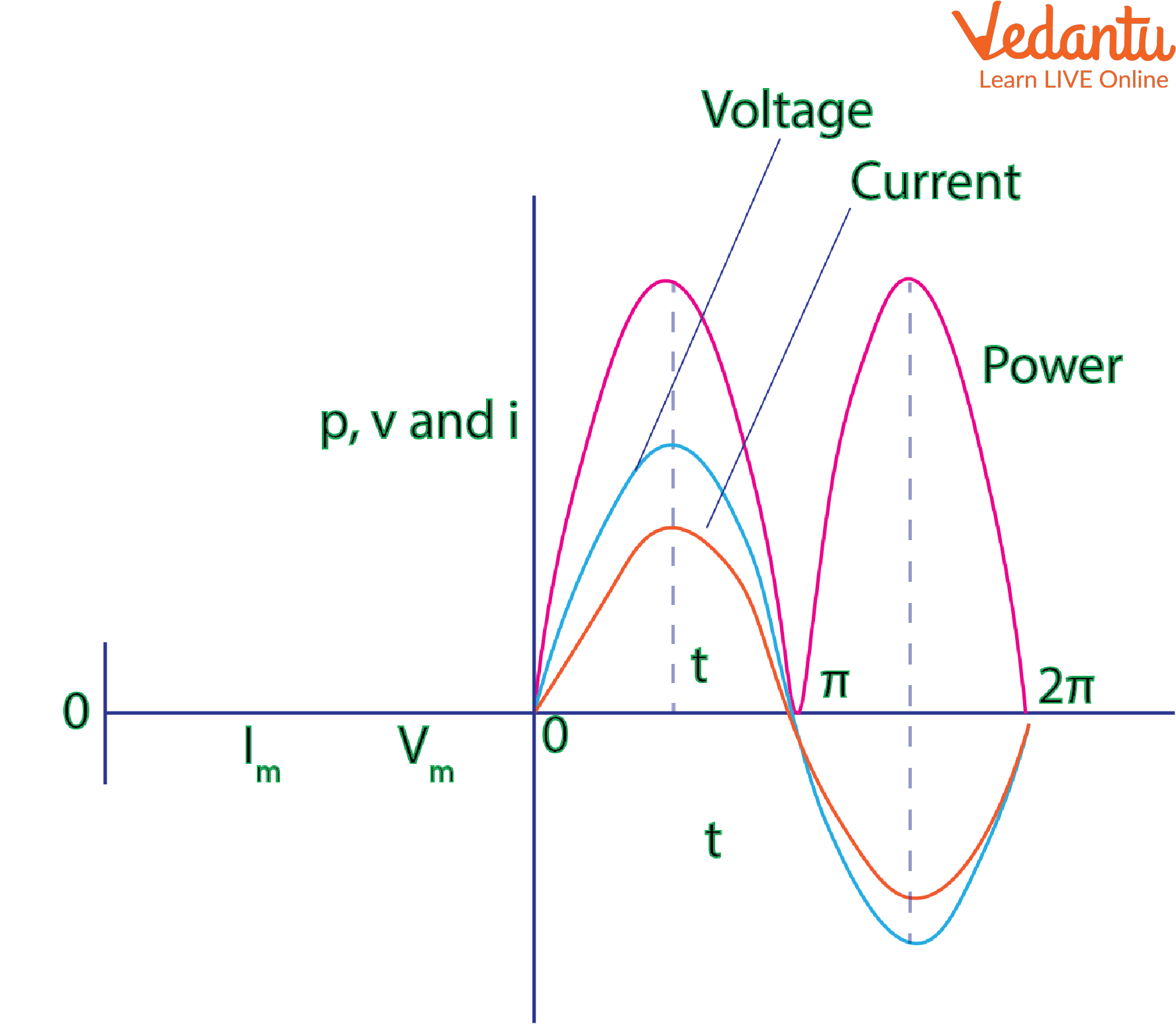
Phasor Diagram Of Pure Resistive Circuit and Waveform Diagram

Derivation of Formulas

The equation of the alternating voltage that is applied across the circuit is

$v={{V}_{m}}\sin \omega t$  …….. (1)

The current flowing through the purely resistive circuit can be derived as

$i=\frac{V}{R}=\frac{{{V}_{m}}}{R}\sin \omega t$   ………. (2)

$i={{I}_{m}}\sin \omega t$         …….. (3)

From (1) and (3), it is clear that no phase difference exists between the voltage applied and the current flowing through a purely resistive circuit. Hence, the current is in phase with the voltage and the phase angle is zero. As a result, both the current and the voltage attain a peak at the same time and for all values of current and voltage, the power curve (shown in the above graph in pink, blue, and red) is positive.

Hence, the equation for instantaneous power is p = vi

$p=\left( {{V}_{m}}\sin \omega t \right)\left( {{I}_{m}}\sin \omega t \right)$

$p=\frac{\left( {{V}_{m}}{{I}_{m}} \right)}{2}2{{\sin }^{2}}\omega t=\frac{{{V}_{m}}}{\sqrt{2}}\frac{{{I}_{m}}}{\sqrt{2}}\left( 1-\cos 2\omega t \right)$

The average power consumption over a complete cycle in a purely resistive circuit is given by

$p=avg\left( \frac{{{V}_{m}}}{\sqrt{2}}\frac{{{I}_{m}}}{\sqrt{2}} \right)-avg\left( \frac{{{V}_{m}}}{\sqrt{2}}\frac{{{I}_{m}}}{\sqrt{2}}\cos 2\omega t \right)$

The maximum current will be when $\omega t={{90}^{\circ }}$ and hence $\cos {{90}^{\circ }}=0$

$P={{V}_{rms}}{{I}_{rms}}$

where ${{V}_{rms}}$  and ${{I}_{rms}}$ are root mean square values of supply voltage and current, respectively, and P is the average power.  

Hence, the power in a purely resistive circuit is given by P = VI

The Power Factor of Pure Resistive Circuits

The Power Factor of a pure resistive circuit is 1 (or unity).

Explanation:

• In a pure resistive circuit, the voltage and current are in phase, meaning their phase angle ($\phi$) is $0^\circ$.

• The power factor ($\text{cos} \phi$) is given by: 

For $\phi = 0^\circ 

$\cos 0^\circ = 1$

Key Points:

1. Maximum Power Transfer: Since the power factor is 1, the circuit is most efficient in transferring power.

2. Power Calculation: The real power (P) is calculated using: $P = V \cdot I \cdot \cos \phi$ Here, cos⁡ϕ=1, so: $P = V \cdot I$

In pure resistive circuits, all the supplied power is converted into useful work or heat, with no reactive power involved.

Pure Inductive Circuit Definition, Circuit Diagram, properties, Phasor diagram and Formula

Definition:

Purely Inductive Circuit

Definition

Such a circuit theoretically has zero resistance and hence zero loss. A back EMF due to the self-inductance of the coil is produced whenever alternating voltage is applied to a purely inductive circuit. Due to the absence of ohmic resistance, the only force that the applied voltage has to overcome is the circuit’s self-inductance.

Pure Inductive Circuit Diagram

Pure Inductive Circuit Properties

• When the voltage and current values are at their positive peak, the value of power is also positive and similarly, when the voltage and current values are at their negative peak, power is also negative.

• The current value changes during a voltage drop and at the instant when the current value is at its peak, the voltage will reach zero.

• The voltage and current are out of phase with each other by 90^o.

Phasor Diagram Of Pure Inductive Circuit and Wavelength Diagram

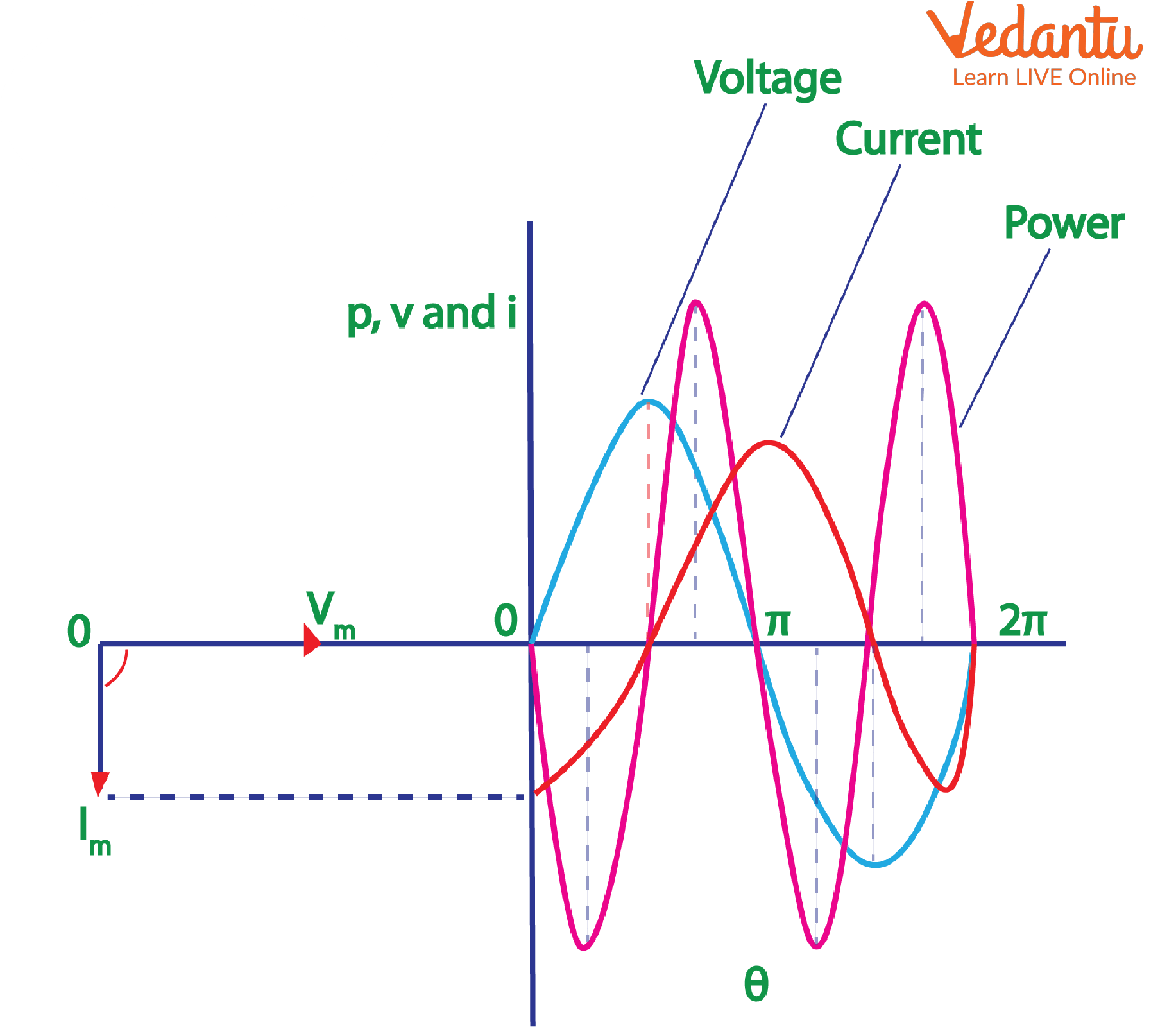
Phasor Diagram Of Pure Inductive Circuit and Waveform Diagram

Derivation of Formulas

The equation of the alternating voltage that is applied across the circuit is

$v={{V}_{m}}\sin \omega t$                 ………. (1)

This results in  current flow along with an induced emf given by

$e=-L\frac{di}{dt}$

Since the induced emf is equal and opposite to the applied voltage,

$v=-\left( -L\frac{di}{dt} \right)$

${{V}_{m}}\sin \omega t=L\frac{di}{dt}$

$di=\frac{{{V}_{m}}}{L}\sin \omega tdt$           ……… (2)

Integrating both sides of (2) we get,

$i=\frac{{{V}_{m}}}{\omega L}\sin \left( \omega t-\frac{\pi }{2} \right)=\frac{{{V}_{m}}}{{{X}_{L}}}\sin \left( \omega t-\frac{\pi }{2} \right)$                         ………. (3)

where X_L is the opposition offered by pure inductance to the flow of alternating current and is called inductive reactance.

The maximum current will be when $\sin \left( \omega t-\frac{\pi }{2} \right)=1$. Hence,

${{I}_{m}}=\frac{{{V}_{m}}}{{{X}_{L}}}$        ……… (4)

Substituting (3) in (4) we get,

$i={{I}_{m}}\sin \left( \omega t-\frac{\pi }{2} \right)$

The instantaneous power in a purely inductive circuit can be derived as follows:

$P = VI$

$P=\left( {{V}_{m}}\sin \omega t \right){{I}_{m}}\sin \left( \omega t+\frac{\pi }{2} \right)$

$P={{V}_{m}}{{I}_{m}}\sin \omega t\cos \omega t$

$P=\frac{{{V}_{m}}{{I}_{m}}}{2}2\sin \omega t\cos \omega t$

$P=\frac{{{V}_{m}}}{\sqrt{2}}\frac{{{I}_{m}}}{\sqrt{2}}\sin 2\omega t$

Or P = 0 i.e., average power consumption in a purely inductive circuit is zero.

Power in Pure Inductive Circuit

In a pure inductive circuit, the power alternates between positive and negative values, and the average power over a complete cycle is zero.

Pure Capacitive Circuit - Definition, Phasor Diagram, and Derivation of Formula

Definition: In this type of circuit, upon supply of alternative voltage, the capacitor gets charged first in one direction and next in another direction. Hence, it charges itself when there is supply and discharges when there is no supply.

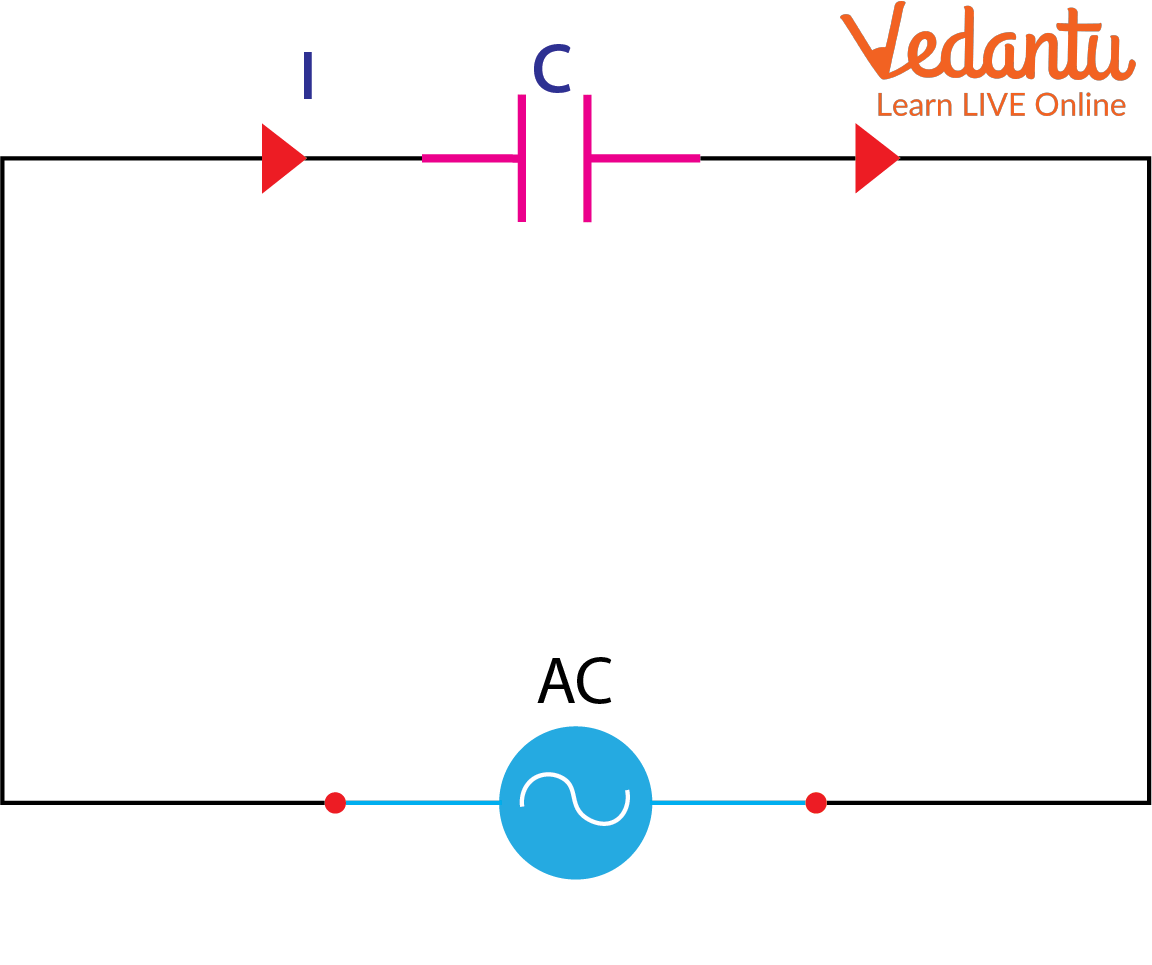
Pure Capacitive Circuit Diagram

Pure Capacitive Circuit Properties

• Current leads voltage by a phase difference of 90^o.

• When there is an increase in voltage, the positive half cycle begins, i.e., the capacitor gets charged and reaches its maximum capacity.

• Similarly, when there is a decrease in voltage, the negative half cycle happens leading to discharge from the capacitor.

• During maximum voltage, current flow is zero.

• Current starts to flow when voltage value decreases to a negative value.

Phasor Diagram Of Pure Capacitive Circuit and Wavelength Diagram

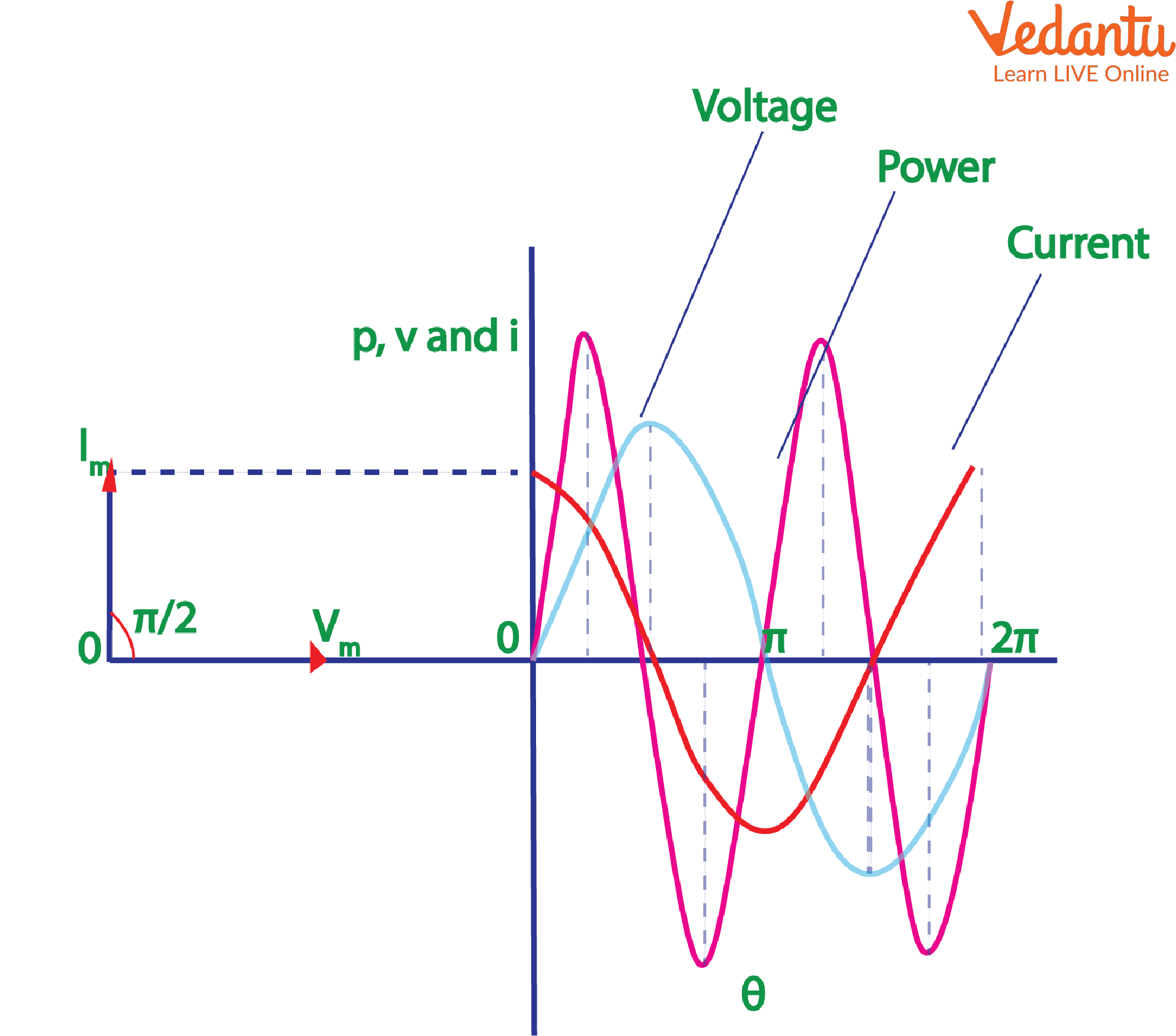
Phasor Diagram Of Pure Capacitive Circuit and Waveform Diagram

Derivation of Formulas

The equation of the alternating voltage that is applied across the circuit is

$v={{V}_{m}}\sin \omega t$    …. (1)

At any point in time, let the charge of the capacitor be

$q=Cv$        …. (2)

The current flowing through the purely capacitive circuit is given by:

$i=\frac{dq}{dt}$

Substituting (2) in the above equation,

$i=\frac{d}{dt}\left( Cv \right)$ …. (3)

$i=\frac{d}{dt}C{{v}_{m}}\sin \omega t=C{{v}_{m}}\frac{d}{dt}\sin \omega t$

$i=\omega C{{v}_{m}}\cos \omega t=\frac{{{V}_{m}}}{1/\omega C}\sin \left( \omega t+\frac{\pi }{2} \right)$

$i=\frac{{{V}_{m}}}{{{X}_{C}}}\sin \left[ \omega t+\frac{\pi }{2} \right]$ …. (4)

where is ${{X}_{C}}=1/\omega C$ the capacitive reactance

Current will be maximum when  $\sin \left( \omega t+\frac{\pi }{2} \right)=1$. Hence,

${{I}_{m}}=\frac{{{V}_{m}}}{{{X}_{C}}}$

On substituting this in (4) we get,

$i={{I}_{m}}\sin \left( \omega t+\frac{\pi }{2} \right)$

The instantaneous power in the capacitor P = VI

$P=\left( {{V}_{m}}\sin \omega t \right){{I}_{m}}\sin \left( \omega t+\frac{\pi }{2} \right)$

$P={{V}_{m}}{{I}_{m}}\sin \omega t\cos \omega t$

$P=\frac{{{V}_{m}}}{\sqrt{2}}\frac{{{I}_{m}}}{\sqrt{2}}\sin 2\omega t$

Or P = 0, i.e., average power consumption in a purely inductive circuit is zero.

Power in Pure Capacitor Circuit

In a pure capacitive circuit, the power alternates between positive and negative values, and the average power over a complete cycle is zero.

Differences Between Purely Resistive Inductive and Capacitive Circuits

Summary

In order to understand a general AC circuit, the capacitance, inductance, and resistance factors must be studied in isolation. It will yield important results about the relationship between current and voltage, the phase difference, and the average power consumption.

JEE Main 2025 Subject-Wise Important Chapters

The JEE Main 2025 subject-wise important chapters provide a focused strategy for Chemistry, Physics, and Maths. These chapters help students prioritise their preparation, ensuring they cover high-weightage topics for better performance in the exam.

Check Other Important Links for JEE Main 2025

Prepare effectively for JEE with our comprehensive study material links, including notes, question papers, sample papers, and mock test series for both JEE Main and Advanced. Access essential resources like formulas, important questions, and preparation tips to boost your performance.