Speed of Electromagnetic Waves - Formulae based Solved Examples

Electromagnetic waves, as the name even portrays that they are the combination of electric and magnetic signals. Generally, when there is a presence of an electric field and a magnetic field at the same time together, this results in electromagnetic waves. Wherein, both the fields of electric and magnetic currents are perpendicular to each other making an angle of 90 degrees. These waves can be produced in a kind of media such as vacuum, air, water or solid material. There is no need for a medium for the propagation of electromagnetic waves. The wavelength and the amplitude of these waves are generally taken into concern for the measurement of their other properties. This is why they are often termed transverse waves. 

Let us dive into the concept of the speed of electromagnetic waves in vacuum and the speed of electromagnetic waves in a medium in detail. 

Electromagnetic Wave Propagation

The source of electromagnetic waves is the charges that are accelerated and can radiate energy in the form of waves. Importantly, the speed of electromagnetic wave depends upon the source of its radiation. Suppose, let's say a charge is oscillating in a medium and it can be able to produce an oscillating electric field thereby giving rise to the oscillating magnetic field. These two oscillating electric and magnetic fields keep moving along and propagate through space as electromagnetic waves.

Let us learn about some characteristics of electromagnetic waves. EM waves travel with the speed of light, i.e. 3 x 10⁸ m/s and both the electric field, E and the magnetic field, B has the same frequency as they keep oscillating and remain in phase with each other throughout the propagation. With these characteristics, we can draw how an EM wave propagates in a medium on the XYZ plane. Say, if the electromagnetic wave is travelling in the positive direction on the x-axis, the electric field will be oscillating parallel to the y-axis and the magnetic field which should be perpendicular to the electric field will be oscillating parallel to the z-axis. Then we would be able to draw these electric and magnetic fields as the sinusoidal functions of the position, x and time, t as shown below.

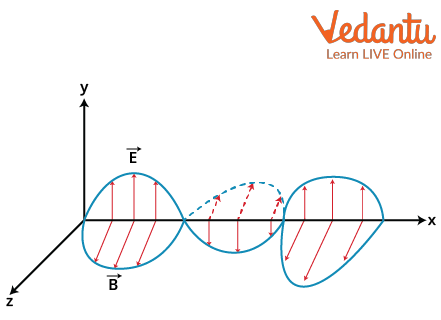
Electromagnetic wave on XYZ plane

Therefore, in the above image, we can see that E and B are perpendicular to each other. These two fields can be written as sinusoidal functions as shown below.

$E=E_{0} \operatorname{Sin}(k x-\omega t)$ and $B=B_{0} \operatorname{Sin}(k x-\omega t)$

These two are functions of the position, x and time, t. 

Wherein, k is known as the wave number, which is defined by the equation, 

$k=\dfrac{2\pi}{\lambda}$        

Where $\lambda$ is known as the wavelength of the Electromagnetic wave. 

Wavelength is the distance between the two amplitudes of a wave and this is the ratio of the velocity of the wave to its frequency in a medium.

I.e.,

$\lambda=\dfrac{c}{f}$ where f is the frequency of the wave and c is the speed of light in a vacuum, i.e., $c=3\times10^{8}~\dfrac{m}{s}$. 

In the above functions of electric and magnetic fields, 𝜔 is the angular frequency of the EM wave and it is given by the formula, $\omega=2\pi f$ where f is the frequency of the EM wave in the medium. 

And, Bo and Eo are the amplitudes of the magnetic and electric fields propagating in an electromagnetic wave whose relation is given by, Eo = CBo where c is the speed of the light. 

Now let us learn about the permittivity and the permeability of electric and magnetic fields to derive the speed of electromagnetic waves in a medium. 

Speed of Electromagnetic Wave in a Medium 

To derive the speed of electromagnetic wave formula, we have to learn about the terms like permittivity and the permeability of electric and magnetic fields. 

Permittivity

Permittivity is the measure of the number of charges needed to produce one unit of electric flux in a given medium. This varies from medium to medium. It is denoted by 𝝴 and its units are farad/metre. In all of the media, the vacuum has the least permittivity and it is denoted by $\epsilon_0$. This is also known as the permittivity of free space and its value is equal to $8.85 \times 10^{-12}~\dfrac{Farad}{metre}$. The ratio of the permittivity of a medium to the permittivity of the vacuum is known as relative permittivity and it is denoted by $\epsilon_r$ 

where $\epsilon_r=\dfrac{\epsilon}{\epsilon_0}$

Permeability 

Permeability is the measure of the ability of a medium to be able to produce magnetic flux in it. This is denoted by the symbol, $\mu$. The permeability of the vacuum is termed as permeability constant, $\mu_0$ and that is equal to $4\pi \times 10^{-7}$. The relative permeability is given by the formula, $\mu_r=\dfrac{\mu}{\mu_0}$   

Now, we know that the speed of electromagnetic wave in free space or vacuum is equal to the speed of the light, $c = 3 \times 10^8~\dfrac{m}{s}$, where c is given by the relation between permittivity and permeability in a vacuum as follows, 

$c_{0}=\dfrac{1}{\sqrt{\varepsilon_{o} \mu_{o}}}=3 \times 10^{8} \dfrac{\mathrm{~m} }{ \mathrm{s}}$

The speed of electromagnetic wave in a medium if it is not a vacuum can be written as 

$\mathbf{c}_{\text {med }}=\dfrac{1}{\sqrt{\mu \varepsilon}}$     - - - - - (1) 

From the definitions of permittivity and permeability, we know that

${\mu}=\mu_{\mathrm{o}} \mu_{\mathrm{r}}$ and $\varepsilon=\varepsilon_{\mathrm{o}} \varepsilon_{\mathrm{r}}$

Putting these two values in equation 1, we obtain 

$\mathbf{C}_{\text {med }}=\dfrac{1}{\sqrt{\mu_{o} \varepsilon_{o} \mu_{r} \varepsilon_{r}}}$

Where $C_{o}=\dfrac{1}{\sqrt{\varepsilon_{o} \mu_{o}}}$ and the above eq becomes as follows, 

$C_{\text {medium }}=\dfrac{C_{0}}{\sqrt{\mu_{r} \varepsilon_{r}}}$

This is the relation between the speed of electromagnetic waves in a medium and the speed of electromagnetic waves in free space. 

Here, $\sqrt{\mu_{r} \varepsilon_{r}}=\dfrac{C_{0}}{C_{\text {medium }}}=n$

Where n is the refractive index of a medium. 

Note: The speed of electromagnetic waves in vacuum is the same even if they are at different wavelengths. Let us solve some problems related to the speed of electromagnetic waves in air or a medium. 

Solved Examples on EM Wave Speed

Example 1: An electromagnetic wave is travelling in a medium whose relative permittivity is equal to 2.25 and relative permeability is equal to 1. Find the speed of the EM wave in that medium. 

Solution:

Given that, Relative permeability, $\mu_r = 1$ and the relative permittivity, $\epsilon_r= 2.25$

And we know $c= 3 \times 10^8~\dfrac{m}{s}$ 

By the speed of electromagnetic wave formula, $C_{\text {medium }}=\dfrac{C_{0}}{\sqrt{\mu_{r} \varepsilon_{r}}}$

Substituting all the given values, we get 

$C_{\text {medium }}=\dfrac{3 \times 10^{8}}{\sqrt{1 \times 2.25}}=2 \times 10^{8} \dfrac{\mathrm{m}}{\mathrm{s}}$

Therefore, the velocity of the EM wave in that medium is $2 \times 10^8~\dfrac{m}{s}$.

Example 2: An electromagnetic wave is travelling through a medium whose equations of electric and magnetic fields are given by, E = 35sin(kx-wt) and B = Bosin(kx-wt). Find the value of  Bo. 

Solution:

Given that, 

E = 35sin(kx-wt) where Eo = 35 Newton/Coulomb  

And we know the relation between the amplitudes of electric and magnetic field functions in an electromagnetic wave as,  Eo = CBo where 

$B_{o}=\dfrac{E_{o}}{C}=\dfrac{35}{3 \times 10^{8}}=11.67 \times 10^{-8}~\text { Tesla }$ 

Therefore, the amplitude of the magnetic field function is $11.67 \times 10^{-8}$ Tesla

Let us conclude what we have learnt till now. 

Conclusion 

In this article, we derived the equation and learnt the relationship between the speed of electromagnetic waves in a medium and in a vacuum. The speed of an electromagnetic wave depends upon the source from which it is radiated.  Electromagnetic waves are transverse waves with sustaining oscillations of electric and magnetic fields in a medium. There are many applications of EM waves in radios, microwave radiations and in infrared radiations.