Question

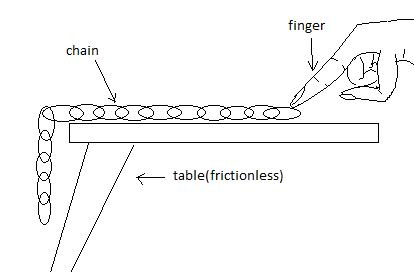
A chain is held on a frictionless table with one-fourth of its length hanging over the edge as shown in figure. If the chain has length $L = 28cm$ and mass $m = 0.012Kg. $how much work is required to pull the hanging part back onto the table?

Answer 1

Hint: On the hanging part of the chain, gravitational force is acting. To pull the string back to the table we need to apply a force which is opposite to the gravitational force. This force will have the same magnitude as the gravitational force, but the direction will be opposite. The work which we need to calculate in this question is the work done against the gravitational force.

Formula used:
Potential energy,
$U = mgh$ (where $U$ stands for the potential energy stored in an object placed at a height, $m$ stands for the mass of the object, $g$ is the acceleration due to gravity ($g = 9.8m{s^{ - 2}}$) and $h$ stands for the height at which the object is stored)

Complete step by step solution:
It is given that one by fourth of the length $L$ is hanging over the edge of the table.
Consider small elements of length $dy$ on the hanging part of the string
Let $m$ be the mass of the string.
Mass per unit length of the string $ = \dfrac{m}{L}$
Mass of each small element of length $L = \left( {\dfrac{m}{L}} \right)dy$
Let the string segment be hanging at a distance $y$below the table-top
Since the origin is at the table top and the string is hanging downwards $y$ will be negative.
The potential energy is $U = mgh$
Substituting the values of $m$ and $h$ in the above equation
The change in potential energy for a small element,
$dU = - \left( {\dfrac{m}{L}} \right)gydy$
The change in potential energy for the string can be obtained by integrating the above equation
$\Rightarrow$ $U = \int {dU} $
Since the length of the segment hanging is $\dfrac{L}{4}$ and it is hanging downwards, to find the potential we have to integrate from $ - \dfrac{L}{4}$ to $0$.
$\Rightarrow$ $U = \int\limits_{\dfrac{{ - L}}{4}}^0 {\dfrac{{ - mg}}{L}} ydy$
Taking the constants out,
$\Rightarrow$ $U = \dfrac{{ - mg}}{L}\int\limits_{\dfrac{{ - L}}{4}}^0 {ydy} $
Integrating the above equation,
$\Rightarrow$ $U = \dfrac{{ - mg}}{L}\left[ {\dfrac{{{y^2}}}{2}} \right]_{\dfrac{{ - L}}{4}}^0$
Applying the limits,
$\Rightarrow$ $U = \dfrac{{ - mg}}{{2L}}\left[ {0 - {{\left( {\dfrac{{ - L}}{4}} \right)}^2}} \right]$
Opening the brackets, we get
$\Rightarrow$ $U = \dfrac{{ - mg}}{{2L}}\left[ {0 - \dfrac{{{L^2}}}{{16}}} \right]$
$\Rightarrow$ $U = \dfrac{{mg}}{{2L}}\left( {\dfrac{{{L^2}}}{{16}}} \right)$
Solving the above equation, we get
$\Rightarrow$ $U = \dfrac{{mgL}}{{32}}$……………………(A)
The values of $m,g,\& L$ is given in the question
$m = 0.012kg$, $L = 28cm$, $g = 9.8m{s^{ - 2}}$
Substituting these values into the equation (A)
$\Rightarrow$ $U = \dfrac{{0.012 \times 9.8 \times 0.28}}{{32}} = 1.029 \times {10^{ - 3}}$
The work done will be equal to this change in potential energy.

The answer is: $1.029 \times {10^{ - 3}}J$.

Note: The work done in pulling the string will be equal to the change in potential energy as the potential energy will change with the change in height. Since the length of the hanging segment of the string will change from $\dfrac{L}{4}$ to $0$ the work done will also change. The gravitational potential depends on the weight of the object and the height of the object. All the values should be converted into MKS values before calculation.