
A particle starting from rest undergoes a rectilinear motion with acceleration
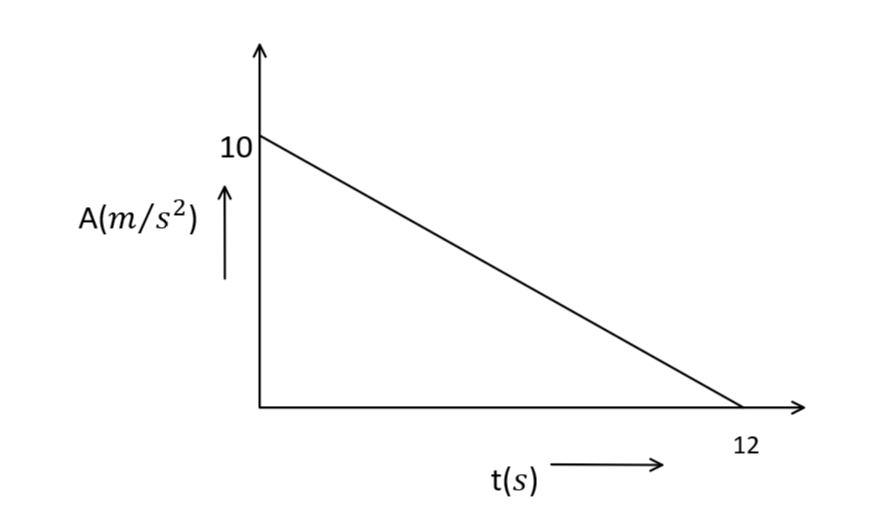
A)
B)
C)
D)
Answer
142.8k+ views
Hint: In this question, we have to find the maximum velocity during the rectilinear motion with acceleration
Complete step by step answer:
In this question, we have to find the maximum velocity during the rectilinear motion with acceleration (
Since, we know that
Or we can write the above equation as,
Therefore, for finding the maximum velocity we have to find the area under the curve where
As we can see in the graph the maximum value of a is
Now, since the graph is in the form of triangle, area under the curve will be,
On putting the values we get,
Hence, the maximum velocity during the rectilinear motion with acceleration (
Note: For this type of question, the best method to solve is to find the area under the curve for the required intervals by integration or else we can do it by the normal method as we did in the above question.
Complete step by step answer:

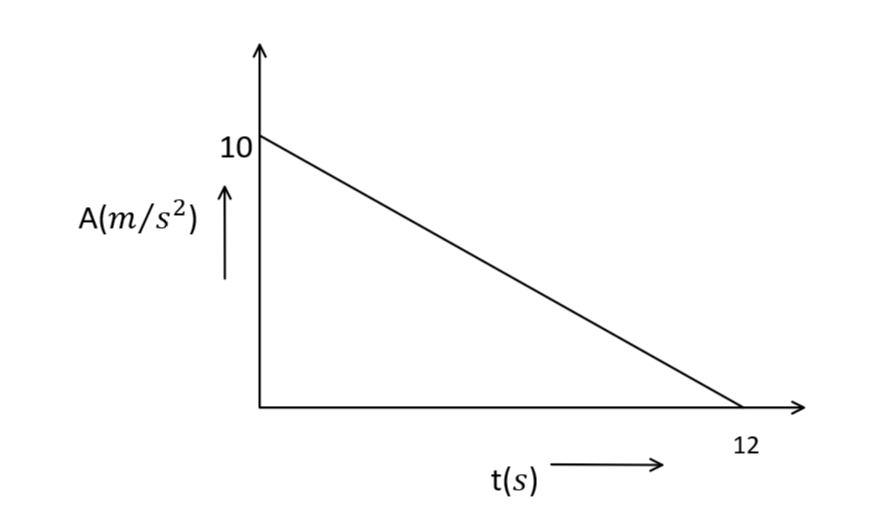
In this question, we have to find the maximum velocity during the rectilinear motion with acceleration (
Since, we know that
Or we can write the above equation as,
Therefore, for finding the maximum velocity we have to find the area under the curve where
As we can see in the graph the maximum value of a is
Now, since the graph is in the form of triangle, area under the curve will be,
On putting the values we get,
Hence, the maximum velocity during the rectilinear motion with acceleration (
Note: For this type of question, the best method to solve is to find the area under the curve for the required intervals by integration or else we can do it by the normal method as we did in the above question.
Latest Vedantu courses for you
Grade 10 | MAHARASHTRABOARD | SCHOOL | English
Vedantu 10 Maharashtra Pro Lite (2025-26)
School Full course for MAHARASHTRABOARD students
₹33,300 per year
EMI starts from ₹2,775 per month
Recently Updated Pages
How to find Oxidation Number - Important Concepts for JEE

How Electromagnetic Waves are Formed - Important Concepts for JEE

Electrical Resistance - Important Concepts and Tips for JEE

Average Atomic Mass - Important Concepts and Tips for JEE

Chemical Equation - Important Concepts and Tips for JEE

Concept of CP and CV of Gas - Important Concepts and Tips for JEE

Trending doubts
JEE Main 2025 Session 2: Application Form (Out), Exam Dates (Released), Eligibility, & More

JEE Main Exam Marking Scheme: Detailed Breakdown of Marks and Negative Marking

JEE Main 2025: Derivation of Equation of Trajectory in Physics

Electric Field Due to Uniformly Charged Ring for JEE Main 2025 - Formula and Derivation

Degree of Dissociation and Its Formula With Solved Example for JEE

Electrical Field of Charged Spherical Shell - JEE

Other Pages
Units and Measurements Class 11 Notes: CBSE Physics Chapter 1

JEE Advanced Marks vs Ranks 2025: Understanding Category-wise Qualifying Marks and Previous Year Cut-offs

NCERT Solutions for Class 11 Physics Chapter 1 Units and Measurements

JEE Advanced 2025: Dates, Registration, Syllabus, Eligibility Criteria and More

Motion in a Straight Line Class 11 Notes: CBSE Physics Chapter 2

JEE Advanced Weightage 2025 Chapter-Wise for Physics, Maths and Chemistry




