
A point charge
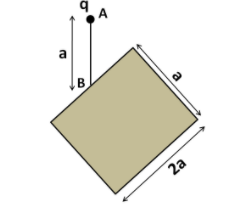
Answer
143.1k+ views
Hint: To solve this question, we need to use the gauss theorem. For this, we need to place the given charge in a closed volume, which has to be generated by using the rectangles given in the question. Then by using the unitary method we can find out the value of required flux through the given rectangle.
Complete step-by-step solution:
We know from the Gauss theorem that the total flux through a closed surface due to a point charge placed within it is given by
So we have to enclose the given point charge within a closed surface, symmetrically. We start by placing a similar rectangle adjacent to the given rectangle, so that the point charge is directly above the centre of the square thus formed, as shown in the figure below.
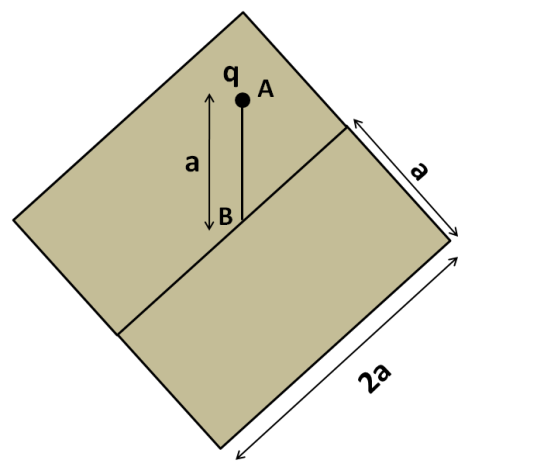
So now we have our point charge placed at above the centre of the square of side
For generating a closed volume, we have to place five additional such squares of side
As the charge is symmetrically present within the cube, so the flux through each of the squares of side
Now, the base square of side
According to the question,
Hence, the flux through the given rectangle is equal to
Note: The application of the gauss theorem does not depend on the symmetric placement of the charge within the closed surface. The charge just needs to be present within it. But for calculating the flux through a particular portion of the closed surface, we need the symmetry.
Complete step-by-step solution:
We know from the Gauss theorem that the total flux through a closed surface due to a point charge placed within it is given by
So we have to enclose the given point charge within a closed surface, symmetrically. We start by placing a similar rectangle adjacent to the given rectangle, so that the point charge is directly above the centre of the square thus formed, as shown in the figure below.

So now we have our point charge placed at above the centre of the square of side
For generating a closed volume, we have to place five additional such squares of side
As the charge is symmetrically present within the cube, so the flux through each of the squares of side
Now, the base square of side
According to the question,
Hence, the flux through the given rectangle is equal to
Note: The application of the gauss theorem does not depend on the symmetric placement of the charge within the closed surface. The charge just needs to be present within it. But for calculating the flux through a particular portion of the closed surface, we need the symmetry.
Latest Vedantu courses for you
Grade 10 | MAHARASHTRABOARD | SCHOOL | English
Vedantu 10 Maharashtra Pro Lite (2025-26)
School Full course for MAHARASHTRABOARD students
₹33,300 per year
EMI starts from ₹2,775 per month
Recently Updated Pages
How to find Oxidation Number - Important Concepts for JEE

How Electromagnetic Waves are Formed - Important Concepts for JEE

Electrical Resistance - Important Concepts and Tips for JEE

Average Atomic Mass - Important Concepts and Tips for JEE

Chemical Equation - Important Concepts and Tips for JEE

Concept of CP and CV of Gas - Important Concepts and Tips for JEE

Trending doubts
JEE Main 2025 Session 2: Application Form (Out), Exam Dates (Released), Eligibility, & More

JEE Main Exam Marking Scheme: Detailed Breakdown of Marks and Negative Marking

JEE Main 2025: Derivation of Equation of Trajectory in Physics

Electric Field Due to Uniformly Charged Ring for JEE Main 2025 - Formula and Derivation

Electric field due to uniformly charged sphere class 12 physics JEE_Main

Degree of Dissociation and Its Formula With Solved Example for JEE

Other Pages
JEE Advanced Marks vs Ranks 2025: Understanding Category-wise Qualifying Marks and Previous Year Cut-offs

JEE Advanced 2025: Dates, Registration, Syllabus, Eligibility Criteria and More

JEE Advanced Weightage 2025 Chapter-Wise for Physics, Maths and Chemistry

Dual Nature of Radiation and Matter Class 12 Notes: CBSE Physics Chapter 11

Formula for number of images formed by two plane mirrors class 12 physics JEE_Main

Electrical Field of Charged Spherical Shell - JEE




