Question

A proton of mass \[1.67 \times {10^{ - 27}}\;kg\] and charge \[1.68 \times {10^{ - 19}}\;C\] is projected with a speed of \[2 \times {10^6}m/s\;\]at an angle of \[{60^o}\]to the \[X\]−axis. If a uniform magnetic field of \[0.10\;T\] is applied along \[Y\]−axis, the path of the proton is
A. a circle of radius \[0.2m\] and time period \[\pi \times {10^{ - 7}}\;s\]
B. a circle of radius \[0.1m\] and time period \[2\pi \times {10^{ - 7}}\;s\]
C. a helix of radius \[0.1m\] and time period \[2\pi \times {10^{ - 7}}\;s\]
D. a helix of radius \[0.2m\] and time period \[4\pi \times {10^{ - 7}}\;s\]

Answer 1

Hint: In the given question, we need to determine the path of a proton. That means we need to find the radius of the circular path and the time period of the particle moving along a circular path. For this, we need to use the following formulae for radius and time period to get the desired result.







Formula used:
The following formulae are used to solve the given question.
The radius of circular path of charged particle is \[r = \dfrac{{mv\sin \theta }}{{qB}}\].
Here, \[r\] indicates the radius of curvature of the path of a charged particle with mass \[m\] as well as charge \[q\] travelling at a speed \[v\] perpendicular to a magnetic field of intensity \[B\].
Also, the time period is \[T = \dfrac{{2\pi m}}{{qB}}\]
Here, \[T\] is the time period.






Complete answer:
Consider the following figure.

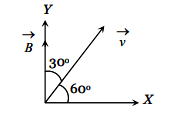
Image: Angle between magnetic field of intensity and velocity
We know that the radius of circular path of charged particle is \[r = \dfrac{{mv\sin \theta }}{{qB}}\]
But \[m = 1.67 \times {10^{ - 27}}\;kg,v = 2 \times {10^6}m/s\;,q = 1.68 \times {10^{ - 19}}\;C,\theta = {30^o},\] and \[B = 0.10\;T\]
This gives, \[r = \dfrac{{1.67 \times {{10}^{ - 27}}\; \times 2 \times {{10}^6} \times \sin {{30}^o}}}{{1.68 \times {{10}^{ - 19}} \times 0.10}}\]
By simplifying, we get
\[r = 0.1{\rm{ }}m\]
Also, the time period is given by
\[T = \dfrac{{2\pi m}}{{qB}}\]
But \[m = 1.67 \times {10^{ - 27}}\;kg\;,q = 1.68 \times {10^{ - 19}}\;C,\] and \[B = 0.10\;T\]
So, we get \[T = \dfrac{{2\pi \times 1.67 \times {{10}^{ - 27}}}}{{1.68 \times {{10}^{ - 19}} \times 0.10}}\]
By solving, we get
\[T = 2\pi \times {10^{ - 7}}{\rm{ S}}\]
Hence, the path of proton is a circular of radius \[0.1{\rm{ }}m\] and time period \[2\pi \times {10^{ - 7}}\;s\].
Therefore, the correct option is (B).






Note:Many students make mistake in simplification part. That means, they may get wrong while calculating the power of ten. Also, it is necessary to use diagrammatic representation to illustrate the given condition.