Question

A rod of length L, having negligible thickness, has a linear mass density that varies from zero at one end to $\lambda$ at the other. Locate the centre of mass.

Answer 1

Hint: We should know that linear density is the measure of a quantity of any characteristic value per unit of length. Linear mass density and linear charge density or we can say the amount of electric charge per unit length are two common examples used in science and engineering. Linear mass density has the dimension mass per length. The SI composite unit of linear mass density is the kilogram per meter. Density can be inferred by knowing the relationship between mass and volume. For any given substance, there is a linear relationship between its mass and its volume: when one variable increases, the other variable increases, and vice versa. On the basis of this concept we have to answer this question.

Complete step by step answer
Let us first write the diagram:

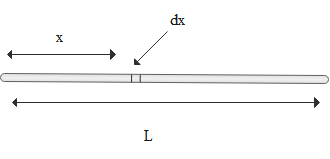
As the rod is forming a linear expression it must be in the x-axis, so we can write that:
Hence, $\mathrm{Y}_{\mathrm{cm}}=0, \mathrm{Z}_{\mathrm{cm}}=0$
For coordinate

$\mathrm{x}_{\mathrm{cm}}=\dfrac{\int_{0}^{\mathrm{L}}

\mathrm{xdm}}{\int_{0}^{\mathrm{L}} \mathrm{L}} \mathrm{dm}=\lambda.

\mathrm{dx}=(\mathrm{A}+\mathrm{Bx}) \mathrm{dx}$

We can say this because the basic expression for any linear equation is $(A+Bx)$.
Hence, we can say that: $\lambda =(A+Bx)$

Therefore, on the integration of the expression we get:

$\mathrm{x}_{\mathrm{cm}}=\dfrac{\int_{0}^{\mathrm{L}}
\mathrm{x}(\mathrm{Ax}+\mathrm{B})
\mathrm{dx}}{\int_{0}^{\mathrm{L}}(\mathrm{A}+\mathrm{Bx})
\mathrm{dx}}=\dfrac{\mathrm{L}(3 \mathrm{A}+2 \mathrm{BL})}{3(2

\mathrm{A}+\mathrm{BL})}$

So, after the evaluation we get that $\left(\dfrac{\mathrm{L}(3 \mathrm{A}+2

\mathrm{BL})}{3(2 \mathrm{A}+\mathrm{BL})}, 0,0\right)$are the coordinates. Since it is

linear in nature, the x-axis equation will have y and z coordinates as zero.
Here coordinates of the centre of mass are $\left(\dfrac{\mathrm{L}(3 \mathrm{A}+2

\mathrm{BL})}{3(2 \mathrm{A}+\mathrm{BL})}, 0,0\right)$.

Note: We should know that the standard form for linear equations in two variables is $(Ax+By)=C$. There are three major forms of linear equations: point-slope form, standard form, and slope-intercept form.
Now to solve such questions it is required to have a knowledge of all the forms of equations. So, the descriptions are as follows. Point-slope is the general form $y-{{y}_{}}=m\left( x-{{x}_{}} \right)$for linear equations. It emphasizes the slope of the line and a point on the line (that is not the y-intercept). The standard form of linear equation is of the type $ax\text{ }+\text{ }by\text{ }=\text{ }c$, the x and y terms are on the left side of the equation and the constant is on the right side. The constant and both of the coefficients must be integers. And lastly, the slope-intercept form is of the kind.$y\text{ }=\text{ }m\text{ }x\text{ }+\text{ }b\text{ }y\text{ }=\text{ }mx\text{ }+\text{ }b\text{ }y=mx+b$.