
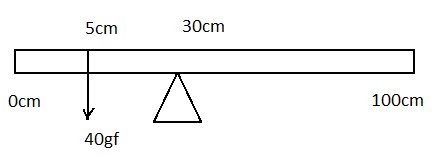
A uniform meter scale is in equilibrium position. Calculate the mass of the rule.
Answer
121.2k+ views
Hint: For solving this question we have to assume that the mass of the ruler be M grams. And the centre of mass of the ruler is at its centre. Therefore, the centre of mass of the ruler is $20cm$ which is away from the hinge. And it is given that the ruler is in equilibrium position. Now, balancing the torques due to mass of the rod which is suspended from the ruler. Knowing all these things we can calculate the mass of the ruler.
Complete step by step solution:
Suppose that the weight of the scale is $m$
And the meter scale is pivoted at $30cm$
And the torques are $40gf$ due to clockwise momentum.
Now,
Let ${T_1} = Mass \times Acceleration$ due to gravity
Now, distance of this weight from pivoted $ = \left( {30 - 5} \right)cm$
Which is $ = 25cm$
So, ${T_1} = 40 \times 25gfcm$
$ \Rightarrow 1000gfcm$
So, now the weight of the scale acts on its centre of mass which is situated at the mid points which is $50cm$
Now, we have to calculate the distance of centre of mass of the scale which is,
$ \Rightarrow 50 - 30$
$ \Rightarrow 20cm$
Now, again calculate for ${T_2}$
Which is weight of rod $ \times $ Distance of the weight pivoted
That is $ \Rightarrow m \times 20$
Now, the sum of clockwise momentum $ = $Sum of anticlockwise momentum.
So, ${T_1} = {T_2}$
Now, substituting the value
$\Rightarrow m = \dfrac{{1000}}{{20}}$
$\Rightarrow m = 50gf$
Hence, the mass of the ruler is $50gf$.
Note: For solving this question we have to remember that the weight of scale and the metre scale is pivoted. A uniform metre scale is kept in equilibrium and a mass is suspended. We have to balance the torque due to the mass of the rod. By using the formula of torque, we can find the mass of the ruler. So, these are some important things which we have to keep in mind while we solve this question to get the correct answer.
Complete step by step solution:
Suppose that the weight of the scale is $m$
And the meter scale is pivoted at $30cm$
And the torques are $40gf$ due to clockwise momentum.
Now,
Let ${T_1} = Mass \times Acceleration$ due to gravity
Now, distance of this weight from pivoted $ = \left( {30 - 5} \right)cm$
Which is $ = 25cm$
So, ${T_1} = 40 \times 25gfcm$
$ \Rightarrow 1000gfcm$
So, now the weight of the scale acts on its centre of mass which is situated at the mid points which is $50cm$
Now, we have to calculate the distance of centre of mass of the scale which is,
$ \Rightarrow 50 - 30$
$ \Rightarrow 20cm$
Now, again calculate for ${T_2}$
Which is weight of rod $ \times $ Distance of the weight pivoted
That is $ \Rightarrow m \times 20$
Now, the sum of clockwise momentum $ = $Sum of anticlockwise momentum.
So, ${T_1} = {T_2}$
Now, substituting the value
$\Rightarrow m = \dfrac{{1000}}{{20}}$
$\Rightarrow m = 50gf$
Hence, the mass of the ruler is $50gf$.
Note: For solving this question we have to remember that the weight of scale and the metre scale is pivoted. A uniform metre scale is kept in equilibrium and a mass is suspended. We have to balance the torque due to the mass of the rod. By using the formula of torque, we can find the mass of the ruler. So, these are some important things which we have to keep in mind while we solve this question to get the correct answer.
Recently Updated Pages
Difference Between Circuit Switching and Packet Switching

Difference Between Mass and Weight

JEE Main Participating Colleges 2024 - A Complete List of Top Colleges

JEE Main Maths Paper Pattern 2025 – Marking, Sections & Tips

Sign up for JEE Main 2025 Live Classes - Vedantu

JEE Main 2025 Helpline Numbers - Center Contact, Phone Number, Address

Trending doubts
JEE Mains 2025: Check Important Dates, Syllabus, Exam Pattern, Fee and Updates

JEE Main Login 2045: Step-by-Step Instructions and Details

Class 11 JEE Main Physics Mock Test 2025

JEE Main Chemistry Question Paper with Answer Keys and Solutions

JEE Main Exam Marking Scheme: Detailed Breakdown of Marks and Negative Marking

JEE Main 2023 January 24 Shift 2 Question Paper with Answer Keys & Solutions

Other Pages
JEE Advanced Marks vs Ranks 2025: Understanding Category-wise Qualifying Marks and Previous Year Cut-offs

NCERT Solutions for Class 11 Physics Chapter 9 Mechanical Properties of Fluids

Units and Measurements Class 11 Notes: CBSE Physics Chapter 1

JEE Advanced 2025: Dates, Registration, Syllabus, Eligibility Criteria and More

NCERT Solutions for Class 11 Physics Chapter 2 Motion In A Straight Line

NCERT Solutions for Class 11 Physics Chapter 7 Gravitation




