
A uniform rod of 20 kg is hanging in a horizontal position with the help of two threads. It also supports a 40 kg mass as shown in the figure. Find the tensions developed in each thread.

Answer
147k+ views
Hint: We will draw a free body diagram. We will calculate tension in string using translational equilibrium using
Complete step by step answer:
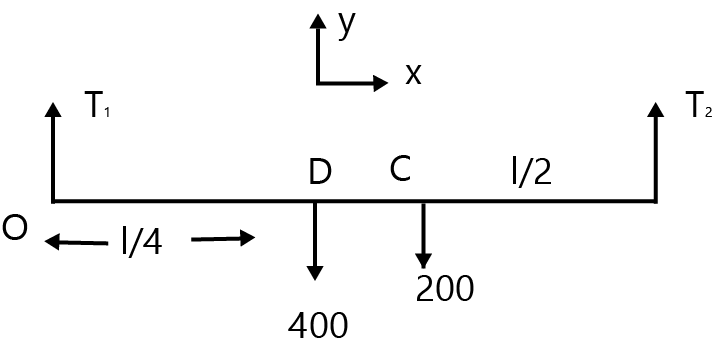
Free body diagram is shown in the figure.
Translational Equilibrium:
A body moving with constant velocity or no acceleration. Then it is said to possess translational equilibrium.
Rotational Equilibrium:
A body experiencing a constant rotational velocity or no angular acceleration.
In this case object shows a rotational motion in only one direction at a constant angular velocity
According to translational equilibrium
According to rotational equilibrium
Applying at A, we get
Therefore, tension in string AB is 600 N and tensions at point D and C is 200 N and 100 N respectively.
Note:
If value of g is taken as
Since the direction in which force is acting at point C and D is opposite to point A and B do opposite signs will be used while doing calculation. Negative signs used while calculating torque indicates direction.
Complete step by step answer:

Free body diagram is shown in the figure.
Translational Equilibrium:
A body moving with constant velocity or no acceleration. Then it is said to possess translational equilibrium.
Rotational Equilibrium:
A body experiencing a constant rotational velocity or no angular acceleration.
In this case object shows a rotational motion in only one direction at a constant angular velocity
According to translational equilibrium
According to rotational equilibrium
Applying at A, we get
Therefore, tension in string AB is 600 N and tensions at point D and C is 200 N and 100 N respectively.
Note:
If value of g is taken as
Since the direction in which force is acting at point C and D is opposite to point A and B do opposite signs will be used while doing calculation. Negative signs used while calculating torque indicates direction.
Recently Updated Pages
How to find Oxidation Number - Important Concepts for JEE

How Electromagnetic Waves are Formed - Important Concepts for JEE

Electrical Resistance - Important Concepts and Tips for JEE

Average Atomic Mass - Important Concepts and Tips for JEE

Chemical Equation - Important Concepts and Tips for JEE

Concept of CP and CV of Gas - Important Concepts and Tips for JEE

Trending doubts
JEE Main 2025 Session 2: Application Form (Out), Exam Dates (Released), Eligibility, & More

JEE Main Exam Marking Scheme: Detailed Breakdown of Marks and Negative Marking

JEE Main 2025: Derivation of Equation of Trajectory in Physics

Electric Field Due to Uniformly Charged Ring for JEE Main 2025 - Formula and Derivation

JEE Main Participating Colleges 2024 - A Complete List of Top Colleges

Degree of Dissociation and Its Formula With Solved Example for JEE

Other Pages
JEE Advanced Marks vs Ranks 2025: Understanding Category-wise Qualifying Marks and Previous Year Cut-offs

JEE Advanced 2025: Dates, Registration, Syllabus, Eligibility Criteria and More

Units and Measurements Class 11 Notes: CBSE Physics Chapter 1

NCERT Solutions for Class 11 Physics Chapter 1 Units and Measurements

Motion in a Straight Line Class 11 Notes: CBSE Physics Chapter 2

JEE Advanced Weightage 2025 Chapter-Wise for Physics, Maths and Chemistry




