
An electron, a proton, a deuteron, and an alpha particle, each having speed are in a region of constant magnetic field perpendicular to the direction of the velocities of the particles. The radius of the circular orbits of these particles is respectively
A.
B.
C.
D.
Answer
138.9k+ views
Hint: When a charged particle moves with definite velocity and enters a uniform magnetic field
Formula used:
The radius,
Here
Complete answer:
When a particle carrying charge
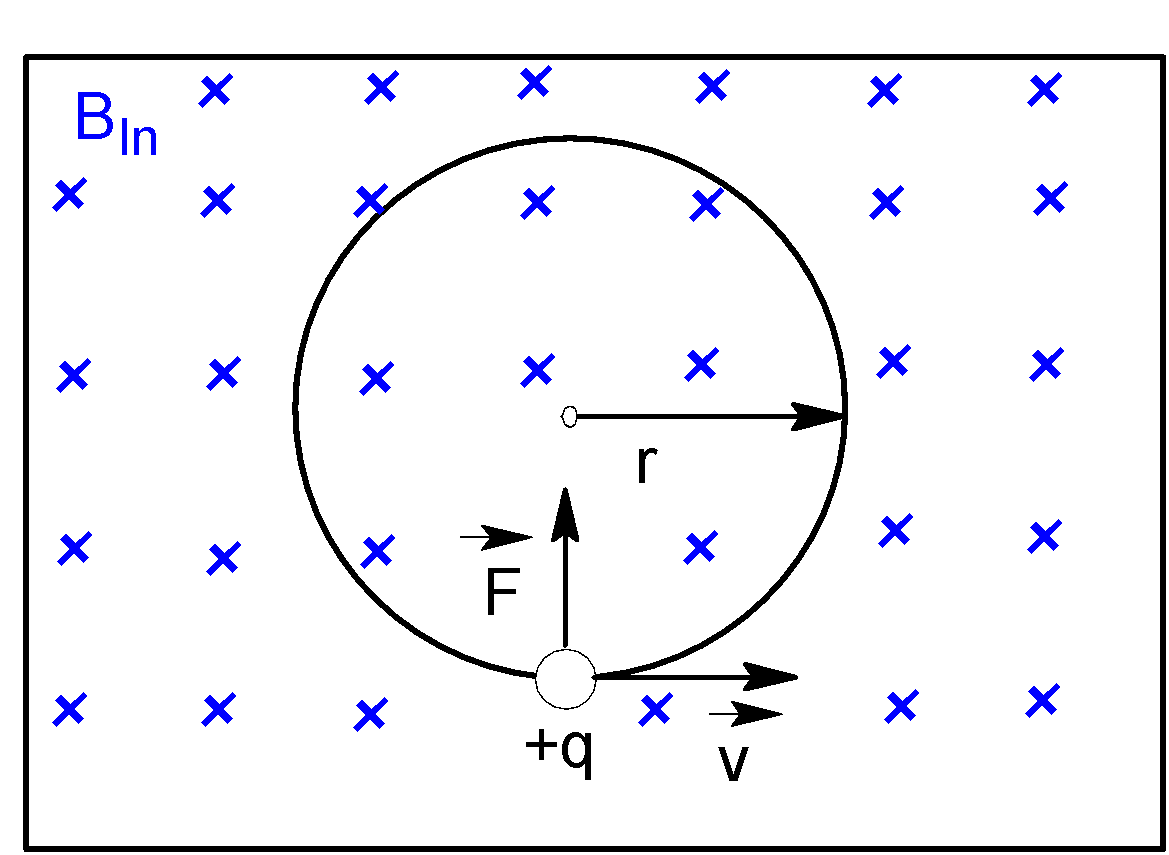
Or,
Or,
As a particle moves in a circular path, then magnetic force becomes a centripetal force
Hence by equating magnetic force with centripetal force,
Or,
Here we have four charged particles: an electron(
Therefore the radius of the circular path mainly depends on
Or,
Let us check
Let the Mass of a proton be
As we know the mass of an electron, deuterium and an alpha particle are
Therefore
Thus, option (C) is correct.
Note:Neutron does not feel any magnetic force while other charged particles experience that force. A neutron is a neutral particle, having no charge. But for charged particle trajectory curvature is proportional to the mass by charge ratio for a definite velocity.
Formula used:
The radius,
Here
Complete answer:
When a particle carrying charge

Or,
Or,
As a particle moves in a circular path, then magnetic force becomes a centripetal force
Hence by equating magnetic force with centripetal force,
Or,
Here we have four charged particles: an electron(
Therefore the radius of the circular path mainly depends on
Or,
Let us check
Let the Mass of a proton be
| Proton | Electron | deuteron | deuteron | |
| Mass( | ||||
| Charge( | | | | |
As we know the mass of an electron, deuterium and an alpha particle are
Therefore
Thus, option (C) is correct.
Note:Neutron does not feel any magnetic force while other charged particles experience that force. A neutron is a neutral particle, having no charge. But for charged particle trajectory curvature is proportional to the mass by charge ratio for a definite velocity.
Recently Updated Pages
Young's Double Slit Experiment Step by Step Derivation

How to find Oxidation Number - Important Concepts for JEE

How Electromagnetic Waves are Formed - Important Concepts for JEE

Electrical Resistance - Important Concepts and Tips for JEE

Average Atomic Mass - Important Concepts and Tips for JEE

Chemical Equation - Important Concepts and Tips for JEE

Trending doubts
JEE Main 2025 Session 2: Application Form (Out), Exam Dates (Released), Eligibility, & More

JEE Main 2025: Derivation of Equation of Trajectory in Physics

A point charge + 20mu C is at a distance 6cm directly class 12 physics JEE_Main

Learn About Angle Of Deviation In Prism: JEE Main Physics 2025

Electric Field Due to Uniformly Charged Ring for JEE Main 2025 - Formula and Derivation

JEE Main 2025: Conversion of Galvanometer Into Ammeter And Voltmeter in Physics

Other Pages
JEE Advanced Marks vs Ranks 2025: Understanding Category-wise Qualifying Marks and Previous Year Cut-offs

Degree of Dissociation and Its Formula With Solved Example for JEE

Electric field due to uniformly charged sphere class 12 physics JEE_Main

Dual Nature of Radiation and Matter Class 12 Notes: CBSE Physics Chapter 11

Formula for number of images formed by two plane mirrors class 12 physics JEE_Main

A conducting loop carrying a current is placed in a class 12 physics JEE_Main




