Question

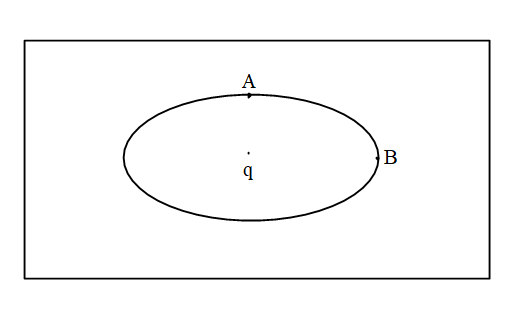
An ellipsoidal cavity is carved within a perfect conductor. A positive charge q is placed at the centre of the cavity. The points A and B are on the cavity surfaces as shown in the figure. Then:

A) Electric field near A in the cavity = Electric field near B in the cavity.
B) Charge density at A= charge density at B.
C) Potential at A=potential at B.
D) Total electric flux through the surface of the cavity is $\dfrac{q}{{{\varepsilon _o}}}$.

Answer 1

Hint: The electric field is defined as the force per unit charge. Equipotential surfaces are defined as those surfaces which are having the same value of potential at every point on their surface. A cavity is a hollow volume inside a conductor.

Formula Used: The formula of the Gauss’s law is given by,
$\phi = \dfrac{{{q_{enclosed}}}}{{{\varepsilon _o}}} = \dfrac{q}{{{\varepsilon _o}}}$
Where $\phi $ is the electric flux through a closed surface of any volume, ${q_{enclosed}}$ is the total charge enclosed in the within the volume and ${\varepsilon _o}$ is the electric constant.

Complete step by step answer:
It is given in the problem that there is an ellipsoidal cavity carved within a perfect conductor and a positive charge q is placed at the centre of the cavity and we need to tell which option is best suited for this problem.
Let us first calculate the value of the flux which flows through the conductor due to the presence of the charge.
The formula of the Gauss’s law is given by,
$\phi = \dfrac{{{q_{enclosed}}}}{{{\varepsilon _o}}} = \dfrac{q}{{{\varepsilon _o}}}$
Where $\phi $ is the electric flux through a closed surface of any volume, ${q_{enclosed}}$ is the total charge enclosed in the within the volume and ${\varepsilon _o}$ is the electric constant.
The electric flux that flows through the surface of the conductor is equal to $\phi = \dfrac{q}{{{\varepsilon _o}}}$.
The conductor carrying the charge is always an equipotential surface also the points A and B are at the surface of the conductor which means that the potential of points A and B are equal.
Since the electric flux through the surface of the conductor is $\phi = \dfrac{q}{{{\varepsilon _o}}}$ and also the potential of points A and points B are equal.
Therefore the correct option for this problem is option C and D.

Note: While solving problems related to flux use Gauss’s law. Students are advised to understand and remember the formula of Gauss’s law as it can help in solving these kinds of problems. The charge inside the cavity decides whether the flux will in inwards or outwards if the charge inside the cavity is unit positive charge then the flux will be outwards from the cavity of the conductor but if the charge is unit negative charge then the flus will be inward moving towards the cavity of the conductor.