
At the first minimum adjacent to the central maximum of a single-slit diffraction pattern the phase difference between the Huygens wavelet from the edge of the slit and the wavelet from the mid-point of the slit is
\[\left( a \right)\]$\dfrac{\pi }{8}radian$
\[\left( b \right)\]$\dfrac{\pi }{4}radian$
\[\left( c \right)\]$\dfrac{\pi }{2}radian$
\[\left( d \right)\]$\pi {\text{ }}radian$
Answer
125.1k+ views
Hint: When we see in single slit diffraction, a line perpendicular to the slit gets superadded. But when we see in double-slit diffraction, the light gets refracted once passing through the slits, to produce an interference pattern on the screen then the light waves commenced from those slits interfere with each other.
Complete step by step Solution:
Suppose there is the screen and there is a single slit placed in front of it. Then there is the distribution of energy on the outlets of the screen. The pattern formed will be in symmetric nature about its axis. Now we have to find the phase difference which should be between the edge of the slit and the wavelet which will be at the midpoint of the slit.
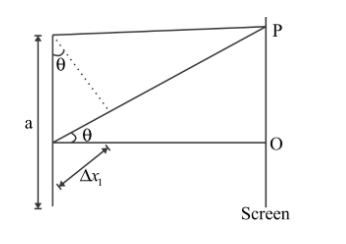
As we already know,
For the first minima at the position P
$ \Rightarrow a\sin \theta = \lambda $
Where $a$will be the length of the slit and $\lambda $will be its wavelength.
So now, phase difference will be calculated and it will be like this
$ \Rightarrow \vartriangle {\lambda _1} = \dfrac{{\vartriangle {x_1}}}{\lambda } \times 2\pi $
As we can see from the figure
$ \Rightarrow \vartriangle {x_1} = \left( {a/2} \right)\sin \theta $
And for first minima in the single-slit diffraction pattern,
$ \Rightarrow a\sin \theta = \lambda $
\[ \Rightarrow \dfrac{a}{2}\sin \theta = \dfrac{\lambda }{2}\]
Now we will put the above value in the $\vartriangle \phi $
$ \Rightarrow \dfrac{{\left( {a/2} \right)\sin \theta }}{\lambda } \times 2\pi $
After solving the above equation, we get
$ \Rightarrow \vartriangle {\phi _1} = \dfrac{\lambda }{{2\lambda }} \times 2\pi $
$ \therefore \pi {\text{ }}radian$
Hence, the option $d$ is the right choice for this question.
Note: Well, single slit diffraction involves sending a beam of light or electrons or alternative objects through one slit, whereas double-slit diffraction involves two slits. With one slim (comparable to the wavelength of the beam) slit, the beam diffracts broadly speaking altogether directions behind the slit and spreads everywhere a downstream viewing screen. With two slim slits, the beams from each slit additionally part generally; however currently the two beams overlap, making an interference pattern at the viewing screen.
Complete step by step Solution:
Suppose there is the screen and there is a single slit placed in front of it. Then there is the distribution of energy on the outlets of the screen. The pattern formed will be in symmetric nature about its axis. Now we have to find the phase difference which should be between the edge of the slit and the wavelet which will be at the midpoint of the slit.

As we already know,
For the first minima at the position P
$ \Rightarrow a\sin \theta = \lambda $
Where $a$will be the length of the slit and $\lambda $will be its wavelength.
So now, phase difference will be calculated and it will be like this
$ \Rightarrow \vartriangle {\lambda _1} = \dfrac{{\vartriangle {x_1}}}{\lambda } \times 2\pi $
As we can see from the figure
$ \Rightarrow \vartriangle {x_1} = \left( {a/2} \right)\sin \theta $
And for first minima in the single-slit diffraction pattern,
$ \Rightarrow a\sin \theta = \lambda $
\[ \Rightarrow \dfrac{a}{2}\sin \theta = \dfrac{\lambda }{2}\]
Now we will put the above value in the $\vartriangle \phi $
$ \Rightarrow \dfrac{{\left( {a/2} \right)\sin \theta }}{\lambda } \times 2\pi $
After solving the above equation, we get
$ \Rightarrow \vartriangle {\phi _1} = \dfrac{\lambda }{{2\lambda }} \times 2\pi $
$ \therefore \pi {\text{ }}radian$
Hence, the option $d$ is the right choice for this question.
Note: Well, single slit diffraction involves sending a beam of light or electrons or alternative objects through one slit, whereas double-slit diffraction involves two slits. With one slim (comparable to the wavelength of the beam) slit, the beam diffracts broadly speaking altogether directions behind the slit and spreads everywhere a downstream viewing screen. With two slim slits, the beams from each slit additionally part generally; however currently the two beams overlap, making an interference pattern at the viewing screen.
Recently Updated Pages
Difference Between Circuit Switching and Packet Switching

Difference Between Mass and Weight

JEE Main Participating Colleges 2024 - A Complete List of Top Colleges

JEE Main Maths Paper Pattern 2025 – Marking, Sections & Tips

Sign up for JEE Main 2025 Live Classes - Vedantu

JEE Main 2025 Helpline Numbers - Center Contact, Phone Number, Address

Trending doubts
JEE Main 2025 Session 2: Application Form (Out), Exam Dates (Released), Eligibility & More

Class 11 JEE Main Physics Mock Test 2025

JEE Main Exam Marking Scheme: Detailed Breakdown of Marks and Negative Marking

JEE Main 2023 January 24 Shift 2 Question Paper with Answer Keys & Solutions

Learn About Angle Of Deviation In Prism: JEE Main Physics 2025

JEE Main 2025: Conversion of Galvanometer Into Ammeter And Voltmeter in Physics

Other Pages
JEE Advanced Marks vs Ranks 2025: Understanding Category-wise Qualifying Marks and Previous Year Cut-offs

NCERT Solutions for Class 11 Physics Chapter 1 Units and Measurements

NCERT Solutions for Class 11 Physics Chapter 9 Mechanical Properties of Fluids

Units and Measurements Class 11 Notes: CBSE Physics Chapter 1

NCERT Solutions for Class 11 Physics Chapter 2 Motion In A Straight Line

NCERT Solutions for Class 11 Physics Chapter 7 Gravitation




