Question

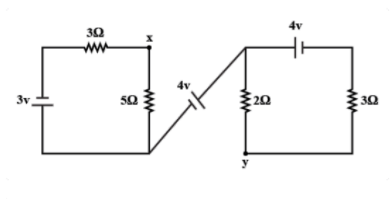
Calculate potential difference between X and Y.

A) $4.275V$
B) $ - 3.725V$
C) $4.527V$
D) $ - 4.527V$

Answer 1

Hint: There are several cells connected in this circuit along with some resistances. We have to determine the terminal potential difference for each cell. Again the emf of cells are also mentioned. We will have to determine the current in the left and right hand side of the middle emf $4V$ in order to determine the potential difference across the resistances.

Complete step by step solution:
Let’s first look at the diagram and determine the direction of the current flowing.
As we know current always flows from the positive terminal of a cell to its negative terminal.
In the left hand side of the diagram, current is flowing in an anti-clockwise direction, and in the right hand side of the circuit, current is flowing anticlockwise too.
The amount of current flowing through the resistors can be found out by applying Ohm’s law.
The mathematical expression for Ohm’s law is,
$V = IR$ ………. (1)
Where $V$ is the potential difference, $I$ is the amount of current, $R$ is the resistance offered by the conductor.
Let's now treat each side of the middle $4V$ emf separately and finally we will take care of the potential difference between points X and Y.
For the left hand side circuit,
Total resistance is $3\Omega + 5\Omega = 8\Omega $ as the resistors are connected in series. We are given that the total emf is $3V$. Substituting these values in the expression for Ohm’s law, we get
$ \Rightarrow 3V = I \times 8\Omega $
$ \Rightarrow I = \dfrac{3}{8}A$
This is the amount of current flowing in the circuit.
Now the potential at point X is
$V = \dfrac{3}{8} \times 5 = \dfrac{{15}}{8}V$ ………. (2)
Now for the right hand side of the circuit,
Total resistance will be \[2\Omega + 3\Omega = 5\Omega \] because these resistors are also connected in series.
Total emf in this circuit is $4V$ .
Substituting these values in the expression for Ohm’s law we get,
$4V = I \times 5\Omega $
$ \Rightarrow I = \dfrac{4}{5}A$
So this amount of current is flowing in the circuit in the right hand side.
So the potential difference at point Y will be,
\[ \Rightarrow V = \dfrac{4}{5} \times 2 = \dfrac{8}{5}V\] ………. (3)
Now, let’s find the potential difference across point X and Y
When we are moving from point X to Y, the potential difference offered by the cell will be $ - 4V$ because we will be moving from negative to positive terminal, because of Kirchhoff’s voltage Law.
Again we are travelling against the flow of current.
So the net potential difference across the points X and Y will be,
$V = \dfrac{{15}}{8} + ( - 4) + ( - \dfrac{8}{5}) = - 5.225V$

So the value of potential difference between points X and Y is $ - 5.225V$.

Note: When applying Kirchhoff’s laws, we need to determine the direction of flow of current first. When travelling against the flow, the voltage has positive value and when along the direction of flow of electric current, the voltage has negative value. A wheat-stone bridge is an application of Kirchhoff’s laws of electrical networks.