




An Introduction to De Broglie Hypothesis
An electron exhibits wave nature under some fixed conditions. Matter waves, Broglie waves, and Schrodinger waves are all terms for waves related to the movement of an electron. De Broglie projected that as light exhibits both wave-like and particle-like properties, matter exhibits wave-like and particle-like properties. This nature was represented as the dual behaviour of matter. On the basis of his observations, Broglie derived a relationship between wavelength and momentum of matter.
De Broglie Principle
The French scientist Louis de Broglie created a bold claim in his doctoral thesis in 1923 (or 1924, counting on the source).
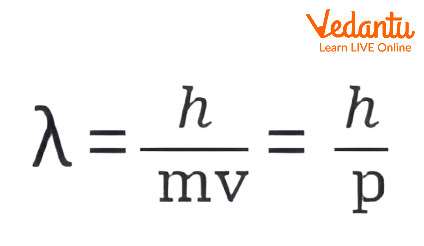
De Broglie Wavelength
According to de Broglie’s theory of matter waves, every particle of matter with linear momentum is also a wave. The quantity of a particle’s linear momentum is inversely proportional to the wavelength of a matter wave related to that particle. This wavelength is known as the de Broglie wavelength. Since it was uncertain whether or not energy should be total energy, kinetic energy, or total relativistic energy with matter, he picked the momentum equation over the energy equation.
De Broglie Principle Equation
Louis de Broglie suggested the non-particle behaviour of matter in 1923. He proposed in his Ph.D. thesis that particles contain wave-like qualities also. By using a series of substitutions de Broglie hypothesised that the particles are holding the properties of waves.
He generated an equation to verify it using Einstein’s renowned mass-energy relation and also Planck’s equation, despite not having the means to test it at the time.
$\lambda \text{=}\frac{h}{mv}=\frac{h}{p}$
Here, 𝜆 is the wavelength, h is Planck's constant, p is momentum, m is mass and v is velocity.
Derivation of De Broglie Wavelength
De Broglie came across his equation through a sequence of substitutions based on well-known theories. He was the first to use Einstein’s famous equation for the relationship between matter and energy:
$E=m{{c}^{2}}$
Here, E is the energy, m is mass and c is the speed of light.
Using Planck’s theory, each quantum of a wave contains a discrete amount of energy that may be calculated using Planck’s equation:
$E=h\upsilon$
Here, h is Planck's constant and 𝝼 is the frequency.
De Broglie hypothesised that the 2 energies would be equivalent as a result of particles and waves sharing the same characteristics:
$m{{c}^{2}}=h\upsilon $
De Broglie proposed velocity (v) for the speed of light since real particles don't travel at the speed of light ( c ).
$m{{v}^{2}}=h\upsilon $
From the above equation, de Broglie replaced v/λ for v in the equation and came across the final expression that connects wavelength and particle speed.
$m{{v}^{2}}=\frac{hv}{\lambda }$
Hence, comparing the above equations we have a tendency to get,
$\lambda =\frac{hv}{m{{v}^{2}}}=\frac{h}{mv}$
Significance of De Broglie Hypothesis
The de Broglie hypothesis demonstrated that wave-particle duality was a fundamental principle shared by both radiation and matter, not just an aberrant behaviour of light. As a result, if the de Broglie wavelength is correctly applied, wave equations may be used to describe the material behaviour. This could be essential for quantum physics to advance. It's currently an accepted part of atomic structure and physics theory.
Though de Broglie’s hypothesis predicts wavelengths for any quantity of matter, there are practical limitations on when it may be used. The wave characteristics of a macroscopic item are so small that they're unobservable in any practical sense, however, they're fascinating to contemplate.
When you think about a table, you imagine it as a solid, stationary object with a particular space. This is often true at the macroscopic level. However, as we zoom in closer to the subatomic level, things become a lot convoluted, and matter doesn't always behave as we expect.
Interesting Facts
In his 1924 Ph.D. thesis, de Broglie postulated the wave nature of electrons and suggested that each matter has wave properties. This idea is eventually called wave-particle duality or the de Broglie hypothesis.
He won the Nobel Prize for Physics in 1929.
Important Question
1. Why is de Broglie wavelength not applicable to a moving football?
Ans. De Broglie wavelength is related to particles showing wave nature like an electron, proton, etc.
Football doesn't exhibit any wave nature and there'll not be any interference and diffraction phenomenon with moving the football.
Hence, de Broglie wavelength isn't applicable to football.
2. Calculate the de Broglie wavelength associated with the electron moving at a speed $2\times {{10}^{5}}m{{s}^{-1}}$. Given: $h=6.625\times {{10}^{-34}}JS$ and ${{m}_{e}}=9.11\times {{10}^{-31}}kg$.
Ans. We know,
$\lambda =\dfrac{h}{p} = \dfrac{h}{m_e v}\\$
$\because p=mv \\$
$\lambda =\dfrac{6.625\times {{10}^{-34}}JS}{9.11\times {{10}^{-31}}kg \times 2 \times {{10}^{5}}m{{s}^{-1}}} \\$
$\lambda =3.636\times {{10}^{-9}}m. \\$
Where h is Planck’s constant; m is the mass of an electron and v is the velocity.
Conclusion
According to the de Broglie hypothesis, a moving material particle behaves like a wave sometimes and like a particle at alternative times. Each moving material particle is connected with a wave. To examine the wave nature of matter particles practically, the de Broglie wavelength should be on the order of the particle size.
FAQs on De Broglie Relationship
1. What is the uncertainty principle?
Heisenberg's uncertainty principle states that there's inherent uncertainty within the act of measurement of a variable of a particle. Usually applied to the position and momentum of a particle, the principle states that the more precisely the position is known the more uncertain the momentum is and vice versa. The formula for the Heisenberg uncertainty principle is: $\Delta p.\Delta x\ge \frac{h}{4\pi }$, where Δ refers to the uncertainty in that variable and h is Planck's constant. If one is aware of the precise momentum of the particle, it's not possible to understand the precise position and vice versa.
2. Does de Broglie wavelength depend on temperature?
The de Broglie wavelength of a molecule (in a sample of an ideal gas) varies inversely as the square root of absolute temperature. The de Broglie wavelength of a molecule (in a sample of an ideal gas) depends on temperature. The Broglie wavelength related to the uncharged particle is: (a) for neutrons: $\lambda =\frac{h}{{{m}_{n}}v}=\frac{h}{\sqrt{2mE}}$ where E is energy. b) For thermal neutrons at normal temperature: E=kT; where T is temperature $\lambda =\frac{h}{\sqrt{2mkT}}$ . (c) For gas molecules; $\lambda =\frac{h}{\sqrt{3mkT}}$ where T is the temperature, m is mass and h is Planck’s constant.
3. What is the consequence of Heisenberg's uncertainty principle?
The Heisenberg Principle greatly impacts practised science and how experiments are designed. To create a measurement, interaction with the particle should occur that may alter its different variables. This can impact a number of the second particle's momentum onto the measured electron, thereby altering it. More correct measurement of the electron's position would need a particle with a smaller wavelength, and thus be more energetic, on the other hand, this would alter the momentum even more during a collision. Consequently, experiments will only gather info about a single variable at a time with any quantity of accuracy.










