
Compare the magnitude of centripetal force and centrifugal force.
Answer
138.6k+ views
Hint According to the third law of motion of Newton, an equal and opposite reaction occurs for any action. The two forces in magnitude and opposite in direction are equivalent. The centrifugal force does not act in motion on the body, the centripetal force is the only force that acts in motion on the body.
Useful formula
Centripetal force,
$F = m{a_c} = m{v^2}r $
Where,
$F $ is the Centripetal force.
\[{a_c}\;\] is the Centripetal acceleration.
$m $ is the mass of the object.
$v $ is the speed or velocity of the object.
$r $ is the radius.
Centrifugal force,
${F_c} = - \dfrac{{m{v^2}}}{r} $
Where,
\[{F_c}\;\]is the Centrifugal force
$m $ is the mass of the object
$v $ is the velocity or speed of the object.
$r $ is the radius.
Complete step by step answer
Given by,
Compare the magnitude of centripetal force and centrifugal force.
As per the concept of the centripetal force,
Centripetal force is the force part that acts in curvilinear motion on an object that is oriented towards the rotation axis or curvature centre.
The Centripetal Force Formula is given squared, divided by the radius, as the product of mass and tangential velocity. That means that the centripetal force can quadruple when the tangential velocity is doubled.
$F = m{a_c} = m{v^2}r $
Centrifugal force:
Centrifugal force is a force that emerges from the inertia of the body and tends to work on a body that moves in a circular direction away from the core around which the body moves.
The formula of the centrifugal force is given as the negative product of square mass and tangential velocity, divided by radius. That means that the centripetal force would be quadrupled upon doubling the tangential velocity.
${F_c} = - \dfrac{{m{v^2}}}{r} $
Here,
Comparison between the centripetal force and centrifugal force:
Centripetal force can be defined as the force variable acting in curvilinear motion on a body that is directed towards the curvature center or rotation axis, while centrifugal force is defined as the apparent force equal to and opposite to the centripetal force, pushing a rotating body away from the center.
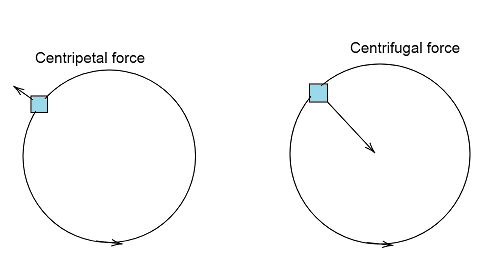
Hence,
Centrifugal force is equal in magnitude and opposite in direction to the centripetal force.
Note Thus, the centripetal force transmitted by the string pulls in on the mass to hold it in its circular direction while twirling a mass on a string, while the centrifugal force transmitted by the string pulls outward at the middle of the path at its point of connection. When released, the centrifugal force is often wrongly thought to cause a body to fly out of its circular direction.
Useful formula
Centripetal force,
$F = m{a_c} = m{v^2}r $
Where,
$F $ is the Centripetal force.
\[{a_c}\;\] is the Centripetal acceleration.
$m $ is the mass of the object.
$v $ is the speed or velocity of the object.
$r $ is the radius.
Centrifugal force,
${F_c} = - \dfrac{{m{v^2}}}{r} $
Where,
\[{F_c}\;\]is the Centrifugal force
$m $ is the mass of the object
$v $ is the velocity or speed of the object.
$r $ is the radius.
Complete step by step answer
Given by,
Compare the magnitude of centripetal force and centrifugal force.
As per the concept of the centripetal force,
Centripetal force is the force part that acts in curvilinear motion on an object that is oriented towards the rotation axis or curvature centre.
The Centripetal Force Formula is given squared, divided by the radius, as the product of mass and tangential velocity. That means that the centripetal force can quadruple when the tangential velocity is doubled.
$F = m{a_c} = m{v^2}r $
Centrifugal force:
Centrifugal force is a force that emerges from the inertia of the body and tends to work on a body that moves in a circular direction away from the core around which the body moves.
The formula of the centrifugal force is given as the negative product of square mass and tangential velocity, divided by radius. That means that the centripetal force would be quadrupled upon doubling the tangential velocity.
${F_c} = - \dfrac{{m{v^2}}}{r} $
Here,
Comparison between the centripetal force and centrifugal force:
Centripetal force can be defined as the force variable acting in curvilinear motion on a body that is directed towards the curvature center or rotation axis, while centrifugal force is defined as the apparent force equal to and opposite to the centripetal force, pushing a rotating body away from the center.

Hence,
Centrifugal force is equal in magnitude and opposite in direction to the centripetal force.
Note Thus, the centripetal force transmitted by the string pulls in on the mass to hold it in its circular direction while twirling a mass on a string, while the centrifugal force transmitted by the string pulls outward at the middle of the path at its point of connection. When released, the centrifugal force is often wrongly thought to cause a body to fly out of its circular direction.
Recently Updated Pages
How to find Oxidation Number - Important Concepts for JEE

How Electromagnetic Waves are Formed - Important Concepts for JEE

Electrical Resistance - Important Concepts and Tips for JEE

Average Atomic Mass - Important Concepts and Tips for JEE

Chemical Equation - Important Concepts and Tips for JEE

Concept of CP and CV of Gas - Important Concepts and Tips for JEE

Trending doubts
JEE Main 2025 Session 2: Application Form (Out), Exam Dates (Released), Eligibility, & More

JEE Main 2025: Derivation of Equation of Trajectory in Physics

Learn About Angle Of Deviation In Prism: JEE Main Physics 2025

Electric Field Due to Uniformly Charged Ring for JEE Main 2025 - Formula and Derivation

JEE Main 2025: Conversion of Galvanometer Into Ammeter And Voltmeter in Physics

Degree of Dissociation and Its Formula With Solved Example for JEE

Other Pages
Units and Measurements Class 11 Notes: CBSE Physics Chapter 1

JEE Advanced Marks vs Ranks 2025: Understanding Category-wise Qualifying Marks and Previous Year Cut-offs

Motion in a Straight Line Class 11 Notes: CBSE Physics Chapter 2

Important Questions for CBSE Class 11 Physics Chapter 1 - Units and Measurement

NCERT Solutions for Class 11 Physics Chapter 1 Units and Measurements

NCERT Solutions for Class 11 Physics Chapter 2 Motion In A Straight Line




