Question

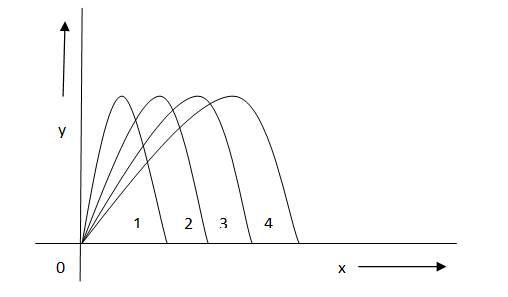
Figure shows four paths for a kicked football. Ignoring the effects of air on the flight, rank the paths according to the initial horizontal velocity component, highest first.

Answer 1

Hint: Kicked football forms a projected motion, therefore we define horizontal range;
Horizontal range of a projectile is the distance from the point of projection to the point where the projectile comes back to the plane of projection.
Formula for horizontal range is:
$R = \dfrac{{{v^2}\sin 2\theta }}{g}$ (v is the velocity, g is the gravitational acceleration, $\theta $ is the angle at which the object is projected) which is directly proportional to the velocity of the object being projected.

Complete step by step solution:
Let us define Horizontal range and horizontal component of velocity in detail.
It is the total horizontal distance from the point of projection to the point where the projectile comes back to the plane of projection. It is denoted by R;
In order to calculate horizontal range R, we shall consider horizontal motion of the projectile. The horizontal motion is uniform. It takes place with constant velocity of horizontal component $v\cos \theta $.
$R = v\cos \theta \times $ time of flight
where time of flight is given by;
$T = \dfrac{{2u\sin \theta }}{g}$
Therefore horizontal range is given by:
$R = v\cos \theta \times \dfrac{{2v\sin \theta }}{g}$.....................1
Component of horizontal velocity: Horizontal component of the velocity is the component of the velocity at which the velocity makes angle of projection it is given as;
$v\cos \theta $, which is directly proportional to horizontal range.
In the figure of the question, the fourth path of the kicked football has the maximum range, thus the fourth path of the football has the highest component of the horizontal velocity, then third, second and first.

Note: Examples of the objects of which shows the projectile motion are: a bomb released from a level flight, a bullet fired from a gun, an arrow released from bow, a javelin thrown by athlete. In all these motions we must neglect the resistance made by air and rotation of earth and the effect due to curvature of earth.