Question

Find the equation of the tangent to the ellipse $7{x^2} + 8{y^2} = 100$ at the point $\left( {2, - 3} \right)$.

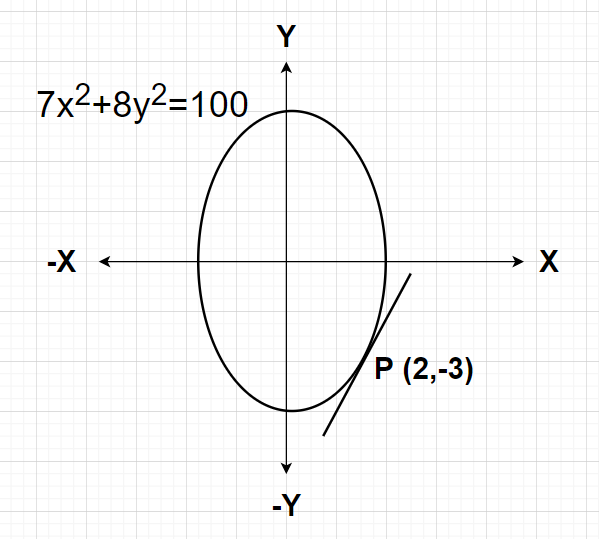
Answer 1

Hint: Here, we will proceed by comparing the given equation of the ellipse with the general equation of any ellipse i.e., $\dfrac{{{x^2}}}{{{a^2}}} + \dfrac{{{y^2}}}{{{b^2}}} = 1$ and then using the general equation of the tangent to the ellipse at any point $\left( {{x_1},{y_1}} \right)$ on the ellipse which is given by $\dfrac{{x{x_1}}}{{{a^2}}} + \dfrac{{y{y_1}}}{{{b^2}}} = 1$.

Complete step-by-step answer:

Given equation of the ellipse is $7{x^2} + 8{y^2} = 100{\text{ }} \to {\text{(1)}}$
Dividing both sides of the equation (1) by 100, we get
$
   \Rightarrow \dfrac{{7{x^2} + 8{y^2}}}{{100}} = \dfrac{{100{\text{ }}}}{{100}} \\
   \Rightarrow \dfrac{{7{x^2}}}{{100}} + \dfrac{{8{y^2}}}{{100}} = 1 \\
   \Rightarrow \dfrac{{{x^2}}}{{\left( {\dfrac{{100}}{7}} \right)}} + \dfrac{{{y^2}}}{{\left( {\dfrac{{100}}{8}} \right)}} = 1 \\
   \Rightarrow \dfrac{{{x^2}}}{{{{\left( {\dfrac{{10}}{{\sqrt 7 }}} \right)}^2}}} + \dfrac{{{y^2}}}{{{{\left( {\dfrac{{10}}{{2\sqrt 2 }}} \right)}^2}}} = 1{\text{ }} \to {\text{(2)}} \\
 $
As we know that the general equation of any ellipse is given by $\dfrac{{{x^2}}}{{{a^2}}} + \dfrac{{{y^2}}}{{{b^2}}} = 1{\text{ }} \to {\text{(3)}}$
By comparing the given equation of the ellipse i.e., equation (2) with the general equation of any ellipse i.e., equation (3), we get
$a = \dfrac{{10}}{{\sqrt 7 }}$ and $b = \dfrac{{10}}{{2\sqrt 2 }}$
For any ellipse $\dfrac{{{x^2}}}{{{a^2}}} + \dfrac{{{y^2}}}{{{b^2}}} = 1$, the equation of the tangent at any point $\left( {{x_1},{y_1}} \right)$ on the ellipse is given by $\dfrac{{x{x_1}}}{{{a^2}}} + \dfrac{{y{y_1}}}{{{b^2}}} = 1{\text{ }} \to {\text{(4)}}$
Since, the point on the given ellipse at which the tangent is to be drawn is $\left( {2, - 3} \right)$ so ${x_1} = 2$ and ${y_1} = - 3$.
By substituting all the values $a = \dfrac{{10}}{{\sqrt 7 }}$, $b = \dfrac{{10}}{{2\sqrt 2 }}$, ${x_1} = 2$ and ${y_1} = - 3$ in the formula given by equation (4), we will get the required equation of the tangent
$
   \Rightarrow \dfrac{{x\left( 2 \right)}}{{{{\left( {\dfrac{{10}}{{\sqrt 7 }}} \right)}^2}}} + \dfrac{{y\left( { - 3} \right)}}{{{{\left( {\dfrac{{10}}{{2\sqrt 2 }}} \right)}^2}}} = 1 \\
   \Rightarrow \dfrac{{2x}}{{\left( {\dfrac{{100}}{7}} \right)}} - \dfrac{{3y}}{{\left( {\dfrac{{100}}{8}} \right)}} = 1 \\
   \Rightarrow \dfrac{{14x}}{{100}} - \dfrac{{24y}}{{100}} = 1 \\
   \Rightarrow \dfrac{{14x - 24y}}{{100}} = 1 \\
   \Rightarrow 14x - 24y = 100 \\
   \Rightarrow 7x - 12y = 50 \\
 $
Therefore, $7x - 12y = 50$ is the required equation of the tangent to the ellipse $7{x^2} + 8{y^2} = 100$ at the point $\left( {2, - 3} \right)$.

Note: In this particular problem, the given point $\left( {2, - 3} \right)$ is on the ellipse because x=2 and y=-3 is satisfying the given equation of the ellipse i.e., $7{x^2} + 8{y^2} = 100$. In the given ellipse b>a because $\dfrac{{10}}{{2\sqrt 2 }} = \dfrac{{10}}{{\sqrt 8 }}$ is greater than $\dfrac{{10}}{{\sqrt 7 }}$ which represents the major axis of the given ellipse is towards y axis and the minor axis is towards x axis as shown in the figure.