
If equation of sound wave is $y = 0.0015\sin (62.4x + 316t)$ , then its wavelength will be
(A) 2 unit
(B) 0.3unit
(C) 0.1unit
(D) 0.2 unit
Answer
138.9k+ views
Hint: The given equation takes the exact form of the progressive wave equation$y = A\sin (\omega t - kx)$. So, on comparing both the wave functions, find out the value of the propagation constant k. k is related to the wavelength by $k = \dfrac{{2\pi }}{\lambda }$ . Now on substituting the values calculate the wavelength of the wave.
Complete step-by-step answer
A mathematical description of the disturbance created by a wave is called a wave function.
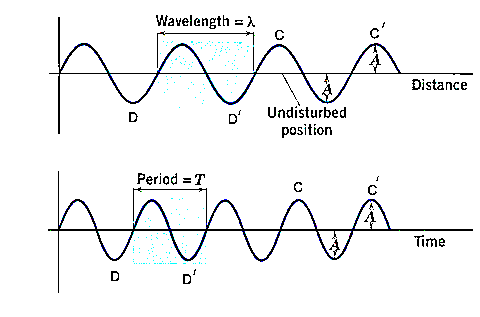
We know that, a progressive wave function is given by,
$y = A\sin (\omega t - kx)$
Where,
Y is the displacement; A is the amplitude; \[\omega \]is the angular frequency; x is the position; k is the propagation constant and t is the time.
$y = 0.0015\sin (62.4x + 316t)$
The given equation is similar to this wave function and therefore has corresponding values. On comparing with the progressive wave function we find out the value of the propagation constant also known as angular wave number.
$k = 62.8$
The number of wavelengths in the distance\[2\pi \]is called the wave number which is given by,
$k = \dfrac{{2\pi }}{\lambda }$
Substitute the value of the angular wave number.
$\lambda = \dfrac{{2 \times 3.14}}{{62.8}}$
$\lambda = 0.1unit$
Hence, the wavelength of the wave is 0.1 units and the correct option is C.
Note: If during the propagation of a progressive wave, the particles of the medium perform SHM about their mean position then the wave is known as a harmonic progressive wave. Various forms of progressive wave function.
$y = A\sin 2\pi \left( {\dfrac{t}{T} - \dfrac{x}{\lambda }} \right)$
$y = A\sin \dfrac{{2\pi }}{T}\left( {t - \dfrac{{xT}}{\lambda }} \right)$
$y = A\sin \dfrac{{2\pi }}{\lambda }\left( {vt - x} \right)$
$y = A\sin \omega \left( {t - \dfrac{x}{v}} \right)$
We can also write it in exponential form
$y = A\dfrac{{{e^{i\omega \left( {t - \dfrac{x}{v}} \right)}} - {e^{ - i\omega \left( {t - \dfrac{x}{v}} \right)}}}}{{2i}}$
Complete step-by-step answer
A mathematical description of the disturbance created by a wave is called a wave function.

We know that, a progressive wave function is given by,
$y = A\sin (\omega t - kx)$
Where,
Y is the displacement; A is the amplitude; \[\omega \]is the angular frequency; x is the position; k is the propagation constant and t is the time.
$y = 0.0015\sin (62.4x + 316t)$
The given equation is similar to this wave function and therefore has corresponding values. On comparing with the progressive wave function we find out the value of the propagation constant also known as angular wave number.
$k = 62.8$
The number of wavelengths in the distance\[2\pi \]is called the wave number which is given by,
$k = \dfrac{{2\pi }}{\lambda }$
Substitute the value of the angular wave number.
$\lambda = \dfrac{{2 \times 3.14}}{{62.8}}$
$\lambda = 0.1unit$
Hence, the wavelength of the wave is 0.1 units and the correct option is C.
Note: If during the propagation of a progressive wave, the particles of the medium perform SHM about their mean position then the wave is known as a harmonic progressive wave. Various forms of progressive wave function.
$y = A\sin 2\pi \left( {\dfrac{t}{T} - \dfrac{x}{\lambda }} \right)$
$y = A\sin \dfrac{{2\pi }}{T}\left( {t - \dfrac{{xT}}{\lambda }} \right)$
$y = A\sin \dfrac{{2\pi }}{\lambda }\left( {vt - x} \right)$
$y = A\sin \omega \left( {t - \dfrac{x}{v}} \right)$
We can also write it in exponential form
$y = A\dfrac{{{e^{i\omega \left( {t - \dfrac{x}{v}} \right)}} - {e^{ - i\omega \left( {t - \dfrac{x}{v}} \right)}}}}{{2i}}$
Recently Updated Pages
How to find Oxidation Number - Important Concepts for JEE

How Electromagnetic Waves are Formed - Important Concepts for JEE

Electrical Resistance - Important Concepts and Tips for JEE

Average Atomic Mass - Important Concepts and Tips for JEE

Chemical Equation - Important Concepts and Tips for JEE

Concept of CP and CV of Gas - Important Concepts and Tips for JEE

Trending doubts
JEE Main 2025 Session 2: Application Form (Out), Exam Dates (Released), Eligibility, & More

JEE Main 2025: Derivation of Equation of Trajectory in Physics

Learn About Angle Of Deviation In Prism: JEE Main Physics 2025

Electric Field Due to Uniformly Charged Ring for JEE Main 2025 - Formula and Derivation

JEE Main 2025: Conversion of Galvanometer Into Ammeter And Voltmeter in Physics

Degree of Dissociation and Its Formula With Solved Example for JEE

Other Pages
Units and Measurements Class 11 Notes: CBSE Physics Chapter 1

JEE Advanced Marks vs Ranks 2025: Understanding Category-wise Qualifying Marks and Previous Year Cut-offs

Motion in a Straight Line Class 11 Notes: CBSE Physics Chapter 2

Important Questions for CBSE Class 11 Physics Chapter 1 - Units and Measurement

NCERT Solutions for Class 11 Physics Chapter 1 Units and Measurements

NCERT Solutions for Class 11 Physics Chapter 2 Motion In A Straight Line




