
If ${w_1}$, ${w_2}$,${w_3}$,${w_4}$ are work done in isothermal, adiabatic, isobaric and isochoric reversible processes, then the correct sequence (for expansion) would be :
(A) ${w_1}$> ${w_2}$>${w_3}$>${w_4}$
(B) ${w_3}$> ${w_2}$>${w_1}$>${w_4}$
(C) ${w_3}$> ${w_2}$>${w_4}$>${w_1}$
(D) ${w_3}$>${w_1}$> ${w_2}$>${w_4}$
Answer
127.8k+ views
Hint: The work done by any thermodynamic process can be given by the area enclosed by the P-V graph by that process. As in the case of isochoric, the volume remains constant. So, no change in volume and hence no work done. So, it will have the least value while in the case of isobaric, the value will be highest.
Complete step by step answer:
First, we will know about the different types of process. What is the difference between these and then we will be able to find out the answer to our question?
The first process is the isothermal process. This may be defined as the process in which thermal meaning temperature is constant. The word ‘iso’ means constant and the word thermal means ‘temperature’. In the isothermal process, the temperature of the system remains constant throughout.
The next process is the adiabatic process. In such a process, The heat and mass is not transformed between the thermodynamic system and its surroundings.
After this, the next process is the isobaric one. The term ‘baric’ is related to pressure. The isobaric process is the one in which the pressure remains constant throughout the process. There may be changes in volume or temperature but the pressure will remain constant.
The last process mentioned is the isochoric one. In this process, the volume of the system remains constant. The term choric stands for the volume.
In the question, it is mentioned that expansion is occurring.
Further, we have that ${w_1}$is the work done during an isothermal process
${w_2}$ is the work done during an adiabatic process
${w_3}$ is the work done during the isobaric process
${w_4}$ is the work done during an isochoric process.
We have the graph for the different processes as-
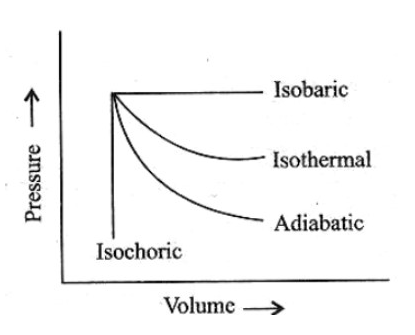
The area under this pressure-volume graph tells us about the work done in any system. If we observe this graph, we can see that
The area under isobaric expansion is the maximum. Thus, the highest work done will be by the isobaric expansion. So, the ${w_3}$will hold the greatest value.
After this, the next maximum area cover is by the isothermal process. So, after this, the ${w_1}$ will be there.
Then after this, comes the area enclosed by the adiabatic process and at last by the isochoric process.
So, the order of work done is as -
${w_3}$>${w_1}$> ${w_2}$>${w_4}$
Thus, the option (D) is the correct answer.
Note: It must be noted that the process is reversible. In any reversible process, the products may go back to the reactant side. The work done by a system is given by PdV. The Pressure-Volume graph is formed as a result of integrating the PdV equation which will give work done.
Complete step by step answer:
First, we will know about the different types of process. What is the difference between these and then we will be able to find out the answer to our question?
The first process is the isothermal process. This may be defined as the process in which thermal meaning temperature is constant. The word ‘iso’ means constant and the word thermal means ‘temperature’. In the isothermal process, the temperature of the system remains constant throughout.
The next process is the adiabatic process. In such a process, The heat and mass is not transformed between the thermodynamic system and its surroundings.
After this, the next process is the isobaric one. The term ‘baric’ is related to pressure. The isobaric process is the one in which the pressure remains constant throughout the process. There may be changes in volume or temperature but the pressure will remain constant.
The last process mentioned is the isochoric one. In this process, the volume of the system remains constant. The term choric stands for the volume.
In the question, it is mentioned that expansion is occurring.
Further, we have that ${w_1}$is the work done during an isothermal process
${w_2}$ is the work done during an adiabatic process
${w_3}$ is the work done during the isobaric process
${w_4}$ is the work done during an isochoric process.
We have the graph for the different processes as-

The area under this pressure-volume graph tells us about the work done in any system. If we observe this graph, we can see that
The area under isobaric expansion is the maximum. Thus, the highest work done will be by the isobaric expansion. So, the ${w_3}$will hold the greatest value.
After this, the next maximum area cover is by the isothermal process. So, after this, the ${w_1}$ will be there.
Then after this, comes the area enclosed by the adiabatic process and at last by the isochoric process.
So, the order of work done is as -
${w_3}$>${w_1}$> ${w_2}$>${w_4}$
Thus, the option (D) is the correct answer.
Note: It must be noted that the process is reversible. In any reversible process, the products may go back to the reactant side. The work done by a system is given by PdV. The Pressure-Volume graph is formed as a result of integrating the PdV equation which will give work done.
Recently Updated Pages
JEE Main 2025 - Session 2 Registration Open | Exam Dates, Answer Key, PDF

Difference Between Vapor and Gas: JEE Main 2024

Area of an Octagon Formula - Explanation, and FAQs

Difference Between Solute and Solvent: JEE Main 2024

Absolute Pressure Formula - Explanation, and FAQs

Carbon Dioxide Formula - Definition, Uses and FAQs

Trending doubts
JEE Main Login 2045: Step-by-Step Instructions and Details

JEE Main Exam Marking Scheme: Detailed Breakdown of Marks and Negative Marking

JEE Main 2023 January 24 Shift 2 Question Paper with Answer Keys & Solutions

JEE Main Participating Colleges 2024 - A Complete List of Top Colleges

JEE Main Course 2025: Get All the Relevant Details

Total number of lone pair electrons in I3 ion is A class 11 chemistry JEE_Main

Other Pages
JEE Advanced 2025: Dates, Registration, Syllabus, Eligibility Criteria and More

JEE Main Chemistry Question Paper with Answer Keys and Solutions

Learn About Angle Of Deviation In Prism: JEE Main Physics 2025

Degree of Dissociation and Its Formula With Solved Example for JEE

Physics Average Value and RMS Value JEE Main 2025

What is the significance of the Heisenberg uncertainty class 11 chemistry JEE_Main




