
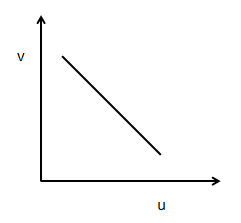
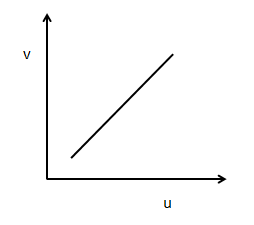
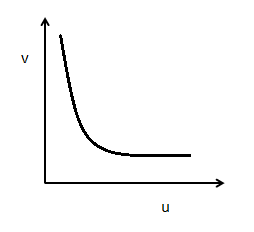
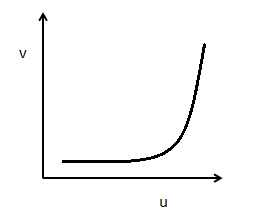
In an experiment to find the focal length of a concave mirror, a graph is drawn between the magnitudes of $u$ and $v$. The graph looks like:
A)
B)
C)
D)

Answer
146.7k+ views
Hint: The image formed is real and inverted when the object is placed in front of a concave mirror and beyond the principal focus of the mirror. In the case of graphs, initially finding the relation between slope, image distance, object distance. to find the nature of the curve.
Complete step by step answer:
The relation between the object distance $u$, the image distance $v$ and the focal length $f$ of the mirror is known as mirror formula. It is given by,
$ \Rightarrow \dfrac{1}{f} = \dfrac{1}{u} + \dfrac{1}{v}$
It is valid for the both concave and convex mirrors, whether the image formed is real or virtual.
Mirror equation can also be written as,
$ \Rightarrow \dfrac{1}{u} = \dfrac{1}{f} - \dfrac{1}{v}$……………….(1)
Graph is plotted by taking image distance $v$ along y-axis and object distance $u$along x-axis.
Slope of the given graphs is written as,
$ \Rightarrow slope = \dfrac{{Y - scale}}{{X - scale}}$
In the graph the slope can be written as the image distance divided by the object distance.
$ \Rightarrow slope = \dfrac{{dv}}{{du}}$ …………………(2)
Now lets us consider equation (1), and differentiate that equation we get,
Using quotient rule,
$ \Rightarrow \dfrac{d}{{dx}}\left( {\dfrac{u}{v}} \right) = \dfrac{{v.\dfrac{{du}}{{dx}} - u.\dfrac{{dv}}{{dx}}}}{{{v^2}}}$
By doing the simplifications we get,
$ \Rightarrow \dfrac{{vdu - vdv}}{{{v^2}}}$
Apply this formula, we get
$ \Rightarrow \dfrac{{u.d(1) - 1.du}}{{{u^2}}} = \dfrac{{f.d(1) - 1.df}}{{{f^2}}} - \dfrac{{v.d(1) - 1.dv}}{{{v^2}}}$
Differentiation of constants gives us zero.
After solving we get,
$ \Rightarrow \dfrac{{ - 1}}{{{u^2}}}du = 0 - \dfrac{{ - 1}}{{{v^2}}}dv$
Then,
$ \Rightarrow \dfrac{{ - 1}}{{{u^2}}}du = \dfrac{1}{{{v^2}}}dv$
Rearranging the above equation, we get,
$ \Rightarrow \dfrac{{dv}}{{du}} = - \dfrac{{{v^2}}}{{{u^2}}}$ …………………(3)
Now comparing equation (2) and (3) we get
$ \Rightarrow slope = - \dfrac{{{v^2}}}{{{u^2}}}$
$\dfrac{{dv}}{{du}}$ is the slope, that is negative. Therefore, either the curve (C) or the curve (A) is right. Since curves (B) and (D) have a positive slope that cannot be done here.
Slope now relies on the $u$ and $v$ values. That is, as per the equation above, it continues to change at any point. So, figure (C) is the correct option.
So, figure (C) is the correct option.
$\therefore $ Correct option is (C).
Note: Focal length of a mirror is the distance between the principal focus and the pole of the mirror. it is denoted by ‘$f$’
Image distance is the distance between the pole of the mirror and image formed. It is denoted by ‘$v$’.
Object distance is the distance between the pole of the mirror and object placed. It is denoted by ‘$u$’.
Complete step by step answer:
The relation between the object distance $u$, the image distance $v$ and the focal length $f$ of the mirror is known as mirror formula. It is given by,
$ \Rightarrow \dfrac{1}{f} = \dfrac{1}{u} + \dfrac{1}{v}$
It is valid for the both concave and convex mirrors, whether the image formed is real or virtual.
Mirror equation can also be written as,
$ \Rightarrow \dfrac{1}{u} = \dfrac{1}{f} - \dfrac{1}{v}$……………….(1)
Graph is plotted by taking image distance $v$ along y-axis and object distance $u$along x-axis.
Slope of the given graphs is written as,
$ \Rightarrow slope = \dfrac{{Y - scale}}{{X - scale}}$
In the graph the slope can be written as the image distance divided by the object distance.
$ \Rightarrow slope = \dfrac{{dv}}{{du}}$ …………………(2)
Now lets us consider equation (1), and differentiate that equation we get,
Using quotient rule,
$ \Rightarrow \dfrac{d}{{dx}}\left( {\dfrac{u}{v}} \right) = \dfrac{{v.\dfrac{{du}}{{dx}} - u.\dfrac{{dv}}{{dx}}}}{{{v^2}}}$
By doing the simplifications we get,
$ \Rightarrow \dfrac{{vdu - vdv}}{{{v^2}}}$
Apply this formula, we get
$ \Rightarrow \dfrac{{u.d(1) - 1.du}}{{{u^2}}} = \dfrac{{f.d(1) - 1.df}}{{{f^2}}} - \dfrac{{v.d(1) - 1.dv}}{{{v^2}}}$
Differentiation of constants gives us zero.
After solving we get,
$ \Rightarrow \dfrac{{ - 1}}{{{u^2}}}du = 0 - \dfrac{{ - 1}}{{{v^2}}}dv$
Then,
$ \Rightarrow \dfrac{{ - 1}}{{{u^2}}}du = \dfrac{1}{{{v^2}}}dv$
Rearranging the above equation, we get,
$ \Rightarrow \dfrac{{dv}}{{du}} = - \dfrac{{{v^2}}}{{{u^2}}}$ …………………(3)
Now comparing equation (2) and (3) we get
$ \Rightarrow slope = - \dfrac{{{v^2}}}{{{u^2}}}$
$\dfrac{{dv}}{{du}}$ is the slope, that is negative. Therefore, either the curve (C) or the curve (A) is right. Since curves (B) and (D) have a positive slope that cannot be done here.
Slope now relies on the $u$ and $v$ values. That is, as per the equation above, it continues to change at any point. So, figure (C) is the correct option.
So, figure (C) is the correct option.
$\therefore $ Correct option is (C).
Note: Focal length of a mirror is the distance between the principal focus and the pole of the mirror. it is denoted by ‘$f$’
Image distance is the distance between the pole of the mirror and image formed. It is denoted by ‘$v$’.
Object distance is the distance between the pole of the mirror and object placed. It is denoted by ‘$u$’.
Recently Updated Pages
How to find Oxidation Number - Important Concepts for JEE

How Electromagnetic Waves are Formed - Important Concepts for JEE

Electrical Resistance - Important Concepts and Tips for JEE

Average Atomic Mass - Important Concepts and Tips for JEE

Chemical Equation - Important Concepts and Tips for JEE

Concept of CP and CV of Gas - Important Concepts and Tips for JEE

Trending doubts
JEE Main 2025 Session 2: Application Form (Out), Exam Dates (Released), Eligibility, & More

JEE Main Exam Marking Scheme: Detailed Breakdown of Marks and Negative Marking

JEE Main 2025: Derivation of Equation of Trajectory in Physics

Electric Field Due to Uniformly Charged Ring for JEE Main 2025 - Formula and Derivation

JEE Main Participating Colleges 2024 - A Complete List of Top Colleges

Degree of Dissociation and Its Formula With Solved Example for JEE

Other Pages
JEE Advanced Marks vs Ranks 2025: Understanding Category-wise Qualifying Marks and Previous Year Cut-offs

JEE Advanced 2025: Dates, Registration, Syllabus, Eligibility Criteria and More

JEE Advanced Weightage 2025 Chapter-Wise for Physics, Maths and Chemistry

Electric field due to uniformly charged sphere class 12 physics JEE_Main

Learn About Angle Of Deviation In Prism: JEE Main Physics 2025

Ideal and Non-Ideal Solutions Raoult's Law - JEE




