
In an experiment to measure the speed of sound by a resonating air column, a tuning fork of frequency 500Hz is used. The length of the air column is varied by changing the water level in the resonance tube. Two successive resonances are heard at the air column with lengths 50.7 cm and 83.9 cm. Which of the following statements are true?
(This question has multiple answers)
Option A: the speed of sound determined from this experiment is
Option B: the end correction in this experiment is
Option C: wavelength of the sound is 66.4 cm.
Option D: the resonance at 50.7 cm corresponds to the fundamental harmonic.
Answer
138.6k+ views
Hint: When two longitudinal waves of same frequency travel through a medium in the opposite directions standing waves are produced.
Complete solution:
In an organ pipe column the sound behaves as a standing wave. The waves resonate harmonically.
There are two types of organ pipes;
Closed organ pipe: One end of the pipe is closed.
Open organ pipe: Both the ends are open.
In an air column filled with water, the conditions are the same as a closed organ pipe.
Hence, we can treat the situation as a closed organ pipe.
Now for standing waves in closed pipe the condition is given by;
Here l=length of air column, n= 0, 1, 2…
The diagram below will help you understand the situation
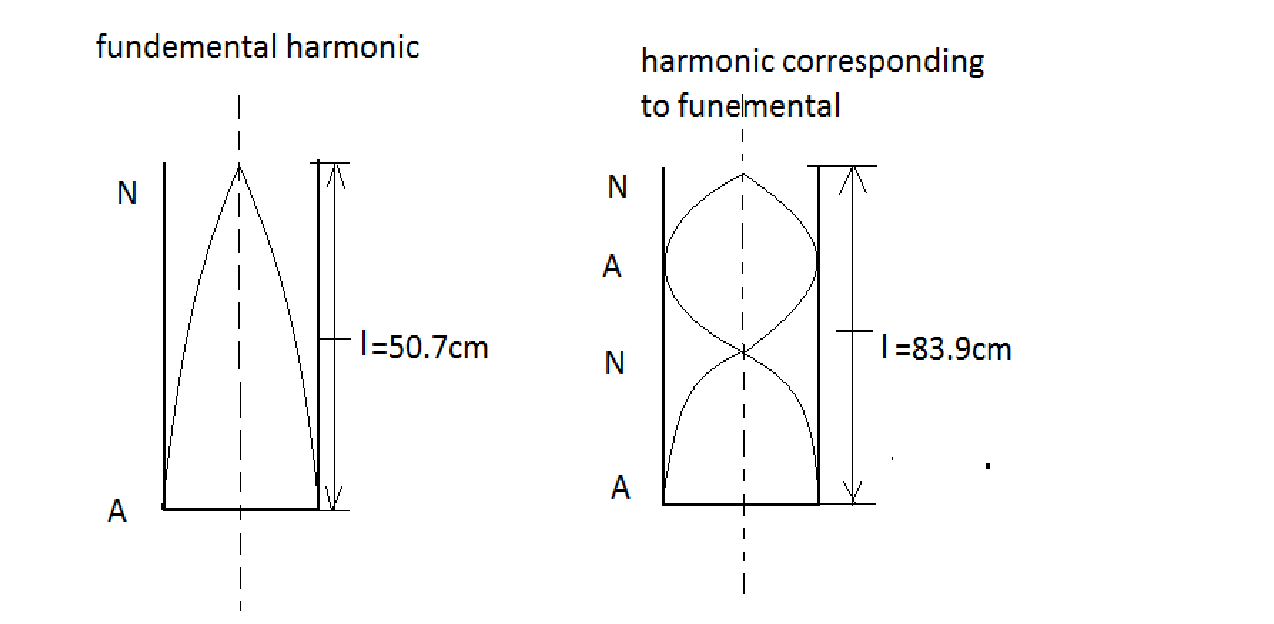
Now the above figure is just an example but not the actual case, as we do not know the number of harmonics.
Now, let us assume that the two successive harmonics at 50.7 and 83.9 cms be
As the difference in length of two successive resonating air columns is half the wavelength of the wave,
Thus,
Hence, wavelength of sound is 66.4cm
Now as
Also, as
50.7 is the third harmonic and so 83.9 will be the fifth harmonic.
Now,
Hence the end correction is 0.9cm.
Now for the speed of sound
Thus option A, option B and option C are correct.
Note:
(1) In the case of air columns, always consider some end correction.
(2) For organ pipe end correction may not be considered.
Complete solution:
In an organ pipe column the sound behaves as a standing wave. The waves resonate harmonically.
There are two types of organ pipes;
Closed organ pipe: One end of the pipe is closed.
Open organ pipe: Both the ends are open.
In an air column filled with water, the conditions are the same as a closed organ pipe.
Hence, we can treat the situation as a closed organ pipe.
Now for standing waves in closed pipe the condition is given by;
Here l=length of air column, n= 0, 1, 2…
The diagram below will help you understand the situation

Now the above figure is just an example but not the actual case, as we do not know the number of harmonics.
Now, let us assume that the two successive harmonics at 50.7 and 83.9 cms be
As the difference in length of two successive resonating air columns is half the wavelength of the wave,
Thus,
Hence, wavelength of sound is 66.4cm
Now as
Also, as
50.7 is the third harmonic and so 83.9 will be the fifth harmonic.
Now,
Hence the end correction is 0.9cm.
Now for the speed of sound
Thus option A, option B and option C are correct.
Note:
(1) In the case of air columns, always consider some end correction.
(2) For organ pipe end correction may not be considered.
Latest Vedantu courses for you
Grade 10 | MAHARASHTRABOARD | SCHOOL | English
Vedantu 10 Maharashtra Pro Lite (2025-26)
School Full course for MAHARASHTRABOARD students
₹31,500 per year
Recently Updated Pages
How to find Oxidation Number - Important Concepts for JEE

How Electromagnetic Waves are Formed - Important Concepts for JEE

Electrical Resistance - Important Concepts and Tips for JEE

Average Atomic Mass - Important Concepts and Tips for JEE

Chemical Equation - Important Concepts and Tips for JEE

Concept of CP and CV of Gas - Important Concepts and Tips for JEE

Trending doubts
JEE Main 2025 Session 2: Application Form (Out), Exam Dates (Released), Eligibility, & More

JEE Main 2025: Derivation of Equation of Trajectory in Physics

Learn About Angle Of Deviation In Prism: JEE Main Physics 2025

Electric Field Due to Uniformly Charged Ring for JEE Main 2025 - Formula and Derivation

JEE Main 2025: Conversion of Galvanometer Into Ammeter And Voltmeter in Physics

Degree of Dissociation and Its Formula With Solved Example for JEE

Other Pages
Units and Measurements Class 11 Notes: CBSE Physics Chapter 1

JEE Advanced Marks vs Ranks 2025: Understanding Category-wise Qualifying Marks and Previous Year Cut-offs

Motion in a Straight Line Class 11 Notes: CBSE Physics Chapter 2

Important Questions for CBSE Class 11 Physics Chapter 1 - Units and Measurement

NCERT Solutions for Class 11 Physics Chapter 1 Units and Measurements

NCERT Solutions for Class 11 Physics Chapter 2 Motion In A Straight Line




