Answer
112.8k+ views
Hint: The ammeters will read the same current. They will read the total current in the circuit. Equivalent circuit will give you the total current.
Complete solution:
Power dissipated from a resistor is given as the product of the square of current flowing through it and the resistance of the resistor.
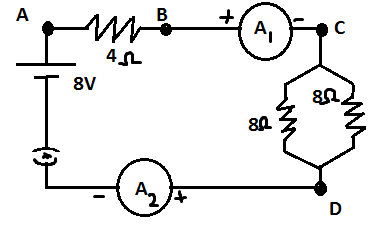
For the given problem, we must first calculate equivalent resistance of the circuit.
Here the two 8 ohms resistors are connected in parallel because the potential drop across the two is the same. Also their starting and ending points are the same as well.
So equivalent of the two 8 ohms resistors is given by;
$R' = \dfrac{{{R_1} \times {R_2}}}{{{R_1} + {R_2}}}$ (resistors in parallel)
Here both the resistors are of 8 ohms;
So, $R' = \dfrac{{8 \times 8}}{{8 + 8}} = \dfrac{{64}}{{16}}$
Hence, $R' = 4\Omega $
Now, the 4 ohms resistor and $R'$ are in series so equivalent resistance of the circuit is given by;
${R_{eq}} = 4 + R' = 4 + 4$
Hence ${R_{eq}} = 8\Omega $
Now with the help of the equivalent resistance we will find the total current in the circuit.
$I = \dfrac{V}{{{R_{eq}}}}$
Hence,$I = \dfrac{8}{8} = 1Amp$, this much current flows through the circuit.
Now this current will flow through the 4 ohms resistor, so, the power dissipated from the 4 ohm resistor is given by;
$P = {I_2}R$
$P = {1^2} \times 4 = 4W$
Hence 4W power will be dissipated from the resistor.
Therefore option A is correct.
Note:
(1) There is one more way of doing this problem, by finding the potential drop across 4 ohms.
(2) The other definition of power is the ratio of square of potential drop across the resistor to the resistance of the resistor.
Complete solution:
Power dissipated from a resistor is given as the product of the square of current flowing through it and the resistance of the resistor.
For the given problem, we must first calculate equivalent resistance of the circuit.
Here the two 8 ohms resistors are connected in parallel because the potential drop across the two is the same. Also their starting and ending points are the same as well.
So equivalent of the two 8 ohms resistors is given by;
$R' = \dfrac{{{R_1} \times {R_2}}}{{{R_1} + {R_2}}}$ (resistors in parallel)
Here both the resistors are of 8 ohms;
So, $R' = \dfrac{{8 \times 8}}{{8 + 8}} = \dfrac{{64}}{{16}}$
Hence, $R' = 4\Omega $
Now, the 4 ohms resistor and $R'$ are in series so equivalent resistance of the circuit is given by;
${R_{eq}} = 4 + R' = 4 + 4$
Hence ${R_{eq}} = 8\Omega $
Now with the help of the equivalent resistance we will find the total current in the circuit.
$I = \dfrac{V}{{{R_{eq}}}}$
Hence,$I = \dfrac{8}{8} = 1Amp$, this much current flows through the circuit.
Now this current will flow through the 4 ohms resistor, so, the power dissipated from the 4 ohm resistor is given by;
$P = {I_2}R$
$P = {1^2} \times 4 = 4W$
Hence 4W power will be dissipated from the resistor.
Therefore option A is correct.
Note:
(1) There is one more way of doing this problem, by finding the potential drop across 4 ohms.
(2) The other definition of power is the ratio of square of potential drop across the resistor to the resistance of the resistor.
Recently Updated Pages
Uniform Acceleration - Definition, Equation, Examples, and FAQs

JEE Main 2021 July 25 Shift 2 Question Paper with Answer Key

JEE Main 2021 July 20 Shift 2 Question Paper with Answer Key

JEE Main 2021 July 22 Shift 2 Question Paper with Answer Key

JEE Main 2021 July 25 Shift 1 Question Paper with Answer Key

JEE Main 2023 (January 30th Shift 1) Physics Question Paper with Answer Key

Trending doubts
JEE Main 2025: Application Form (Out), Exam Dates (Released), Eligibility & More

Angle of Deviation in Prism - Important Formula with Solved Problems for JEE

JEE Main Chemistry Question Paper with Answer Keys and Solutions

Average and RMS Value for JEE Main

JEE Main 2025: Conversion of Galvanometer Into Ammeter And Voltmeter in Physics

Inductive Effect and Acidic Strength - Types, Relation and Applications for JEE

Other Pages
JEE Advanced Marks vs Ranks 2025: Understanding Category-wise Qualifying Marks and Previous Year Cut-offs

Dual Nature of Radiation and Matter Class 12 Notes CBSE Physics Chapter 11 (Free PDF Download)

Displacement-Time Graph and Velocity-Time Graph for JEE

Degree of Dissociation and Its Formula With Solved Example for JEE

Diffraction of Light - Young’s Single Slit Experiment

JEE Main 2025: Derivation of Equation of Trajectory in Physics