Maximum and Minimum Values of Trigonometric Functions for JEE

Trigonometric functions are the functions defined on the basis of the angles of a triangle. These functions describe the relationship established between the sides and angles of a triangle. The basic trigonometric functions are sin, cosine, tangent, secant, cosecant, and cotangent. Various trigonometric identities emerge from these basic trigonometric functions and are formed with the help of the relationship of compound angles of the triangles. 

As the trigonometric functions are cyclic, all trigonometric functions are defined in certain intervals, i.e., each of them has its respective domain and range. According to their domain and range, the maximum and minimum values of these trigonometric functions were predetermined. This article also answers questions like how to find maximum and minimum values of a trigonometric function. It also determines the maximum and minimum value of the quadratic equation formula.

What are Mathematical Functions?

Mathematical functions are those functions that establish the relationship between the one independent variable and the dependent variable. These functions are used to describe the relationship which is used for formulating the physical relationship of practical application and procuring their solution. There are various types of mathematical functions such as polynomial function, trigonometric function, exponential function, and complex function.

Basics of Trigonometric Function

As discussed in the first section, the trigonometric function is the cyclic function that is used to describe the relationship between the sides and angles of the triangle. The basic trigonometric functions are defined with the help of a right-angled triangle in the reference to angle A.

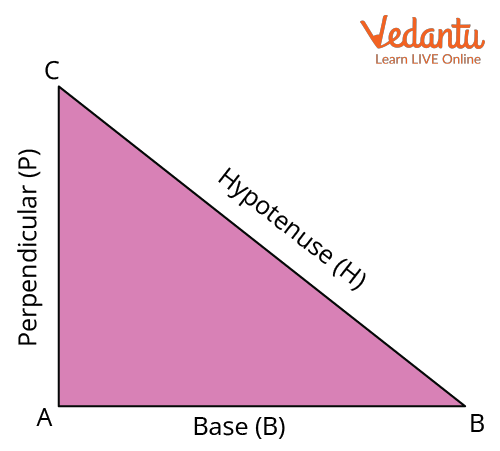
Image : Right-Angled Triangle ABC

There are various basic trigonometric functions which are defined below:

• Sin 

The sin function of an angle is the ratio of the perpendicular to the hypotenuse of the right-angled triangle. The formula for the sin function of angle A is shown below:

$Sin~{C}=\dfrac{\text{perpendicular}}{\text{Hypotenuse}}$

• Cosine

The cosine function of an angle is the ratio of the base to the hypotenuse of the right-angled triangle. The formula for the cosine function of angle A is shown below:

$Cosine~{C}=\dfrac{\text{Base}}{\text{Hypotenuse}}$

• Tangent

The tangent function of an angle is the ratio of the perpendicular to the base of the right-angled triangle. The formula for the tangent function of angle A is shown below: 

$Tangent{C}=\dfrac{\text{Perpendicular}}{\text{Base}}$

• Cotangent

The cotangent function of an angle is the ratio of the base to the perpendicular of the right-angled triangle. It is also known as the reciprocal of the tangent function. The formula for the cotangent function of angle A is shown below:

$Cotangent~{C}=\dfrac{\text{Base}}{\text{Perpendicular}}$

• Secant

The secant function of an angle is the ratio of the hypotenuse to the base of the right-angled triangle. It is the reciprocal of the cosine function. The formula for the secant function of angle A is shown below: 

$Secant~C=\dfrac{\text{Hypotenuse}}{\text{Base}}$

• Cosecant

The cosecant function of an angle is the ratio of the hypotenuse to the perpendicular of the right-angled triangle. It is the reciprocal of a sine function. The formula for the cosecant function of angle A is shown below:

$Cosecant~C=\dfrac{\text{Hypotenuse}}{\text{Perpendicular}}$

Determination of Maximum and Minimum Value of Trigonometric Functions

The maximum and minimum values of the basic trigonometric functions depend upon the domain and range of the respective trigonometric functions. The domain belongs to the angles of the trigonometric functions and the range of the trigonometric functions belongs to the values of the trigonometric function on those angles. The maximum and minimum values of trigonometric functions depend upon the range of the trigonometric functions. The maximum and minimum values of the trigonometric function are given below:

Quadratic Equation

A quadratic equation is the equation of a second-degree polynomial where it has a minimum of one term which is a higher degree 2. It is one of the types of a polynomial function. In this quadratic equation, the constant ‘a’ cannot be zero. The general form of the quadratic equation is given below:

ax2+bx+c

In the above equation, the equation with one variable having a higher degree 2 is x and a, b, and c are constants. Various methods used for solving the quadratic equation are factorization, using square roots, discriminant formula, and completing the square.

Maximum and Minimum Value of Quadratic Equation Formula

The maximum and minimum values of the quadratic equation will be determined with the help of the quadratic formula which is given below: 

$D=b^2-4ac$

For determining the maximum and minimum value of the quadratic formula, the equation is given as:

$\begin{align} &y=a x^{2}+b x+c \\ &a x^{2}+b x+c-y=0 \end{align}$

For finding the zeros of the above equation by discriminant formula:

$\begin{align} &b^{2}-4 a(c-y) \geq 0 \\ &b^{2}-4 a c+4 a y \geq 0 \\ &4 a y \geq 4 a c-b^{2} \end{align}$                                 …. (1)

For determining the minimum and maximum values of quadratic equation, the value of constant a keeping greater than zero and less than zero 

When (a > 0), using equation (1),

$\begin{align} &4 a y \geq 4 a c-b^{2} \\ &y \geq 4 a c-\frac{b^{2}}{4 a} \end{align}$

It can be seen that whenever the value of constant a is greater than zero, it will give the minimum value of y. Therefore, the minimum value of the quadratic equation is $4ac-\dfrac{b^2}{4a}$. 

When (a < 0), using equation (1),

$\begin{align} &4 a y \geq 4 a c-b^{2} \\ &y \leq 4 a c-\frac{b^{2}}{4 a} \end{align}$

It can be seen that whenever the value of constant a is smaller than zero, it will give the maximum value of y. Therefore, the maximum value of the quadratic equation is $4ac-\dfrac{b^2}{4a}$. 

Conclusion

This article concludes with an understanding of the trigonometric function and quadratic equation. It gives the maximum and minimum values of the trigonometric function. It also shares the determination of the maximum and minimum values of the quadratic equation formula. The entire article has a better understanding of the theoretical concepts of trigonometry and polynomials. It has also answered queries like how to find the maximum and minimum values of trigonometric functions. It also finds out the maximum and minimum value of the quadratic equation formula.