Acceleration Due to Gravity

Gravity is a term that is used in Physics to describe the mutual attraction between objects which have mass or energy. It is importantly described by the general theory of relativity, which was proposed by Einstein that gravity is not a force but the curvature of space-time which resulted from the non-uniform distribution of mass on the Earth. Earth gravity can be stated as the force by which the Earth attracts other bodies towards its centre. The strength of the gravity field is almost equivalent to the acceleration of all the bodies which are under its influence. 

Newton’s theory of gravitation states that the force which keeps any two bodies in mutual attraction should be directly proportional to the masses of those two bodies and inversely proportional to the square of the distance between them. This is written in mathematical form as 

$F_{12}=F_{21}=\dfrac{Gm_1m_2}{r^2}$

Here, F12 is the force of attraction by the 1st body on the second one. 

F21 is the force of attraction exerted by the 2nd body on the first one.

G is called the gravitational constant. 

m1 and m2 are the masses of body 1 and body 2, respectively, whereas r is the distance between the two bodies. Unit of gravity is $\dfrac{Nm^2}{Kg^2}$.

Now, let us understand what is the acceleration due to gravity g and the variation in g according to gravity. 

Acceleration Due to Gravity in a Nutshell 

If an object gets an acceleration due to the gravitational force acting on it, then that acceleration is named acceleration due to gravity. Its SI units are the same as that of the acceleration, $\dfrac{m}{s^2}$. It is represented by the small letter g. 

Let’s derive the acceleration due to gravity formula by assuming a test mass m and the source mass M. If the test mass is dropped from a height toward the ground, its velocity starts changing and acceleration is produced. The force by which it has got the acceleration is the gravitational force.

Now, according to Newton’s law of gravitation and Newton’s law of motion,

We can equate the forces as shown below. 

$F=ma=\dfrac{G\,m_1\,m_2}{r^2}$ 

Substituting m1 = M and m2 = m, we obtain 

$ma=\dfrac{GMm}{r^2}$

where m on both sides gets cancelled and we get an equation for the acceleration. 

i.e., $a=\dfrac{GM}{r^2}$

It is evident that this acceleration of the object is due to the presence of gravitational force on that object. This is why the acceleration is named acceleration due to gravity (g). To get acceleration due to the gravity value on the surface of the Earth, let’s put in the below values.

The value of $G=6.67\times 10^{-11}\dfrac{Nm^2}{Kg^2}$ and M is the mass of the Earth, whereas r is the radius of the Earth. If we substitute these values in the above-derived equation, we get the value of g as $9.8~\dfrac{m}{s^2}$, which is constant for every object on earth disregarding their mass.  

Note: This value of g gets affected by some of the factors like altitude above the earth, depth below and the motion of the Earth.

Now, let’s have some insights on how the acceleration due to gravity value varies with height and depth. 

Value of g on Earth

We know that formula for the acceleration due to gravity on the surface of the earth is:

\[g = \frac{GM_{e}}{R_{e}^{2}}\].

Where, 

\[G = \text{Universal gravitational constant; whose value is } 6.673 \times 10^{-11} Nm^{2}kg^{-1}\]

\[M_{e}  = 6 \times 10^{24} kg\]

\[R_{e} = 6.4 \times 10^{6}m\]

On putting these values in the above formula, we get the value of g at the surface of the earth as 9.8 ms-2.  However, this value varies with height.

The dimensional formula for g is \[[M^{0}LT^{-2}]\].

Variation in Acceleration Due to Gravity g

Let us assume the test mass at a height ‘h’ from the surface of the Earth. Now, the source mass of the Earth is taken at its centre. So, the distance between the two masses is R+h, not R.  

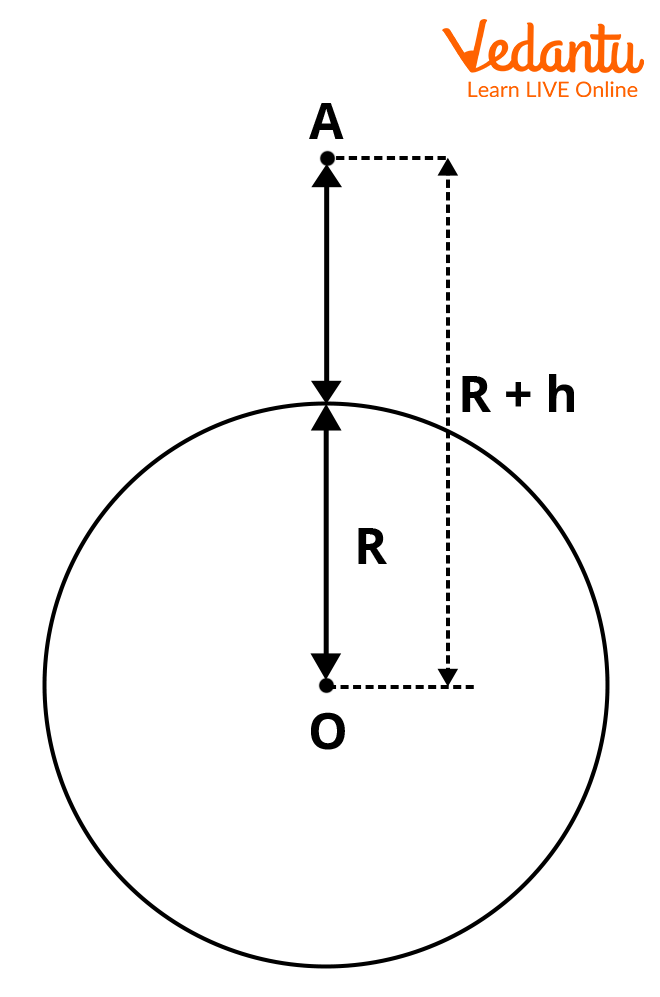
Variation in g due to height

Substituting r = R+h in the gravity formula, we get 

$F=\dfrac{GMm}{(R+h)^2}$  - - - - (1)

This is the equation of force acting on the object due to gravitation and will be equal to the force acting on the object due to acceleration due to gravity at a height h. 

i.e. F = mgh  - - - - (2)

Let’s take the value gh as the acceleration due to gravity at the height ‘h’. 

Thereby equating equation (1) and (2) equations, we get 

$mg_h=\dfrac{GMm}{R^2\left(1+\dfrac{h}{r}\right)^2}$

After the cancellation of m on both sides and the substitution of ‘g’ in place of $\dfrac{GM}{R^2}$

we get the following equation, 

$g_h=\dfrac{g}{\left(1+\dfrac{h}{R}\right)^2}=g\left(1+\dfrac{h}{R}\right)^{-2}$

$g_h=g\left(1-\dfrac{2h}{R}\right)$..............(expanded using Taylor series) 

Here h<<R as the radius of the earth is incomparable to the value of the height, so the next terms of Taylor's series are neglected. 

Similarly, we have a formula if the object is deep below the Earth’s surface. Let us assume an object is at the depth of D from the centre of the Earth. The volume of the enclosed mass in this depth changes as the radius changes. But the density remains constant. 

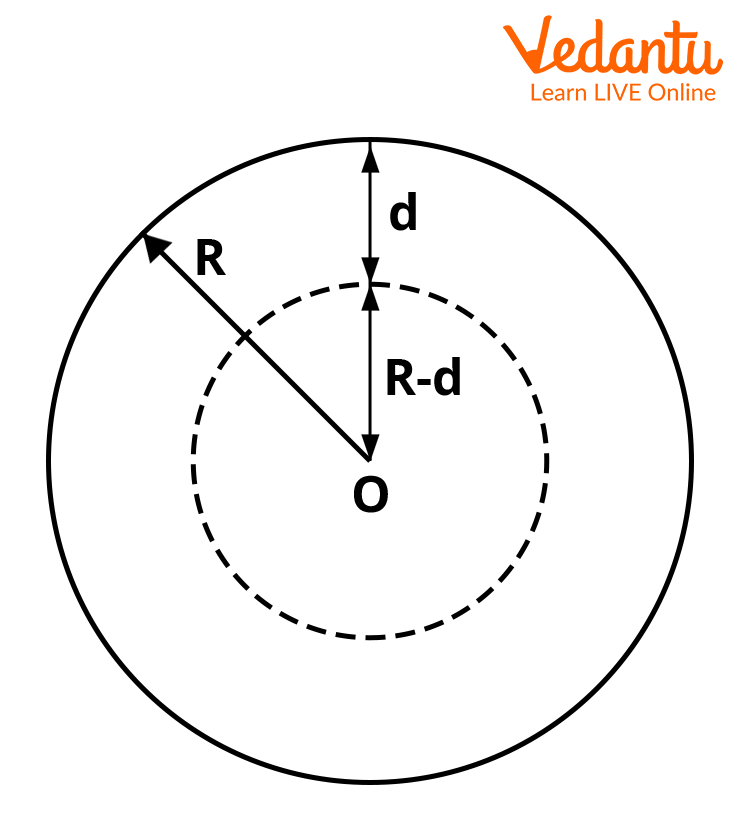
Variation in Gravity due to Depth

Let us take the equation of the density of the object at two locations. 

As we know, the formula of density is given by the equation, 

Density, $\rho=\dfrac{\text { Mass }}{\text { Volume }}$

At radius $R, \rho=\dfrac{M}{\dfrac{4}{3} \pi \mathrm{R}^{3}}$

At radius $R-D, \rho_{d}=\dfrac{M_{d}}{\dfrac{4}{3} \pi(R-D)^{3}}$

Now, $\rho=\rho_{d}$

$\begin{align} &\dfrac{M}{\dfrac{4}{3} \pi \mathrm{R}^{3}}=\dfrac{M_{d}}{\dfrac{4}{3} \pi(R-D)^{3}} \\ &\text { i.e., } M_{d}=M \dfrac{(R-D)^{3}}{R^{3}}=M\left(1-\dfrac{d}{R}\right)^{3} \end{align}$

Here, we got the relation between the masses at radius R and  R – D. 

Let us take the equation of acceleration due to gravity at the radius R – D, we obtain 

$\begin{align}&g_{d}=\dfrac{G M_{d}}{(R-D)^{2}}=\frac{G M_{d}}{R^{2}\left(1-\dfrac{D}{R}\right)^{2}} \\&=>\dfrac{GM\left(1-\dfrac{D}{R}\right)^{3}}{R^{2}\left(1-\dfrac{D}{R}\right)^{2}}=g\left(1-\dfrac{D}{R}\right)\end{align}$

Therefore, we obtained the relation,

$g_d=g\left(1-\dfrac{D}{R}\right)$

Where D is the depth. 

Conclusion 

As we can see in both the cases of acceleration due to gravity formula, when the object is placed at a height above the Earth's surface or at a depth below the Earth's surface, acceleration due to gravity decreases and becomes less than the normal value that is on the surface of the Earth. Hereby, we can infer that the value of acceleration due to gravity gets affected by the height and the depth of the object with respect to the surface of the Earth. Therefore, we learned what is the acceleration due to gravity g and variation in g through this article. 

Acceleration due to gravity is a vector quantity, as it has both magnitude and direction. As we know through the gravity formula that the force of gravitation is inversely proportional to the square of the distance. The distance between the poles and the centre of the Earth is lesser with regard to the distance between the equator and the centre. This results in a higher value of acceleration due to gravity at poles.