Electrochemistry Class 12 NCERT Solutions FREE PDF Download!
FAQs on NCERT Solutions for Class 12 Chemistry Chapter 2 Electrochemistry
1. What is Electrochemistry all About?
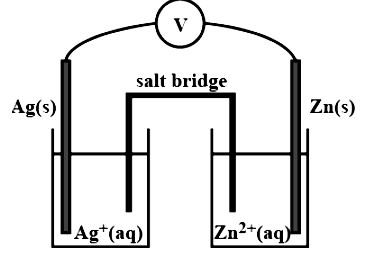
Electrochemistry deals with the relationship between Chemistry and electricity. It focuses on how electricity can be produced from chemical changes and how chemical changes, in turn, produce electricity. It discusses how reactions can take place in a battery between a cathode and an anode with electrolyte in between them.
Electrochemical reactions are that branch of study where any chemical reaction such as in a battery produces an electric current or where the application of electricity produces a chemical reaction. Chemistry class 12 Chapter 2 exercise solutions can be quite challenging for most students.
Students having difficulty with this chapter can refer to Electrochemistry Class 12 NCERT Solutions.
2. How to Prepare for Electrochemistry?
As a common denominator of preparation, just studying the chapter at least three times will suffice. Students can then make notes on the chapter and tackle the exercises. Ch 3 Chemistry Class 12 is quite a tough chapter so students can make use of Electrochemistry Class 12 solutions.
After studying the chapters students can begin their preparation on past year papers and sample papers. These will help students to gain confidence and experience in solving these types of problems.
Another trick of preparing for Electrochemistry is that students should clearly understand when electrical changes give rise to chemical changes and when chemical changes give rise to electrical changes.
3. Where can I Find Solutions to Class 12 Chapter 2 Electrochemistry?
Students can find class 12 electrochemistry NCERT solutions on Vedantu's website. The solutions are presented in a light, clear, and straightforward way. They are easily downloadable in pdf format and they are free of cost. Whenever students have any difficulty with their understanding they can refer to the solutions provided.
class 12 electrochemistry NCERT solutions is not an easy chapter. Students struggle to understand concepts of anode, cathode and electrolyte. They get confused with these topics. Also, conductivity and resistivity are difficult concepts to explain. Students should know that perseverance is the only key to cracking Electrochemistry.
4. What is Molar conductivity according to Chapter 2 of Class 12 Chemistry?
Molar conductivity can be defined as the conducting power of all the ions. It is formed by dissolving one mole of electrolyte in the solution. Molar conductivity is not constant. The efficiency of a given electrolyte in conducting electricity in a solution is known by molar conductivity.
5. What is Kohlrausch’s Law? Explain?
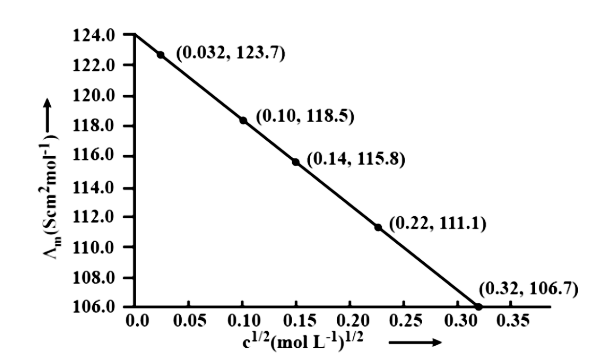
An equivalent conductivity of an electrolyte at an infinite solution is equal to the sum of conductances of anions and cations. This is called Kohlrausch's law. Students can refer to NCERT Solutions to learn the definitions, which are given in a simple and understanding way. Kohlrausch's law of migration of ions helps in calculating any electrolyte of λ o. But for the weak electrolytes, the value can be determined if we know Ëm ° and Λm at C, which is a given concentration.
6. What is electrical potential? How can you define the magnitude of the electrical potential?
The amount of work required to move a unit charge from a reference point to a particular point against the electric field is known as electrical potential. The magnitude of the electrical potential depends on the amount of work done in moving an object from one point to another against the electric field. Whenever there is a movement of the object against the electric field there is a gain of some energy. This is known as electrical potential energy.
7. What is Gibbs free energy?
Gibbs free energy is the quantity used to measure the amount of work done in a thermodynamic system when temperature and pressure are constant. There are many problems in this chapter. Students have to be thorough with the formula to solve the problems. You can also refer to NCERT Solutions and practice formula-based problems to properly understand the topic. Gibbs Law is also a reduced chemical potential when it attains equilibrium. This is also known as free enthalpy, and also it serves as an impulsiveness measure with content variables of temperature and pressure.
8. What is Electronic Conductance?
Electrical conductance through metals is called metallic or electronic conductance which is due to the movement of electrons, which depends on the nature of the structure of the metal, the number of valence electrons per atom and temperature(decrease or increase in temperature). As the electrons enter at one end and go out through the other end, the composition of the metallic conductor remains unchanged. Students can refer to Electrochemistry class 12 exercise solutions to get better practice for exams.
9. What is the main point of class 12 electrochemistry?
Understanding the interplay of chemical reactions and electrical energy forms the core of electrochemistry, as elucidated in class 12 chemistry ch 2 NCERT solutions.
10. What are the applications of electrochemistry according to Class 12 Chemistry Ch 2 NCERT Solutions?
Class 12 Chemistry Ch 2 NCERT Solutions Electrochemistry applications include batteries, corrosion prevention, electrolysis in metal extraction, and fuel cells.
11. How is electrochemistry used in day-to-day life?
Electrochemistry plays a vital role in various everyday applications such as powering electronic devices with batteries, electroplating objects for decoration, and purifying water through electrolysis, as elucidated in class 12 chemistry electrochemistry NCERT solutions.
12. Why is it important to study electrochemistry?
Understanding electrochemistry is crucial for advancements in energy storage, corrosion control, and chemical production. Students can also access Class 12 Chemistry Electrochemistry NCERT Solutions for better understanding.
13. What is a fuel cell in electrochemistry?
A fuel cell is an electrochemical device that converts chemical energy directly into electrical energy, commonly used in vehicles and stationary power plants. Chemistry Class 12 Chapter 2 Exercise Solutions is the best resource for practising and Expertising in Electrochemistry.