Comprehensive Guide to Electrostatics for JEE Main 2025 Preparation
Electrostatics is a crucial chapter in Physics for JEE Main 2025, forming the foundation for understanding electric forces, fields, and potential. This topic explores the behaviour of charges at rest, covering fundamental principles like Coulomb's law, electric field intensity, electric potential, and the concept of capacitance. Electrostatics not only tests your conceptual understanding but also strengthens problem-solving skills critical for competitive exams.
In this guide, we will break down the essential concepts, formulas, and tips to help you master Electrostatics according to the latest JEE Main 2025 Syllabus. This article provides you step-by-step explanations of each concepts and practice problems to boost your preparation.
JEE Main Physics Syllabus JEE Main Physics Revision Notes JEE Main Physics Important Questions JEE Main Physics Difference Between JEE Main Physics Question Papers
Important Topics of Electrostatics Chapter
Coulomb’s Law.
Electric field and electric lines of force.
Electric field due to continuous charge distribution.
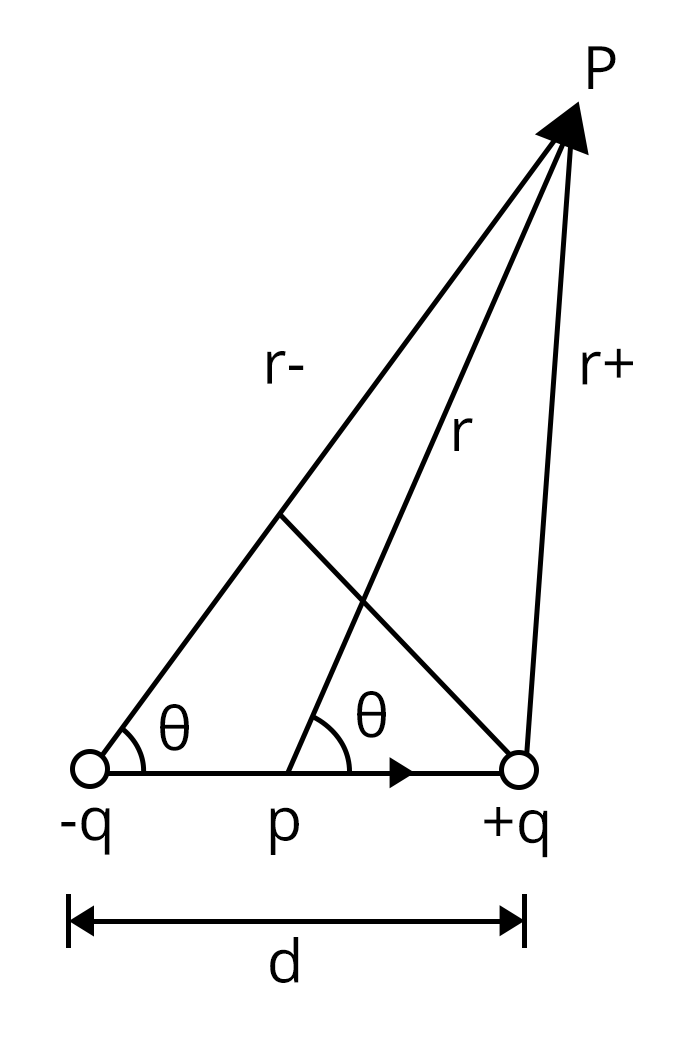
Electric dipole and dipole moment.
Gauss Law.
Electric potential and Equipotential surface.
Electric potential due to the various charge distributions.
Capacitance of a capacitor.
Grouping of Capacitance.
Electrostatics Important Concepts for JEE Main
What is Electrostatic?
Electrostatics is the study of electric charges at rest. It involves the interaction between charged particles and the forces and fields they create. Coulomb's law is a fundamental principle in electrostatics that describes the force between two-point charges.
Characteristics of Electrically Charged Objects
The important characteristics of electrically charged objects are-
Like charges repel which means + repels +, - repels -
Unlike charges attract which means + attracts - and vice versa.
There is no net charge on a neutral object which means the charge is conserved. If the fur and plastic rod were neutral at first. After the rod becomes charged by the fur, the negative charge of the fur will be transferred to the plastic rod. The net negative charge on both fur and rod are equal.
Limitations of Coulomb's Law
Coulomb's law can be applied only for the point charges which are at rest.
Coulomb’s law is applicable only in the cases where the inverse square law is obeyed.
Where the charges are in an arbitrary shape, it is difficult to imply Coulomb's law as it is not possible to determine the location between two objects.
Coulomb’s law cannot be directly used to calculate charge on big planets.
Applications of Gauss's Law:
Field Due to Infinitely Long Uniformly Charged Straight Wire: Using Gauss's law, it can be shown that the electric field (E) due to an infinitely long uniformly charged straight wire is inversely proportional to the distance from the wire. Mathematically, E = λ / (2πε₀r), where λ is the linear charge density and r is the distance from the wire.
Uniformly Charged Infinite Plane Sheet: For a uniformly charged infinite plane sheet, the electric field is uniform and perpendicular to the sheet. The electric field (E) can be calculated as E = σ / (2ε₀), where σ is the surface charge density.
Uniformly Charged Thin Spherical Shell: Within a uniformly charged thin spherical shell, the electric field is zero. This result highlights the power of Gauss's law in establishing the electric field behavior in a symmetric system.
List of Important Formulas for Electrostatics Chapter
JEE Main Electrostatics Solved Examples
1. The electric potential in a region is given by V=(6x-8xy2-8y+6yz-4x2) volt. Then the electric field acting on a point charge of 2C placed at the origin will be …
Ans: Given,
The electric potential in a region , V=(6x-8xy2-8y+6yz-4x2) volt
The electric field in the region can be obtained from the electric potential using the formula
To find the electric field at origin, put x=0, y=0 and z=0 in equation (1)
The force acting on the charge q due to electric E is given by
Key point: Electric field at a point can be obtained by the differentiation of electric potential with respect to distance.
2. A parallel plate capacitor with oil between plates (dielectric constant of oil k=2) has a capacitance C. If the oil is removed, then the capacitance of the capacitor becomes…
Ans: Let A be the area of the capacitor and d be the distance between the plates of the capacitor.
The formula for the capacitance of the parallel plate capacitor with oil as a dielectric is given by,
The capacitance of the parallel plate capacitor when oil is removed is
Divide equation (2) by (1) to obtain the new capacitance C’ when oil is removed in terms of initial capacitance C.
When the oil is removed from the parallel plate capacitor, its capacitance is reduced by a factor of k.
Key point: The capacitance of a parallel plate capacitor depends on the dielectric medium and the dimensions of the capacitor. It does not depend on the charge and potential difference across the capacitor.
Previous Year Questions from JEE Paper
1. Two equal capacitors are first connected in series and then in parallel. The ratio of equivalent capacitances in the two cases will be (JEE 2021)
Sol:
Let the capacitances of each capacitor be C.
In the series connection of two capacitors, the equivalent capacitances is given by

For the capacitors in parallel connection, the equivalent capacitance is given by
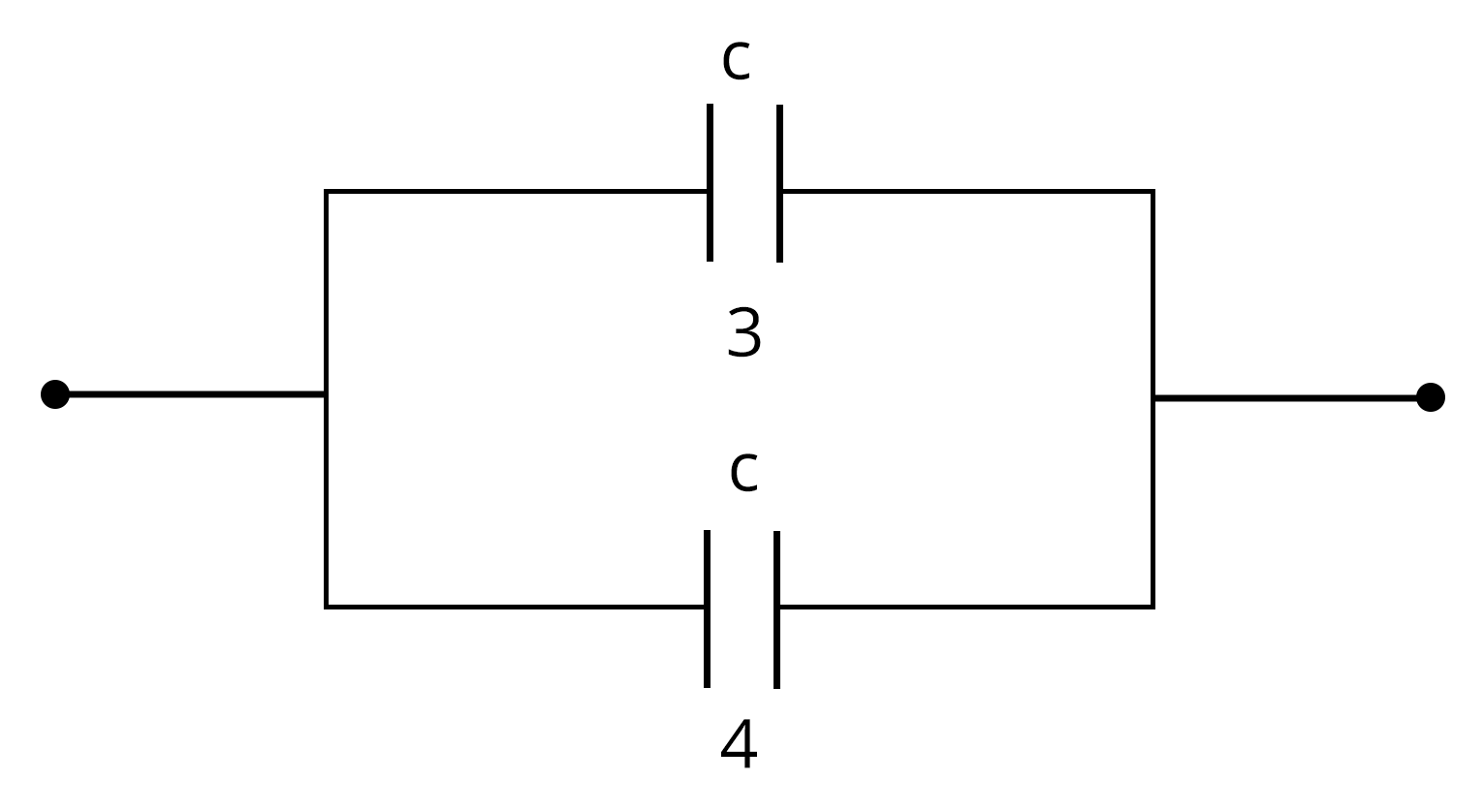
The ratio of the equivalent capacitances in series and the parallel connection is obtained by dividing equation (1) and equation (2).
The ratio of the equivalent capacitances of series and parallel connection is 1:4
Key point: In series connection, equivalent capacitance is equal to the reciprocal of the sum of the reciprocal of each capacitance. For parallel connection, equivalent capacitance is equal to the sum of each individual capacitance.
2. A point charge of +12 μC is at a distance 6 cm vertically above the centre of a square of side 12 cm as shown in the figure. The magnitude of electric flux through the square will be _____ ✕ 103 Nm2/C. (JEE 2021 Feb)
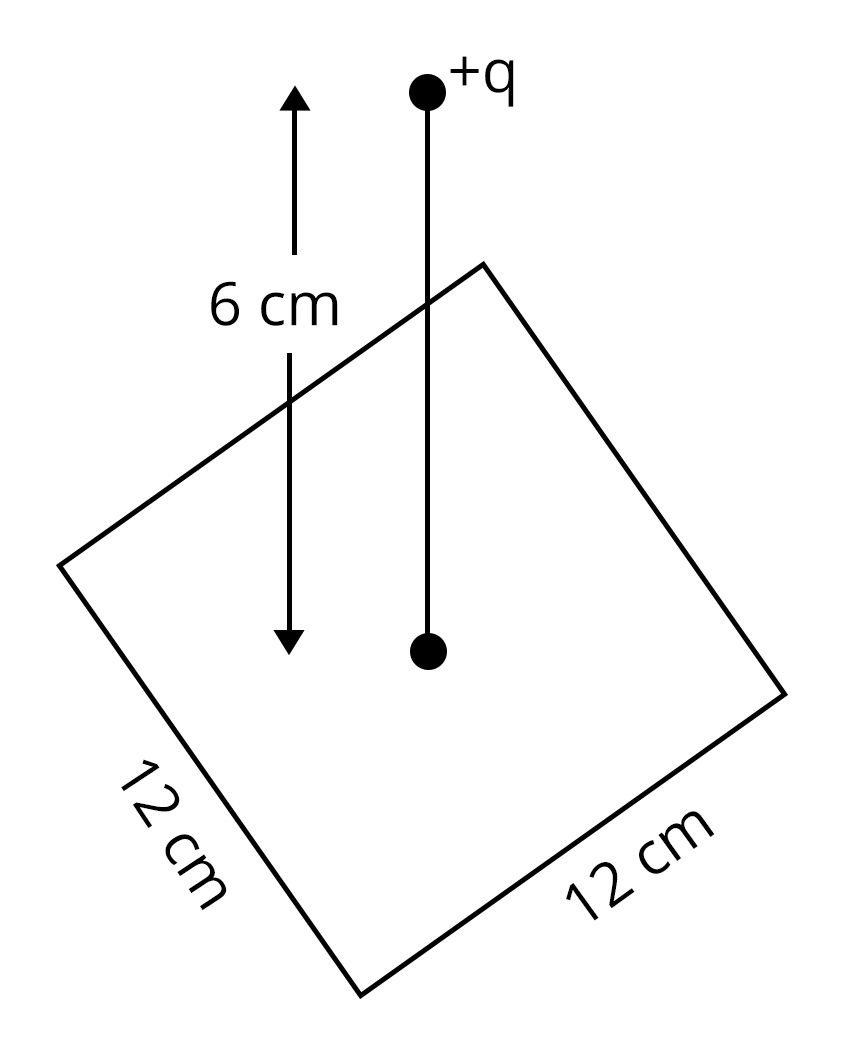
Sol. To find the electric flux, we use Gauss law and assume a Gaussian surface in the form of a cube of side length 12 cm in such a way that charge is located at the centre of the cube.
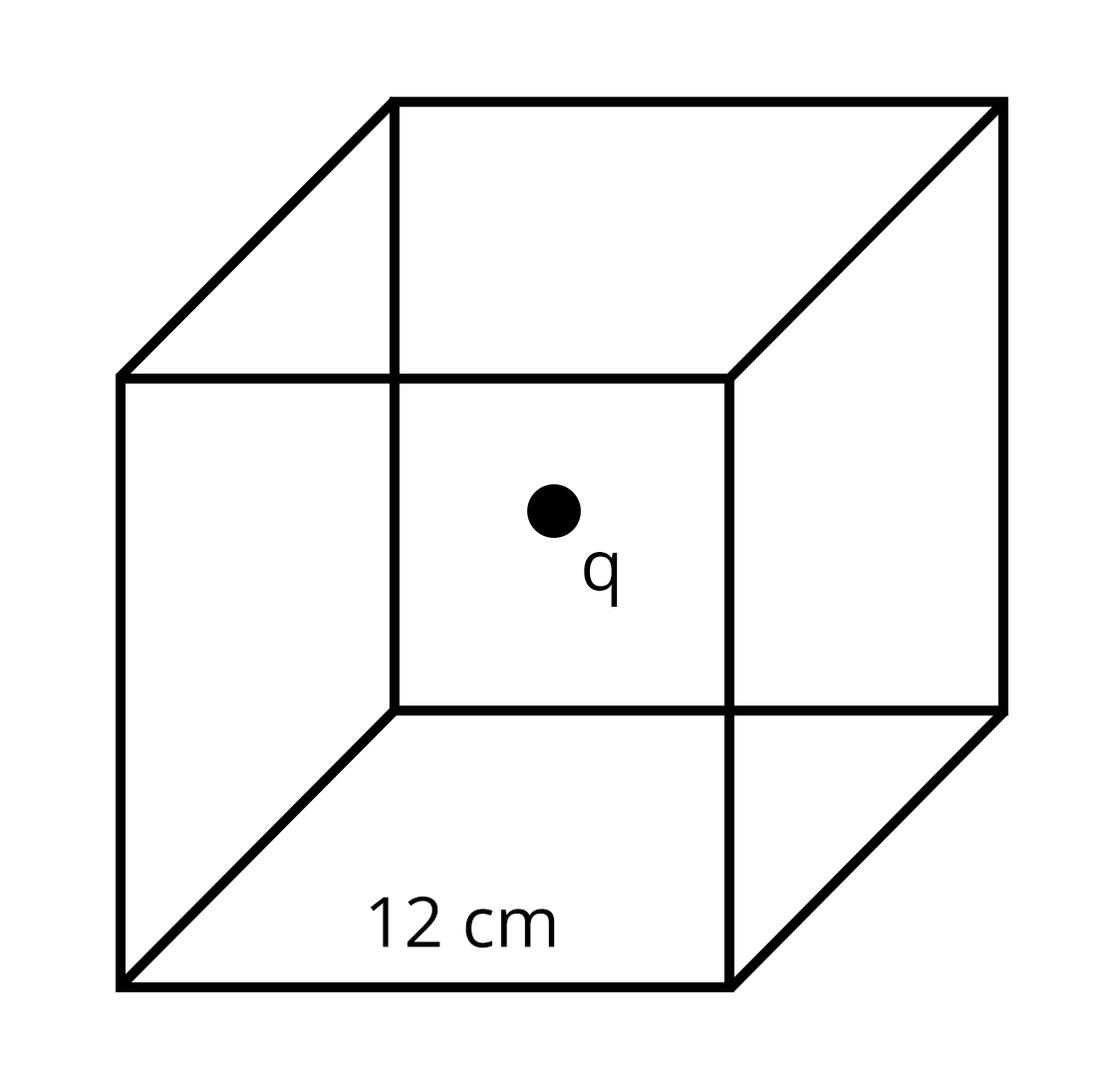
Applying Gauss law, total flux linked with the cube is given by
The electric flux(ɸ) through the square we want to find is 1/6 times the total flux.
Therefore, the flux through the square will be 226 × 103 Nm2/C.
Key point: Gauss Law is applicable to any Gaussian surface irrespective of size and shape.
Practice Questions
1. There is 10 mC of charge at the centre of a circle of radius 10 cm. The work done in moving a charge of 1mC around the circle once is
Answer: 0 J
2. A parallel plate capacitor having a plate separation of 2 mm is charged by connecting it to a 300 V supply. The energy density is
Ans: 0.1 J/m3
JEE Main Physics Electrostatics Study Materials
Here, you'll find a comprehensive collection of study resources for Electrostatics designed to help you excel in your JEE Main preparation. These materials cover various topics, providing you with a range of valuable content to support your studies. Simply click on the links below to access the study materials of Electrostatics and enhance your preparation for this challenging exam.
JEE Main Physics Chapters 2025
JEE Main Physics Study and Practice Materials
Explore an array of resources in the JEE Main Physics Study and Practice Materials section. Our practice materials offer a wide variety of questions, comprehensive solutions, and a realistic test experience to elevate your preparation for the JEE Main exam. These tools are indispensable for self-assessment, boosting confidence, and refining problem-solving abilities, guaranteeing your readiness for the test. Explore the links below to enrich your Physics preparation.
Conclusion
In this article, we're diving into the fascinating world of Electrostatics in physics, a key topic in JEE Main. We'll explore the fundamental concepts, problem-solving strategies, and essential equations. You'll uncover the secrets of electric charges, fields, and forces. Everything you need is right here, so you don't have to search high and low. We provide simple, downloadable PDFs filled with clear explanations and practice questions to supercharge your exam prep. Get ready to ace your exams with a solid understanding of Electrostatics.
Important Study Materials Links for JEE Exams
Electrostatics for JEE Main 2025: Key Concepts and Applications

 Share
ShareFAQs on Electrostatics for JEE Main 2025: Key Concepts and Applications
1. What is Electrostatics?
Electrostatics is the branch of physics that deals with the study of electric charges at rest. It focuses on the forces, fields, and potentials created by stationary charges.
2. Why is Electrostatic Chapter Of Class 12 Important?
Electrostatics is a fundamental chapter in Class 12 Physics, forming the basis for understanding advanced topics like electric fields, capacitors, and circuits, which are crucial for competitive exams like JEE and NEET.
3. Can you provide key Electrostatics Class 12 Formulas?
Some important Electrostatics Class 12 Formulas include:
Coulomb’s Law:
Electric Field:
Potential Energy:
Capacitance:
4. What are some real-life Electrostatics Examples?
Electrostatics Examples include:
Static electricity causing a balloon to stick to a wall.
Lightning as a discharge of static charges.
Electrostatic precipitators used for pollution control.
5. How can I prepare for the Electrostatic Chapter Of Class 12?
To prepare for Electrostatics Class 12, focus on understanding the concepts of electric fields, forces, and potential. Practice solving numerical problems and revise formulas regularly.
6. What types of questions are common in Electrostatics for competitive exams?
Questions often focus on concepts like Coulomb’s law, electric field and potential, capacitors, and charge distributions. Problems involving derivations and numerical calculations are also common.
7. How is Electrostatics related to other chapters in Class 12 Physics?
Electrostatics lays the foundation for topics like Current Electricity, Magnetism, and Electromagnetic Induction, making it essential for understanding the interconnected concepts of physics.
8. Are there any shortcuts for solving Electrostatics problems?
While understanding the concepts is key, some shortcuts involve remembering standard results for specific charge configurations, such as electric fields due to point charges, dipoles, and infinite planes.
9. What is the significance of the Electrostatics chapter in the JEE Main syllabus?
Electrostatics is one of the high-weightage chapters in JEE Main Physics. Questions from this chapter often test analytical and problem-solving skills, making it a scoring topic.
10. How do I apply Electrostatics formulas effectively in problems?
To apply Electrostatics formulas effectively, first identify the given quantities and required output. Choose the appropriate formula, substitute values carefully, and simplify step-by-step.














 Watch Video
Watch Video