Intensity in Young’s Double Slit Experiment - Important Topic for JEE

The Young’s Double Slit Experiment, or YDSE, is one of the most important experiments that laid the foundations for Modern Physics. It is an experiment that demonstrates the interference effect of light from two slits. This experiment showed the wave nature of light by showing that light interferes with itself and produces interference patterns. There has been a lot of debate about the nature of light, and Newton himself believed that light had a corpuscular nature. Huygens thought that light was a wave. However, due to Newton’s stature at that time, his view prevailed. The experimental proof of Huygen's belief came with the YDSE in 1801. 

Young sent coherent light through a pair of vertical slits, and it diffracted into a pattern on the screen kept in front of the slits. The pattern was spread out horizontally, and there were numerous lines on the screen. Without interference and diffraction, light simply would have made two lines on the screen, but this was not the case. This showed that light has a wave nature. 

Experimental Setup of YDSE

The experimental setup of Young’s double-slit experiment is shown below.

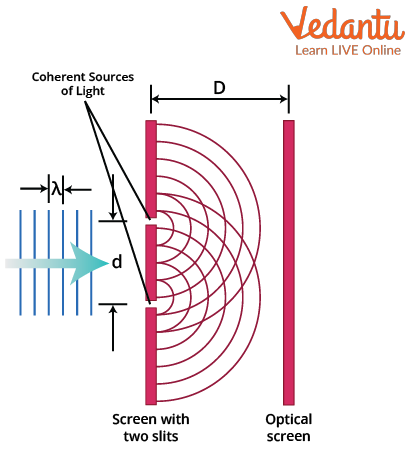
Experimental Setup of Young’s Double-Slit Experiment

Light wave theory was better understood through Young's double-slit experiment. A screen or photodetector is located far away from the slits and is denoted by the letter "D." In Young's original double-slit experiment, coherent sources were created by transmitting diffracted light from a single source through two additional slits. At that time coherent sources were not available, but today, we can instead use lasers, which act as coherent sources. One could ask a question about why coherent sources were used for this experiment. Coherent sources were used to maintain a constant phase difference between the two sources of light. 

We will now calculate and derive the results of the YDSE.

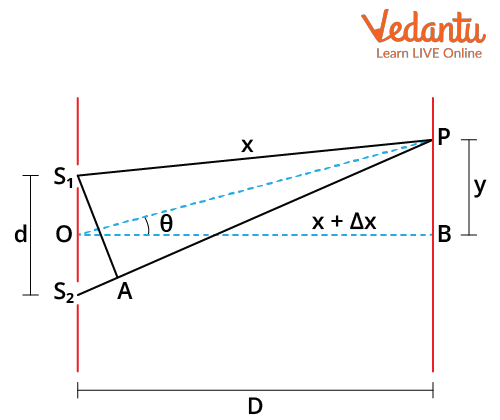
Detailed Diagram of YDSE

As we can see from the above diagram, l1-l2, is the path difference between the two waves from the slits. 

Analysis of YDSE

Consider a monochromatic light source called "S" that is separated from two slits by a great distance. S1 and S2 are in the same plane as S. S serves as the source for both S1 and S2, making them two reliable coherent sources. We need coherent sources to get the proper interference pattern. 

A screen is located at a distance "D" from the apertures S1 and S2. It receives the light after passing through these slits. The letter "d" stands for the distance between two slits.

It was observed that if any one of the slits was not open, an interference pattern was not formed. So both slits have to be open in order to get interference. The light waves from both slits travel different distances on their way to the screen. From the diagram above, we can see that S1P=x and S2P=x+$\Delta$x. 

We can represent the light from the two sources as

$\begin{align} &y_{1}=A \sin (k x-\omega t) \\ &y_{2}=B \sin (k(x+\Delta x)-\omega t) \end{align}$

Here, A and B are the amplitudes of the waves, ω is the angular frequency of the wave, and k is the wave number.

Y2 can be simplified as

$\begin{align} &y_{2}=B \sin (k x-\omega t+k \Delta x) \\ &y_{2}=B \sin (k x-\omega t+\phi) \end{align}$

Here, $\phi$ is the phase difference between the two waves. It can be written as

$\begin{align} &\phi=k \Delta x \\ &\phi=\dfrac{2 \pi}{\lambda} \Delta x \end{align}$

When light interferes, the resultant of this interference can be given by the superposition principle. We have the displacement of the resultant wave as

$\begin{align} &y=y_{1}+y_{2} \\ &y=A \sin (k x-\omega t)+B \sin (k x-\omega t+\phi) \end{align}$

We can further simplify it as

$\begin{align} &y=A \sin (k x-\omega t)+B(\sin (k x-\omega t) \cos \phi+\cos (k x-\omega t) \sin \phi) \\&y=A \sin (\mathrm{kx}-\omega \mathrm{t})+B \sin (\mathrm{kx}-\omega \mathrm{t}) \cos \phi+B \cos (\mathrm{kx}-\omega \mathrm{t}) \sin \phi \\ &y=\sin (\mathrm{kx}-\omega \mathrm{t})(\mathrm{A}+\mathrm{B} \cos \phi)+\cos (\mathrm{kx}-\omega \mathrm{t})(B \sin \phi) \end{align}$

Now, we can simplify this equation further by writing

$\begin{align} &A+B \cos \phi=x \cos \theta \\ &B \sin \phi=x \sin \theta \end{align}$

If we substitute these in the equation before, we get

$\begin{align} &y=x \sin (k x-\omega t) \cos \theta+x \cos (k x-\omega t) \sin \theta \\ &y=x(\sin (k x-\omega t) \cos \theta+\cos (k x-\omega t) \sin \theta) \\ &y=x \sin (k x-\omega t+\theta) \end{align}$

So we find that the resultant wave is indeed a sine wave that has an amplitude x and has a phase difference of $\theta$ from the first wave. 

Now, to find the amplitude x of the resultant wave, we can square both sides and then add the equations

$\begin{align} &A+B \cos \phi=x \cos \theta \\ &B \sin \phi=x \sin \theta \end{align}$

We get

$\begin{align} &(x \cos \theta)^{2}+(x \sin \theta)^{2}=(A+B \cos \phi)^{2}+(B \sin \phi)^{2} \\&x^{2} \cos ^{2} \theta+x^{2} \sin ^{2} \theta=A^{2}+2 A B \cos \phi+B^{2} \cos ^{2} \phi+B^{2} \sin ^{2} \phi \\ &x^{2}\left(\cos ^{2} \theta+\sin ^{2} \theta\right)=A^{2}+2 A B \cos \phi+B 2\left(\cos ^{2} \phi+\sin ^{2} \phi\right) \\&x^{2}=A^{2}+B^{2}+2 A B \cos \phi \\ &x=\sqrt{A^{2}+B^{2}+2 A B \cos \phi} \end{align}$

This is the amplitude of the resultant wave, and it is dependent on the amplitudes of the individual waves and a cross term with the cosine of the phase difference between them. 

Constructive and Destructive Interference

We have already said before that we get an interference pattern on the screen. This interference pattern consists of alternate dark and bright spots of light at equal distances from the central bright pattern, which we call the central maxima. We can now formulate a mathematical approach for both the constructive and destructive interference conditions.

We have the amplitude of the resultant wave as

$x=\sqrt{A^{2}+B^{2}+2 A B \cos \phi}$

From this equation, it is clear that the maximum and minimum value of x depends on the cross term between a and b. The maximum value will be

$x=\sqrt{A^{2}+B^{2}+2 A B}$

This maximum value comes when 

$\begin{gather} \cos \phi=1 \\ \phi=2 n \pi \end{gather}$

But we know that

$\phi=\dfrac{2 \pi}{\lambda} \Delta x$

Substituting this, we get

$\begin{align} &\dfrac{2 \pi}{\lambda} \Delta x=2 n \pi \\ &\Delta x=n \lambda \end{align}$

So we see that the waves interfere constructively when the path difference is an integral multiple of the wavelength. These are the conditions for constructive interference. 

Similarly, the minimum value is

$x=\sqrt{a^2+b^2-2ab}$

The minimum value comes when

$\begin{align} &\cos \phi=-1 \\ &\phi=(2 n+1) \pi \\ &\dfrac{2 \pi}{\lambda} \Delta x=(2 n+1) \pi \\ &\Delta x=\left(n+\dfrac{1}{2}\right) \lambda \end{align}$

So we can say that the waves interfere destructively if the path difference is equal to an odd half integral multiple of the wavelength. 

These are the conditions for destructive interference.

The formulas in this section are called ydse formulas. 

Fringe Width

Fringe width refers to the distance between two consecutive bright fringes or any two consecutive dark fringes. From the detailed diagram of YDSE, we can see that D or OB is the separation between the slits and the screen. Similarly, d is the separation between the slits. When the experiment is performed the distance between the slits is too small and the screen is also placed at a good distance from the slits. So we can say that, d < < D.

This is obviously an approximation but a useful one. Under this approximation we could say that paths S1P and S2P are approximately parallel to each other and we could also say that S1A is almost perpendicular to S1P, S2P, and OP. By approximation, we could then say that, $\angle A S_{1} S_{2}=\theta=\angle P O B$

And because D>>d, this angle will be very small and so,

$\tan \theta \approx \sin \theta \approx \theta$

These are small angle approximations. So the path difference can be written as

$\Delta x=P S_{2}-P S_{1} \approx P S_{2}-P A$

We can write this as

$\begin{align} &\Delta x=S_{2} A=d \sin \theta \\ &\Delta x \approx d \tan \theta \\ &\Delta x \approx d \dfrac{y}{D} \end{align}$

Here y is the distance at which the fringes are formed from the point B in the YDSE diagram. Now for bright fringes we know that

$\Delta x=n\lambda$

So, for bright fringes we can write

$\begin{align} &n \lambda=\dfrac{d y}{D} \\ &\dfrac{n \lambda D}{d}=y \end{align}$

Here n is an integer and for different values of n we can write y as

$y=0, \pm \dfrac{D \lambda}{d}, \pm \dfrac{2 D \lambda}{d}, \cdots$

Similarly for dark fringes we can write the distance from the point B as,

$\begin{align} &y=\left(n+\dfrac{1}{2}\right) \dfrac{D \lambda}{d} \\ &y=\pm \dfrac{D \lambda}{2 d}, \pm \dfrac{3 D \lambda}{2 d}, \pm \dfrac{5 D \lambda}{2 d} \end{align}$

Now the distance between any two consecutive fringes can be calculated. Let’s calculate it for bright fringes by taking the first two values. This will give the fringe width w.

$\begin{align} &w=\dfrac{D \lambda}{d}-0 \\ &w=\dfrac{D \lambda}{d} \end{align}$

This will be the same for dark fringes too, let’s show it now. We’ll take the first two values for dark fringes and calculate the distance between these dark fringes.

$\begin{align} &w=\dfrac{3 D \lambda}{2 d}-\dfrac{D \lambda}{2 d} \\ &w=\dfrac{D \lambda}{d} \end{align}$

The fringe width in Young’s double slit experiment is hence given as

$w=\dfrac{D\lambda}{d}$

The fringe width depends on the distance between the slits, the distance between the slits and the screen and the wavelength of light used.

Intensity in YDSE

What is the intensity of light? We will first understand the meaning of intensity. The brightness or quantity of light produced by a particular lighting source is referred to as the light's intensity. It is a way to quantify how much power, weighted by wavelength, a light source emits. There are specialised high and low light intensity fixtures, lamps, and bulbs, and light intensity changes depending on the lamp source. For instance, fluorescent lights are regarded as "cool" or low-intensity light sources, whereas high-intensity discharge lamps emit light with high intensity. The S.I. unit of the intensity of light is Candela.

The intensity of the wave formula is

$I=\dfrac{1}{2} \varepsilon_{0} a^{2}$

Here, $\varepsilon_{0}$ is the permittivity of free space and a is the amplitude of the wave. This equation is also known as the intensity formula. 

We see that the intensity of the light formula is given by the fact that it is directly proportional to the square of the amplitude. In this case, the amplitude of the resultant wave is,

$x=\sqrt{a^{2}+b^{2}+2 a b \cos \phi}$

This means that the intensity of the resultant wave is,

$I \propto x^{2}$

$I \propto a^{2}+b^{2}+2 a b \cos \phi$

$I=a^{2}+b^{2}+2 a b \cos \phi$

We have taken the proportionality constant to be 1 for the sake of convenience.

For maximum intensity we have, 

$\begin{align} &\cos \phi=1 \\ &\phi=2 n \pi \\ &I=a^{2}+a^{2}+2 a^{2} \\ &I=4 a^{2} \end{align}$

For minimum intensity,

$\begin{align} &\cos \phi=-1 \\ &\phi=(2 n+1) \pi \end{align}$

The minimum intensity is,

$\begin{align} &I=2 a^{2}-2 a^{2} \\ &I=0 \end{align}$

These expressions describe the intensity distribution in the interference pattern. In the interference pattern, we can see that the 2a1a2 is simply transferred from minima to maxima points. The net intensity actually remains constant, or we can say that the average intensity remains conserved. 

Conclusion

Young performed the double-slit experiment in 1801, and it proved the wave nature of light which was highly debatable at that time. Newton believed that light was corpuscular in nature, while Huygens believed that light had a wave nature. Young’s experiment was a big break-through experiment in Physics. The light from two coherent light sources was allowed to pass through two slits and interference patterns were observed on the screen. This interference pattern had bright and dark alternate fringes. This was only possible if the light was a wave. The intensity of the light wave is directly proportional to the square of the amplitude of the wave. The maximum intensity of the fringe in the interference pattern is 4a2 and the minimum intensity was 0.