




Exploring the Behaviour and Calculation of Magnetic Field Intensity Using Ampère's Law
The magnetic field generated by an infinite wire in a cylindrical configuration is a fundamental concept in electromagnetism and an essential topic for students preparing for JEE Main 2025. When an electric current flows through a conductor, it creates a magnetic field around it, and understanding this behaviour is critical for solving related problems in physics. For a cylindrical conductor, the magnetic field depends on factors such as the geometry of the wire (solid or hollow) and the region where the field is being measured—inside or outside the cylinder. Using laws like Ampère's Law, we can calculate the magnetic field and analyse its variation with distance, providing insights into its intensity and direction. This topic not only forms the basis for theoretical understanding for JEE Main but also has practical applications in devices like solenoids, motors, and inductors.
Magnetic Field Due to Infinite Wire in Cylinder
When an electric current is passed in a cylindrical wire, a magnetic field is generated. The generated magnetic field and its variation depends mainly on the type of wire i.e., solid or hollow and the region where we are measuring the magnetic field. The regions can be distinguished as follows:
Outside the Cylinder
By using Ampere's law, we can calculate the magnetic field due to a cylindrical wire outside the cylindrical wire.
In all cases like in case of solid cylinder, thin hollow cylinder as well as thick hollow cylinder, we can write magnetic field at P as
$ \oint{\vec{B}\cdot d\vec{l}}={{\mu }_{0}}i\Rightarrow B\int{dl=}{{\mu }_{0}}i $
$ B\times 2\pi r={{\mu }_{0}}i\Rightarrow {{B}_{out}}=\frac{{{\mu }_{0}}i}{2\pi r} $
In all the above mentioned cases, BSurface $=\frac{{{\mu }_{0}}i}{2\pi R}$
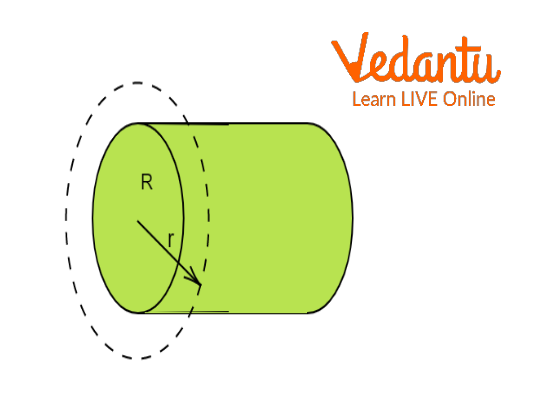
Magnetic Field Outside a Solid Cylinder
Magnetic Field Inside A Hollow Cylinder
Here we will find what is the magnetic field inside a hollow cylindrical pipe. Magnetic field inside the hollow cylinder will be zero at any point. Also the electric field due to the hollow cylinder is zero. This can be proved by Gauss law. Gauss law states that the total electric flux through a closed sphere is equal to the total charge enclosed inside the sphere divided by the electrical permittivity. So, if there is no electric field, then there is no active charge passing through a cylindrical conductor. And thus, there will be no magnetic field inside the hollow cylinder.
Let us take a point at a distance r from the centre of the hollow cylinder but inside the cylinder. According to the Gauss law,
${{\phi }_{r}}=\frac{{{Q}_{in}}}{{{\varepsilon }_{0}}}$
${{\phi }_{r}}=\frac{{{Q}_{in}}}{{{\varepsilon }_{0}}} $
$E\times 4\pi {{r}^{2}}=\frac{0}{{{\varepsilon }_{0}}} $
$E=0$
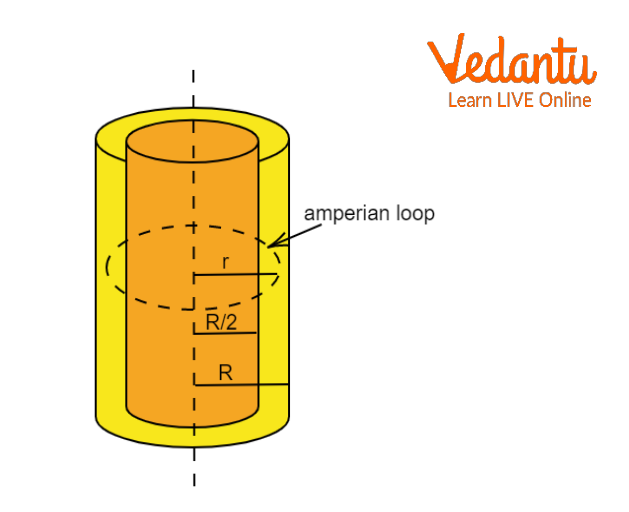
Magnetic Field Inside the Hollow Cylinder
Thus , the magnetic field inside the hollow cylinder will be zero at any point.
Inside the Solid Cylinder
If we are talking about the magnetic field at distance r from the axis where r<R then current enclosed in the region( i’) will be less than the total current i.
Current density is uniform so
$i'=i\times \frac{A'}{A}=i\frac{{{r}^{2}}}{{{R}^{2}}}$
Hence at point inside,$\oint{\vec{B}\cdot d\vec{l}}={{\mu }_{0}}i'$
$B=\frac{{{\mu }_{0}}ir}{2\pi {R^2}}$
Inside the Thick Part of Hollow Cylinder
As current density is constant, current enclosed in a loop can be given as
$ {i}'=i\times \frac{{{A}'}}{A} $
$ =i\frac{\left( {{r}^{2}}-R_{1}^{2} \right)}{\left( R_{2}^{2}-R_{1}^{2} \right)}$
So magnetic field at point Q is, $\oint{\vec{B}\cdot d\vec{l}}={{\mu }_{0}}i'$
$B=\frac{{{\mu }_{0}}i\left( {{r}^{2}}-R_{1}^{2} \right)}{2\pi \left( R_{2}^{2}-R_{1}^{2} \right)}$
Magnetic Field Inside the Thick Part of Hollow Cylinder
Magnetic Field Due to Infinite Wire
Coming back to the main case, an infinitely long wire placed in space is carrying some current through it. As there is movement of electric charges, there shall be generation of a magnetic field. For an infinitely long wire, thickness is negligible compared to length. So, our main concern is calculating the magnetic field outside the wire. So, we can resolve it in the same way as the magnetic field outside the cylinder, which is ${{B}_{out}}=\frac{{{\mu }_{0}}i}{2\pi r},$where r is distance from the axis.
So, we have the general Magnetic Field Due To Infinite Wire Formula ie.
Magnetic Field Due to Infinite Current Carrying Wire
Considering the nature, the magnetic field lines of an infinitely long wire are circular and centred at the wire, and they are identical in every plane perpendicular to the wire. Since the field decreases with distance from the wire, the spacing between field lines must decrease as well.
The direction of the magnetic field may be found by right hand thumb rule. If you hold the wire with your right hand so that your thumb points along the current then movement of fingers will travel in the same sense as the magnetic field vector. The direction of field lines can also be simulated by placing a compass needle near the wire. When current will be passed through the wire, the needles will align themselves tangent to the circle passing through them with the centre on the axis of the wire.
Same observation can be recorded by sprinkling iron filings on a horizontal surface. When the current is passed, iron filings will align themselves the same as compass needles.
Applications of Magnetic Field Due to Infinite Wire in Cylinder
The magnetic field generated by an infinite current-carrying wire is used in electromagnetic induction to create electric currents in nearby conductors. This principle is widely applied in the functioning of transformers and inductors.
Cylindrical conductors are used in creating magnetic shielding to protect sensitive electronic components from external magnetic fields. Understanding the magnetic field behaviour is essential for designing such shields.
The concept is utilised in the design and functioning of electromechanical devices like electric motors and generators, where cylindrical wires and their magnetic fields play a significant role.
The magnetic field generated by high-current cylindrical wires in power transmission lines is studied to minimise electromagnetic interference and energy losses in the system.
Cylindrical wire configurations are used in magnetic sensors to measure magnetic fields accurately in various industrial and scientific applications.
The infinite cylindrical wire model helps simplify complex magnetic field problems, making it a useful tool in theoretical studies and practical experiments in magnetostatics.
Conclusion
Magnetic Field Due To Infinite Current Carrying Wire is a special case of magnetic field generated by cylinders. As the length of the wire extends up to infinity, the intensity will only be dependent on the radial distance from the axis and obviously the current. We approached the concept that the line integral of the magnetic field around a closed path is equal to total current flowing through the area bounded by that closed path. We can apply this for other conductors as well to find the intensity of the Magnetic Field Due To Wire.
JEE Main 2025 Subject-Wise Important Chapters
The JEE Main 2025 subject-wise important chapters provide a focused strategy for Chemistry, Physics, and Maths. These chapters help students prioritise their preparation, ensuring they cover high-weightage topics for better performance in the exam.
Important Study Materials Links for JEE Exams
FAQs on Magnetic Field Due to Infinite Wire in Cylinder for JEE Main Physics 2025
1. Why is the behavior of the magnetic field the same for both solid and hollow cylinders when calculating outside the surface?
When we calculate the magnetic field outside the surface, the diameter of the wire is very small compared to the distance at which intensity is being calculated. Also, the effects of change of distance cancel each other out. So, it can be treated as a single wire placed at the axis and the variation of magnetic field is same for both the cases.
2. How does the magnetic field depend on the direction of the current?
The polarity of current decides the direction of flow of magnetic field as well. If the polarity of current is reversed then the direction of flow of magnetic field lines will also be revered. We can visualise the directions by using the second form of right-hand thumb rule. If you point your thumb in the direction of current flow, the travel of fingers when you will wrap them, will depict the direction of magnetic field lines. So, you will be able to see that when you reverse the direction of current then you need to point the thumb in the opposite direction as before, the movement of fingers will also be in the opposite direction. This is the change in direction of the magnetic field when current is reversed.
3. How does the magnetic field inside a hollow cylinder compare to that inside a solid cylinder?
The magnetic field inside a hollow cylinder is zero, as there is no current enclosed within the cylindrical surface for any point inside the shell. However, inside a solid cylinder, the magnetic field is proportional to the distance from the center, increasing linearly with the radial distance.
4. What happens to the magnetic field at the center of a current-carrying wire?
At the center of a current-carrying wire, the magnetic field is strongest, and its direction follows the right-hand thumb rule. The intensity of the field depends on the current and the radius of the wire. For a wire with a radius rrr, the field strength decreases with distance from the wire's surface, becoming weaker as the distance increases.
5. How does the magnetic field change with distance from an infinite wire?
For an infinite straight wire carrying a constant current, the magnetic field decreases inversely with distance from the wire. The field intensity is given by$B = \dfrac{\mu_0 I}{2 \pi r}$, where III is the current and r is the distance from the wire.
6. Why is the magnetic field outside a cylinder with current the same for both solid and hollow cylinders?
For both solid and hollow cylinders, the magnetic field outside the cylinder is the same because the current enclosed by any Gaussian surface outside the cylinder is effectively the same. This is due to the fact that the contribution to the magnetic field depends only on the total enclosed current, not the shape of the current distribution.
7. How can we use Ampère’s Law to calculate the magnetic field in a cylinder?
Ampère’s Law states that the integral of the magnetic field along a closed loop is proportional to the total current enclosed by that loop. For an infinite current-carrying cylinder, we can choose a circular loop around the cylinder, and Ampère’s Law helps derive the magnetic field at a distance r from the axis, given by $B = \dfrac{\mu_0 I}{2 \pi r}$, where I is the enclosed current.
8. What is the significance of the right-hand thumb rule in determining the direction of magnetic field?
The right-hand thumb rule helps visualise the direction of the magnetic field around a current-carrying conductor. If you point the thumb of your right hand in the direction of current flow, your curled fingers will indicate the direction of the magnetic field lines. This rule is fundamental in understanding the orientation of the magnetic field.
9. Does the magnetic field produced by an infinite wire depend on the wire's material?
No, the magnetic field produced by an infinite wire depends only on the current and the distance from the wire, not on the material of the wire. However, the material may affect the wire’s resistance and current-carrying capacity, but the magnetic field calculation itself is independent of the material.
10. Can the magnetic field be measured directly at a point in the vicinity of a current-carrying wire?
Yes, the magnetic field can be measured using instruments like a magnetometer or a Hall effect sensor, which can detect the magnetic field intensity at any point around the current-carrying wire. The strength of the field is inversely proportional to the distance from the wire.










