Projectile Motion - JEE

When we throw an object in the air, we notice that the path traced by the object in order to reach the ground will be a parabola or parabolic path. So, we can describe the projectile motion as a motion of a particle that moves in a parabolic path, the name itself comes from the particle's trajectory. There are specific parameters that affect the projectile motion, such as the maximum height, range, etc… In this article, we will discuss the important parameters of a projectile motion in detail. Let's begin!!

What is Projectile Motion?

When an object is in flight and is not propelled by any fuel is known as the projectile. A particle or body moves in the atmosphere under the influence of gravity and following a parabolic path is known as projectile motion. When we consider the motion of a projectile, we presume certain factors as listed below:

1. There would be no effect due to the curvature of the earth.

2. No effect due to rotation of the earth.

3. We don't consider the air resistance which affects the motion.

4. During the motion of trajectory, the acceleration due to gravity is always downwards and remains constant throughout the motion.

Types of Projectile Motion

1. Horizontal Projectile Motion

When a body is projected from a certain height with a specific velocity, at any instant, the horizontal velocity remains the same $({{a}_{x}}=0)$.

2. Oblique Projectile Motion

When a body is projected at a certain angle with the horizontal, it is resolved into two components. One is along the vertical direction and another one is horizontal. These components are independent of each other.

Resolving The Velocity Components

The initial velocity can be expressed in terms of x components and y components

${{u}_{x}}=u.\cos \theta $, and ${{u}_{y}}=u.\sin \theta $ where u is the initial velocity and $\theta $ is the angle with which the object is projected.

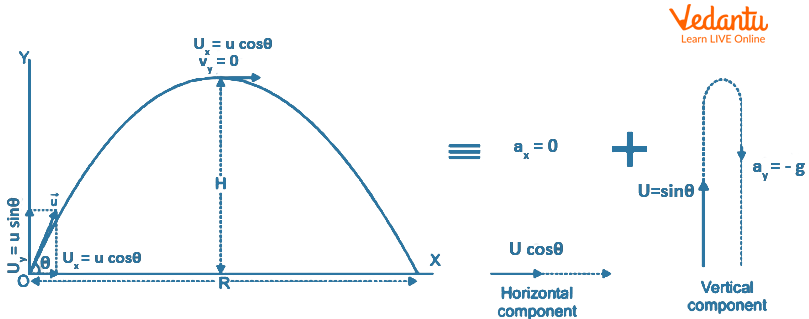
Representing the Projectile Motion and the Resolved Components of Vertical and Horizontal Components of Velocity

Range: What is Range and its Brief Study

The horizontal range of a projectile is the distance travelled by the object between the launch and landing points. Now let us find the expression for the range of a projectile.

Equation of Trajectory

Let’s say $\theta $be the angle with which the particle is launched.  The expression for the horizontal distance covered is given by:

$R={{u}_{x}}\times T$ 

where T is the time of flight. 

Using the equation of trajectory,

$S=ut+\frac{1}{2}g{{t}^{2}}$

The time over which the displacement S becomes zero is given by,

$0={{u}_{y}}T-\frac{1}{2}g{{T}^{2}}\text{ or T=}\frac{2{{u}_{y}}}{g}\text{ or T=}\frac{2u\sin \theta }{g}$

That is Range, 

$R=\frac{2{{u}^{2}}\sin \theta \cos \theta }{g}=\frac{{{u}^{2}}\sin 2\theta }{g}$

Let’s see when the R is going to be the maximum for a given velocity. We can see that the range depends on $\theta $ and the initial velocity u. 

The maximum value that $\sin 2\theta $ can take is 1. 

$\sin 2\theta =1$,  $\theta ={{45}^{{}^\circ }}$ is the value of the angle of projection for which we can obtain the maximum range.

Also, the expression for the maximum range R is as follows:

${{R}_{\max }}=\frac{{{u}^{2}}}{g}$

Time of Flight and Its Dependence on Projectile Motion

The time of flight can be defined as the time taken by the object to reach the ground once it is projected. 

Equations of Motion: Time of Flight

The time-of-flight expression is formulated using the equation of motion:

That is, $g{{T}^{2}}=2(2{{u}_{y}}t-{{S}_{y}})$,where $u_{y}=sin \theta$  and ${{S}_{y}}=0$, which is our vertical displacement.

On rearranging the parameters in terms of T, we get

$T=\frac{2u\sin \theta }{g}$

From this, we can say that the time of flight depends on the magnitude of initial velocity and the angle of the projectile.

Maximum Height of a Projectile

The maximum height of a projectile is defined when its vertical component of the velocity is zero. In other words, we can say that at the highest point or at the maximum height, the vertical component of the velocity is zero. Using this idea, we can find the expression for the maximum height. 

Consider the equation $v_{y}^{2}-u_{y}^{2}=2gs$, where v and u are the final and initial velocities, and S is the displacement.

Here, at a higher point, S=H, ${{v}_{y}}=0$

On substituting the values in the equation,

$H=\frac{{{u}^{2}}{{\sin }^{2}}\theta }{2g}$

So, the H depends on the angle of the projectile and the magnitude of the velocity with which the object is projected.

For the height to be maximum for a given velocity, ${{\sin }^{2}}\theta =1$, i.e., 

$\theta ={{90}^{{}^\circ }}$

${{H}_{\max }}=\frac{{{u}^{2}}}{2g}$

Summary

In this article, we have learnt the major parameters of projectile motion. We derived the formula for the range of the projectile, height, and time of flight projectile. We studied the types of projectile. From the above discussion, we can conclude that projectile motion is one of the most important concepts of one and 2D motion and the parameters of the projectile motion help us in understanding the Physics behind it.