JEE Main 2025: Physics Projectile Motion on Inclined Plane

Range of Projectile Motion on Inclined Plane

Let us assume a ball is thrown upwards on an inclined plane, which makes an angle θ0 with the horizontal surface of the earth. The ball is thrown with the velocity u and it makes an angle ⍺ with the inclined plane. The ball, which is in a projectile motion, will have an initial velocity, acceleration, and final velocity. Each quantity can be divided into two components in the XY plane, as shown in the figure. 

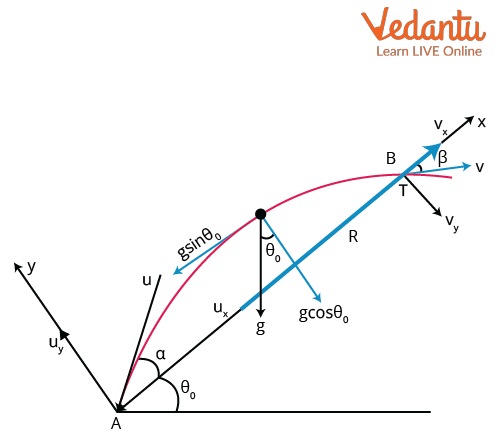
Projectile Motion on Inclined Plane 

In the above figure, we can see that the path of the ball or projectile is from A to B.  The inclined surface makes an angle θ0 with the horizontal and the ball is thrown with the initial velocity u at an angle of ⍺ with the inclined surface. 

The X-axis is taken parallel to the inclined plane and the Y-axis is perpendicular to the inclined plane, as shown in the figure. The horizontal and vertical components of initial velocity in the X and Y directions are ux and uy , respectively.

Here, ux  = ucos⍺ and uy = usin⍺

The acceleration on a projectile is the acceleration due to gravity and it is acting downwards in a negative XY axes direction. So, it has a negative sign. The components are divided as shown in the figure.

I.e., $a_{x}=-g \sin \theta_{0}$ and $a_{y}=-g \cos \theta_{0}$

$a_{y}=-g \cos \theta_{0}$

The distance travelled by the projectile from A to B is in the x-direction, So Sx = R where R is the range and Sy = 0. 

According to the Laws of motion, $\mathrm{S}=u t+\dfrac{1}{2} a t^{2}$

Let us take the equation of distance in the y-direction, i.e., Sy = 0 

I.e., $S_{y}=u_{y} t+\dfrac{1}{2} a_{y} t^{2}$

Putting the values of uy and ay in the above equation, 

$\begin{align} &(u \sin \alpha) T-\dfrac{1}{2}\left(g \cos \theta_{o}\right) T^{2}=0 \\ &2(u \sin \alpha) T=\left(g \cos \theta_{o}\right) T^{2} \\ &T=\dfrac{2 u \sin \alpha}{g \cos \theta_{o}} \end{align}$

This is the time of flight formula of a projectile on an inclined plane. Here, T is the total time taken to travel the distance from A to B and this is known as the time of flight. 

Now, let us take the distance in the x-direction and substitute the x components in it. 

$\begin{align} &S_{x}=u_{x} t+\dfrac{1}{2} a_{x} t^{2} \\ &R=(u \cos \alpha) T-\dfrac{1}{2}\left(g \sin \theta_{o}\right) T^{2} \end{align}$

Putting the value of T in this equation, 

$\begin{align} &R=(u \cos \alpha) \dfrac{2 u \sin \alpha}{g \cos \theta_{o}}-\dfrac{1}{2}\left(g \sin \theta_{o}\right)\left(\dfrac{2 u \sin \alpha}{g \cos \theta_{o}}\right)^{2} \\ &R=\dfrac{2 u^{2} \sin \alpha \cos \alpha}{g \cos \theta_{o}}-\dfrac{2 u^{2} \sin ^{2} \alpha \sin \theta_{o}}{g \cos ^{2} \theta_{o}} \end{align}$ 

$\begin{align}&R=\dfrac{u^{2} \sin 2 \alpha}{g \cos \theta_{o}}-\dfrac{2 u^{2} \sin ^{2} \alpha \sin \theta_{o}}{g \cos ^{2} \theta_{o}} \quad \text { As } 2 \sin \alpha \cos \alpha=\sin 2 \alpha \\ &R=\dfrac{u^{2}}{g \cos ^{2} \theta_{o}}\left[\left(\sin 2 \alpha \cos \theta_{0}\right)-\sin \theta_{0} 2 \sin ^{2} \alpha\right]\end{align}$

We know, $2 \sin ^{2} \alpha=1-\cos 2 \alpha$

$\begin{align} &R=\dfrac{u^{2}}{g \cos ^{2} \theta_{0}}\left[\left(\sin 2 \alpha \cos \theta_{0}\right)-\sin \theta_{0}(1-\cos 2 \alpha)\right] \\ &R=\dfrac{u^{2}}{g \cos ^{2} \theta_{0}}\left[\sin 2 \alpha \cos \theta_{0}-\sin \theta_{0}+\sin \theta_{0} \cos 2 \alpha\right]\end{align}$

$\text {Therefore } \sin 2 \alpha \cos \theta_{0}+\sin \theta_{0} \cos 2 \alpha=\sin \left(2 \alpha+\theta_{0}\right)$

$R=\dfrac{u^{2}}{g \cos ^{2} \theta_{0}}\left[\sin \left(2 \alpha+\theta_{0}\right)-\sin \theta_{0}\right]$

Therefore, the equation of the range of projectile formula on the inclined plane is given by 

$R=\dfrac{u^{2}}{g \cos ^{2} \theta_{0}}\left[\sin \left(2 \alpha+\theta_{0}\right)-\sin \theta_{0}\right]$

If the projectile is projected in the downward direction of the inclined plane, then the distance is taken in the negative x direction, i.e., - R and the angle is taken as ⍺^| , as shown in the figure. 

Projectile down the inclined plane

Here $\alpha^{\prime}+\alpha=180$, so $\alpha=180-\alpha^{\prime}$

$\begin{align} &-R=\dfrac{u^{2}}{g \cos ^{2} \theta_{o}}\left[\sin \left(2\left(180-\alpha^{\prime}\right)+\theta_{0}\right)-\sin \theta_{0}\right] \\ &-R=\dfrac{u^{2}}{g \cos ^{2} \theta_{o}}\left[\sin \left\{360-\left(2 \alpha^{\prime}-\theta_{0}\right)\right\}-\sin \theta_{0}\right]\end{align}$

$\begin{align} & -R=\dfrac{u^{2}}{g \cos ^{2} \theta_{o}}\left[-\sin \left(2 \alpha^{1}-\theta_{0}\right)-\sin \theta_{0}\right] \\ & R=\dfrac{u^{2}}{g \cos ^{2} \theta_{o}}\left[\sin \left(2 \alpha^{\prime}-\theta_{0}\right)+\sin \theta_{0}\right]\end{align}$ 

This is the range of projectiles down the inclined plane formula. Let us look into the equation of the trajectory of the projectile. 

Equation of Trajectory of Projectile 

Let us assume a general projectile on a horizontal plane is thrown with the velocity of u at an angle $\alpha$ with the horizontal surface, and the acceleration is the acceleration due to gravity, which acts along only the y-direction. 

The horizontal distance, $x = (u\cos{\alpha})t$ as there is no acceleration due to gravity in the horizontal direction. 

I.e.,$ \mathrm{t}=\dfrac{x}{u \cos \alpha}$ 

The vertical distance,

$y=u \sin \alpha . t-\dfrac{1}{2} g t^{2}$

$\begin{align} &\text { Where } t=\dfrac{x}{u \cos \alpha}\\ & y=(u \sin \alpha) \dfrac{x}{u \cos \alpha}-\dfrac{1}{2} g\left(\dfrac{x}{u \cos \alpha}\right)^{2}\\ &y=x \tan \alpha-\dfrac{g x^{2}}{2 u^{2} \cos ^{2} \alpha}\\ &y=x \tan \alpha-\dfrac{x^{2}}{\frac{2 u^{2} \cos ^{2} \alpha}{g}}\end{align}$ 

$\begin{align} &y=x \tan \alpha-\dfrac{x^{2}}{\dfrac{2 u^{2} \cos \alpha \sin \alpha}{g}} \dfrac{\sin \alpha}{\cos \alpha}\\ &y=x \tan \alpha-\dfrac{x^{2}}{\dfrac{u^{2} \sin 2 \alpha}{g}} \tan \alpha\\ &\text {Let } \dfrac{u^{2} \sin 2 \alpha}{g}=R\\ &y=x \tan \alpha\left(1-\dfrac{x}{R}\right) \end{align}$

This is the projectile motion on inclined plane equation. 

Let us solve a problem on the concept of projectile motion using the inclined plane formula. 

Example: A ball is thrown from the foot of an inclined plane in an upward direction with a velocity of 10$\dfrac{m}{s}$ with an angle of 30 degrees with the inclined surface. If the inclined surface is making an angle of 30 degrees with the horizontal, find the range of the ball on the inclined plane. 

Solution: 

Given that, the initial velocity of the ball, u = 10$\dfrac{m}{s}$ 

The angle between the inclined surface and the horizontal is $\theta_0$ = 30^o 

The initial velocity makes an angle, $\alpha$ = 30^o 

We know the formula of the range travelled by the ball on a plane in an inclined position, 

$R=\dfrac{u^{2}}{g \cos ^{2} \theta_{0}}\left[\sin \left(2 \alpha+\theta_{0}\right)-\sin \theta_{0}\right]$

Putting all the values given in the equation, 

$\begin{align}&R=\dfrac{10^{2}}{10 \cos ^{2} 30}[\sin (2(30)+30)-\sin 30] \\ &R=\dfrac{10 \times 4}{3}\left[1-\dfrac{1}{2}\right] \\&R=\dfrac{40}{3} \cdot \dfrac{1}{2} \\ &R=\dfrac{20}{3}=6.67 \mathrm{~m} \end{align}$

Therefore, the distance travelled by the ball on the inclined plane is 6.67 metres. 

Conclusion

Projectile motion is a concept which studies the motion of objects in the presence of the gravitational field. Therefore, we learned about the range of projectile formulas on an inclined plane through this article. We also learnt how to derive the equation for the trajectory of a projectile and also the time of flight formula on the plane in an inclined position through this article.

JEE Main 2025 Subject-Wise Important Chapters

The JEE Main 2025 subject-wise important chapters provide a focused strategy for Chemistry, Physics, and Maths. These chapters help students prioritise their preparation, ensuring they cover high-weightage topics for better performance in the exam.

Check Other Important Links for JEE Main 2025 

Prepare effectively for JEE with our comprehensive study material links, including notes, question papers, sample papers, and mock test series for both JEE Main and Advanced. Access essential resources like formulas, important questions, and preparation tips to boost your performance.