Spherical Capacitor - Theory and Its Formula based Numerical Problems for JEE

A capacitor is an electric device and it works as batteries do. However, the way they both work is completely different. We know that a battery is an electronic device that converts chemical energy into electrical energy. At some point, we all have used a battery, whether to play with a toy or in clocks, television remotes, etc. A capacitor on the other hand is an electrical device that stores electrical energy in the form of an electric field. There are different types of capacitors and they all store charges.

In this article, we will be looking at how to calculate capacitance and then we will move on to spherical capacitors.

The Capacitance of a Capacitor

A capacitor is a two-terminal electrical component that stores energy in the form of an electric charge. It is made up of two electrical conductors that are separated by a certain distance. The space between the conductors can be filled with a vacuum or a dielectric, which is an insulating substance. Capacitance refers to the capacitor's ability to store charges.

Capacitors store energy by separating oppositely charged terminals. A parallel plate capacitor is the most basic form, consisting of two metal plates separated by a gap. One can always insert a dielectric between the two plates of a capacitor to enhance the capacitance. Capacitors come in a variety of shapes, sizes, lengths, girths, and materials. Despite the variety of capacitors available to us in the market, the basic operation principle behind them remains the same.

Suppose that two plates of a capacitor hold opposite charges +Q and -Q. If the potential difference between the plates is V, then we know that the charge is directly proportional to the potential difference introduced between the plates. This means,

$Q\propto V$

We can introduce a constant of proportionality C.

$\begin{align} &Q=C V \\ &C=\dfrac{Q}{V} \end{align}$

The unit of capacitance is Farad or F.

The constant of proportionality introduced in the above equation is capacitance. We can now define capacitance as the ratio of change in the electric charge to the corresponding change in the electric potential.

This formula of capacitance can be used to calculate the capacitance of any kind of capacitor. Depending on the use, a capacitor's capacitance might be constant or variable. It may appear from the equation that 'C' is dependent on charge and voltage. The capacitance is dependent on the capacitor's shape and size. It is also dependent on the dielectric introduced between the plates of the capacitor.

The Capacitance of a Spherical Capacitor

As the name suggests, spherical capacitors consist of two concentric conducting shells. It is also known as a spherical plate capacitor. Consider a spherical capacitor having two spherical shells of radii R1 and R2. Now, we know that the two plates of a capacitor have equal and opposite charges. Let the two shells in our case of spherical capacitors have equal and opposite charges +Q and -Q respectively.

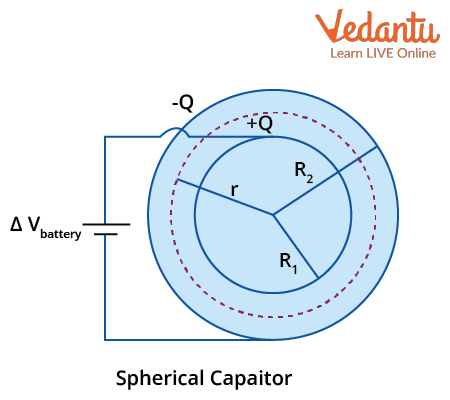
Image: Spherical Capacitor

To find the charge on the surface of the conductors, we can apply Gauss’ law. We will apply Gauss’ law over the spherical Gaussian surface of radius r. The Gaussian surface we use will be concentric with our conductor shells. We will look at the spherical capacitor derivation.

If a charge Q is enclosed inside a Gaussian surface then we can write,

$\oint_{E . \mathrm{d} A}=\dfrac{Q}{\varepsilon_{0}}$

Since the electric field is pointing radially outwards and the magnitude is the same over the Gaussian surface, we can write,

$\begin{align} &E \cdot \oint_{\mathrm{d} A}=\dfrac{Q}{\varepsilon_{0}} \\ &E \cdot 4 \pi r^{2}=\dfrac{Q}{\varepsilon_{0}} \\ &E=\dfrac{Q}{\varepsilon_{0} \cdot 4 \pi r^{2}} \end{align}$

Now we also know that,

$V=-\int E \cdot \mathrm{d} r$.

Inserting the value of E and the limits from R1 to R2, we can calculate the potential as,

$\begin{align} &V=-\int_{R_{1}}^{R_{2}} \dfrac{Q}{4 \pi \varepsilon_{0} r^{2}} \mathrm{~d} r \\ &V=-\dfrac{Q}{4 \pi \varepsilon_{0}} \int_{R_{1}}^{R_{2}} \dfrac{\mathrm{d} r}{r^{2}} \\ &V=-\dfrac{Q}{4 \pi \varepsilon_{0}}\left\{-\dfrac{1}{r}\right\}_{R_{1}}^{R_{2}} \\ &V=\dfrac{Q}{4 \pi \varepsilon_{0}}\left\{\dfrac{1}{R_{1}}-\dfrac{1}{R_{2}}\right\} \\ &V=\dfrac{Q}{4 \pi \varepsilon_{0}} \dfrac{\left(R_{2}-R_{1}\right)}{R_{2} R_{1}} \end{align}$

Now we know that,

$C=\dfrac{Q}{V}$

From the above equation for V we can write,

$\dfrac{Q}{V}=4 \pi \varepsilon_{0} \dfrac{R_{1} R_{2}}{R_{2}-R_{1}}$.

Hence, capacitance can be written as,

$C=4 \pi \varepsilon_{0} \dfrac{R_{1} R_{2}}{R_{2}-R_{1}}$

This is the capacitance of a spherical capacitor or the capacitance of a sphere. It is also known as the spherical capacitor formula.

Energy Stored in a Capacitor

The charges are placed on the conductors of the capacitor when they are connected to any battery or a power source. These charges can then be used to work. This work is done by allowing them to move toward each other inside the circuit. The total energy that can be retrieved from a fully charged capacitor is given by the equation:

$U=\dfrac{1}{2} C V^{2}$

This equation can also be written as,

$\begin{align} &U=\dfrac{Q^{2}}{2 C} \\ &U=\dfrac{1}{2} Q V \end{align}$

As discussed before, capacitors work a lot like batteries; more precisely, rechargeable batteries. The main difference between batteries and capacitors lies in the ways that they store energy within them. In batteries, the electrical energy is derived from the chemical reaction that takes place within it. Capacitors store electrical energy by storing charges.

Numerical Examples on Capacitance of Capacitor

Example 1: A spherical capacitor has an inner sphere of radius 5 cm and an outer sphere of radius 10 cm. The outer sphere is earthed. Assume there is air in the space between the spheres. What is the capacitance of the capacitor?

Solution:

Given that,

R1=5 cm=0.05 m

R2=10 cm=0.1 m

We know that, $\varepsilon_{0}=8.85 \times 10^{-12} \mathrm{C}^{2} \mathrm{~N}^{-1} \mathrm{~m}^{-2}$

The formula for the capacitance of the spherical capacitor is,

C=4 $\pi \varepsilon_{0} \dfrac{R_{1} R_{2}}{R_{2}-R_{1}}$.

Putting in all the values we got,

$\begin{align} &C=4 \times 3.14 \times 8.85 \times 10^{-12} \times \dfrac{0.1 \times 0.05}{0.1-0.05} \\ &C=1.127 \times 10^{-12} \mathrm{~F} \\ &C=1.127 \mathrm{pF} \end{align}$

This is the capacitance of the capacitor.

Example 2: Calculate the capacitance of an isolated sphere of radius 45cm.

Solution:

To solve this problem, we need to find the formula for the capacitance of an isolated sphere. An isolated sphere can be considered to be a spherical capacitor where the outer radius is infinity. Applying this to the formula of the capacitance of spherical capacitor gives,

$\begin{align} &C=4 \pi \varepsilon_{0} \dfrac{1}{\dfrac{1}{R_{1}}-\dfrac{1}{R_{2}}} \\ &C=4 \pi \varepsilon_{0} \dfrac{1}{\dfrac{1}{R_{1}}-\dfrac{1}{\infty}} \\ &C=4 \pi \varepsilon_{0} \dfrac{1}{\dfrac{1}{R_{1}}-0} \\ &C=4 \pi \varepsilon_{0} R_{1} \end{align}$

Now, the given radius is 45cm=0.45m. So the capacitance will be,

$\begin{align} &C=4 \times 3.14 \times 8.85 \times 10^{-12} \times 0.45 \\ &C=50.02 \times 10^{-12} \mathrm{~F} \\ &C=50.02 \mathrm{pF} \end{align}$

The capacitance is 50.02 pF.

Conclusion

The capacitor is an electric device that stores electrical energy by storing charges between its conductor plates. It works quite like a rechargeable battery, but the difference lies in the way that these devices store energy. The capacitors come in a variety of shapes and sizes and they are manufactured depending on the requirements.

The spherical capacitor is a type of capacitor that has two concentric shells and the charges are stored on the surface of these shells. If the inner shell has radius R1 and the outer shell has radius R2, then the capacitance of a spherical capacitor is given as,

$C=4 \pi \varepsilon_{0} \dfrac{R_{1} R_{2}}{R_{2}-R_{1}}$

The energy stored by a capacitor is given by the equation,

$U=\dfrac{1}{2} C V^{2}$