System of Particles - Centre of Mass with Solved Examples for JEE

What is a system of particles? The term "system of particles" refers to a well-defined collection of many particles. We can also say that a system of particles consists of several particles that may or may not interact with, or be connected. They could be rigid body particles in translational motion. When particles interact, they exert force on one another. The internal force of the system refers to the forces of mutual interaction between the particles of the system.

Internal forces are always found in pairs of equal magnitude and opposite directions. In addition to internal forces, external forces may act on all or some of the particles. The term external force refers to a force acting on any one particle that is included in the system by another body outside the system.

Centre of Mass of a System of Particles

The centre of mass of a system of particles can be defined as the average position of all the parts of a system, or as the mean location of mass distribution in space. It is the point at which a force is applied, resulting in linear acceleration without angular acceleration. The motions of this point are congruent to the motions of a single particle, and the mass of this particle is equivalent to the sum of all individual particles of the system, by surrounding bodies or by the action of a field of force exerted directly on that particle.

For a system consisting of number of particles, masses are $m_{1}, m_{2}, m_{3}, \ldots \ldots m_{n}$ and their position vectors are respectively $r_{1}, r_{2}, r_{3}, r_{4} \ldots \ldots r_n$. With respect to the origin of the chosen frame of reference, rcm is the position vector of centre of mass and with respect to the origin, is:

$\overrightarrow{r_{c m}}=\dfrac{m_{1} \vec{r}_{1}+m_{2} \vec{r}_{2}++m_{3} \vec{r}_{3}+\ldots \ldots \ldots+m_{n} \vec{r}_{n}}{m_{1}+m_{2}++m_{3}+\ldots \ldots \ldots+m_{n}}$

Here,

If, M is the total mass of the system,

$M=m_{1}+m_{2}+m_{3} \ldots \ldots \ldots \ldots+m_{n}$

Then,

$\overrightarrow{r_{c m}}=\dfrac{m_{1} \overrightarrow{r_{1}}+m_{2} \vec{r}_{2}+m_{3} \vec{r}_{3}+\ldots \ldots \ldots \ldots{ }_{n} \vec{r}_{n}}{M}$

$M \overrightarrow{r_{c m}}=m_{1} \vec{r}_{1}+m_{2} \vec{r}_{2}+m_{3} \overrightarrow{r_{3}}+\ldots \ldots \ldots \ldots m_{n} \vec{r}_{n}$ …….(1)

Let us suppose an instant of a system consisting of a large number of particles

$m_{1}, m_{2}, m_{3}, \ldots \ldots . m_{n}$ and their positions vectors from chosen reference frame with respect to the origin is as follows: $\vec{r}_{1}, \vec{r}_{2}, \vec{r}_{3}, \vec{r}_{4} \ldots \ldots \vec{r}_{n}$

The position vectors will change as the time passes, which defines that the system is in motion. Particles having masses: $m_{1}, m_{2}, m_{3}, \ldots \ldots . m_{n}$

located at the position with position vectors as $\vec{r}_{1}, \vec{r}_{2}, \vec{r}_{3}, \vec{r}_{4} \ldots \vec{r}_{n} $ and are moving with velocity having the velocity vectors $\overrightarrow{v_{1}}+\overrightarrow{v_{2}}+\overrightarrow{v_{3}}+\ldots \ldots \ldots \ldots \overrightarrow{v_{n}}$

We will have the centre of mass of the system located at $r_{cm}$ moving with velocity $V_{cm}$ as;

$M \overrightarrow{V_{c m}}=m_{1} \overrightarrow{v_{1}}+m_{2} \overrightarrow{v_{2}}+m_{3} \overrightarrow{v_{3}}+\ldots \ldots \ldots m_{n} \vec{v}_{n}$…….(2)

The translational motion of the entire system is represented by the mass centre motion. The total linear momentum of all particles must equal the total linear momentum of the system due to translational motion of the centre of mass (or mass centre). We can also write the above eq (1) and (2) as follows:

$M \overrightarrow{r_{c m}}=\sum m_{i} \vec{r}_{i}$ ……(3)

$M \overrightarrow{V_{c m}}=\sum m \vec{V}_{i}$……(4)

From the above eq (3) the location of centre of mass of system of particles or discrete particles can be written as follows:

$\vec{r}_{c m}=\frac{\sum m_{i} \vec{r}_{i}}{M}$ ……. (5)

In the Cartesian coordinate system, the position vector of centre of mass in terms of position components can be written as:

$\begin{align} &X_{c m}=\dfrac{\sum m_{i} x_{i}}{M} \\ &Y_{c m}=\dfrac{\sum m_{i} y_{i}}{M} \\ &Z_{c m}=\dfrac{\sum m_{i} z_{i}}{M} \end{align}$

Linear Momentum of System of Particles

For a single particle the linear momentum is written as follows:

$p = mv$

From Newton’s second law, we have the force for a single particle, given by:

$F=\dfrac{dp}{d t}$

Here, F represents the force of the particle. For ‘ n ‘ number of particles, the total linear momentum of a system of particles is written as:

$P=p_{1}+p_{2}+\ldots \ldots+p_{n} $ ……(6)

When we have momentum for a system, it can be written as;

$m_{1} v_{1}+m_{2} v_{2}+\ldots \ldots \ldots .+m_{n} v_{n}$

Also, we know that the velocity of the centre of mass can be written as follows:

$V=\dfrac{\sum m_{i} \vec{V}_{i}}{M}$

$M \overrightarrow{V_{c m}}=\sum m_{i} \vec{V}_{i}$ ………(7)

So, comparing the equations (6) and (7), we get:

P=MV

As a result, the total linear momentum of a particle system is equal to the product of the system's total mass and the velocity of its centre of mass.

By differentiating the above equation with respect to time, we get:

$\dfrac{d P}{d t}=M \dfrac{d V}{d t}=M A$

Here , $MA$ is the external force and $\dfrac{dV}{dt}$ is the acceleration of the centre of mass. So,

$\dfrac{d P}{d t}=F_{ext}$

This equation is simply Newton's second law applied to a particle system.

If the total external force acting on the system is zero i.e, $F_{ext} = 0$ then,

$\dfrac{d P}{d t}=0$

This implies that P is a constant. When the total force acting on a particle's system equals zero, the total linear momentum of the system is constant or conserved. This is simply the law of total linear momentum conservation in a particle system. This explains the dynamics of a system of particles.

Solved Examples on System of Particles

Example 1: A uniform steel rod of 5 m in length is bent in a 90 degree angle at its mid point. Determine the position of the centre of mass from the corners at the bent.

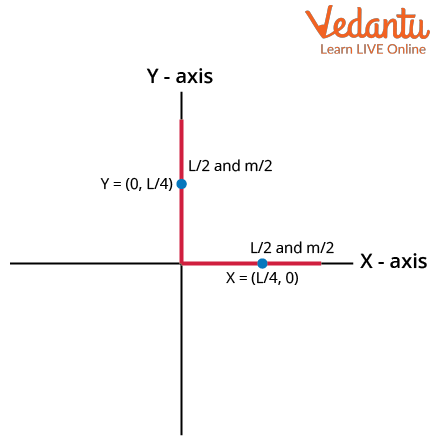
Image: Question Diagram

Solution:

X and Y-coordinate of centre of mass can be determined as follows:

$\begin{align} &X=\dfrac{m_{1} x_{1}+m_{2} x_{2}}{m_{1}+m_{2}} \\ &Y=\dfrac{m_{1} y_{1}+m_{2} y_{2}}{m_{1}+m_{2}} \end{align}$

Substituting the values from the figure given, we will have:

$\begin{align} &X=\dfrac{\dfrac{m}{2} \times \dfrac{L}{4}+\dfrac{m}{2} \times 0}{\dfrac{m}{2}+\dfrac{m}{2}}=\dfrac{\dfrac{m}{2} \times \dfrac{L}{4}}{m} \\ &X=\dfrac{L}{8} \\ &Y=\dfrac{\dfrac{m}{2} \times 0+\dfrac{m}{2} \times \dfrac{L}{4}}{\dfrac{m}{2}+\dfrac{m}{2}}=\dfrac{\dfrac{m}{2} \times \dfrac{L}{4}}{m} \\ &Y=\dfrac{L}{8} \end{align}$

From the figure we can write the position vector from the corner as $\dfrac{L}{8}(i+j)$.

So, the magnitude of the position vector from the corner will be:

$\begin{align} & \Rightarrow \sqrt{\left(\dfrac{L}{8}\right)^{2}+\left(\dfrac{L}{8}\right)^{2}} \\ & \Rightarrow \left(\dfrac{L}{8}\right) \sqrt{2} \end{align}$

Hence, the position of the centre of mass from the corners at the bent is $\dfrac{L}{8}(i+j)$ and its magnitude is $\left(\dfrac{L}{8}\right) \sqrt{2}$.

Example 2: Two point masses 8 kg and 4 kg are at 5 m and 10 m from the origin on X-axis. Locate the position of the centre of mass of the two point masses from the origin.

Solution:

Let us take, m1 = 8 kg and m2 = 4 kg

To find centre of mass from the origin:

The point masses are at positions as given in the question which is $x_1 = 5\,m, x_2 = 10\,m$ from the origin along the X axis.

The centre of mass Xcm can be obtained using the equation:

$\begin{align} X_{c m} &=\dfrac{\sum m_{i} x_{i}}{M} \\ X_{c m} &=\dfrac{8 \times 5+4 \times 10}{8+4} \\ X_{c m} &=6.66 m \end{align}$

Hence, the position of the centre of mass of the two point masses from the origin is 6.66 m.

Conclusion

In short, a system of particles is a well-defined collection of many particles that may or may not interact with or be connected. They could be rigid body particles in translational motion. When particles interact, they exert force on one another. We talked about the system of particles, the system of particles’ centre of mass and the system of particles momentum. As a result, this article assists us in clearly understanding the topic. Thus, the article discusses the concepts of system particles thoroughly.