Question

What is produced in a rigid reflecting plane for displacement waves?
A) Beats
B) Node and antinode
C) Antinode
D) Node

Answer 1

Hint: When waves are propagating in the medium with time, it produces displacement on both sides of their direction of propagation. The maximum displacement of waves is called the amplitude. A vibrating rope tied toward one side will create a standing wave, the wave train, subsequent to showing up at the fixed finish of the rope, will be pondered back and superimposed itself as another train of waves in a similar plane. Due to obstruction between the two waves, the resultant abundance of the two waves will be the total of their individual amplitudes.

Complete step by step solution:
We present a figure of displacement wave in which consistently there are positions (N) along the rope, called nodes, at which there is no development by any means. There the two wave trains are consistently in resistance. On either side of a node is a vibrating antinode (A). The antinodes substitute toward uprooting with the goal that the rope at any moment takes after a diagram of the numerical capacity called the sine function.

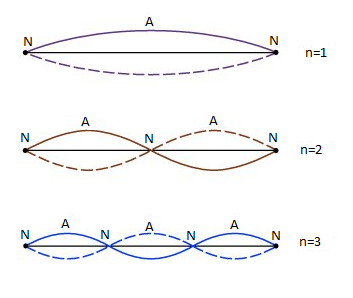
Hence it is clear that when a displacement wave incident at a rigid reflecting surface, makes Nodes and Antinodes. The mode of vibration in the waves depends on the number of nodes and antinodes formed.

Note: The best example of understanding of nodes and antinodes is open and closed organ pipes. Tied string is also an example of understanding nodes and antinodes. The expression of displacement/stationary wave in numeral form is –
\[y = 2a\cos( 2\pi x/\lambda) \sin (2\pi t/T)\]
Where \[x = \]displacement of wave in x-direction
             \[\lambda = \] Wavelength of wave
              \[t = \] Instant time
              \[T = \] Time period of wave