
Radius of the largest circle which passes through the focus of the parabola ${{y}^{2}}=4x $ and contained in it, is
(a). 8
(b). 4
(c). 2
(d). 5
Answer
141k+ views
Hint: To find the radius of the largest circle which passes through the focus of the parabola and is contained in the parabola, we will find the centre of the parabola by making the general equation of circle pass through the focus of the parabola and solve it in terms of radius of the circle.
Complete step by step answer:
We have the equation of parabola as ${{y}^{2}}=4x $.
We want to find the radius of the largest circle which passes through the focus of the parabola.
We know the focus of the parabola of the form \[{{y}^{2}}=4ax\] is \[(a,0)\] .
Thus, the focus of the parabola \[{{y}^{2}}=4x\] is \[(1,0)\] .
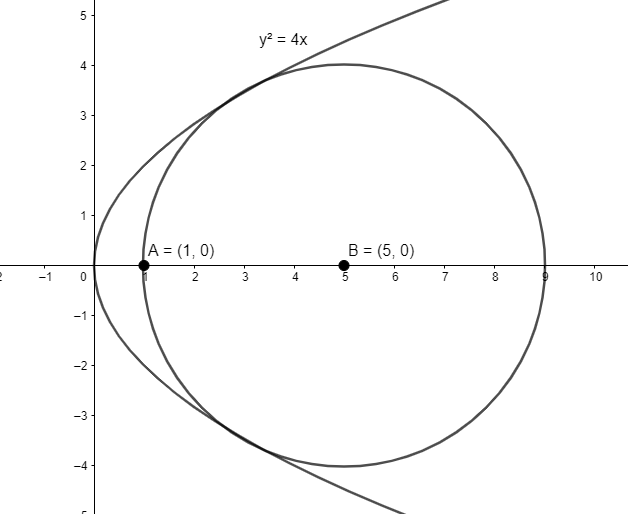
As we want the largest circle contained in parabola \[{{y}^{2}}=4x\] and the circle passes through focus of parabola, the centre of the circle lies on \[x\] -axis.
Let’s assume that the centre of the circle is \[(h,0)\] .
Let the radius of the circle be \[r\] .
As the circle passes through \[(1,0)\] , we have \[h=1+r\] . \[(1)\]
We know the equation of circle passing through the point \[(h,k)\] is of the form \[{{(x-h)}^{2}}+{{(y-k)}^{2}}={{r}^{2}}\] .
So, the equation of our circle is \[{{(x-h)}^{2}}+{{y}^{2}}={{r}^{2}}\]
Substituting the value of \[h\] from equation \[(1)\] , we get \[{{(x-1-r)}^{2}}+{{y}^{2}}={{r}^{2}}\] .
\[\Rightarrow {{x}^{2}}+{{r}^{2}}+1-2x-2xr+2r+{{y}^{2}}={{r}^{2}}\]
\[\Rightarrow {{x}^{2}}+{{y}^{2}}-2x-2xr+1+2r=0\]
But, we know \[{{y}^{2}}=4x\] . Substituting this in the above equation, we get \[{{x}^{2}}+4x-2x-2xr+1+2r=0\] .
\[\Rightarrow {{x}^{2}}+2x-2xr+1+2r=0\] \[(2)\]
The above quadratic equation will have equal roots because the circle intersects the parabola.
We know that when the quadratic equation of the form \[a{{x}^{2}}+bx+c=0\] has equal roots, we have \[{{b}^{2}}-4ac=0\] .
Substituting \[b=2-2r,c=1+2r\] from equation \[(2)\] to the above formula, we get \[{{(2-2r)}^{2}}=4(1+2r)\] .
On further solving this equation, we get
\[\begin{align}
& \Rightarrow 4+4{{r}^{2}}-8r=4+8r \\
& \Rightarrow 4{{r}^{2}}-16r=0 \\
& \Rightarrow 4r(r-4)=0 \\
& \Rightarrow r=0,r=4 \\
\end{align}\]
But the radius of the circle can’t be 0, so, the required answer is \[r=4\] .
Hence, the correct answer is 4.
Note: We can’t solve the question without the fact that the circle passes through the focus of the parabola and touches the parabola at some point. Also, one must know that a circle with 0 radius is just a point.
Complete step by step answer:
We have the equation of parabola as ${{y}^{2}}=4x $.
We want to find the radius of the largest circle which passes through the focus of the parabola.
We know the focus of the parabola of the form \[{{y}^{2}}=4ax\] is \[(a,0)\] .
Thus, the focus of the parabola \[{{y}^{2}}=4x\] is \[(1,0)\] .

As we want the largest circle contained in parabola \[{{y}^{2}}=4x\] and the circle passes through focus of parabola, the centre of the circle lies on \[x\] -axis.
Let’s assume that the centre of the circle is \[(h,0)\] .
Let the radius of the circle be \[r\] .
As the circle passes through \[(1,0)\] , we have \[h=1+r\] . \[(1)\]
We know the equation of circle passing through the point \[(h,k)\] is of the form \[{{(x-h)}^{2}}+{{(y-k)}^{2}}={{r}^{2}}\] .
So, the equation of our circle is \[{{(x-h)}^{2}}+{{y}^{2}}={{r}^{2}}\]
Substituting the value of \[h\] from equation \[(1)\] , we get \[{{(x-1-r)}^{2}}+{{y}^{2}}={{r}^{2}}\] .
\[\Rightarrow {{x}^{2}}+{{r}^{2}}+1-2x-2xr+2r+{{y}^{2}}={{r}^{2}}\]
\[\Rightarrow {{x}^{2}}+{{y}^{2}}-2x-2xr+1+2r=0\]
But, we know \[{{y}^{2}}=4x\] . Substituting this in the above equation, we get \[{{x}^{2}}+4x-2x-2xr+1+2r=0\] .
\[\Rightarrow {{x}^{2}}+2x-2xr+1+2r=0\] \[(2)\]
The above quadratic equation will have equal roots because the circle intersects the parabola.
We know that when the quadratic equation of the form \[a{{x}^{2}}+bx+c=0\] has equal roots, we have \[{{b}^{2}}-4ac=0\] .
Substituting \[b=2-2r,c=1+2r\] from equation \[(2)\] to the above formula, we get \[{{(2-2r)}^{2}}=4(1+2r)\] .
On further solving this equation, we get
\[\begin{align}
& \Rightarrow 4+4{{r}^{2}}-8r=4+8r \\
& \Rightarrow 4{{r}^{2}}-16r=0 \\
& \Rightarrow 4r(r-4)=0 \\
& \Rightarrow r=0,r=4 \\
\end{align}\]
But the radius of the circle can’t be 0, so, the required answer is \[r=4\] .
Hence, the correct answer is 4.
Note: We can’t solve the question without the fact that the circle passes through the focus of the parabola and touches the parabola at some point. Also, one must know that a circle with 0 radius is just a point.
Recently Updated Pages
Difference Between Mutually Exclusive and Independent Events

Difference Between Area and Volume

JEE Main Participating Colleges 2024 - A Complete List of Top Colleges

JEE Main Maths Paper Pattern 2025 – Marking, Sections & Tips

Sign up for JEE Main 2025 Live Classes - Vedantu

JEE Main 2025 Helpline Numbers - Center Contact, Phone Number, Address

Trending doubts
JEE Main 2025 Session 2: Application Form (Out), Exam Dates (Released), Eligibility, & More

JEE Main Exam Marking Scheme: Detailed Breakdown of Marks and Negative Marking

JEE Main 2025: Derivation of Equation of Trajectory in Physics

Electric Field Due to Uniformly Charged Ring for JEE Main 2025 - Formula and Derivation

Learn About Angle Of Deviation In Prism: JEE Main Physics 2025

Degree of Dissociation and Its Formula With Solved Example for JEE

Other Pages
JEE Advanced Marks vs Ranks 2025: Understanding Category-wise Qualifying Marks and Previous Year Cut-offs

JEE Advanced Weightage 2025 Chapter-Wise for Physics, Maths and Chemistry

NCERT Solutions for Class 11 Maths Chapter 6 Permutations and Combinations

JEE Main 2025: Conversion of Galvanometer Into Ammeter And Voltmeter in Physics

Electron Gain Enthalpy and Electron Affinity for JEE

Electrical Field of Charged Spherical Shell - JEE




