
The graph between the time period and the length of the simple pendulum is a ____________.
A) straight line
B) curve
C) ellipse
D) parabola
Answer
140.4k+ views
Hint: The time period of a simple pendulum refers to the time taken to complete one oscillation by the bob. It depends only on the length of the pendulum and is found to be proportional to the square root of the length of the pendulum.
Formula Used:
The time period of a simple pendulum of length
Complete step by step answer:
Step 1: Describe how the time period of the simple pendulum varies with its length.
A simple pendulum constitutes a bob attached to one end of an inextensible string of negligible mass and length
The time period of a simple pendulum is given by,
where
Equation (1) can also be expressed as,
On squaring both sides we get,
Here, the acceleration due to gravity
So, the equation (2) can be expressed as
The equation
Therefore, the graph between the time period and length of a simple pendulum must be a parabola as shown below:
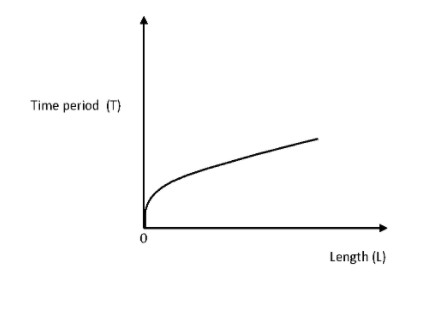
Note: One oscillation of the simple pendulum corresponds to one complete to and fro motion of the bob. The bob, initially at rest, is raised to the left to induce oscillation. It will then move to the extreme position on the right side and then swing to the left to return to its starting point. This constitutes one oscillation. This is repeated. The time period of the pendulum is independent of the amplitude of oscillation. For a constant length, no matter how far the extreme positions of the bob are the period of oscillation remains the same.
Formula Used:
The time period of a simple pendulum of length
Complete step by step answer:
Step 1: Describe how the time period of the simple pendulum varies with its length.
A simple pendulum constitutes a bob attached to one end of an inextensible string of negligible mass and length
The time period of a simple pendulum is given by,
where
Equation (1) can also be expressed as,
On squaring both sides we get,
Here, the acceleration due to gravity
So, the equation (2) can be expressed as
The equation
Therefore, the graph between the time period and length of a simple pendulum must be a parabola as shown below:

Note: One oscillation of the simple pendulum corresponds to one complete to and fro motion of the bob. The bob, initially at rest, is raised to the left to induce oscillation. It will then move to the extreme position on the right side and then swing to the left to return to its starting point. This constitutes one oscillation. This is repeated. The time period of the pendulum is independent of the amplitude of oscillation. For a constant length, no matter how far the extreme positions of the bob are the period of oscillation remains the same.
Latest Vedantu courses for you
Grade 10 | MAHARASHTRABOARD | SCHOOL | English
Vedantu 10 Maharashtra Pro Lite (2025-26)
School Full course for MAHARASHTRABOARD students
₹33,300 per year
EMI starts from ₹2,775 per month
Recently Updated Pages
Difference Between Circuit Switching and Packet Switching

Difference Between Mass and Weight

JEE Main Participating Colleges 2024 - A Complete List of Top Colleges

JEE Main Maths Paper Pattern 2025 – Marking, Sections & Tips

Sign up for JEE Main 2025 Live Classes - Vedantu

JEE Main 2025 Helpline Numbers - Center Contact, Phone Number, Address

Trending doubts
JEE Main 2025 Session 2: Application Form (Out), Exam Dates (Released), Eligibility, & More

JEE Main 2025: Derivation of Equation of Trajectory in Physics

JEE Main Exam Marking Scheme: Detailed Breakdown of Marks and Negative Marking

Learn About Angle Of Deviation In Prism: JEE Main Physics 2025

Electric Field Due to Uniformly Charged Ring for JEE Main 2025 - Formula and Derivation

JEE Main 2025: Conversion of Galvanometer Into Ammeter And Voltmeter in Physics

Other Pages
Units and Measurements Class 11 Notes: CBSE Physics Chapter 1

JEE Advanced Marks vs Ranks 2025: Understanding Category-wise Qualifying Marks and Previous Year Cut-offs

NCERT Solutions for Class 11 Physics Chapter 1 Units and Measurements

Motion in a Straight Line Class 11 Notes: CBSE Physics Chapter 2

Important Questions for CBSE Class 11 Physics Chapter 1 - Units and Measurement

NCERT Solutions for Class 11 Physics Chapter 2 Motion In A Straight Line




