
The slopes of isothermal and adiabatic curves are related as:
A) Isothermal curve slope = Adiabatic curve slope
B) Isothermal curve slope = $\gamma \times $adiabatic slope
C) Adiabatic curve slope = $\gamma \times $isothermal slope
D) Adiabatic curve slope = $\dfrac{1}{2} \times $isothermal curve slope
Answer
444.7k+ views
Hint: Before we understand about these processes, it is important to understand the significance of the term slope. The slope represents the steepness of the curve, which is represented by the ratio of how high the curve is moving to the width of the curve. Hence, if we draw a tangent at the curve, the slope is given by tan of the angle made by the tangent with the horizontal.
The slope of the tangent drawn to a curve is equal to the derivative of the curve at the point where it touches the curve. Thus,
$Slope = \tan \theta = \dfrac{{dy}}{{dx}}$
Complete step by step answer:
Let us understand the meaning of the isothermal and adiabatic process in a thermodynamic system.
Isothermal process is a process that takes place in the system under constant temperature. The equation that represents an isothermal process is –
$PV = C$
where P = pressure and V = volume and C = constant
Adiabatic process is a process which takes place with zero heat transfer from the system or surroundings. The equation that represents an adiabatic process is –
$P{V^\gamma } = C$
where C = constant and $\gamma $ is the ratio of specific heats calculated at constant pressure and constant volume.
$\gamma = \dfrac{{{C_p}}}{{{C_v}}}$
Plotting these two processes on a pressure v/s volume graph, we obtain as follows:
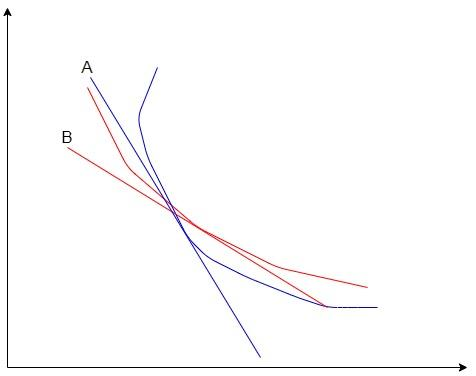
The red curve represents the isothermal process and the blue curve represents the adiabatic process. A and B are the slopes of the isothermal and adiabatic curves respectively. We can see from the graph, that the slope of adiabatic is higher than that of the isothermal process.Let us prove that.
If we differentiate the equation of the isothermal process, we obtain the slope of the line B in the above graph.
$PV = C$
Differentiating with respect to V,
$V\dfrac{{dP}}{{dV}} + P\dfrac{{dV}}{{dV}} = 0$
$ \Rightarrow V\dfrac{{dP}}{{dV}} = - P\dfrac{{dV}}{{dV}}$
$ \Rightarrow \dfrac{{dP}}{{dV}} = - \dfrac{P}{V}$
Hence, the slope of line A = $ - \dfrac{P}{V}$
Similarly, differentiating the equation for adiabatic process, we get the slope of line B.
$P{V^\gamma } = C$
Differentiating with respect to V,
$P\dfrac{{d\left( {{V^\gamma }} \right)}}{{dV}} + {V^\gamma }\dfrac{{dP}}{{dV}} = 0$
$ \Rightarrow {V^\gamma }\dfrac{{dP}}{{dV}} = - P\dfrac{{d\left( {{V^\gamma }} \right)}}{{dV}}$
$ \Rightarrow {V^\gamma }\dfrac{{dP}}{{dV}} = - P\gamma {V^{\gamma - 1}}$
\[\Rightarrow \dfrac{{dP}}{{dV}} = \dfrac{{ - P\gamma {V^{\gamma - 1}}}}{{{V^\gamma }}} = - P\gamma {V^{\gamma - 1 - \gamma }} = - P\gamma {V^{ - 1}}\]
\[\therefore \dfrac{{dP}}{{dV}} = - \gamma \dfrac{P}{V}\]
Comparing the slopes, we see that the slope of the adiabatic curve is $\gamma $ times the slope of the isothermal curve.
Hence, the correct option is Option C.
Note: The value $\gamma $ is referred to as the adiabatic index. If nothing is given, the value of adiabatic index for normal conditions of air should be taken as 1.4 approximately, while solving problems in adiabatic processes.
The slope of the tangent drawn to a curve is equal to the derivative of the curve at the point where it touches the curve. Thus,
$Slope = \tan \theta = \dfrac{{dy}}{{dx}}$
Complete step by step answer:
Let us understand the meaning of the isothermal and adiabatic process in a thermodynamic system.
Isothermal process is a process that takes place in the system under constant temperature. The equation that represents an isothermal process is –
$PV = C$
where P = pressure and V = volume and C = constant
Adiabatic process is a process which takes place with zero heat transfer from the system or surroundings. The equation that represents an adiabatic process is –
$P{V^\gamma } = C$
where C = constant and $\gamma $ is the ratio of specific heats calculated at constant pressure and constant volume.
$\gamma = \dfrac{{{C_p}}}{{{C_v}}}$
Plotting these two processes on a pressure v/s volume graph, we obtain as follows:

The red curve represents the isothermal process and the blue curve represents the adiabatic process. A and B are the slopes of the isothermal and adiabatic curves respectively. We can see from the graph, that the slope of adiabatic is higher than that of the isothermal process.Let us prove that.
If we differentiate the equation of the isothermal process, we obtain the slope of the line B in the above graph.
$PV = C$
Differentiating with respect to V,
$V\dfrac{{dP}}{{dV}} + P\dfrac{{dV}}{{dV}} = 0$
$ \Rightarrow V\dfrac{{dP}}{{dV}} = - P\dfrac{{dV}}{{dV}}$
$ \Rightarrow \dfrac{{dP}}{{dV}} = - \dfrac{P}{V}$
Hence, the slope of line A = $ - \dfrac{P}{V}$
Similarly, differentiating the equation for adiabatic process, we get the slope of line B.
$P{V^\gamma } = C$
Differentiating with respect to V,
$P\dfrac{{d\left( {{V^\gamma }} \right)}}{{dV}} + {V^\gamma }\dfrac{{dP}}{{dV}} = 0$
$ \Rightarrow {V^\gamma }\dfrac{{dP}}{{dV}} = - P\dfrac{{d\left( {{V^\gamma }} \right)}}{{dV}}$
$ \Rightarrow {V^\gamma }\dfrac{{dP}}{{dV}} = - P\gamma {V^{\gamma - 1}}$
\[\Rightarrow \dfrac{{dP}}{{dV}} = \dfrac{{ - P\gamma {V^{\gamma - 1}}}}{{{V^\gamma }}} = - P\gamma {V^{\gamma - 1 - \gamma }} = - P\gamma {V^{ - 1}}\]
\[\therefore \dfrac{{dP}}{{dV}} = - \gamma \dfrac{P}{V}\]
Comparing the slopes, we see that the slope of the adiabatic curve is $\gamma $ times the slope of the isothermal curve.
Hence, the correct option is Option C.
Note: The value $\gamma $ is referred to as the adiabatic index. If nothing is given, the value of adiabatic index for normal conditions of air should be taken as 1.4 approximately, while solving problems in adiabatic processes.
Recently Updated Pages
How to find Oxidation Number - Important Concepts for JEE

How Electromagnetic Waves are Formed - Important Concepts for JEE

Electrical Resistance - Important Concepts and Tips for JEE

Average Atomic Mass - Important Concepts and Tips for JEE

Chemical Equation - Important Concepts and Tips for JEE

Concept of CP and CV of Gas - Important Concepts and Tips for JEE

Trending doubts
JEE Main 2025 Session 2: Application Form (Out), Exam Dates (Released), Eligibility, & More

JEE Main Exam Marking Scheme: Detailed Breakdown of Marks and Negative Marking

JEE Main 2025: Derivation of Equation of Trajectory in Physics

Electric Field Due to Uniformly Charged Ring for JEE Main 2025 - Formula and Derivation

JEE Main Participating Colleges 2024 - A Complete List of Top Colleges

Degree of Dissociation and Its Formula With Solved Example for JEE

Other Pages
JEE Advanced Marks vs Ranks 2025: Understanding Category-wise Qualifying Marks and Previous Year Cut-offs

JEE Advanced 2025: Dates, Registration, Syllabus, Eligibility Criteria and More

Units and Measurements Class 11 Notes: CBSE Physics Chapter 1

NCERT Solutions for Class 11 Physics Chapter 1 Units and Measurements

Motion in a Straight Line Class 11 Notes: CBSE Physics Chapter 2

JEE Advanced Weightage 2025 Chapter-Wise for Physics, Maths and Chemistry




