




Let’s Understand More About the Addition of Integers
Integers are whole numbers that can be positive, negative, and zero. Adding an integer needs a specific set of rules. The addition of integers rules is important to understand as they have to be used while adding and subtracting integers rules. We have one set of common rules which hold good for the addition and subtraction of integers.
To understand adding integers rules, let’s jump right in!
Introduction to Addition of Integers
Integers are defined as numbers including negative numbers (numbers with a ‘-’ sign), positive numbers, and zero. Only whole numbers (0,1,2,3,....) come under integers. Fractions, decimals, and mixed fractions do not fall under integers as they do not fall under whole numbers.
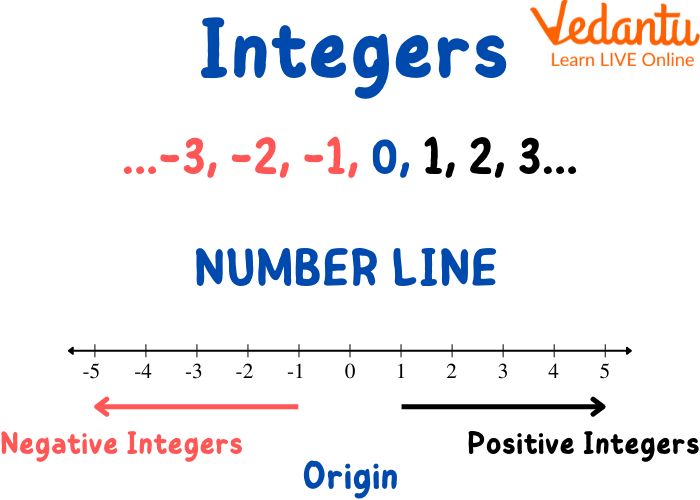
Number Line
Integer Rules
We can perform various mathematical operations using integers but adding and subtracting integers rules will be discussed in this article. Firstly adding integers rules will be discussed and by using those rules the concept of adding integers will get easy for you.

Rules for the Addition of Integers
Rules for Adding Integers
There are special rules for adding integers (adding integers rules). As integers have symbols along with the value of the number, it is important to follow these rules while adding.
For simplicity purposes and to understand the core concepts better, we are going to consider the addition of 2 digits having ‘+’ or ‘-’ signs.
How to Add Integers
Case 1- When Both the Digits are Positive
When the 2 numbers to be added are both positive, we add both the digits and assign their symbol as positive.
For example, 3 + 4 = 7
Remember that we usually use positive digits and thus it is not always necessary to mention ‘+’ before a positive number.
We add positive digits 3 and 4 to obtain a positive sum 7
Addition of 2 Positive Integers
Case 2- When Both the Digits are Negative
When the 2 numbers to be added are both negative, we add both the digits and assign their symbol as negative.
For example, (-3) + (-4) = (-7)
Remember that it is important to mention ‘-’ before a negative number.
We add negative digits 3 and 4 to obtain a negative sum which is (-7)
Remember the following phrase
“While adding the same signs, keep the signs!”
Case 3- When the Bigger Number has a Positive Sign
When the bigger number of the 2 digits added is positive, we subtract the smaller number from the bigger number and assign the symbol to be positive
For example, (-3) + 4 = 1
We are subtracting 4-3 which is 1 and assigning it the symbol of the bigger number which is positive, our answer as +1
Addition and Subtraction of Integers
Case 4- When the Bigger Number has a Negative Sign
When the bigger number of the 2 digits added is negative, we subtract the smaller number from the bigger number and assign its symbol to be negative
For example, 3 + (-4) = (-1)
We are simply subtracting 4-3 which is 1 and assigning it the symbol of the bigger number which is negative, our answer as (-1)
Remember the following phrase
“While adding opposite signs, subtract and keep the sign of the bigger number!”

Adding Negative Integer to Positive Integer
Solved Examples
Q1. Find the sum of (+14) + (-19)
Ans: Here both the numbers have different signs and a more significant number has a negative sign
So we subtract 19 - 14 = 5
We assign a ‘-’ sign as the bigger number is negative.
The answer is -5
Q2. Find the sum of (-18) + (-13)
Ans: Here both the numbers have the same negative sign
So we add 18 + 13 = 31
We assign a ‘-’ sign as both numbers are negative.
The answer is -31
Q3. Find the sum of (-10) + (+16)
Ans: Here both the numbers have different signs and the more significant number has a positive sign
So we subtract 16 - 10 = 6
We assign a ‘+’ sign as the bigger number is positive.
The answer is +6
Q4. Find the sum of (+13) + (+7)
Ans: Here both the numbers have the same positive sign
So we add 13 + 7 = 20
We assign a ‘+’ sign as both numbers are positive.
The answer is 20
Q5. Find the sum of (-5) + (-8)
Ans: Here both the numbers have the same negative sign
So we add 5 + 8 = 13
We assign a ‘-’ sign as both numbers are negative.
The answer is -13
Practice Questions
Q1. Find the sum of 72 + 34.
Ans: 106
Q2. Find the sum of (-56) + 28.
Ans: (-28)
Q3. Find the sum of 89 + (-37).
Ans: 52
Q4. Find the sum of (-91) + (-15).
Ans: -106
Q5. Find the sum of 43 + 23.
Ans: 66
Summary
The addition of integers has certain rules based on the signs and values of the integers. To summarize how to add integers, we have 4 cases. When both the numbers to be added are positive or negative, the numbers are added and their signs are kept the same as the number added. When a bigger number added is positive, we subtract the given numbers and keep the positive signs to the sum obtained. When a bigger number added is negative, we subtract the given numbers and keep the negative sign to the sum obtained.
FAQs on Addition of Integers Rules
1. Why are there specific sets of rules for the addition of integers?
Integers have 2 factors to deal with when we consider each digit. Each integer has a value and a sign. These specific sets of rules tell us how to add integers in a correct manner considering their signs and their values. So it is important to apply the above rules while adding or subtracting integers.
Initially, these rules might seem time-consuming, but with a bit of practice applying these rules become easier.
2. What are the applications of integers in real life?
Temperature measurement is the use of integers that is the most practical. The temperature's positive and negative characteristics help us understand, evaluate, and gauge temperature changes. In addition to detecting temperature, banks employ integers to calculate credits and debits.
3. Which jobs use integers?
Example of a Job Using Integers
Science Careers. Even while some scientists work with integers, they also make up a significant fraction of all careers.
Jobs in business. In business, integers are also used in computer programming, sports, and other fields.











